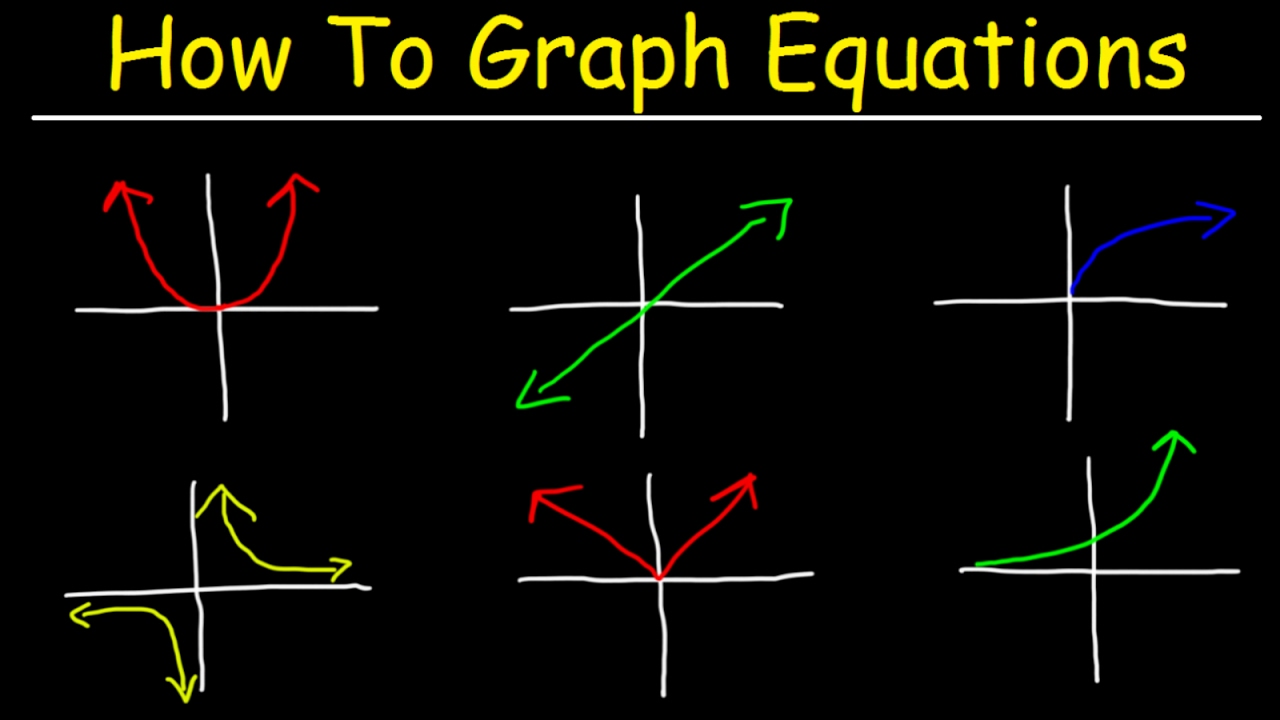
Calculus AB Homework Day 1 - Review 1: Functions

Michelle Krummel

27 Aug 201724:59

EducationalLearning

32 Likes 10 Comments

TLDRThis educational video script offers a comprehensive review of various function types, including linear, quadratic, power, absolute value, logarithmic, and trigonometric functions. It delves into their forms, equations, and graphical representations, providing step-by-step methods to identify and solve for specific parameters using given points. The script also covers how to determine the domain and range of functions, making it an invaluable resource for students looking to strengthen their understanding of algebraic concepts.

Takeaways

📚 The video provides a comprehensive review of different types of functions, including linear, quadratic, power, absolute value, and more.
🔍 It explains how to identify the function family that matches a given equation, such as recognizing a linear function in point-slope form or a quadratic in standard form.
📈 The video introduces the concept of the floor function, which rounds down to the nearest integer, and contrasts it with the ceiling function, which rounds up.
📊 The logistic function is highlighted for its use in modeling population growth with a carrying capacity, and the inverse function is also discussed.
📉 The script covers the constant function, polynomial functions, and the sine function, which can be referred to as a trigonometric or sinusoidal function.
🔢 The process of solving an exponential function using given points on a graph is demonstrated, emphasizing the use of substitution or linear combination methods.
📐 For quadratic functions, the video shows how to write the equation in vertex form and factored form, using the vertex and x-intercepts to determine the coefficients.
📘 The absolute value function is explored, with the vertex form of the equation and how to use additional points to find the unknown coefficient.
📙 The natural logarithm function is identified by its key points and the base is determined by the y-intercept.
📚 The video script also covers the tangent function, identifying it by its period and vertical asymptotes, and explains how to write its equation.
🎶 For trigonometric functions, the amplitude, period, and phase shift are discussed to derive the correct sine or cosine equation.
📌 The rational function is analyzed by identifying horizontal and vertical asymptotes, which help in determining the factors of the numerator and denominator.
📏 Lastly, the script examines the square root or radical function, focusing on transformations such as shifts and stretches to find the correct equation.

Q & A

What is the general form of a linear function in point-slope form?
-The general form of a linear function in point-slope form is y - y1 = m(x - x1), where (x1, y1) is a point on the line and m is the slope of the line.
How can you identify the y-intercept of a quadratic function in standard form?
-In the standard form of a quadratic function, ax^2 + bx + c, the y-intercept is the constant term 'c'.
What is the floor function and how does it relate to rounding numbers?
-The floor function is a mathematical function that rounds a real number down to the nearest integer less than or equal to the number itself. For example, the floor of 3.2 is 3.
What is a logistic function used for and what does it model?
-A logistic function is used for modeling population growth in situations where there is a carrying capacity for the population, meaning growth is limited by certain constraints.
What is an inverse function and how does it differ from a linear function?
-An inverse function is a function that 'reverses' another function, meaning that applying the inverse function to the result of the original function returns the original input. It is different from a linear function, which has a constant rate of change and is represented by a straight line in a graph.
What is the difference between a constant function and a polynomial function?
-A constant function is a special type of function where the output (y-value) does not change regardless of the input (x-value). A polynomial function, on the other hand, is a function involving a finite sum of terms, each term being a product of a power of the variable and a coefficient.
How can you determine the base of a logarithmic function from a given graph?
-If a logarithmic function has a point (a, 1) on its graph, where 'a' is the x-value, it suggests that the base of the logarithmic function is 'a', because log base 'a' of 'a' equals 1.
What is the domain and range of an exponential function of the form y = a * b^x?
-The domain of an exponential function is all real numbers, as it is defined for any real value of x. The range, however, is all real numbers greater than 0, since exponential functions never produce negative or zero outputs.
How do you write a quadratic equation in factored form given the vertex and x-intercepts?
-Given the vertex and x-intercepts, a quadratic equation in factored form is written as y = a(x - r)(x - s), where 'a' is a constant, and 'r' and 's' are the x-intercepts.
What is the general form of an absolute value function and how can you determine its vertex?
-The general form of an absolute value function is y = a |x - h| + k, where 'a' is a constant, 'h' is the x-coordinate of the vertex, and 'k' is the y-coordinate of the vertex.
How can you identify the amplitude and period of a sine function from its graph?
-The amplitude of a sine function is the distance from the sinusoidal axis to the maximum point on the curve. The period can be determined by measuring the distance from one maximum to the next maximum, which for a basic sine function is 2π. If the graph has a different period, it indicates a transformation of the basic sine function.
What is the domain and range of a rational function, and how can you determine them from its graph?
-The domain of a rational function is all real numbers except for the values of x that make the denominator zero, as these points create vertical asymptotes. The range is all real numbers except for the value at the horizontal asymptote, if one exists. These can be determined from the graph by identifying the x-values where the function is undefined and the y-value where the horizontal asymptote lies.
How can you determine the equation of a radical function from its graph?
-By identifying key points on the graph, such as the point where the function crosses the y-axis and any shifts from the parent function, you can determine the equation of a radical function. For example, if the graph is a shifted square root function, the equation might be y = a√(x - h) + k, where 'a' is a stretch factor, 'h' is the horizontal shift, and 'k' is the vertical shift.

Outlines

00:00

📚 Introduction to Function Families

This paragraph introduces various types of functions, starting with linear functions in point-slope form and moving on to quadratic functions in standard form. It also covers power functions, the greatest integer (floor) function, and the ceiling function. The paragraph then delves into less common functions such as the logistic function for modeling population growth with carrying capacity, the inverse function, constant function, polynomial functions in standard form, and sine functions as trigonometric or sinusoidal functions. It also touches on linear equations in slope-intercept form and absolute value functions with vertices, rational functions with polynomials in the numerator and denominator, radical functions, and ends with a mention of logarithmic functions.

05:01

🔍 Solving Exponential and Quadratic Equations

The second paragraph focuses on solving exponential and quadratic equations using given points on a graph. For the exponential function, the method involves substituting the coordinates of two points into the general exponential form to create a system of equations, which can be solved using substitution or linear combination, with a preference for the latter. The solution yields the base 'a' and exponent 'B' of the exponential function. For the quadratic function, the vertex and x-intercepts are used to write the equation in factored form, with the constant 'a' being determined by substituting another point into the equation. The domain and range for both functions are discussed, with the exponential function having a domain of all real numbers and a range of all positive real numbers, while the quadratic function's domain is all real numbers with a range from the vertex value to infinity.

10:05

📉 Analyzing Absolute Value and Logarithmic Functions

This paragraph discusses the process of identifying and solving absolute value and logarithmic functions from a graph. The absolute value function is characterized by its vertex and the behavior of the graph, leading to the determination of the equation's parameters 'a', 'H', and 'K'. The logarithmic function's analysis involves recognizing the parent logarithmic function's characteristics, such as the vertical asymptote and x-intercept, and using a point on the graph to deduce the base of the logarithm. The domain and range for the absolute value function are described, with the domain being all real numbers and the range from negative infinity to a specific value, while the logarithmic function's domain is all positive real numbers and the range is all real numbers.

15:08

📈 Trigonometric and Tangent Function Analysis

The fourth paragraph examines the characteristics of trigonometric functions, specifically the tangent function, and how to determine its equation from a graph. Key points such as the period, vertical asymptotes, and the function's behavior at specific angles are used to infer the equation. The domain is described as all real numbers except for the points of discontinuity, which are the vertical asymptotes. The range of the tangent function is all real numbers. Additionally, the paragraph touches on the sine function, discussing how to identify the amplitude, period, and phase shift from the graph to form the sine equation.

20:12

📊 Rational Functions and Radical Transformations

The final paragraph discusses rational functions, identifying horizontal and vertical asymptotes to deduce the form of the equation. The process involves guessing and checking with plotted points to confirm the equation. The domain and range of the rational function are explained, with the domain excluding the points of vertical asymptotes and the range excluding the value of the horizontal asymptote. The paragraph also covers the identification of a radical function, likely a square root function, by observing shifts and potential stretches on the graph. The equation is confirmed by checking it against given points, and the domain and range are described accordingly.

Mindmap

Keywords

💡Linear function

A linear function is a mathematical relationship between two variables that forms a straight line when graphed. In the video, the linear function is introduced in point-slope form, which is a way to express the function by using a point on the line and the slope, denoted as 'a'. It is fundamental in understanding the basic behavior of a straight line, and the script provides the example of the equation in point-slope form to illustrate this concept.

💡Quadratic function

A quadratic function is a polynomial of degree two, and its graph is a parabola. The video script describes it in standard form, which is useful for identifying the y-intercept and other characteristics such as the vertex and direction of the parabola. The quadratic function is a key concept in the video, as it is used to demonstrate how to identify and write equations for parabolas given certain points and intercepts.

💡Power function

A power function is a function of the form f(x) = x^n, where 'n' is a positive integer. In the video, the script assumes 'B' to be a positive integer and introduces the power function as a type of function that can be identified by its specific form. The power function is relevant to the video's theme as it represents a family of functions that are fundamental in algebra and calculus.

💡Greatest integer function

Also known as the floor function, the greatest integer function rounds a given number down to the nearest whole number. The video script uses the term to describe a function that 'rounds down,' providing the example of rounding 3.2 down to 3. This concept is essential for understanding how certain mathematical operations can be applied to non-integer values to produce integer results.

💡Logistic function

The logistic function is an S-shaped curve often used to model growth processes that are subject to carrying capacity constraints, such as population growth. The video introduces the logistic function as a type of function that is not commonly encountered but is important for specific applications, such as in biology and economics.

💡Inverse function

An inverse function is a function that 'reverses' another function, meaning that applying the inverse function to the result of the original function returns the original input. In the video, the inverse function is one of the function families discussed, and it is a fundamental concept in understanding the relationship between functions and their inverses.

💡Constant function

A constant function is a function that has the same output value for every input value. In the video, it is described as a special type of linear and polynomial function. The script uses the constant function to illustrate a function with a specific property, which is that its rate of change is zero, making it a horizontal line when graphed.

💡Polynomial function

A polynomial function is an expression involving a sum of powers in a single variable. The video script mentions polynomial functions in the context of standard form and other forms. Polynomial functions are central to the video's theme as they encompass a wide range of mathematical expressions and their graphical representations.

💡Trigonometric function

Trigonometric functions, such as sine and cosine, are functions of an angle in a right-angled triangle and have various applications in mathematics, physics, and engineering. The video script introduces the sine function as a type of trigonometric function, which is periodic and oscillates between -1 and 1. It is used to demonstrate the properties of sinusoidal functions.

💡Rational function

A rational function is a function that is the ratio of two polynomials. The video script describes a rational function with a horizontal asymptote at y = 0 and vertical asymptotes at x = -2 and x = 2. Rational functions are important in the video as they illustrate the behavior of functions with both horizontal and vertical asymptotes, which are key features in their graphs.

💡Exponential function

An exponential function is a function where the variable is in the exponent. The video script discusses solving an exponential equation given two points on its graph, illustrating how to determine the base and coefficient of the function. Exponential functions are relevant to the video's theme as they represent growth or decay processes and are fundamental in various scientific fields.

💡Logarithmic function

A logarithmic function is the inverse of an exponential function and is used to solve for the exponent when the base and result are known. The video script describes the natural logarithm function and its properties, such as the vertical asymptote at the y-axis and the x-intercept at (1,0). Logarithmic functions are essential in the video as they demonstrate the inverse relationship between exponential growth and decay.

💡Absolute value function

An absolute value function represents the distance of a number from zero on a number line, regardless of direction. The video script introduces an absolute value function with a vertex at (2,4) and explains how to determine the coefficient 'a' by using a point on the graph. Absolute value functions are important in the video as they show how to model situations where distance is considered without regard to direction.

💡Slope-intercept form

The slope-intercept form of a linear equation is y = mx + b, where 'm' is the slope and 'b' is the y-intercept. The video script explains that the slope-intercept form is a common way to write linear equations, emphasizing the importance of identifying the slope and y-intercept from the equation's coefficients. This concept is crucial for understanding the basic properties of linear relationships.

💡Piecewise function

A piecewise function is a function that is defined by multiple pieces, each with its own equation. The video script mentions a piecewise function as a type of function that can be represented by different mathematical expressions depending on the input value. Piecewise functions are relevant to the video's theme as they demonstrate how to model situations with different behaviors under different conditions.

Highlights

Introduction to a video tutorial on day 1 homework assignment focusing on function review.

Explanation of identifying function families from given general equations.

Discussion on linear functions and their representation in point-slope form.

Identification of a quadratic function in standard form and its y-intercept.

Introduction to power functions and their properties assuming B is a positive integer.

Description of the greatest integer or floor function and its rounding down property.

Explanation of the ceiling function as the opposite of the floor function, rounding up.

Introduction to logistic functions and their use in modeling population growth with carrying capacity.

Identification of inverse functions and their mathematical properties.

Explanation of constant functions as a special type of linear and polynomial function.

Introduction to polynomial functions in standard form and their characteristics.

Discussion on sine functions, also known as trigonometric or sinusoidal functions.

Explanation of linear equations in standard form and the importance of identifying coefficients.

Introduction to absolute value functions with a vertex and their graphical representation.

Explanation of rational functions involving polynomials and their properties.

Introduction to radical functions and their graphical characteristics.

Discussion on linear equations in slope-intercept form and their basic understanding.

Introduction to logarithmic functions and their basic properties and transformations.

Explanation of quadratic functions in factored form and how to derive them from given points.

Introduction to piecewise functions and their stepwise nature.

Explanation of exponential functions in vertex form and solving them using given points.

Introduction to reciprocal functions and their mathematical properties.

Solving an exponential equation using a system of equations derived from graph points.

Discussion on the domain and range of functions, specifically for exponential functions.

Introduction to the process of solving for the equation of a quadratic function given its vertex and x-intercepts.

Explanation of absolute value functions and solving for their equation using given points.

Introduction to logarithmic functions and verifying their equation using specific points.

Explanation of the domain and range of logarithmic functions and their restrictions.

Introduction to tangent functions and their key properties such as period and vertical asymptotes.

Discussion on the domain of tangent functions and how to express it with exceptions.

Introduction to trigonometric functions, specifically sine, and their equation formulation.

Explanation of rational functions with vertical and horizontal asymptotes and their equation derivation.

Introduction to radical functions, their shifts, and the formulation of their equations.

Final discussion on the domain and range of the derived radical function and its properties.

Transcripts

Browse More Related Video

Calculus AB Homework Day 2 - Review 2: Functions and Trig

PreCalculus Midterm Exam Review

How To Graph Equations - Linear, Quadratic, Cubic, Radical, & Rational Functions

Functions and Graphs | Precalculus

Ch. 2.7 Combining Functions

How To Graph Functions Using an Online Calculator

Related Tags

Math Education Function Families Linear Equations Quadratic Functions Absolute Value Logistic Growth Trigonometric Functions Rational Functions Polynomial Equations Algebra Tutorial Graph Analysis Homework Help Educational Content Math Solutions Function Graphs Slope Intercept Factored Form Vertex Form Domain Range