Lecture 16: 10 Numerical Problems on Degrees of Freedom/Mobility of Planar Mechanisms | Kutzback |
TLDRIn this informative video, Dr. V Jayakumar, a lecturer for mechanical engineering students, explains the concept of degrees of freedom in planar mechanisms using Kutzberg's criterion. The video is structured around solving 10 numerical problems, guiding viewers through the process of identifying the number of links, binary and ternary joints, and higher pairs in given mechanisms. By applying the Kutzberg formula, which calculates degrees of freedom as 3n - 2j - h (where n is the number of links, j is the number of lower pairs, and h is the number of higher pairs), the video demonstrates how to determine if a mechanism forms a structure, is statically indeterminate, or has constrained motion. The step-by-step approach and clear explanations make complex mechanical engineering concepts accessible to students, enhancing their understanding of the subject.
Takeaways
- 📚 Dr. V Jayakumar's channel focuses on creating lecture videos for mechanical engineering students.
- 🔍 The Kutzberg criterion is used to determine the degrees of freedom for planar mechanisms, which is calculated as (3n - 2l - h).
- 🔢 In the formula, n represents the number of links, l is the number of lower pairs (binary joints), and h is the number of higher pairs.
- 🏗️ If the degrees of freedom equals zero, the mechanism forms a structure.
- ⚙️ A negative degree of freedom indicates a statically indeterminate structure.
- 🚀 A positive degree of freedom implies the mechanism has constrained motion, requiring a certain number of inputs to operate.
- 📝 The video script provides a step-by-step approach to solving numerical problems related to degrees of freedom.
- 🔬 It's important to correctly identify the number of links, binary joints, and higher pairs in a mechanism to apply the Kutzberg criterion accurately.
- 🤔 The video encourages students to pause and attempt problems on their own before revealing the solutions.
- 📐 Examples in the script illustrate how to identify ternary links and distinguish them from binary and higher pairs.
- 📈 The script covers a range of problems, from simple to complex, to demonstrate the application of the Kutzberg criterion in various scenarios.
- 📚 The video concludes with an invitation for viewers to like, share, subscribe, and enable notifications for future content.
Q & A
What is the Kutzberg criterion used for?
-The Kutzberg criterion is used for determining the degrees of freedom of planar mechanisms. It helps to calculate how many independent motions a mechanism can have.
What does the Kutzberg criterion formula represent?
-The Kutzberg criterion formula is given as degrees of freedom = 3 * n - (2 * l - h) - 1, where n is the number of links, l is the number of lower pairs (binary joints), and h is the number of higher pairs (like line or point contacts).
What does it mean if the degrees of freedom of a mechanism is zero?
-If the degrees of freedom is zero, it means that the mechanism forms a structure, indicating that it does not have any independent motion.
What is the implication of a mechanism having a negative degree of freedom?
-A negative degree of freedom indicates that the mechanism is statically indeterminate, which means it has more constraints than necessary to maintain equilibrium.
What does a positive degree of freedom signify for a mechanism?
-A positive degree of freedom signifies that the mechanism has constrained motion, meaning it requires a certain number of inputs to achieve motion.
How is the number of links in a mechanism determined?
-The number of links in a mechanism is determined by counting all the distinct parts, including the fixed link, which is always numbered as link one.
What is a binary joint and how is it identified in a mechanism?
-A binary joint is a joint that connects two links. It is identified by looking for connections between two links in the mechanism, which are typically represented by circles in diagrams.
What is the significance of a ternary link in the context of the Kutzberg criterion?
-A ternary link is a single link that connects three other links. It is significant because it affects the count of binary joints, as one ternary link is equivalent to two binary joints in the Kutzberg criterion.
How are higher pairs (h) identified in a mechanism?
-Higher pairs are identified by looking for point or line contacts between two links. These are represented by higher pairs (h) in the Kutzberg criterion and affect the degrees of freedom calculation.
What is the process for solving the numerical problems presented in the script?
-The process involves identifying the number of links (n), counting the binary joints (l), identifying any ternary or higher pairs (h), and then substituting these values into the Kutzberg criterion formula to find the degrees of freedom.
What is the practical application of understanding degrees of freedom in mechanical engineering?
-Understanding degrees of freedom is crucial in mechanical engineering for designing mechanisms with the desired motion characteristics, such as in the creation of clamping mechanisms or other kinematic chains.
Outlines
📚 Introduction to Degrees of Freedom in Mechanical Engineering
Dr. V Jayakumar introduces a lecture series aimed at mechanical engineering students, focusing on the concept of degrees of freedom in planar mechanisms. He references a previous video on basic concepts and announces the intention to solve numerical problems using Kutzberg's criterion. The formula for degrees of freedom is presented as 3n - (1 + 2l - h), where n is the number of links, l is the number of lower pairs (binary joints), and h is the number of higher pairs (notches or cam joints). The implications of different degrees of freedom values on the mechanism's motion are explained, with structures, statically indeterminate structures, and mechanisms with constrained motion being discussed. The video encourages viewers to engage with the material by pausing to solve problems or solving along with the video.
🔍 Problem Solving: Determining Degrees of Freedom Using Kutzberg's Criterion
The video script delves into solving numerical problems to determine the degrees of freedom of various mechanisms using Kutzberg's criterion. Each problem involves identifying the number of links (n), binary joints (l), and higher pairs (h). The first problem involves a mechanism with seven links and eight binary joints, resulting in a degree of freedom of 2, indicating the need for two inputs for constrained motion. The second problem features a mechanism with six links, seven binary joints, and one ternary joint, which is equivalent to two binary joints, leading to a degree of freedom of 1, suggesting one input is needed for motion. The process is repeated for subsequent problems, with careful attention paid to identifying the correct number of links and joints, and the absence of higher pairs in most cases.
🛠️ Analyzing Planar Mechanisms for Degrees of Freedom
The script continues with the analysis of planar mechanisms, emphasizing the importance of correctly identifying the number of links and joints. It explains the process of counting binary and ternary joints and converting ternary joints into an equivalent number of binary joints for the calculation. The script also highlights the significance of recognizing higher pairs when present. Each problem is methodically solved, with the degrees of freedom calculated based on the given mechanism's characteristics. The results range from mechanisms that form structures with zero degrees of freedom to statically indeterminate structures with negative degrees of freedom, and mechanisms with constrained motion having positive degrees of freedom.
🔧 Applying Kutzberg's Criterion to Complex Mechanisms
The script provides a detailed walkthrough of applying Kutzberg's criterion to more complex mechanisms, including those with sliding joints and roller joints. It clarifies the difference between binary and ternary joints, and the importance of identifying higher pairs, such as point or line contacts, which are considered higher pairs. The video script methodically solves problems, demonstrating how to count the number of links and joints accurately, and how to convert ternary joints into binary equivalents. The degrees of freedom for each mechanism are calculated, revealing whether they form structures, are statically indeterminate, or have constrained motion.
🎓 Conclusion and Encouragement for Further Learning
The final paragraph of the script wraps up the video by summarizing the process of determining degrees of freedom in planar mechanisms using Kutzberg's criterion. It emphasizes the importance of understanding the concepts and encourages viewers to practice problem-solving. The script concludes with a call to action, inviting viewers to like, share, subscribe, and enable notifications for the channel to stay updated with the latest educational content. The video ends with a thank you note and a farewell message, signaling the end of the educational session.
Mindmap
Keywords
💡Degrees of Freedom
💡Kutzberg Criterion
💡Mechanical Engineering
💡Planar Mechanisms
💡Binary Joints
💡Ternary Link
💡Higher Pairs (HP)
💡Numerical Problems
💡Statically Indeterminate
💡Constrained Motion
💡Mechanism Structure
Highlights
Introduction to a lecture series by Dr. V Jayakumar aimed at mechanical engineering students.
Explanation of Kutzberg's criterion for determining degrees of freedom in planar mechanisms.
Degrees of freedom formula: F = 3(n - 1) - 2j - h, where n is the number of links, j is the number of lower pairs, and h is the number of higher pairs.
Interpretation of degrees of freedom results: zero for structure, negative for statically indeterminate, and positive for constrained motion.
Guidance on how to approach and solve numerical problems using Kutzberg's criterion.
Step-by-step process to determine the number of links in a mechanism.
Differentiation between binary and ternary joints in mechanisms.
Clarification on identifying higher pairs (h) in mechanisms.
Problem-solving approach for determining degrees of freedom for a mechanism with seven links.
Explanation of how to count binary joints correctly, including sliding joints.
Conversion of ternary joints into equivalent binary joints for calculation purposes.
Demonstration of how to handle mechanisms with higher pairs like roller joints.
Use of Kutzberg's criterion in solving 10 numerical problems throughout the video.
Practical application of degrees of freedom in designing clamping mechanisms.
Emphasis on the importance of correctly identifying the type of joints in mechanisms.
Invitation for viewers to pause and attempt problems before revealing solutions.
Encouragement to like, share, subscribe, and enable notifications for more educational content.
Transcripts
Browse More Related Video
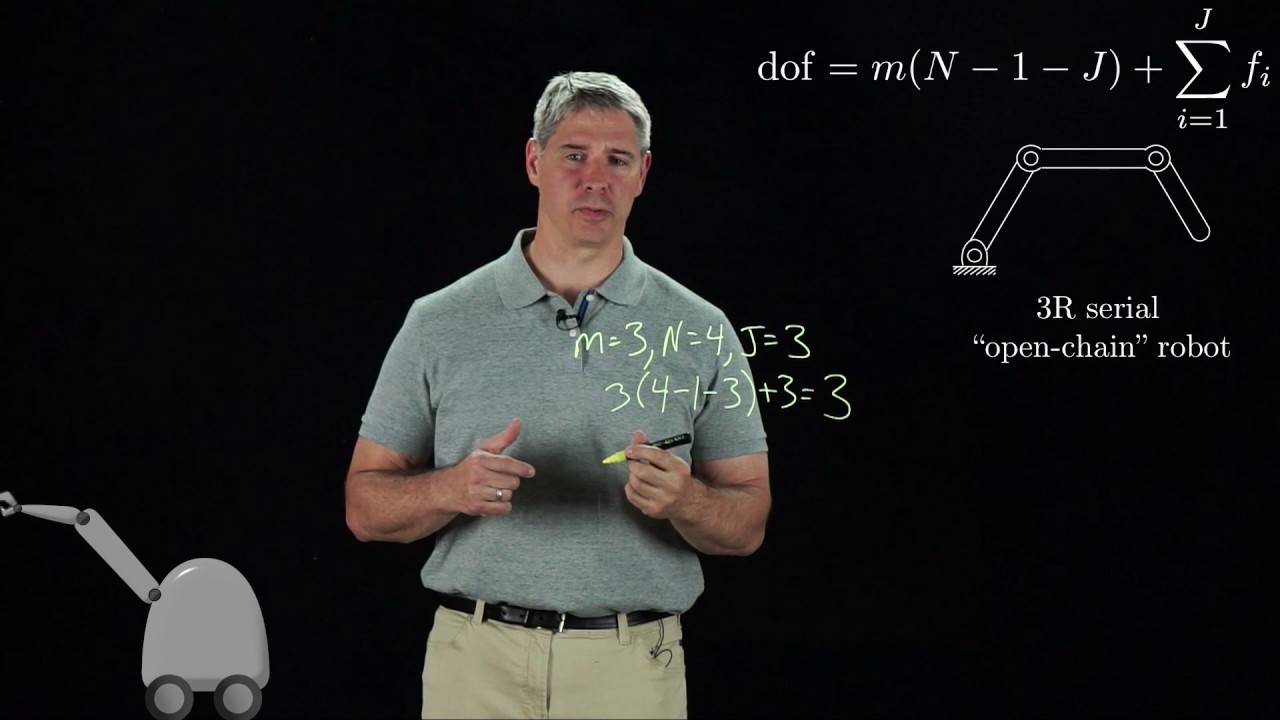
Modern Robotics, Chapter 2.2: Degrees of Freedom of a Robot

Degrees Of Freedom Explained | What is Degrees of freedom | Degrees of freedom in statistics

Understanding Degrees of Freedom

Understand prismatic joint and how it affects the degrees of freedom in a system
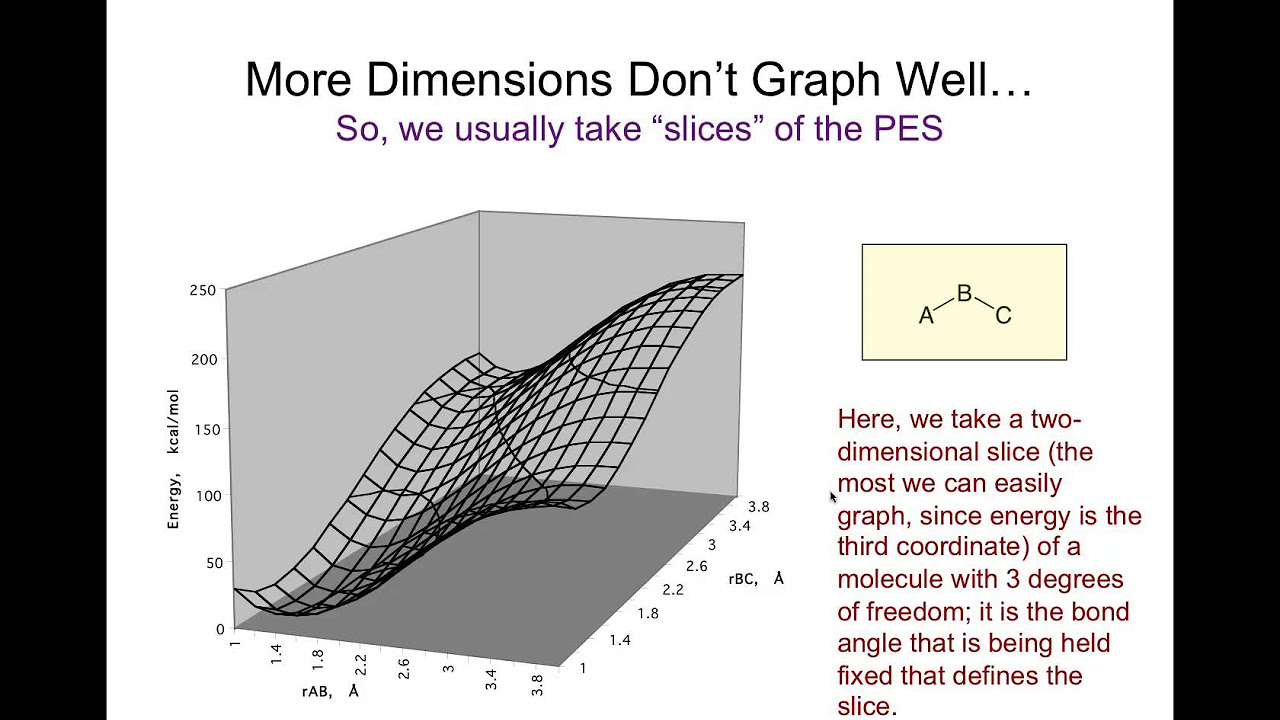
CompChem.02.01 The Potential Energy Surface — Preamble to the Basic Force Field Part I

Introduction to Degrees of Freedom
5.0 / 5 (0 votes)
Thanks for rating: