Evaluating Limits with L’Hopital’s Rule and the Second Fundamental Theorem of Calculus
TLDRThis educational video tutorial delves into solving limits using L'Hopital's Rule and the Second Fundamental Theorem of Calculus. The instructor methodically explores two example problems where limits yield a 0/0 form, necessitating the use of L'Hopital's Rule. In each example, derivatives of integrals are taken, substituting limits and simplifying to find the solutions. The first example demonstrates finding the limit of a ratio involving the integral of cosine and the sine function, resulting in a simple fraction. The second covers a more complex integral over a cubic function, concluding with a clear step-by-step derivation of the answer. This approachable explanation aims to demystify these calculus concepts for students.
Takeaways
- 📚 First, always attempt to evaluate limits by direct substitution, as it's the initial approach for every limit problem.
- ➗ When encountering an indeterminate form like 0/0, utilize L'Hôpital's rule to resolve the limit by taking the derivatives of the numerator and denominator.
- 🔄 Remember to differentiate between L'Hôpital's rule and the quotient rule; they are not the same and are used in different scenarios.
- 🧮 For the numerator, replace the variable of integration (T) with the upper bound (X) and multiply by the derivative of the upper bound.
- ∫ Apply the second fundamental theorem of calculus to find the derivative of the integral in the numerator when using L'Hôpital's rule.
- 📉 Evaluate the derivative of the denominator by differentiating the function without the integral.
- 🔢 Perform direct substitution again after differentiating, replacing the variable with the value it approaches.
- 🚫 Be cautious of integrals from the same starting point; the result will be zero, as seen in the first example.
- 🔁 In the second example, the integral is from a constant to a constant (1 to 1), which also results in zero.
- 📌 The second example demonstrates using the second fundamental theorem to find the derivative of the integral in the numerator.
- 🤓 Even though these types of limits are not very common, they may seem intimidating but can be managed with the right approach.
- ✨ The video aims to demystify limits involving L'Hôpital's rule and the second fundamental theorem, encouraging viewers to tackle them with confidence.
Q & A
What is the first step to evaluate a limit involving an integral?
-The first step is to try to evaluate by direct substitution, which is a common approach for every limit problem.
What does L'Hôpital's rule allow us to do when we encounter an indeterminate form such as 0/0?
-L'Hôpital's rule allows us to take the limit of the derivative of the numerator over the derivative of the denominator to resolve the indeterminate form.
What is the difference between L'Hôpital's rule and the quotient rule?
-L'Hôpital's rule is used to evaluate limits with indeterminate forms by taking derivatives, while the quotient rule is a method for finding the derivative of a quotient of two functions.
How do you find the derivative of the numerator when applying L'Hôpital's rule to the first limit in the script?
-You replace every instance of 'T' in the integrand with the upper bound 'X', and then multiply by the derivative of the upper bound, which is 1 in this case.
What is the integral result from 0 to 0 of cosine of 3t dt?
-The integral from 0 to 0 of any function, including cosine of 3t, is 0.
What is the final result of the first limit using L'Hôpital's rule?
-The final result of the first limit is 1/6, after taking the derivatives and applying direct substitution.
What is the second limit problem presented in the script?
-The second limit problem is the limit as X approaches 1 of the integral from 1 to X squared of (5t cube minus 3) dt over (X cube minus 1).
Why does direct substitution give 0 for both the numerator and denominator in the second limit problem?
-Direct substitution gives 0 because when X approaches 1, the upper and lower bounds of the integral become the same (1 to 1), resulting in an integral of 0.
How is the second fundamental theorem of calculus used to find the derivative of the numerator in the second limit?
-The second fundamental theorem is used by replacing every instance of 'T' in the integrand with the upper bound 'X squared', and then multiplying by the derivative of the upper bound.
What is the final result of the second limit using L'Hôpital's rule?
-The final result of the second limit is 4/3, after taking the derivatives, applying direct substitution, and simplifying the expression.
Why are limits involving L'Hôpital's rule and the second fundamental theorem considered somewhat intimidating?
-These types of limits can be intimidating because they involve a combination of advanced calculus concepts and techniques, and they are not encountered as frequently, which can make them seem more complex.
Outlines
📚 Applying L'Hôpital's Rule and the Second Fundamental Theorem of Calculus
The video begins with an introduction to evaluating limits involving L'Hôpital's rule and the second fundamental theorem of calculus. The first example focuses on the limit as X approaches zero of an integral from 0 to X of cosine of 3t DT divided by sine of 6x. Initially, direct substitution is attempted, which leads to an indeterminate form of 0/0. L'Hôpital's rule is then applied by taking the derivatives of the numerator and denominator. The derivative of the integral is found by replacing the variable T with the upper bound X and multiplying by the derivative of the upper bound. The final result is obtained by direct substitution, yielding a simplified answer of 1/6. The process is demonstrated step by step, emphasizing the importance of not confusing L'Hôpital's rule with the quotient rule.
🔢 Evaluating Another Limit Using Direct Substitution and L'Hôpital's Rule
The second example in the video deals with the limit as X approaches 1 of an integral from 1 to X squared of 5t cubed minus 3 DT divided by X cubed minus 1. Similar to the first example, direct substitution is attempted, but it results in an indeterminate form of 0/0. L'Hôpital's rule is then utilized, and the video demonstrates the process of finding the derivatives of the numerator and denominator. The second fundamental theorem of calculus is applied to find the derivative of the integral in the numerator. The upper bound X squared is used to replace T in the integrand, and the derivative of the upper bound is multiplied to find the derivative of the integral. The denominator's derivative is straightforward as it involves a simple power function. After finding the derivatives, direct substitution is performed again, this time with X replaced by 1, leading to a final answer of 4/3. The video concludes by emphasizing that while these types of limits may seem intimidating, they are manageable with a systematic approach.
Mindmap
Keywords
💡L'Hopital's Rule
💡Second Fundamental Theorem of Calculus
💡Direct Substitution
💡Indeterminate Form
💡Derivative
💡Integral
💡Cosine Function
💡Sine Function
💡Quotient Rule
💡Antiderivative
💡Exponential Function
Highlights
Introduction to limits involving L'Hôpital's rule and the second fundamental theorem of calculus.
Direct substitution is the first approach to evaluate limits, especially when the limit results in 0/0.
The integral from 0 to X of cosine of 3t DT over sine of 6x is the first limit problem discussed.
Substituting X in the integral results in an integral from 0 to 0, which simplifies to 0.
The limit as X approaches 0 of sine of 6X also results in 0, leading to an indeterminate form of 0/0.
L'Hôpital's rule is applied to resolve the indeterminate form by taking derivatives of the numerator and denominator.
Derivative of the numerator involves replacing T with X and multiplying by the derivative of the upper bound.
Derivative of the denominator is the product of the derivative of the argument and the derivative of the upper bound.
Direct substitution in the derivatives results in a simplified ratio of 1/6.
Second limit problem involves the integral from 1 to X squared of 5t cube minus 3 DT over X cube minus 1.
Direct substitution in the second limit also results in an integral from 1 to 1, simplifying to 0.
L'Hôpital's rule is again applied to the second limit, leading to derivatives of the numerator and denominator.
The second fundamental theorem of calculus is used to find the derivative of the numerator.
Derivative of the numerator is calculated by replacing T with X squared and multiplying by the derivative of the upper bound.
Derivative of the denominator is straightforward as it involves the derivative of a polynomial function.
Direct substitution in the second limit's derivatives results in a final answer of 4/3.
The video emphasizes that while these types of limits are not common, they are manageable and not as daunting as they appear.
The presenter hopes the viewers find the explanation helpful and wishes them good luck with similar problems.
Transcripts
Browse More Related Video

Introduction to l'Hôpital's rule | Derivative applications | Differential Calculus | Khan Academy

Calculus AB Homework 6.6: Fundamental Theorem of Calculus Part II

Example of Using L'Hopital's Rule Twice to Evaluate a Limit
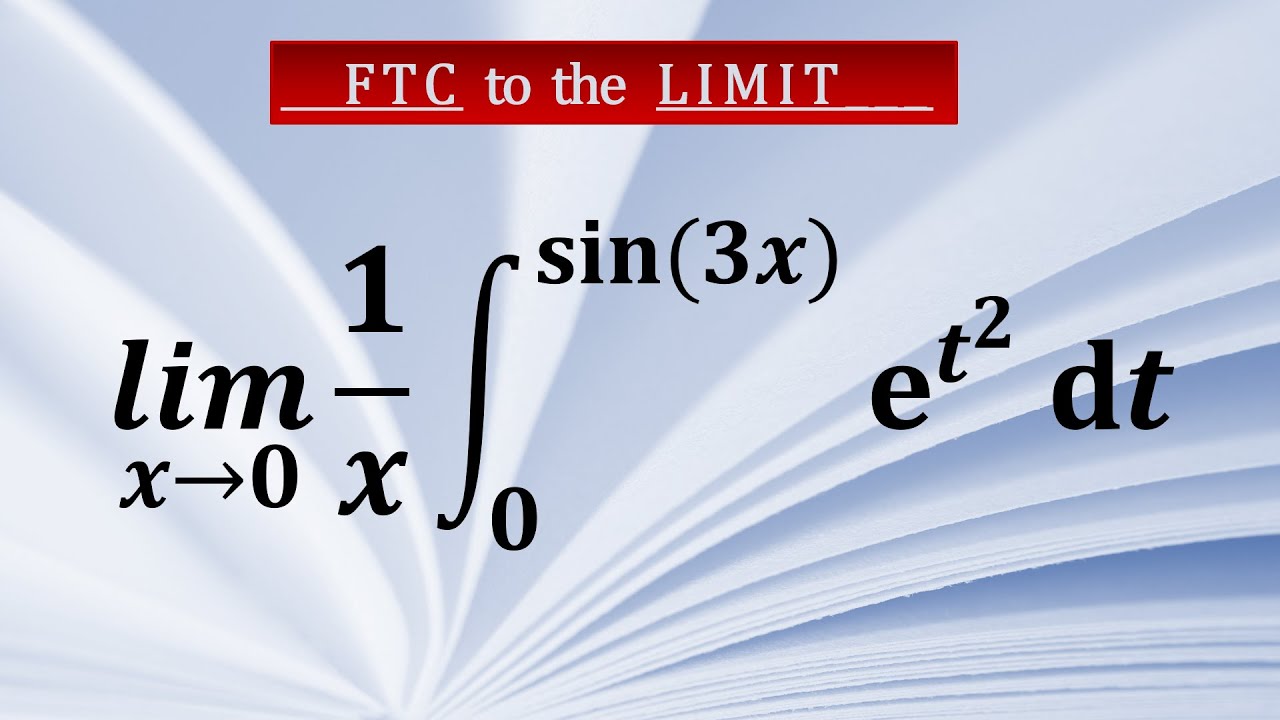
Limit problem with Fundamental Theorem of Calculus

Calculus: The Squeeze Theorem Full Tutorial

L'Hôpital's rule example 1 | Derivative applications | Differential Calculus | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: