Limit problem with Fundamental Theorem of Calculus
TLDRThis educational video tackles a complex limit problem involving integration, a concept typically introduced after understanding the fundamental theorem of calculus. The host demonstrates the process of finding the limit of 1 over X multiplied by an integral, highlighting the importance of direct substitution and the indeterminate form. The video dives into applying L'Hôpital's Rule, a technique for solving limits that result in 0/0 or ∞/∞, and concludes with the correct evaluation of the limit using the fundamental theorem of calculus, revealing the answer to be three. The host encourages viewers to engage with the content and continue their learning journey.
Takeaways
- 📚 The video discusses a limit problem involving integration, which is typically encountered after learning the fundamental theorem of calculus.
- 🔍 When faced with a limit problem where 'X' approaches a finite number, the first step is to attempt direct substitution into the function.
- ⚠️ Direct substitution in this case leads to an indeterminate form of '0/0', which means the limit cannot be taken directly and requires further manipulation.
- 📉 The script illustrates that dividing by zero is problematic, as it leads to an undefined expression, emphasizing the need for alternative approaches.
- 🧩 The video explains that when integrating from the same number to the same number, the result is always zero, a key concept from the fundamental theorem of calculus.
- 🔄 To resolve the indeterminate form, the script suggests rewriting the expression as a fraction and applying L'Hôpital's Rule, which involves taking the derivatives of the numerator and the denominator.
- 📐 L'Hôpital's Rule is applicable when the limit results in '0/0' or '∞/∞', and it involves differentiating the numerator and the denominator until a determinate limit can be found.
- 📈 The derivative of an integral from a constant to a function of 'X' can be found using the fundamental theorem of calculus, which simplifies to evaluating the function at the upper limit and multiplying by the derivative of the upper limit function.
- 🔑 The script demonstrates the application of the chain rule in finding the derivative of a composite function, which is crucial in applying L'Hôpital's Rule.
- 📝 After applying L'Hôpital's Rule and evaluating at 'X=0', the video concludes that the limit of the given expression is '3'.
- 🌟 The video encourages continuous learning and engagement with the material, urging viewers to like, share, subscribe, and comment to continue their educational journey.
Q & A
What is the main topic of the video?
-The main topic of the video is solving a limit problem involving integration, which is typically encountered after learning the fundamental theorem of calculus.
Why is the limit of 1 over X problematic when X approaches zero?
-The limit of 1 over X as X approaches zero is problematic because it results in a division by zero, which is undefined in standard arithmetic.
What is the first step suggested for solving limit problems where X approaches a finite number?
-The first step suggested is to plug in the value of X (in this case, zero) into the function to see what happens.
What is the result of plugging in X equals zero into the given function?
-Plugging in X equals zero results in an indeterminate form of infinity times zero, which cannot be directly evaluated.
Why is the integral from the same number to the same number always zero?
-According to the fundamental theorem of calculus, the integral of a function from a constant to the same constant is zero because it represents the net area under the curve, which is non-existent in this case.
What is the indeterminate form encountered in the video?
-The indeterminate form encountered is 0/0, which occurs when both the numerator and the denominator approach zero.
What rule is suggested to resolve the indeterminate form 0/0?
-L'Hôpital's Rule is suggested to resolve the indeterminate form 0/0 by taking the derivatives of the numerator and the denominator and then re-evaluating the limit.
What is L'Hôpital's Rule, and when is it applicable?
-L'Hôpital's Rule states that if the limit of the ratio of two functions results in an indeterminate form like 0/0 or ∞/∞, then the limit of that ratio is equal to the limit of the ratio of their derivatives, provided the latter limit exists.
How does the fundamental theorem of calculus help in finding the derivative of an integral?
-The fundamental theorem of calculus states that the derivative of an integral from a constant to a function of X is the function evaluated at the upper limit of integration, multiplied by the derivative of the upper limit function.
What is the final answer to the limit problem presented in the video?
-The final answer to the limit problem is three, which was obtained by applying L'Hôpital's Rule and evaluating the derivatives at X equals zero.
What is the significance of the video's closing message 'Don't Stop Learning'?
-The closing message 'Don't Stop Learning' is an encouragement for viewers to continue their educational journey, implying that learning is an ongoing process and should not be halted.
Outlines
📚 Introduction to the Limit Problem
The video begins with an introduction to a limit problem involving calculus concepts. The presenter explains that limits of this nature are typically encountered after learning integration and the fundamental theorem of calculus. The video aims to demonstrate how to approach a limit where the function involves an integral. The presenter emphasizes the importance of first attempting direct substitution for limits, which in this case leads to an indeterminate form of infinity times zero. This situation calls for the application of L'Hôpital's Rule, a technique for dealing with indeterminate forms by taking derivatives of the numerator and denominator.
🔍 Applying L'Hôpital's Rule to the Limit Problem
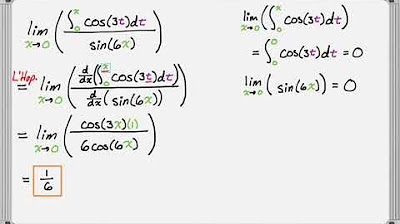
In this paragraph, the presenter dives deeper into the application of L'Hôpital's Rule to the given limit problem. The video explains that when direct substitution results in an indeterminate form of 0/0, taking the derivatives of the numerator and denominator can help resolve the limit. The presenter outlines the process of applying L'Hôpital's Rule, which involves differentiating the integral function with respect to its variable and evaluating the result at the point of interest, which in this case is as X approaches zero. The video also revisits the fundamental theorem of calculus to guide the differentiation process of the integral.
🎯 Solving the Limit Using Calculus Theorems
The final paragraph of the script concludes the limit problem by applying the fundamental theorem of calculus and L'Hôpital's Rule. The presenter calculates the derivative of the integral function and evaluates it at the given point. Using the chain rule, the derivative of the exponential function is found, and the result is multiplied by the derivative of the sine function, which is the argument of the exponential. After evaluating the derivatives at X equals zero, the presenter finds that the limit is three. The video ends with an encouragement to continue learning and a prompt for viewers to like, subscribe, and comment.
Mindmap
Keywords
💡Limit
💡Integration
💡Fundamental Theorem of Calculus
💡Direct Substitution
💡Indeterminate Form
💡L'Hôpital's Rule
💡Derivative
💡Chain Rule
💡Sine Function
💡Exponential Function
💡Integral Evaluation
Highlights
Introduction to a limit problem involving integration, which is typically encountered after learning the fundamental theorem of calculus.
The initial approach to evaluate the limit by direct substitution, which often leads to an indeterminate form when X approaches a finite number.
The concept that when integrating from the same number to the same number, the result is zero, regardless of the function.
Explanation of the indeterminate form '0/0' and the necessity to apply L'Hôpital's Rule to resolve it.
L'Hôpital's Rule is introduced as a method to take the derivative of the numerator and denominator to resolve indeterminate forms.
Demonstration of rewriting the limit problem into a form that allows the application of L'Hôpital's Rule.
The fundamental theorem of calculus is referenced to explain the derivative of an integral from a constant to a function of X.
Application of the chain rule in the context of differentiating the integral with respect to X.
The process of evaluating the derivative of the integral at the function's argument, which involves substituting the argument into the function.
The simplification of the derivative of the integral by applying the fundamental theorem of calculus and the chain rule.
Final evaluation of the limit after applying L'Hôpital's Rule, resulting in the answer of three.
Emphasis on the importance of learning and understanding calculus concepts to solve complex problems.
Encouragement for viewers to share, subscribe, and comment if they found the video educational.
Closing remarks with a motivational message to keep learning and living.
The video ends with a reminder to not stop learning and a sign-off.
Transcripts
Browse More Related Video

CALCULUS SPEEDRUN || Limits || Episode 1
Evaluating Limits with L’Hopital’s Rule and the Second Fundamental Theorem of Calculus
Understanding Limits and L'Hospital's Rule
Limits by rationalizing | Limits and continuity | AP Calculus AB | Khan Academy

Justifying our Answers on Improper Integrals
Limits Involving Sine and Cosine & The Squeeze Theorem!
5.0 / 5 (0 votes)
Thanks for rating: