Exam Walkthrough | Calc 1, Test 3 | Integration, FTC I/II, Optimization, u-subs, Graphing

Dr. Trefor Bazett

6 Nov 201834:28

EducationalLearning

32 Likes 10 Comments

TLDRThe video script is a comprehensive walkthrough of a calculus test from the University of Cincinnati. It begins with an explanation of the test's purpose, encouraging students to attempt the problems independently before checking the solutions in the video. The presenter methodically works through various calculus problems, starting with finding antiderivatives and applying initial conditions, proceeding to net change rate problems, and utilizing definite integrals to solve real-world scenarios like water flow in and out of a tank. The video also covers limit calculations using Riemann sums, the additive property of integrals, and the application of the fundamental theorem of calculus. It delves into more complex problems involving logarithmic differentiation, optimization in relation to constructing a cost-effective tank, and concludes with a detailed analysis of functions and their derivatives, including determining intervals of increase/decrease and concavity. The presenter emphasizes the importance of understanding and applying calculus concepts to a range of mathematical challenges.

Takeaways

📚 The video is a walkthrough of a calculus test from the University of Cincinnati, emphasizing the importance of attempting the test independently before watching the solutions.
🔑 The first problem involves finding an antiderivative of a given derivative function, with the added condition that F(0) = 3, leading to the determination of F(π).
💧 A net change problem is solved using definite integrals to calculate the change in water flow in and out of a tank over a two-minute period.
📈 The concept of Riemann sums is applied to approximate the area under a curve using right endpoints, which is crucial for understanding the limit of a sum as it approaches a definite integral.
🔍 The video covers the use of the additivity of domains property to break down a larger integral into the sum of smaller integrals, simplifying the calculation process.
🧮 An example of a Riemann sum using left endpoints is provided, illustrating how to calculate the sum for a given function over a specified interval.
📌 The Fundamental Theorem of Calculus is highlighted in solving an integral involving a derivative of an integral, showcasing the relationship between differentiation and integration.
🟡 The process of graphing functions using given information about their derivatives and second derivatives is demonstrated, helping to visualize the behavior of the functions.
📐 Optimization problems, such as minimizing the cost of constructing a tank with a given volume, are solved using calculus, emphasizing the practical applications of the subject.
🟠 Techniques for solving integrals using substitution are discussed, with a focus on identifying the appropriate substitution that simplifies the integral.
📉 The concept of concavity and its relation to the second derivative is explored, showing how to determine where a function is concave up or down and find inflection points.
📈 The video concludes with a graphing problem that synthesizes the information about a function, its derivative, and second derivative to plot a comprehensive graph.

Q & A

What is the main purpose of the video?
-The main purpose of the video is to guide viewers through an entire calculus test, allowing them to attempt the test on their own first and then use the video to verify their answers or understand specific problems.
What is the first problem in the calculus test about?
-The first problem involves finding the antiderivative of a given derivative function, with the additional condition that F(0) is equal to 3, to determine the value of F at pi.
How does the video approach the net change problem?
-The video approaches the net change problem by calculating the definite integral from 0 to 2, representing the first two minutes of water flow into and out of a tank, to find the net change during that period.
What is the significance of the right Riemann sum in the video?
-The right Riemann sum is used to approximate the definite integral by using the right endpoints of the intervals, which is important for understanding how to calculate areas under curves using different methods of Riemann sums.
How does the video handle the limit problem involving a sum?
-The video uses the properties of integrals and the concept of additivity of domains to break down the limit problem into smaller integrals that can be solved more easily.
What is the role of the Fundamental Theorem of Calculus in the video?
-The Fundamental Theorem of Calculus is used to convert differentiation problems into integration problems and vice versa, allowing for the simplification of complex problems by recognizing the relationship between functions and their antiderivatives.
How does the video address the Riemann sum problem?
-The video calculates the Riemann sum using left endpoints over three intervals, providing a step-by-step method to find the sum of the function values at the endpoints multiplied by the width of the intervals.
What is the strategy for solving the optimization problem involving a tank?
-The strategy involves setting up a cost function based on the surface area of the tank, using the volume constraint to express one variable in terms of another, and then finding the critical points to determine the minimum cost.
How does the video handle the graphing problem?
-The video uses the given components of the function, such as its derivative and second derivative, to determine the function's behavior, including its increasing/decreasing intervals, concavity, and asymptotes, to sketch the graph.
What is the importance of the second derivative in the video?
-The second derivative is used to determine the concavity of the function, which helps in understanding the shape of the graph and identifying inflection points.
How does the video ensure that the final answer is correct?
-The video ensures correctness by verifying the antiderivative matches the integrand when the limits of integration are plugged in, performing unit analysis, and using algebraic manipulations to simplify and check the solutions.

Outlines

00:00

📚 Calculus Test Walkthrough

This paragraph introduces a calculus test from the University of Cincinnati. The speaker encourages viewers to attempt the test independently before using the video as a resource to check answers. The focus is on solving the first problem, which involves finding an antiderivative with a specific constant. The process includes integrating the given derivative, applying the initial condition, and calculating the value of the antiderivative at a specific point.

05:03

💧 Net Change in Water Flow

The second paragraph deals with a net change problem involving water flow into and out of a tank. The inflow and outflow rates are given by mathematical expressions, and the task is to calculate the net change over the first two minutes. This involves setting up and solving a definite integral that represents the difference between inflow and outflow rates over time.

10:07

🔢 Riemann Sum and Logarithmic Rules

The third paragraph covers a Riemann sum problem, where the goal is to evaluate a definite integral using the right endpoint method over the interval from 0 to 2. The speaker also discusses the additivity of domains and logarithmic rules in integration, providing a step-by-step approach to solving the given problem.

15:09

📈 Accumulation Functions and Derivatives

The fourth paragraph introduces the concept of accumulation functions and their derivatives. The speaker explains how to interpret an accumulation function from a given graph and how to find its derivative, which is simply the integrand of the original function. The paragraph also covers how to determine the intervals where the function is increasing and the location of absolute maxima.

20:11

🧮 U-Substitution and Optimization

The fifth paragraph delves into integration techniques, specifically U-substitution. The speaker illustrates how to find antiderivatives of complex functions by choosing appropriate substitution variables. An optimization problem is also presented, involving the dimensions of a tank with a given volume and cost constraints, aiming to minimize the cost.

25:11

📉 Function Analysis and Graphing

The sixth paragraph focuses on analyzing and graphing functions based on their derivatives and second derivatives. The speaker discusses how to determine the intervals where the function is increasing or decreasing, as well as where it is concave up or down. The process of finding horizontal and vertical asymptotes is also covered, leading to a comprehensive graph of the function.

30:13

📊 Graphing Functions with Asymptotes

The seventh and final paragraph continues the graphing theme, emphasizing the importance of understanding the behavior of functions near asymptotes. The speaker provides a method for graphing functions by utilizing known points, critical numbers, and concavity to sketch an accurate representation of the function's graph.

Mindmap

Keywords

💡Calculus Test

A calculus test is an examination that assesses students' understanding of calculus concepts, including limits, derivatives, integrals, and series. In the video, the speaker is going through an entire calculus test, specifically test number three from the University of Cincinnati, to help students understand and verify their answers to various calculus problems.

💡Derivative

The derivative is a fundamental concept in calculus that represents the rate of change of a function with respect to its variable. In the context of the video, the speaker discusses finding the antiderivative of a given derivative function, which is crucial for solving the first problem presented on the test.

💡Antiderivative

An antiderivative, also known as an indefinite integral, is a function whose derivative is equal to the original function. The video demonstrates how to find the antiderivative of a given function, which is a common task in calculus courses, and uses the initial condition f(0) = 3 to determine the constant of integration.

💡Net Change

Net change refers to the overall difference after all additions and subtractions have been considered. In the video, the speaker addresses a net change problem involving water flow into and out of a tank, which is calculated using definite integrals to represent the total inflow and outflow over a given time period.

💡Definite Integral

A definite integral is a mathematical concept that calculates the area under a curve between two points on the x-axis. The video script includes a problem where the speaker uses definite integrals to find the net change of water in a tank over the first two minutes, illustrating how integrals can be applied to real-world scenarios.

💡Riemann Sum

A Riemann sum is a method for approximating the definite integral of a function. It involves summing the product of function values and interval widths at specific points within the interval. The video discusses using a right Riemann sum to approximate an integral, showcasing an alternative approach to calculating areas under a curve.

💡Fundamental Theorem of Calculus

The Fundamental Theorem of Calculus is a key theorem that links the concept of the definite integral to derivatives, stating that the definite integral of a derivative is equal to the original function. The speaker in the video refers to this theorem when discussing the relationship between an integral and its derivative, particularly in the context of solving a limit problem.

💡Chain Rule

The chain rule is a derivative rule used in calculus for functions that are composed of two or more functions. It allows for the derivative of the outer function to be found by multiplying the derivative of the inner function by the derivative of the outer function. The video script mentions using the chain rule when dealing with an integral where the upper limit is a function of x.

💡Optimization Problem

An optimization problem involves finding the maximum or minimum value of a function, often subject to constraints. In the video, the speaker presents an optimization problem related to minimizing the cost of constructing a tank with a given volume, which requires using calculus to find the critical points that yield the minimum cost.

💡Concavity

Concavity refers to the curvature of a function, indicating whether the function curves upward (concave up) or downward (concave down). The video discusses determining the concavity of a function by analyzing the sign of its second derivative, which helps in graphing the function and understanding its behavior.

💡Critical Points

Critical points are points on a function where the derivative is either zero or undefined. They often correspond to local maxima, local minima, or points of inflection. The video script includes an example of finding critical points by setting the derivative of a cost function equal to zero and then using the second derivative to verify that these points correspond to a minimum.

Highlights

The video presents an entire calculus test from the University of Cincinnati, offering a unique opportunity for students to practice.

The presenter encourages viewers to attempt the test independently before watching the solutions, fostering active learning.

The first problem involves finding an antiderivative with a specific constant, showcasing the application of integration techniques.

The video demonstrates the use of the Fundamental Theorem of Calculus to solve for definite integrals, a key concept in calculus.

A net change problem is tackled, illustrating how to calculate the change in quantity over time using integrals.

The concept of right Riemann sums is introduced to approximate definite integrals, demonstrating a method for numerical integration.

The video covers the use of logarithmic rules in integration, highlighting the importance of recognizing and applying these rules correctly.

The application of the additivity of domains property is shown, allowing for the breakdown of complex integrals into simpler parts.

A Riemann sum problem using left endpoints is solved, emphasizing the importance of selecting the correct points for summation.

The video explains how to find the antiderivative of a function involving a quotient, using algebraic manipulation to simplify the process.

An example of using substitution in integration is provided, with a focus on choosing an appropriate substitution variable.

The concept of accumulation functions and their derivatives is explored, linking integrals to their rate of change.

The video discusses how to find the critical points of a function to determine its maximum or minimum values.

An optimization problem is solved, applying calculus to real-world scenarios such as minimizing construction costs.

The use of the second derivative test to verify the nature of critical points is demonstrated, ensuring a thorough analysis.

The video concludes with a graphing problem, integrating various components of calculus to visualize function behavior.

The importance of understanding the domain, intercepts, and asymptotes when graphing functions is emphasized.

The presenter uses a step-by-step approach to graphing, providing a clear method for visualizing complex functions.

Transcripts

Browse More Related Video

Calculus AB Homework 6.5: Accumulated Change and Average Value

AP Calculus BC 2003 Multiple Choice (calculator) - Questions 76 - 92

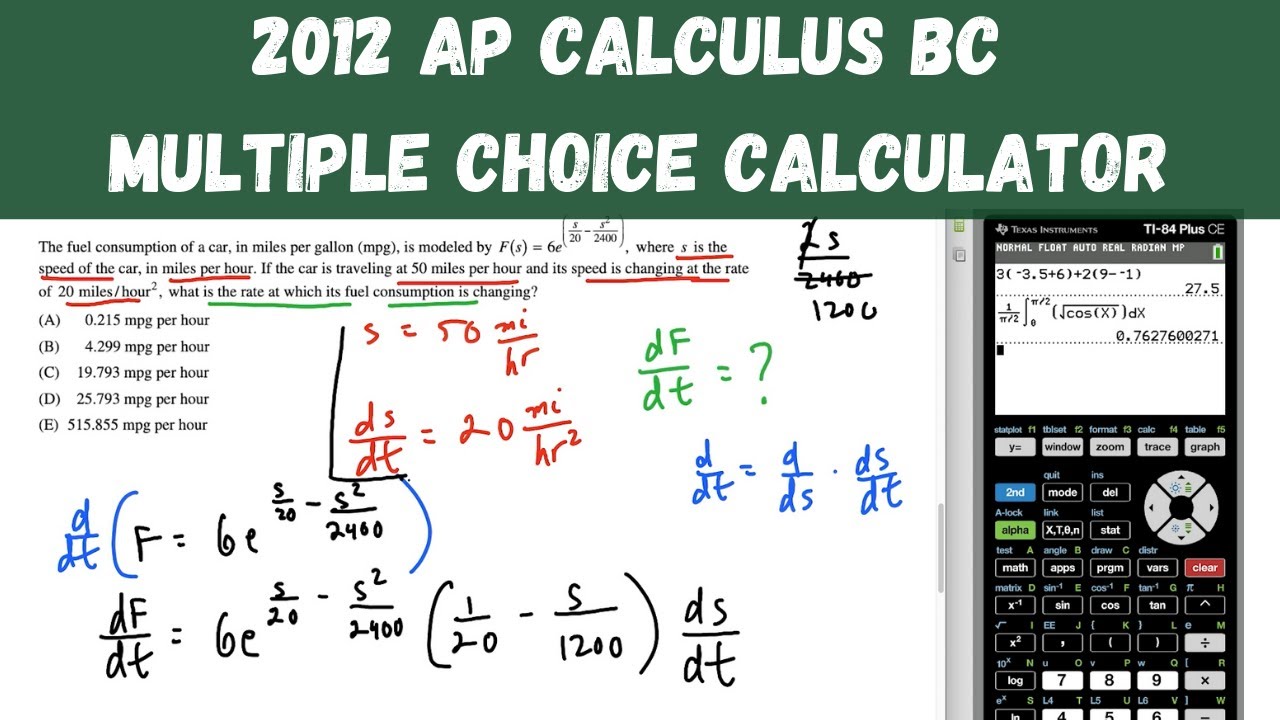
AP Calculus BC Practice Exam 2012 - Calculator Multiple Choice questions 76-92

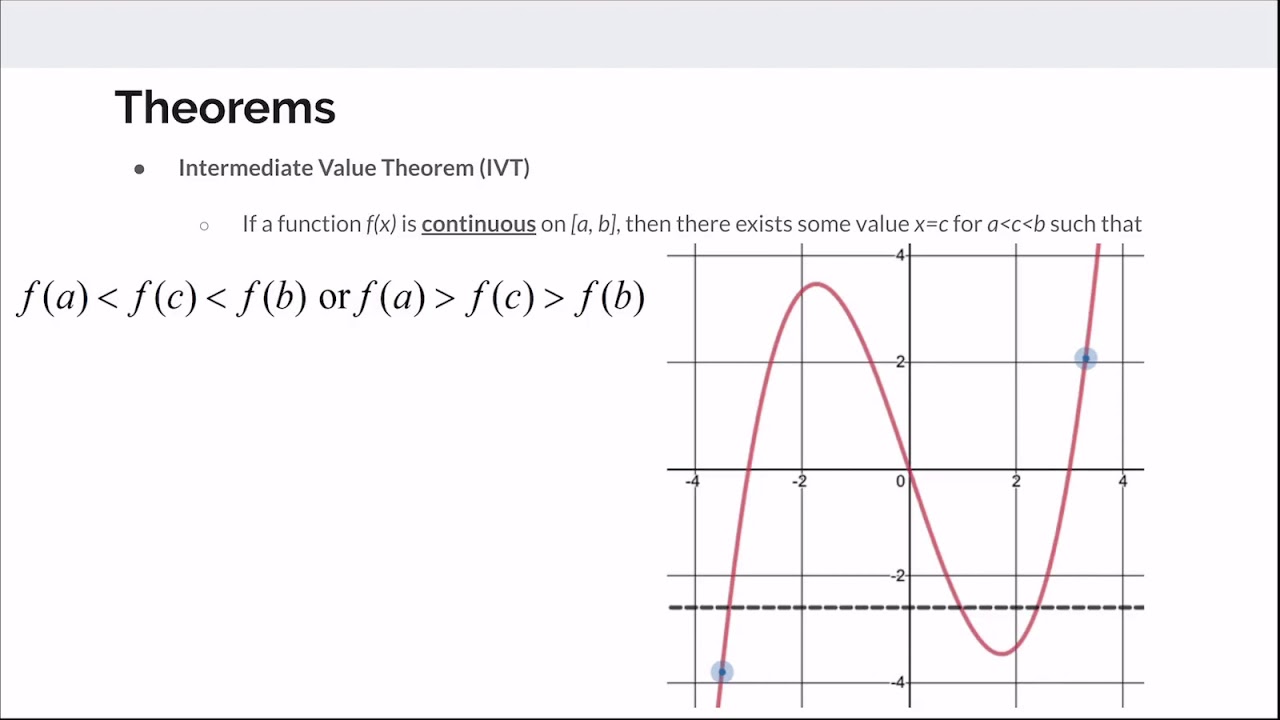
AP Calculus AB Review

AP Calculus AB 2012 Multiple Choice (calculator) - Questions 76 - 92

Business Midterm Exam Review Solutions Part 2

Related Tags

Calculus Test Derivatives Integrals Optimization University of Cincinnati Math Tutorial Test Analysis Educational Content Mathematical Concepts Exam Preparation Academic Video