PreCalculus - Matrices & Matrix Applications (17 of 33) How to Multiply (Different Size) Matrices

Michel van Biezen
9 Jun 201504:02
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a clear and concise tutorial on matrix multiplication, focusing on the rules for multiplying non-square matrices of different sizes. It emphasizes the importance of matching the number of columns in the first matrix with the number of rows in the second matrix to perform the operation. The script provides a step-by-step example, multiplying a 2x3 matrix with a 3x1 matrix, and explains how to calculate each element of the resulting 2x1 matrix. The explanation is enriched with a practical method to visualize the process, ultimately demonstrating the product matrix. The content is both informative and engaging, making complex mathematical concepts accessible to viewers.

Takeaways
  • 🧠 Matrix multiplication is possible between non-square matrices of different sizes, provided the number of columns in the first matrix equals the number of rows in the second.
  • 📏 The dimensions of the resulting product matrix are determined by the number of rows of the first matrix multiplied by the number of columns of the second matrix.
  • 🤔 The process of matrix multiplication involves multiplying elements of the rows of the first matrix by the corresponding elements of the columns of the second matrix and summing these products.
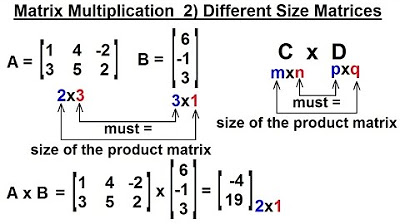
  • 🔢 For the given example, matrix A is a 2x3 matrix (two rows, three columns), and matrix B is a 3x1 matrix (three rows, one column).
  • 🙌 The multiplication of matrix A and B is feasible because the number of columns in A (3) matches the number of rows in B (3).
  • 📍 The resulting product matrix from A and B will be a 2x1 matrix (two rows, one column).
  • 🔍 The first element of the product matrix is calculated by multiplying elements from the first row of matrix A with the first column of matrix B and summing them (1*6 + 4*-1 + -2*3).
  • 📈 The second element is found by multiplying the second row of matrix A by the single column of matrix B (3*6 + 5*-1 + 2*3).
  • 📝 The final product matrix, in this case, is a 2x1 matrix with elements [1*6 + 4*-1 + -2*3, 3*6 + 5*-1 + 2*3] which simplifies to [-4, 19].
  • 👓 The script provides a step-by-step guide on how to perform matrix multiplication, including a practical example with specific numbers for clarity.
Q & A

  • What is the prerequisite for multiplying two matrices of different sizes?

    -The number of columns in the first matrix must be equal to the number of rows in the second matrix.

  • If matrix A has dimensions M by N and matrix B has dimensions P by Q, what will be the dimensions of the resulting product matrix?

    -The resulting product matrix will have dimensions M by Q, with M rows and Q columns.

  • What are the dimensions of matrix A in the given example?

    -Matrix A has dimensions of 2 by 3, which means it has 2 rows and 3 columns.

  • What are the dimensions of matrix B in the given example?

    -Matrix B has dimensions of 3 by 1, which means it has 3 rows and 1 column.

  • Can non-square matrices be multiplied together?

    -Yes, non-square matrices can be multiplied together as long as the number of columns of the first matrix is equal to the number of rows of the second matrix.

  • How is the first element of the resulting matrix in the example calculated?

    -The first element is calculated by multiplying the elements of the first row of the first matrix (A) with the elements of the first column of the second matrix (B), which results in (1 * 6) + (4 * -1) + (-2 * 3).

  • What is the process for calculating the second element of the resulting matrix in the example?

    -The second element is calculated by multiplying the elements of the second row of the first matrix (A) with the elements of the single column of the second matrix (B), resulting in (3 * 6) + (5 * -1) + (2 * 3).

  • What is the resulting product matrix in the example?

    -The resulting product matrix is a 2 by 1 matrix with elements [1 * 6 + 4 * (-1) + (-2) * 3, 3 * 6 + 5 * (-1) + 2 * 3], which simplifies to [6 - 4 - 6, 18 - 5 + 6] or [-4, 19].

  • How can one keep track of the calculations when multiplying matrices?

    -One can use a visual or physical method, such as marking with a pencil or moving fingers along the rows and columns, to keep track of the elements being multiplied and their positions in the matrices.

  • What happens when the number of columns in the first matrix does not equal the number of rows in the second matrix?

    -The matrices cannot be multiplied together because the dimensions are not compatible for matrix multiplication.

  • What is the significance of the order of matrix multiplication?

    -The order of matrix multiplication is significant because it affects the resulting product matrix. Changing the order can lead to a different product or may even result in an incompatible operation.

Outlines
00:00
🤖 Matrix Multiplication Overview

This paragraph introduces the concept of matrix multiplication, emphasizing the requirement for the matrices to be of compatible sizes. It explains that the number of columns in the first matrix must equal the number of rows in the second matrix for multiplication to be possible. The resulting product matrix's dimensions are also discussed, highlighting that it will have the number of rows from the first matrix and the number of columns from the second matrix.

📊 Non-Square Matrices Multiplication Example

The paragraph presents a specific example of multiplying two non-square matrices, matrix A and matrix B. It details the dimensions of both matrices, a 2x3 (two rows, three columns) and a 3x1 (three rows, one column), and confirms that they are compatible for multiplication as the number of columns in the first equals the number of rows in the second. The expected size of the resulting product matrix is also explained, which in this case is a 2x1 matrix.

🧮 The Multiplication Process

This part of the script delves into the actual process of multiplying the two matrices. It provides a step-by-step explanation of how to calculate each element of the resulting matrix by performing the necessary multiplications and additions. The example concludes with the final product, demonstrating the outcome of the matrix multiplication for the given matrices A and B.

Mindmap
Keywords
💡Matrix Multiplication
Matrix multiplication is a mathematical operation that involves taking two matrices - arrays of numbers arranged in rows and columns - and using a specific procedure to combine them into a third matrix. In the video, it is explained that for matrix multiplication to be possible, the number of columns in the first matrix must be equal to the number of rows in the second matrix. The resulting matrix will have dimensions equal to the number of rows of the first matrix multiplied by the number of columns of the second matrix.
💡Non-square Matrices
Non-square matrices are matrices that do not have the same number of rows and columns. This is in contrast to square matrices, which have equal numbers of rows and columns. In the video, the focus is on non-square matrices and their ability to be multiplied together under certain conditions. The script uses non-square matrices as examples to demonstrate the process of matrix multiplication.
💡Matrix Dimensions
Matrix dimensions refer to the size of a matrix, which is defined by the number of rows and columns it contains. Understanding matrix dimensions is crucial for matrix multiplication because it determines whether or not two matrices can be multiplied and what the resulting matrix's dimensions will be.
💡Rows and Columns
In the context of matrices, rows and columns are the horizontal and vertical arrangements of numbers or elements, respectively. Rows are listed horizontally, while columns are listed vertically. The script emphasizes the importance of the number of columns in the first matrix matching the number of rows in the second matrix for multiplication to occur.
💡Resulting Matrix
The resulting matrix, also known as the product matrix, is the output matrix obtained from multiplying two matrices together. The size and elements of this matrix are determined by the dimensions and elements of the original matrices and the rules of matrix multiplication.
💡Dot Product
The dot product is a mathematical operation that takes two equal-length sequences of numbers (usually coordinate vectors) and returns a single number. This operation is used to calculate the elements of the resulting matrix in matrix multiplication. Each element of the product matrix is the dot product of the corresponding row of the first matrix and the column of the second matrix.
💡Element-wise Calculation
Element-wise calculation refers to the process of computing each element of the resulting matrix by multiplying and adding corresponding elements from the rows of one matrix and the columns of the other. This is the fundamental operation in matrix multiplication, where each cell in the product matrix is calculated individually based on the cells in the input matrices.
💡Mathematical Operation
Mathematical operations are the various processes or steps that are applied to numbers or mathematical objects to calculate or transform them. In the context of the video, matrix multiplication is a specific mathematical operation that combines two matrices into a new one following a set of rules.
💡Arrays
Arrays, in the context of mathematics and computer science, are data structures that store a collection of elements, typically of the same data type, in a contiguous block of memory. In the video, matrices are a specific type of two-dimensional array, where each element is an individual number arranged in rows and columns.
💡Linear Algebra
Linear algebra is a branch of mathematics that deals with linear equations, vectors, matrices, and transformations. It provides a powerful framework for expressing and solving problems involving multiple variables. The video's topic of matrix multiplication is a fundamental concept in linear algebra, as it is used in various applications, from solving systems of linear equations to computer graphics and machine learning.
💡Mathematical Procedures
Mathematical procedures refer to the step-by-step processes used to solve mathematical problems or perform calculations. In the video, the detailed procedure for multiplying two matrices is explained, including the conditions that must be met and the specific steps to calculate each element of the resulting matrix.
Highlights

Matrix multiplication is demonstrated with non-square matrices of different sizes.

Two matrices can be multiplied if the number of columns in the first matrix equals the number of rows in the second matrix.

The resulting matrix's dimensions are the product of the first matrix's rows and the second matrix's columns.

Matrix A is a 2x3 matrix, representing two rows and three columns.

Matrix B is a 3x1 matrix, representing three rows and one column.

Matrix A and B can be multiplied because the number of columns in A equals the number of rows in B.

The resulting product matrix will be a 2x1 matrix, having two rows and one column.

The multiplication process involves taking elements from the rows of the first matrix and columns of the second matrix.

The first element of the resulting matrix is calculated by multiplying corresponding elements of the first row of Matrix A and the first column of Matrix B.

A mnemonic technique is suggested for matrix multiplication, using physical movement to track the position of the elements.

The top element of the resulting matrix is found by multiplying and adding elements from the first row of A and the first column of B.

The second element of the product matrix is calculated by using the second row of A and the single column of B.

The final product of matrices A and B is a 2x1 matrix with the values [19, -4].

The example provided illustrates the practical application of matrix multiplication with non-square matrices.

The transcript serves as an educational resource for understanding the process and requirements of matrix multiplication.

The method described can be applied to various mathematical and computational problems involving matrices.

The transcript explains the concept in a step-by-step manner, making it accessible for learners at different levels.

Transcripts

Browse More Related Video

PreCalculus - Matrices & Matrix Applications (17 of 33) How to Multiply (Different Size) Matrices

PreCalculus - Matrices & Matrix Applications (16 of 33) How to Multiply (Square) Matrices

Matrix Multiplication and Associated Properties

7.2.3 Matrix Multiplication

Multiplying a matrix by a column vector | Matrices | Precalculus | Khan Academy

How To Multiply Matrices - Quick & Easy!

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
LinearAlgebraMatrixMultiplicationMathematicsEducationNonSquareMatricesMathTutorialsElectronAppProgrammingTechExplainersMathematicsEducation