Calculus - The basic rules for derivatives
TLDRIn this engaging video from MySecretMathTutor, the basics of derivatives in calculus are explored. The video introduces the derivative operator and explains its role in differentiating parts of a function. Key takeaways include the rule that derivatives can be split over addition and subtraction, the fact that the derivative of a constant is zero, and that the derivative of x is one. The video also covers the power rule for constants multiplied by variables and the unique property of the natural exponential function e^x, whose derivative remains e^x. Several examples are worked through to illustrate these concepts, showing how to apply these basic rules to find derivatives of more complex functions. The video concludes by encouraging viewers to explore additional derivative rules, such as the power, product, quotient, and chain rules, for a deeper understanding of calculus.
Takeaways
- 📚 Start with the basics: Understand the concept of derivatives as the rate of change of a function.
- 🔍 Derivative operator: Recognize the symbol used to denote taking the derivative of a function.
- ➕➖ Splitting rule: Derivatives can be split over addition and subtraction, allowing you to take the derivative of each term separately.
- 🔢 Constant rule: The derivative of a constant is always zero, as there is no change with respect to the variable.
- 🆔 Identity rule: The derivative of x (or any variable) is 1, representing the basic rate of change.
- ✖️ Constant multiplication: When a constant is multiplied by a variable, the constant can be factored out in front of the derivative.
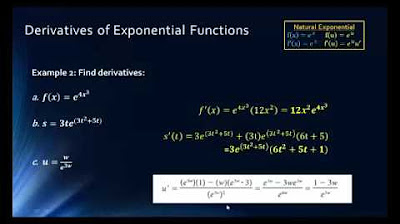
- 🔁 Exponential rule: The derivative of e^x (natural exponential function) is the same function, e^x.
- 📉 Zero derivative for constants: Any term without a variable, such as the square root of a constant, will have a derivative of zero.
- 📈 Linear term derivative: The derivative of a term like 5x is 5, pulling the coefficient in front of the derivative of x, which is 1.
- 🔁 Power rule preview: Anticipate learning the power rule for derivatives of terms like x^2 and x^3 in future lessons.
- 📚 Further learning: Encouragement to watch additional videos on more complex derivative rules like the power rule, product rule, quotient rule, and chain rule.
Q & A
What is the derivative operator used for in calculus?
-The derivative operator is used to take the derivative of a piece of a function, indicating the rate at which the function is changing at a certain point.
How does the derivative operator behave when applied to a constant?
-When the derivative operator is applied to a constant, the result is 0, because the rate of change of a constant is zero.
What is the derivative of a single x with respect to x?
-The derivative of a single x with respect to x is 1, as it represents the rate of change of a linear function.
How can you handle the derivative of a function that consists of multiple terms combined by addition or subtraction?
-You can handle it by applying the derivative operator to each term separately, due to the linearity of differentiation, which allows you to split the derivative over addition and subtraction.
What happens when you multiply a constant by a function and then take the derivative?
-The constant can be factored out in front of the derivative operator, so you end up with the constant times the derivative of the function itself.
What is special about the derivative of the natural exponential function e^x?
-The derivative of e^x is the same as the original function, e^x, meaning it does not change when you take its derivative.
If a function is f(x) = 5 * sqrt(2), what is its derivative?
-Since there is no x in the function, it's a constant, and the derivative of a constant is 0. So the derivative of f(x) is 0.
What is the derivative of the function f(x) = 3 + x?
-Using the rule for addition, the derivative of 3 is 0 (since it's a constant) and the derivative of x is 1. Therefore, the derivative of f(x) is 1.
How do you find the derivative of the function f(x) = 5x + 1?
-The derivative of 5x is 5 (since you bring the constant out front) and the derivative of 1 is 0 (since it's a constant). So the derivative of f(x) is 5.
What is the derivative of the function f(x) = x - 3x + sqrt(5)?
-The derivative of x is 1, the derivative of -3x is -3, and the derivative of sqrt(5) (a constant) is 0. So the derivative of f(x) is 1 - 3, which simplifies to -2.
What is the derivative of the function f(x) = 3e^x - 4x?
-Using the rules for constants and the special rule for e^x, the derivative of 3e^x is 3e^x and the derivative of -4x is -4. So the derivative of f(x) is 3e^x - 4.
Why are the basic rules of derivatives important for more complex functions?
-The basic rules of derivatives are the foundation for understanding and calculating more complex derivatives. They allow you to break down complicated functions into simpler parts and apply the rules accordingly.
Outlines
📚 Introduction to Derivatives
This paragraph introduces the concept of derivatives in calculus. It explains that derivatives are used to find the rate of change of a function and that there are many rules to calculate them. The video focuses on the basic rules, suggesting viewers also watch videos on the power rule, product rule, quotient rule, and chain rule for a comprehensive understanding. The derivative operator is introduced as a notation to denote taking the derivative of a function. The paragraph also emphasizes the ability to split derivatives over addition and subtraction, the fact that the derivative of a constant is zero, and that the derivative of x is one. It concludes with an introduction to the derivative of a natural exponential, e^x, which is its own function.
🔍 Applying Basic Derivative Rules
This paragraph delves into applying the basic rules of derivatives through several examples. It starts with the derivative of a constant multiplied by a number, which is zero, and then moves on to the derivative of a sum of constants and variables. The paragraph explains how to handle constants multiplied by variables by moving the constant outside the derivative operator. It also revisits the special case of the derivative of e^x, which is e^x itself. The examples provided include finding the derivatives of functions such as 5 times the square root of 2, 3 + x, 5x + 1, x - 3x + the square root of 5, and 3e^x - 4x. Each example demonstrates the process of breaking down the function into simpler parts, applying the basic rules, and combining the results to find the overall derivative. The paragraph concludes by encouraging viewers to explore more complex derivative rules in additional videos.
Mindmap
Keywords
💡Derivative
💡Derivative Operator
💡Basic Rules of Derivatives
💡Sum Rule
💡Constant Rule
💡Constant Multiple Rule
💡Power Rule
💡Natural Exponential Function
💡Product Rule
💡Quotient Rule
💡Chain Rule
Highlights
The video begins with an introduction to the basic rules of derivatives in calculus.
Derivatives can be split over addition and subtraction, allowing for the derivative of each term to be taken separately.
The derivative operator is introduced as a notation for taking the derivative of a function.
Constants, when differentiated, result in zero, whereas the derivative of a single x is one.
The power rule for derivatives is mentioned as a topic for a follow-up video.
When a constant is multiplied by a variable, the constant can be moved outside the derivative operator.
The derivative of a natural exponential function, e^x, is the same as the original function.
The derivative of a constant multiplied by a function is calculated by multiplying the constant by the derivative of the function.
The video provides an example of finding the derivative of a function that is a constant times the square root of a number, resulting in zero.
The derivative of a sum of a constant and a variable is shown to be the derivative of the variable, which is one.
For a function consisting of a constant times a variable plus a constant, the derivative is the constant times the derivative of the variable.
An example is given for a function with multiple terms, showing how to apply the derivative operator to each term separately.
The derivative of a function with a constant minus a constant times a variable plus the square root of a number is derived.
The video concludes with an example of a function involving an exponential term and a linear term, emphasizing the use of the basic rules.
The importance of understanding basic derivative rules before moving on to more complex rules like the power, product, quotient, and chain rules is emphasized.
The video encourages viewers to visit MySecretMathTutor.com for more math videos.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: