Concentration Changes Over Time - AP Chem Unit 5, Topic 3
TLDRThe video script provides an in-depth exploration of integrated rate laws, which are essential tools for determining the order of a chemical reaction with respect to a specific reactant. Jeremy Kug explains that by plotting the concentration of a reactant against time, one can discern the reaction order through the shape of the resulting graph. A straight line indicates a zeroth-order reaction, a natural logarithm plot suggests a first-order reaction, and a reciprocal plot points to a second-order reaction. The script further delves into calculating rate constants and concentrations at various times using integrated rate laws. It also covers the concept of half-life, particularly relevant for first-order processes like nuclear decay. The presenter demonstrates how to apply these laws to solve example problems, emphasizing the importance of understanding and correctly applying these principles for AP Chemistry students.
Takeaways
- 📈 **Integrated Rate Laws**: These laws help determine the order of a reaction with respect to a certain reactant using graphical methods.
- 📊 **Graph Analysis**: By plotting reactant concentration versus time, the type of graph (straight line, natural log, or reciprocal) indicates the reaction order (zeroth, first, or second).
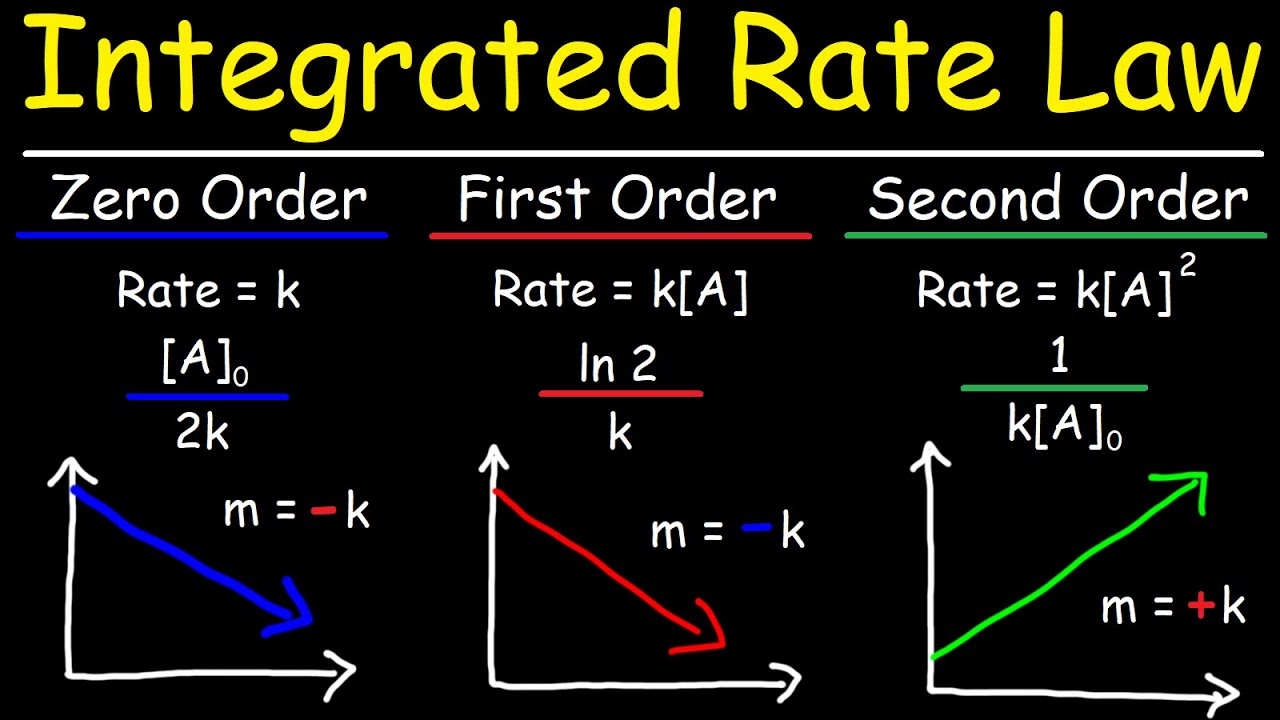
- 🔍 **Zeroth Order Reaction**: If the concentration vs. time plot is a straight line, the reaction is zeroth order, and the rate law is rate = k[A]^0, where k is the rate constant.
- 🔢 **First Order Reaction**: A straight line in the natural log of concentration vs. time plot signifies a first order reaction, with the rate law rate = k[A].
- 🔴 **Second Order Reaction**: A straight line in the reciprocal of concentration vs. time plot indicates a second order reaction, with the rate law rate = k[A]^2.
- ⚖️ **Rate Constant Determination**: For zeroth, first, and second order reactions, the rate constant (k) can be found by calculating the slope of the respective straight line graph.
- 🧮 **Integrated Rate Law**: For first order reactions, the integrated rate law allows for the calculation of reactant concentration at any given time, using the equation ln[A]t - ln[A]0 = -kt.
- ⏳ **Half-Life Calculation**: The time for half of the initial reactant to be depleted in a first order process is called the half-life and can be calculated using the equation t½ = 0.693 / k.
- 🔬 **Example Problem Solving**: The script provides examples of how to use integrated rate laws to find the rate constant and concentration at a specific time for both first and second order reactions.
- ⚠️ **Significance of Half-Life**: The half-life is particularly important for first order processes, such as radioactive decay, and helps understand how long it takes for a substance to decay.
- 📚 **Study Tips**: The script suggests that understanding and memorizing the integrated rate laws and their respective equations is crucial for AP Chemistry students.
Q & A
What is the significance of integrated rate laws in chemical kinetics?
-Integrated rate laws are crucial in chemical kinetics as they provide a means to determine the order of a reaction with respect to a certain reactant, which is essential for understanding the reaction mechanism and predicting how changes in concentration will affect the reaction rate.
How can one determine if a reaction is zeroth order based on a graph?
-If a plot of the concentration of the reactant on the y-axis versus time on the x-axis results in a straight line, the reaction is zeroth order. This indicates that the concentration decreases at a constant rate over time, independent of the reactant's concentration.
What is the rate law for a zeroth order reaction?
-The rate law for a zeroth order reaction is given by rate = k, where k is the rate constant. Since anything raised to the zero power is one, the reactant concentration does not appear in the rate expression.
How is the rate constant (K) determined for a zeroth order reaction?
-The rate constant (K) for a zeroth order reaction is determined by calculating the absolute value of the slope of the straight line obtained when plotting concentration versus time. The slope is found using the rise over run method.
What does a straight line in a plot of the natural logarithm of concentration versus time indicate?
-A straight line in a plot of the natural logarithm of the reactant concentration versus time indicates that the reaction is first order. The rate law for such a reaction is rate = k[A], where [A] is the concentration of the reactant.
How is the rate constant for a first order reaction found?
-The rate constant for a first order reaction is found by determining the slope of the straight line from a plot of the natural logarithm of the reactant concentration versus time, taking the absolute value of the slope, and using it as the rate constant (k).
What does a plot of the reciprocal of the reactant concentration versus time represent for a second order reaction?
-For a second order reaction, a plot of the reciprocal of the reactant concentration (1/[A]) versus time results in a straight line. The rate law for a second order reaction is rate = k[A]^2.
How is the rate constant for a second order reaction determined?
-The rate constant for a second order reaction is determined by taking the slope of the straight line from a plot of the reciprocal of the reactant concentration versus time. Since the slope is positive for a second order reaction, the rate constant (k) is equal to the slope.
What is an integrated rate law, and how is it used?
-An integrated rate law is a mathematical equation derived from the rate law by integrating with respect to time. It is used to calculate the concentration of reactants at any given time throughout the course of a reaction, which is particularly useful for determining the amount of reactant remaining after a certain period.
What is the formula for calculating the half-life of a first order reaction?
-The formula for calculating the half-life (t1/2) of a first order reaction is t1/2 = 0.693 / k, where k is the rate constant of the reaction.
How does the concept of half-life apply to first order processes in the natural world?
-The concept of half-life is particularly relevant to first order processes such as nuclear decay in the natural world. It describes the time required for half of the initial amount of a reactant to be depleted, which is a key parameter in understanding the decay process.
What is the general approach to solving problems using integrated rate laws?
-The general approach to solving problems using integrated rate laws involves identifying the correct integrated rate law for the order of the reaction, plugging in the given values for reactant concentrations and time, and then using algebra to solve for the unknown variable, which could be the concentration at a future time, the rate constant, or the time to reach a certain concentration.
Outlines
🔍 Determining Reaction Order with Integrated Rate Laws
Jeremy Kug introduces integrated rate laws as a method to determine the order of a reaction with respect to a certain reactant using a single experiment. He explains that by plotting the concentration of the reactant against time, one can discern the order of the reaction. If a straight line is obtained, the reaction is zeroth order, with the rate constant K being the absolute value of the slope. If the plot is not linear, further graphs are made, such as the natural logarithm of the reactant concentration versus time for a first-order reaction, or the reciprocal of the concentration versus time for a second-order reaction. The rate law and rate constant are derived from the slope of the respective linear plots.
📈 Analyzing Reaction Order Through Graphs
The script provides an example of analyzing the order of a reaction with respect to NO2 using three different graphs. The straight line in the reciprocal plot indicates a second-order reaction. The rate law for this reaction is derived, incorporating the rate constant k and the concentration of NO2 squared. The rate constant is determined by calculating the slope of the linear graph. Additionally, integrated rate laws are introduced as a means to calculate the concentration of a reactant at any given time during the reaction.
🧮 Calculating Concentration and Rate Constant
The video script details how to calculate the concentration of a reactant after a certain period for a first-order process using the integrated rate law. An example calculation is provided, where the concentration of a reactant is found after 60 seconds with a given rate constant and initial concentration. The script also explains how to determine the rate constant of a reaction given the change in concentration over time. Furthermore, the concept of half-life for first-order processes is explored, including its derivation from the integrated rate law and its importance in understanding decay processes.
⏳ Half-Life and Second-Order Integrated Rate Law
The script discusses the derivation of the half-life equation for first-order reactions, showing that the half-life is equal to the natural logarithm of 2 divided by the rate constant. An example problem calculates the half-life of a process given its rate constant. Additionally, the second-order integrated rate law is introduced, and an example problem demonstrates how to use it to find the time it takes for the concentration of a reactant to decrease to a certain level, given the rate constant and initial concentration.
📚 Summary of Integrated Rate Laws
The video concludes with a summary of the integrated rate laws for zeroth, first, and second-order reactions, including their respective rate laws, units for the rate constant K, and the integrated rate law expressions. The script emphasizes that these laws can be used to plug in values and solve for unknowns using algebra, which is a skill most AP Chemistry students are expected to master. The video encourages viewers to engage with the content and look forward to the next sections on chemical kinetics.
Mindmap
Keywords
💡Integrated Rate Laws
💡Reaction Order
💡Rate Law
💡Graph
💡Slope
💡Half-Life
💡First Order Reaction
💡Second Order Reaction
💡Zeroth Order Reaction
💡Integrated Rate Law
💡Natural Logarithm
Highlights
Integrated rate laws provide a tool to determine the order of a reaction with respect to a certain reactant.
A single experiment can be used to determine the reaction order, as opposed to multiple experiments.
Graphing the concentration of the reactant against time can indicate the reaction's order if the resulting graph is a straight line.
A straight line in the concentration vs. time graph indicates a zeroth order reaction, with a constant rate over time.
The rate law for a zeroth order reaction is rate equals K times the reactant to the zero power, simplifying to rate equals K.
The rate constant K can be determined by the absolute value of the slope of the concentration vs. time graph for a zeroth order reaction.
If the plot of the natural logarithm of the reactant concentration vs. time is a straight line, the reaction is first order.
For a first order reaction, the rate law is rate equals K times the reactant, and the rate constant is the slope of the ln(concentration) vs. time graph.
A plot of the reciprocal of the reactant concentration vs. time that results in a straight line indicates a second order reaction.
The rate law for a second order reaction is rate equals K times the reactant squared, with the rate constant equal to the slope of the 1/concentration vs. time graph.
The order of a reactant in a reaction can be determined graphically using integrated rate laws.
An example problem demonstrates how to determine the order of a reaction and the rate constant using graphical methods.
Integrated rate laws can be used to calculate the concentration of a reactant at any time throughout the reaction.
The first order integrated rate law is derived using calculus and is useful for calculating concentrations over time.
The half-life of a first order process is the time it takes for half of the initial reactant to be depleted and can be calculated using the rate constant.
The half-life equation for a first order process is derived from the integrated rate law and is an important concept in nuclear decay.
The second order integrated rate law is used to calculate the time it takes for a reactant's concentration to decrease to a certain level.
Zero order integrated rate laws are straightforward and involve simple plug-and-chug algebraic manipulation.
Integrated rate laws are essential for AP Chemistry students and are provided on the AP exam.
Transcripts
Browse More Related Video

AP Chem Unit 5 Review - Kinetics in 10 Minutes!
12.33 | Use the data provided to graphically determine the order and rate constant of the following
Chemical Kinetics practice problems - complete review
14.2 Rate Laws | General Chemistry
Kinetics: Initial Rates and Integrated Rate Laws
Integrated Rate Laws - Zero, First, & Second Order Reactions - Chemical Kinetics
5.0 / 5 (0 votes)
Thanks for rating: