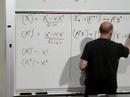Lectures 2 & 3 | Quantum Entanglements, Part 3 (Stanford)
TLDRThe video script is a comprehensive lecture on electromagnetism, delivered by a professor from Stanford University. It begins by discussing the fundamental forces that electric and magnetic fields exert on charged particles, which is encapsulated in the basic equation involving the force on a charged particle, its electric charge, and the fields themselves. The lecturer then transitions into a detailed exploration of Maxwell's equations, which are pivotal in understanding how charged particles influence electric and magnetic fields, and vice versa. A significant portion of the lecture is dedicated to demonstrating the relativistic invariance of these equations, emphasizing their consistency across different reference frames, a concept central to Einstein's theory of relativity. The script also delves into the concept of electromagnetic waves, explaining how charges and currents can generate these waves, which travel at the speed of light. The lecturer uses the framework of special relativity to show that the principles governing the interaction of light with matter are the same in all reference frames. The script concludes by highlighting the revolutionary nature of these findings in physics, which have reshaped our understanding of the universe.
Takeaways
- 📚 The fundamental equation for the force on a charged particle is F = q(E + v × B), where F is the force, q is the charge, E is the electric field, v is the velocity, and B is the magnetic field.
- 🧲 Maxwell's equations govern how electric and magnetic fields are determined in space and how they are affected by charged particles and currents.
- 🌌 The influence of electric and magnetic fields on charged particles is reciprocal; if fields can exert forces on particles, then particles must also be able to exert forces on fields to conserve energy.
- 🚀 Maxwell's equations, when written in terms of tensors, show that the laws of electromagnetism are the same in all reference frames, which is crucial for the theory of relativity.
- 🔋 An electric charge creates an electric field that spreads out from the charge, similar to how a fluid might spread out from a pipe, but in three dimensions.
- 🔌 An electric current, which is a flow of electric charges through a wire, generates a magnetic field that circulates around the wire.
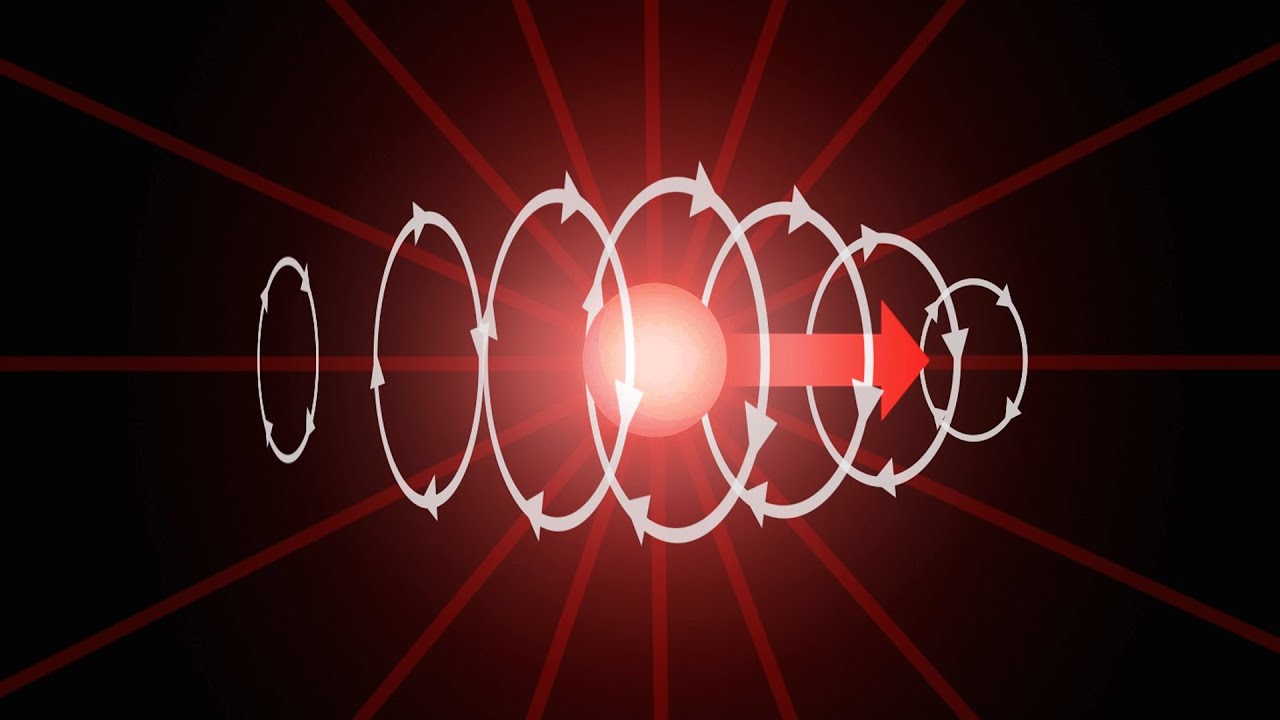
- ⚡ When a charge is moved, the electric field rearranges itself by spreading out a wave of rearrangement, which travels at the speed of light.
- 🌊 Maxwell's equations can be used to describe electromagnetic waves, such as light, which are transverse waves with electric and magnetic fields oscillating perpendicular to each other and the direction of wave propagation.
- 📐 The divergence of the electric field is related to the charge density, and the divergence of the magnetic field is always zero, indicating that magnetic fields do not emanate from or terminate at points like electric fields do.
- 🔗 The cross product is a vector operation that results in a vector, while the dot product of two vectors results in a scalar.
- ⏱️ The wave equation for electromagnetic fields can be derived from Maxwell's equations, leading to the understanding that light and other electromagnetic waves travel at the speed of light in a vacuum.
Q & A
What is the fundamental equation that describes the force on a charged particle?
-The basic equation is F = q(E + v × B), where F is the force on the charged particle, q is the electric charge of the particle, E is the electric field, v is the velocity of the particle, and B is the magnetic field.
What are Maxwell's equations?
-Maxwell's equations are a set of four fundamental equations that describe the behavior of electric and magnetic fields, how they are produced, and how they interact with charged particles.
How do electric and magnetic fields influence charged particles?
-Electric and magnetic fields influence charged particles by exerting forces on them, which can cause the particles to move or change direction. This is described by the Lorentz force law.
What is the significance of Maxwell's equations in the context of the theory of relativity?
-Maxwell's equations are significant in the theory of relativity because they demonstrate that the laws of physics, specifically the laws governing electric and magnetic fields, are the same in all reference frames. This is crucial for understanding how light can move with the same speed in every frame of reference.
What is the role of the electromagnetic field tensor in expressing Maxwell's equations?
-The electromagnetic field tensor, an anti-symmetric tensor made up of electric and magnetic field components, allows Maxwell's equations to be written in a form that is covariant, meaning it has the same form in every reference frame. This is essential for maintaining the relativistic invariance of the equations.
How do charges and currents affect electric and magnetic fields?
-Charges and currents can create electric and magnetic fields. For example, an electric charge creates an electric field that spreads out from the charge, while an electric current flowing through a wire generates a magnetic field that circulates around the wire.
What is the relationship between a changing magnetic field and electromagnetic waves?
-A changing magnetic field can induce an electric field, and vice versa, which together can form an electromagnetic wave. This is the basis for phenomena such as radio wave transmission and the operation of antennas.
What is the divergence of a vector field, and how does it relate to the electric field?
-The divergence of a vector field is a scalar quantity that results from taking the dot product of the del operator with the vector field. For the electric field, the divergence is related to the charge density according to one of Maxwell's equations, which states that the divergence of the electric field is equal to the charge density divided by the permittivity of free space.
What is the curl of a vector field, and how does it relate to the magnetic field?
-The curl of a vector field is a vector quantity that results from taking the cross product of the del operator with the vector field. In the context of Maxwell's equations, the curl of the magnetic field is related to the electric field and its time derivative, as well as any electric currents present.
What is the physical interpretation of the electric field satisfying a wave equation?
-The physical interpretation of the electric field satisfying a wave equation is that electromagnetic waves, such as light, can propagate through space. The wave equation describes how these waves can move with a constant speed (the speed of light) and how the electric and magnetic fields oscillate in these waves.
How does the presence of charges and currents modify Maxwell's equations?
-The presence of charges and currents modifies Maxwell's equations by adding terms to the equations that represent the charge density (ρ) and the current density (J). These additions ensure that the equations accurately describe how electric charges and currents generate and affect electric and magnetic fields.
Outlines
📚 Introduction to Electromagnetism and Maxwell's Equations
The video begins with an introduction to the study of electromagnetism, focusing on the influence of electric and magnetic fields on charged particles. It emphasizes the importance of understanding how these fields exert forces. The lecturer also introduces Maxwell's equations, which are fundamental in describing how charged particles affect electric and magnetic fields. The concept of the constancy of the speed of light in all reference frames, a cornerstone of the theory of relativity, is discussed in relation to Maxwell's equations.
🌌 The Effects of Charges on Electric and Magnetic Fields
The paragraph delves into the effects that electric charges and currents have on the generation of electric and magnetic fields. It explains how an electric charge produces an electric field and a moving charge (current) generates a magnetic field. The lecturer also discusses how these fields rearrange when a charge is moved, and how this rearrangement propagates as a wave, leading to the concept of electromagnetic waves. The principle that charges and currents can affect fields is highlighted, as is the idea that this interaction conserves energy.
📐 Review of Wave Equations and Field Concepts
This section reviews the concept of wave equations and fields, particularly scalar fields, which are quantities that can vibrate and form wave fields. The lecturer explains the form of the wave equation and how it can be expressed in a covariant form, invariant under changes in the reference frame. The solutions to the wave equation are also discussed, including functions of space and time that represent waves moving in different directions.
🔢 Mathematics of Cross Products, Dot Products, Curl, and Divergence
The video provides a mathematical review of cross products and dot products of vectors, which are essential for understanding vector calculus. It defines the cross product and illustrates how it results in a vector perpendicular to the plane containing the two original vectors. The dot product is introduced as a scalar quantity resulting from the multiplication of two vectors. The concepts of curl and divergence are also explained, which are operations involving the derivatives of vector components.
🧲 Maxwell's Equations and Their Covariance
Maxwell's equations are presented in their basic form, relating electric and magnetic fields to their sources, charges, and currents. The lecturer discusses the challenge of expressing these equations in a form that is invariant across different reference frames. The goal is to rewrite Maxwell's equations using tensors, which are mathematical objects that transform in a specific way under changes in the reference frame, thus maintaining the equations' form.
🤔 Implications of Maxwell's Equations for Electromagnetic Fields
The paragraph explores the implications of Maxwell's equations, particularly in the context of electromagnetic fields. It discusses how these equations allow for the propagation of electromagnetic waves, which include light waves. The lecturer explains that these waves are transverse, with electric and magnetic fields oscillating perpendicular to the direction of wave propagation. The constancy of the speed of light is also emphasized, showing that Maxwell's equations predict that light travels at the same speed in all reference frames.
🔋 Charge Density and Current Density in Electromagnetic Theory
The concept of charge density and current density is introduced, explaining how they modify Maxwell's equations in the presence of charges and currents. The lecturer defines charge density as the amount of charge per unit volume and current density as the flow of charge per unit area per unit time. The conservation of charge is highlighted as a fundamental principle of nature, stating that the total charge in a closed system remains constant.
🏗️ Gauss's Theorem and Its Relation to Maxwell's Equations
Gauss's theorem, also known as the divergence theorem, is introduced as a mathematical relationship between a surface integral of a vector field and the volume integral of the divergence of that vector field. The lecturer uses this theorem to establish a connection between the charge density and the current density, leading to the continuity equation. This equation, relating the time rate of change of charge density to the divergence of the current, is shown to be a tensor equation, thus invariant in all reference frames.
🌟 Conclusion on the Relativistic Invariance of Electromagnetism
The final paragraph concludes the discussion by emphasizing that all the equations of electromagnetism, including those involving charges and currents, can be written in a form that is invariant across all reference frames. This invariance is crucial for the theory of relativity and shows that the laws of electromagnetism, as described by Maxwell's equations, are consistent with the postulate that the speed of light is constant in all reference frames. The video ends by reiterating the significance of these findings for the field of physics.
Mindmap
Keywords
💡Electric and Magnetic Fields
💡Maxwell's Equations
💡Charged Particles
💡Relativity
💡Electromagnetic Waves
💡Tensor
💡Lorentz Transformations
💡Wave Equation
💡Speed of Light
💡Action and Reaction
💡Charge Density and Current Density
Highlights
The program discusses the fundamental principles of electromagnetism, focusing on the influence of electric and magnetic fields on charged particles.
Maxwell's equations are introduced as the governing equations that describe how electric and magnetic fields are affected by charged particles.
The concept of action and reaction is explored, emphasizing that charged particles must also affect electric and magnetic fields, leading to energy conservation.
The Lorentz force law is presented in a covariant form, illustrating how it remains consistent across different reference frames.
The importance of the constancy of the speed of light in all reference frames is highlighted, a principle central to the theory of relativity.
The transformation properties of scalars, vectors, and tensors are discussed in the context of expressing physical laws in a reference frame-independent manner.
The electric field created by a point charge and the magnetic field generated by an electric current are described through visual analogies.
The phenomenon of electromagnetic waves is explained, showing how moving charges can produce waves that propagate at the speed of light.
The wave equation is derived from Maxwell's equations, demonstrating that electromagnetic fields can form waves that travel through space.
The properties of electromagnetic waves, such as being transverse waves with the electric and magnetic fields perpendicular to the direction of wave propagation, are discussed.
The concept of charge density and its role in modifying Maxwell's equations in the presence of charges is introduced.
The continuity equation, relating the change in charge density to the divergence of the current, is derived and shown to be invariant across reference frames.
The program concludes by emphasizing that the laws of electromagnetism, including the generation and behavior of light waves, are the same in all reference frames.
The mathematical theorem known as Gauss's theorem is applied to establish a relationship between surface integrals and volume integrals of vectors.
The program demonstrates how Maxwell's equations can be written in a form that is manifestly covariant, ensuring their validity in all inertial reference frames.
The implications of Maxwell's equations are explored, showing that they allow for the propagation of electromagnetic waves in a medium-free space.
The program concludes with a discussion on how the principles of electromagnetism laid the groundwork for Einstein's theory of relativity.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: