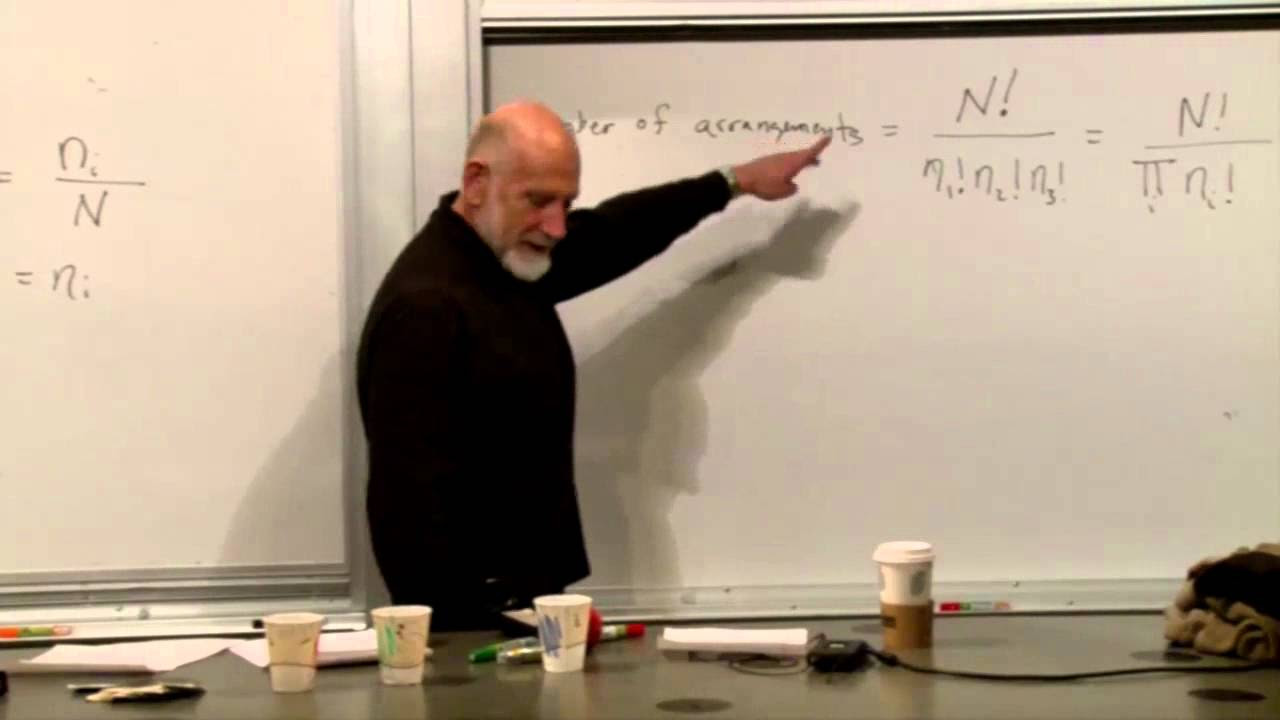Statistical Mechanics Lecture 6
TLDRThe provided video script is an in-depth exploration of the principles of thermodynamics and statistical mechanics, focusing on the behavior of gases. It discusses the limitations of physical devices in measuring intended quantities, using the example of a spring balance to illustrate the concept of measurement within a limited range. The lecturer delves into the relationship between measurable quantities like the length of a stretched spring or the height of mercury in a thermometer and the forces or temperatures they correspond to. The script also covers the ideal gas law, its breakdown, and the concept of potential energy between particles. The importance of considering the density of molecules and the range of forces in a gas is highlighted. The calculation of the partition function for a system of molecules is explained, emphasizing the approximations made for dilute gases. The script further touches on the mathematical concept of exact and inexact differentials, relating them to the physical concepts of work and heat in thermodynamics. The lecturer clarifies that heat and work are not state functions but depend on the path taken during a process, whereas the total energy of a system is a state function. This nuanced discussion is meant to provide a clear understanding of the complexities involved in the study of thermodynamics and statistical mechanics.
Takeaways
- 📏 The accuracy of physical devices is limited to a certain range, as exemplified by a spring balance used to measure force.
- 🔍 In a lab, quantities like the length of a stretched spring or the height of mercury in a thermometer can be measured and are often found to be proportional to other quantities, such as force or temperature, within a limited range.
- ⚖️ The potential energy between molecules, often negligible in an ideal gas, becomes significant in a real gas at higher densities where molecules are closer together.
- 🔄 Thermometers measure temperature based on the thermal equilibrium between the mercury and its environment, with the height of the mercury column being proportional to the temperature.
- ⏱️ Equilibrium in a thermometer is reached when it stops changing, which depends on the thermal conductivity of the material and the density of the surrounding environment.
- 🌡️ The ideal gas law breaks down when the density is high or the range of the intermolecular forces is significant, leading to deviations from the expected behavior of an ideal gas.
- 📉 The partition function for a system of weakly interacting particles can be calculated by expanding the exponential of the potential energy in a Taylor series and considering the first few terms.
- 🔗 The potential energy between any two particles is considered negligible beyond a certain distance, which simplifies calculations and assumptions in statistical mechanics.
- 🧮 The total potential energy of a system is the sum of the potential energies between all pairs of particles, which can be a significant factor in the system's behavior at high densities.
- 📐 The partition function for a weakly interacting gas is the product of the partition function for an ideal gas and a correction factor that accounts for the potential energy between particles.
- 🤔 The concept of exact and inexact differentials is crucial in thermodynamics, with heat being an inexact differential because the total heat added to a system during a cycle depends on the path taken, not just the initial and final states.
Q & A
What is the main limitation of physical devices when measuring a quantity they are intended to measure?
-Physical devices can only measure what they are intended to measure over a limited range. They may not measure the exact mathematical quantity at all times, especially when pushed beyond their designed limits.
How does a spring balance work in measuring force?
-A spring balance measures force by connecting it to an object and pulling on the object. The length of the spring's stretch is used as a measure of the force applied. However, if the force is too great, the spring can break, indicating a limitation in its measuring range.
What is the relationship between the length of a spring's displacement and the force applied?
-Over a limited range of force, the length of a spring's displacement is proportional to the force applied. This relationship is linear if the function is smooth and does not vary in a non-linear way over that range.
What factors could the length of a spring's displacement depend on besides the force applied?
-Besides the force applied, the length of a spring's displacement could depend on factors such as temperature and air pressure. However, these factors are often ignored due to their relatively small variations.
How does the height of a mercury column in a thermometer relate to the temperature?
-The height of a mercury column in a thermometer is proportional to the temperature, assuming the thermometer is in equilibrium with its environment. The relationship is linear over a small range, which is used to define the temperature scale.
What is the significance of the term 'U0' in the context of potential energy between molecules?
-The term 'U0' represents the integral of the potential energy function over the volume where the potential is significant. It is a key parameter that determines the strength of the potential energy and its distribution, which is crucial for understanding the behavior of a weakly interacting gas.
How does the ideal gas law break down when considering the forces between molecules?
-The ideal gas law breaks down when the density is high or the range of the force between molecules is long. In these cases, the potential energy between molecules becomes significant compared to the kinetic energy, and the gas behaves differently from the ideal gas model.
What is the partition function and why is it important in statistical mechanics?
-The partition function, denoted as Z, is a mathematical function that encodes the statistical properties of a system in equilibrium at a given temperature and volume. It is important because it allows for the calculation of thermodynamic quantities such as energy, pressure, and entropy.
How does the partition function for a weakly interacting gas differ from that of an ideal gas?
-For a weakly interacting gas, the partition function includes a correction factor that accounts for the potential energy between molecules. This correction is in addition to the partition function of an ideal gas, which only considers kinetic energy.
What is the first law of thermodynamics and how is it expressed mathematically?
-The first law of thermodynamics is a statement of energy conservation. It is expressed as ΔE = -PΔV + TΔS, where ΔE is the change in energy, P is the pressure, ΔV is the change in volume, T is the temperature, and ΔS is the change in entropy.
Why is heat not considered a state function in thermodynamics?
-Heat is not considered a state function because the total amount of heat added to or removed from a system during a cycle depends on the path taken, rather than the initial and final states of the system. This is demonstrated by the fact that the line integral of heat around a closed path is not zero, indicating that heat is an inexact differential.
Outlines
🔍 The Limitations of Physical Measurement Devices
The paragraph discusses the inherent limitations of physical devices in accurately measuring the quantities they are designed for. It uses the example of a spring balance to illustrate that while these devices can measure force within a certain range, they are not perfectly accurate. The speaker also touches on how other factors such as temperature and air pressure can affect measurements, but these are typically minor and can be ignored. The focus is on establishing a relationship between measurable quantities and the forces or conditions they depend on.
🌡️ Thermometers and the Concept of Equilibrium
This section delves into the operation of thermometers, highlighting that they measure the temperature of the environment only when in equilibrium with it. The importance of the thermometer's design, such as holding it vertically, is emphasized to ensure accurate readings. The discussion also explores the time it takes for a thermometer to equilibrate with its surroundings and the factors that can affect this, including the thermal conductivity of the materials involved.
🔄 Ideal Gas Law and Its Breakdown
The speaker explores the ideal gas law and the conditions under which it begins to break down. This is approached by considering the forces between gas particles and how these interactions can alter the behavior of an ideal gas. The concept of weakly interacting particles is introduced, and the potential energy between particles is incorporated into the calculations. The goal is to understand when the ideal gas approximation is valid and when the interactions between particles become significant.
📐 Calculating the Partition Function for Interacting Molecules
The paragraph focuses on setting up a mathematical problem to calculate the partition function for a system of molecules with potential energy between them. The total energy of the system is expressed as the sum of kinetic energies and potential energies between all pairs of molecules. The integral over the potential energy is emphasized as a crucial step in the calculation, and the concept of a small parameter expansion is introduced to manage the complexity of the problem.
🤔 The Importance of the Small Parameter in Statistical Mechanics
This section emphasizes the role of a small parameter in statistical mechanics calculations. The potential energy between molecules is considered small compared to the kinetic energy, allowing for an expansion in a power series. The integral involving the potential energy is identified as a key quantity that determines the strength of the potential's effect on the system. The units and significance of this integral are discussed in detail.
🧮 Partition Function and Its Relation to the Ideal Gas
The speaker breaks down the partition function for a gas with interactions between molecules. The partition function is separated into a product of a momentum integral and a position integral. The momentum integral is recognized as the same as that for an ideal gas, while the position integral involves the potential energy between pairs of particles. The partition function is then expressed as the product of the ideal gas partition function and a correction factor.
📉 Taylor Series Expansion and Its Application to Partition Functions
The paragraph discusses the use of the Taylor series expansion to approximate the logarithm of a partition function that includes a small correction term. The focus is on expanding the logarithm of (1 - x) for small x, which simplifies to -x for the purposes of the calculation. The speaker then applies this to the partition function, emphasizing the importance of the small parameter in the calculation and how it allows for a manageable expansion.
🔧 Deriving the Energy and Pressure of a Gas from the Partition Function
The speaker calculates the energy and pressure of a gas using the partition function derived from statistical mechanics. The energy of the gas is found by differentiating the logarithm of the partition function with respect to beta (the inverse temperature). The pressure is then determined by differentiating the logarithm of the partition function with respect to volume, at a fixed temperature. The resulting expressions for energy and pressure include terms from the ideal gas and correction terms due to particle interactions.
🌐 Density and Temperature Effects on Gas Behavior
The paragraph explores how the behavior of a gas, specifically its energy and pressure, is affected by density and temperature. The speaker explains that while the ideal gas law holds when the potential energy between particles is much smaller than their kinetic energy, deviations occur at higher densities. The discussion also touches on how the pressure of a gas is influenced by both the density and temperature, with the pressure being proportional to the density and the temperature.
🔧 The Role of Molecular Forces in Gas Properties
The speaker discusses the role of molecular forces, such as electrostatic forces, in determining the properties of a gas. It is noted that molecular forces typically exhibit a repulsive behavior at short distances, followed by an attractive tail at longer distances. The implications of these forces on the pressure of a gas are considered, with repulsive forces leading to increased pressure when molecules are forced close together.
🧠 Understanding the Mathematical Framework of Statistical Mechanics
The paragraph emphasizes the mathematical approach to statistical mechanics, where the calculation of partition functions and energy formulas is a straightforward process, albeit one that requires careful manipulation of symbols. The speaker highlights that while the calculations are formulaic, the interpretation of the results requires insight and an understanding of the physical phenomena they represent.
📐 The Concept of Exact and Inexact Differentials in Thermodynamics
The speaker introduces the concept of exact and inexact differentials in the context of thermodynamics. It is explained that not every pair of functions that can be written as differentials necessarily represent the differential of a well-defined function. The criterion for a pair of functions to be the derivatives of a function is the vanishing of their curl. The implications of this for the calculation of heat and work in thermodynamic processes are discussed.
🔄 The Path Dependency of Heat and Work in Thermodynamics
The paragraph concludes with a discussion on the path dependency of heat and work in thermodynamics. It is shown that while the total energy change in a cyclic process is zero, the heat added or work done can depend on the specific path taken during the process. This is demonstrated through the use of a curl test, which confirms that heat is not an exact differential, meaning it is not a state function and its value depends on the process path.
Mindmap
Keywords
💡Physical Device
💡Spring Balance
💡Thermometer
💡Equilibrium
💡Ideal Gas Law
💡Partition Function (Statistical Mechanics)
💡Potential Energy
💡Kinetic Energy
💡Thermal Conductivity
💡Surface to Volume Ratio
💡Taylor Series
Highlights
The discussion emphasizes that no physical device can measure its intended mathematical quantity at all times, only within a limited range.
An example is given about spring balances, which only accurately measure force up to a certain limit before they break.
The concept that measurements in a laboratory often depend on more than just the primary variable, such as temperature or air pressure, is introduced.
The idea that certain dependencies, like air pressure, can often be ignored due to their minimal impact is discussed.
The relationship between the length of a spring's displacement and the force applied is explored, noting that it's linear only within a limited range.
The impact of the local gravity field on the measurement of temperature with a mercury thermometer is considered.
The importance of a thermometer being in equilibrium with its environment for accurate temperature measurement is highlighted.
The time taken to achieve thermal equilibrium is discussed, which depends on the thermal conductivity of the materials involved.
The breakdown of the ideal gas law is explored, particularly when molecular interactions cannot be ignored.
An explanation is provided for how the ideal gas approximation can be improved by considering weakly interacting particles.
The partition function for a system of molecules, including potential energy, is derived using statistical mechanics.
The approximation used for the partition function is discussed, highlighting the assumptions made for dilute gases.
The calculation of the energy of a weakly interacting gas is detailed, showing how it differs from that of an ideal gas.
The pressure of a gas is related to its density and temperature, with corrections due to particle interactions.
The concept of exact and inexact differentials is explained, with examples to illustrate the difference.
The first law of thermodynamics is introduced, relating changes in energy to heat and work done by the system.
The conditions under which heat and work are considered exact or inexact differentials are explored, with implications for the path dependency of these quantities.
The practical implications of the path dependency of heat and work on the efficiency of engines and other thermodynamic processes are discussed.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: