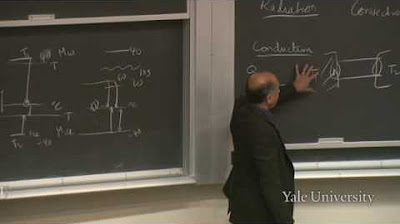23. The Second Law of Thermodynamics and Carnot's Engine
TLDRProfessor Ramamurti Shankar's lecture delves into the intricacies of thermodynamics, focusing on the First Law and its implications for the behavior of ideal gases. He explains the concept of state variables, the equation of state PV = nRT, and the quasi-static, reversible processes that gases undergo. Shankar illustrates how internal energy, heat, and work interplay during changes in a gas's state, providing examples such as isothermal expansion and compression. He also introduces specific heat capacities, C_v and C_P, and explains their significance in relation to a gas's temperature and volume changes. The lecture further explores adiabatic processes, where no heat is exchanged, leading to temperature drops as the gas expands. Through these discussions, Shankar lays the groundwork for understanding the Second Law of Thermodynamics and the concept of entropy, which will be covered in subsequent lectures.
Takeaways
- 📚 The First Law of Thermodynamics is discussed in the context of an ideal gas system, emphasizing the relationship between pressure (P), volume (V), and temperature (T), encapsulated by the equation PV = nRT.
- 🔍 The state of a gas is defined by its pressure and volume, and these macroscopic properties are sufficient to describe the state without needing to consider the microscopic details of the individual molecules.
- ⚖️ The concept of internal energy (U) is introduced as a state variable that depends only on the state of the system, not on its history, and is related to the kinetic energy of the gas molecules.
- 🔄 The First Law of Thermodynamics is expressed as the total change in internal energy (∆U) being equal to the heat input (∆Q) minus the work done by the gas (∆W).
- 🌡️ For an ideal gas, the internal energy is a function only of temperature, which is a key point when considering processes such as heating up the gas or doing work by compressing or expanding it.
- 🔁 The process of changing the state of a gas is described, including the idea of a quasi-static, reversible process that allows the gas to remain near equilibrium at all times.
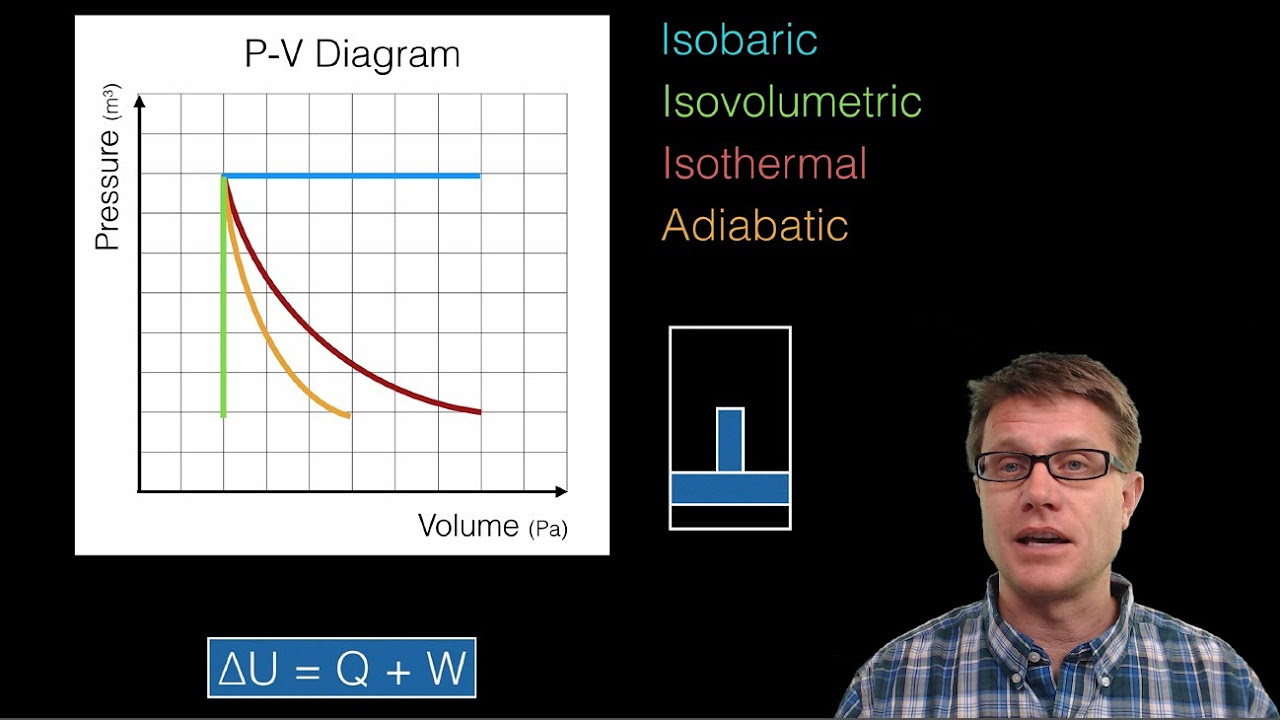
- 📈 The work done by the gas during an isothermal expansion is calculated using the integral of pressure with respect to volume, which is represented by the area under the curve in a PV diagram.
- 🔥 The heat input is shown to be equal to the work done by the gas during an isothermal process, since the internal energy change is zero and the process is reversible.
- 📊 Specific heat capacities, C_V and C_P, are defined for a gas, with C_V being the heat capacity at constant volume and C_P at constant pressure, leading to the conclusion that C_P > C_V for an ideal gas.
- 🔧 The work done in a thermodynamic cycle, such as the Carnot cycle, is equal to the area enclosed by the loop in the PV plane, and the net work depends on the direction of the cycle.
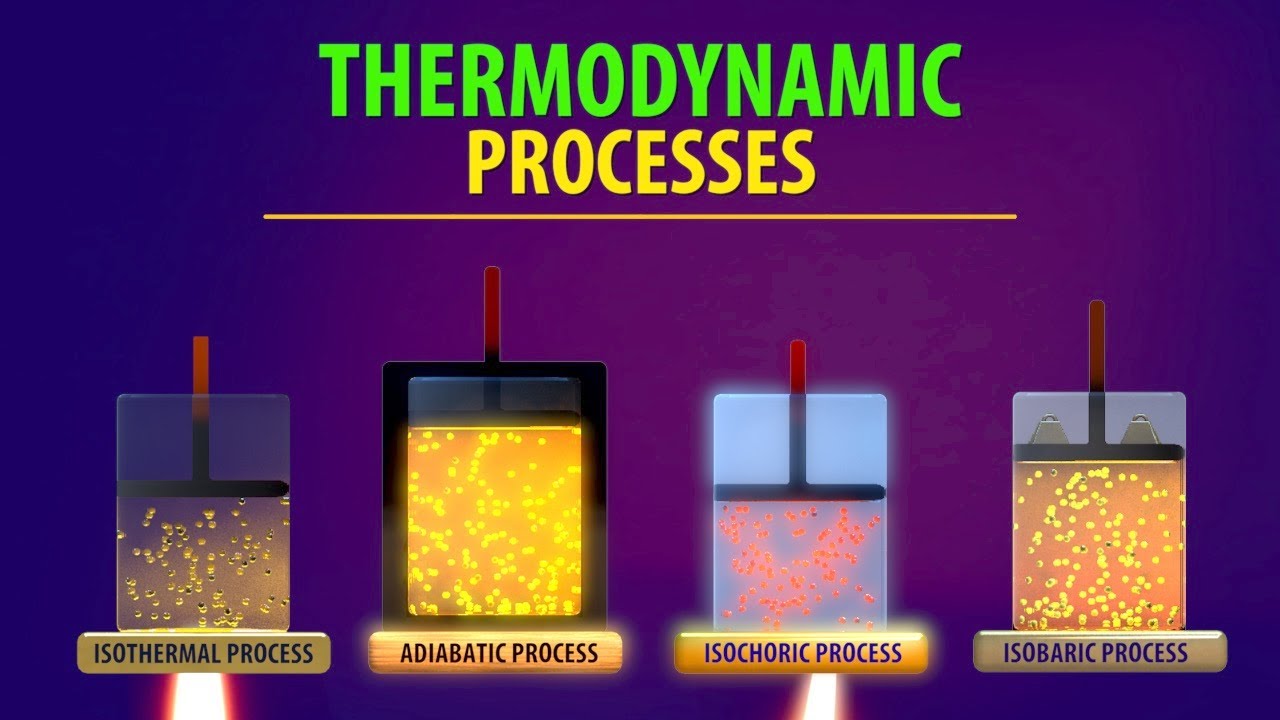
- ♻️ The concept of an adiabatic process is introduced, where no heat is exchanged with the surroundings (∆Q = 0), leading to a different relationship between pressure, volume, and temperature compared to isothermal processes.
Q & A
What is the First Law of Thermodynamics discussed in the script?
-The First Law of Thermodynamics discussed in the script is about the change in internal energy of a system. It states that the total change in internal energy (∆U) is equal to the heat input (∆Q) minus the work done by the gas (∆W), or mathematically, ∆U = ∆Q - ∆W.
What is a quasi-static process in thermodynamics?
-A quasi-static process is one that is carried out so slowly that the system remains in equilibrium at every step. It is not quite static, but as slow as you like, allowing the system to be in equilibrium at all times during the process.
What is the significance of the equation PV = nRT in the context of the script?
-The equation PV = nRT is significant as it represents the equation of state for an ideal gas. It shows that if you know the pressure (P) and volume (V) of a gas, you do not need temperature as another variable to define the state of the gas, because the temperature can be derived from this equation.
How does the script describe the concept of internal energy in relation to the state of a gas?
-The script describes internal energy as a state variable that depends on the position in the PV diagram, which represents the state of the gas. It does not depend on the history of the gas. The internal energy is associated with the kinetic energy of the gas molecules and can be represented as 3/2 kT per atom or 3/2 nRT when considering moles.
What are the two ways to change the energy of an ideal gas as described in the script?
-The two ways to change the energy of an ideal gas are by heating it up, which involves transferring energy at a molecular level from a hotter object (like a hotplate) to the gas, and by doing work on the gas, which can be achieved by compressing or expanding the gas, thus changing its volume and consequently its energy.
What is an isothermal process and how does it relate to work done by the gas?
-An isothermal process is one in which the temperature of the system remains constant. In the script, it is explained that during an isothermal expansion, the work done by the gas is equal to the heat input into the gas because there is no change in internal energy, as the temperature remains constant.
What is the concept of specific heat for a gas as discussed in the script?
-The specific heat for a gas, as discussed in the script, is the amount of heat required to produce a certain temperature change for one mole of the gas. It is important to distinguish between specific heat at constant volume (Cv) and specific heat at constant pressure (Cp), which take into account whether the gas expands or is constrained during the heating process.
How does the script explain the relationship between the specific heats Cv and Cp for an ideal gas?
-The script explains that for an ideal gas, the specific heat at constant pressure (Cp) is always greater than the specific heat at constant volume (Cv). The relationship between them is given by the formula Cp = Cv + R, where R is the gas constant. For an ideal monoatomic gas, the ratio of Cp to Cv (denoted as γ) is 5/3.
What is an adiabatic process in the context of the script?
-An adiabatic process, as described in the script, is a process in which the system (such as a gas) changes its volume but is completely thermally isolated, meaning no heat can flow into or out of the system (∆Q = 0). This results in a change in the internal energy and temperature of the system as it does work on or against the surroundings.
How does the script introduce the concept of entropy and its relation to the Second Law of Thermodynamics?
-The script introduces entropy as a quantity that will be defined and used to explain the Second Law of Thermodynamics. It suggests that the entropy of the universe always increases and that this principle will account for the irreversibility of certain processes, such as heat flowing from cold to hot without any work being done.
Outlines
📚 Introduction to Thermodynamics and the First Law
Professor Shankar begins by revisiting the First Law of Thermodynamics, which was discussed in a previous lecture. He sets the context by describing a thermodynamic system, typically an ideal gas within a piston and cylinder setup. The system's state is defined by macroscopic properties like pressure and volume, represented graphically. The microscopic state, though complex, is not of interest at this macroscopic level. The equation of state, PV = nRT, is highlighted as a fundamental relation that eliminates the need for temperature as an independent variable when pressure and volume are known. The lecture emphasizes the importance of quasi-static and reversible processes for understanding changes in the state of a system, and introduces the concept of internal energy as a state variable dependent solely on the system's current state, not its history.
🔧 The First Law of Thermodynamics and Energy Change in Gases
The paragraph delves deeper into the First Law of Thermodynamics, which quantifies the energy change of a gas. It states that the change in internal energy (∆U) is equivalent to the heat input (∆Q) minus the work done by the gas (∆W). The discussion explores the two primary ways to change a gas's energy: by heating it or by doing work on it through volume changes. The formula for work done during an isothermal process is derived, highlighting the relationship between pressure, volume, and temperature in an ideal gas. The calculation of work done is illustrated with an example of an isothermal expansion, where the work is represented by the area under a pressure-volume graph, and the integral of pressure times volume change is evaluated.
🔄 Understanding Specific Heat Capacities and the Role of Gas Expansion
This section introduces the concept of specific heat capacities for gases, which is essential for understanding how gases respond to temperature changes. It clarifies that for gases, the amount of heat required to raise the temperature depends on the number of moles, not the mass. The distinction between two types of specific heat, C_V (at constant volume) and C_P (at constant pressure), is made clear. The paragraph explains that when a gas expands, some of the heat input is used to do work, thus the temperature change is less than it would be if no work were done. This leads to the conclusion that C_P is always greater than C_V for an ideal gas, with the ratio of C_P to C_V being a constant value known as γ, which is 5/3 for a monoatomic ideal gas.
🔄 The Concept of Adiabatic Processes in Thermodynamics
The concept of adiabatic processes is introduced, where a gas undergoes a change in volume without any exchange of heat with the surroundings (∆Q = 0). This is contrasted with isothermal processes where temperature is maintained constant through heat exchange. The paragraph explains that during an adiabatic expansion, the gas does work on the surroundings, leading to a decrease in internal energy and thus a drop in temperature. The discussion leads to the question of determining the relationship between pressure and volume (P as a function of V) during an adiabatic process, setting the stage for the application of the First Law of Thermodynamics to derive this relationship.
📚 Deriving the Relationship Between Pressure and Volume in Adiabatic Processes
Building on the First Law of Thermodynamics and the condition of no heat exchange (∆Q = 0), this section derives the mathematical relationship between pressure and volume for an adiabatic process. The derivation starts with the equation ∆Q = ∆U + P∆V and uses the ideal gas law PV = RT to relate changes in temperature and volume. The integral of the resulting equation leads to a logarithmic relationship between the final and initial states of temperature and volume. The constant that arises from this relationship is interpreted as the product of pressure and volume raised to a certain power (γ), which remains constant throughout the adiabatic process.
🔄 The Carnot Engine and the Second Law of Thermodynamics
The script transitions into discussing the Carnot engine, which is a theoretical construct used to explore the Second Law of Thermodynamics. Carnot's postulate, an early version of the Second Law, states that it is impossible to construct an engine that transfers heat from a cold body to a hot body without any other effects. This postulate is used to argue that there is an upper limit to the efficiency of heat engines, which cannot be 100%. The Carnot engine operates between two isothermals at different temperatures, T_1 and T_2, and involves a cycle of expansion, cooling, compression, and heating, all while either absorbing or rejecting heat and performing work. The efficiency of the Carnot engine is expressed in terms of the temperatures of the heat reservoirs and is derived to be 1 - T_2/T_1, highlighting that no engine can surpass the efficiency of a Carnot engine.
🔄 Efficiency of Carnot Engine and Theoretical Implications
This section concludes the discussion on the Carnot engine by emphasizing its theoretical importance. The efficiency of the Carnot engine, being dependent solely on the temperatures of the heat reservoirs and not on the properties of the working substance, sets a maximum limit for all heat engines. It is stated that no real engine can surpass the efficiency of a Carnot engine, making it a fundamental concept in thermodynamics. The script hints at the connection between this result and the concept of entropy, which will be further explored in subsequent lectures. The Carnot engine serves as a benchmark for understanding the inherent inefficiencies in energy conversion processes and introduces the broader implications of the Second Law of Thermodynamics.
Mindmap
Keywords
💡First Law of Thermodynamics
💡Quasi-static process
💡Reversible process
💡State variable
💡Internal energy
💡Isothermal process
💡Specific heat
💡Adiabatic process
💡Cycles and closed processes
💡Entropy
Highlights
Introduction to the First Law of Thermodynamics and its implications for a system in equilibrium.
Explanation of macroscopic properties of an ideal gas, such as pressure and volume, and their representation on a PV diagram.
Discussion on the concept of state variables and how internal energy is a function of state, not history.
Illustration of how to change the state of a gas quasi-statically and reversibly to maintain equilibrium.
Derivation of the relationship between pressure, volume, and temperature using the equation PV = nRT.
Explanation of how the internal energy of an ideal gas is determined solely by its temperature.
Introduction of the two ways to change the energy of a gas: through heat input and work done.
Calculation of work done by a gas during an isothermal expansion using the integral of pressure-volume product.
Demonstration of the relationship between work done and heat input during an isothermal process.
Introduction to the concept of specific heat for gases and the distinction between C_V and C_P.
Derivation of the specific heats C_V and C_P for an ideal gas and the significance of the ratio γ.
Explanation of the difference between adiabatic and isothermal processes and their effects on gas temperature.
Calculation of work done in a non-isothermal process involving both expansion and compression.
Discussion on the physical interpretation of heat and work in thermodynamic processes.
Introduction to the concept of entropy and its role in the Second Law of Thermodynamics.
Carnot's postulate and its implications for the efficiency of heat engines.
Description of the Carnot cycle and its significance in setting the upper limit for engine efficiency.
Calculation of the efficiency of a Carnot engine and the proof that it is the most efficient cycle possible.
Conclusion on the importance of understanding the theoretical limits of engine efficiency and the role of entropy in thermodynamics.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: