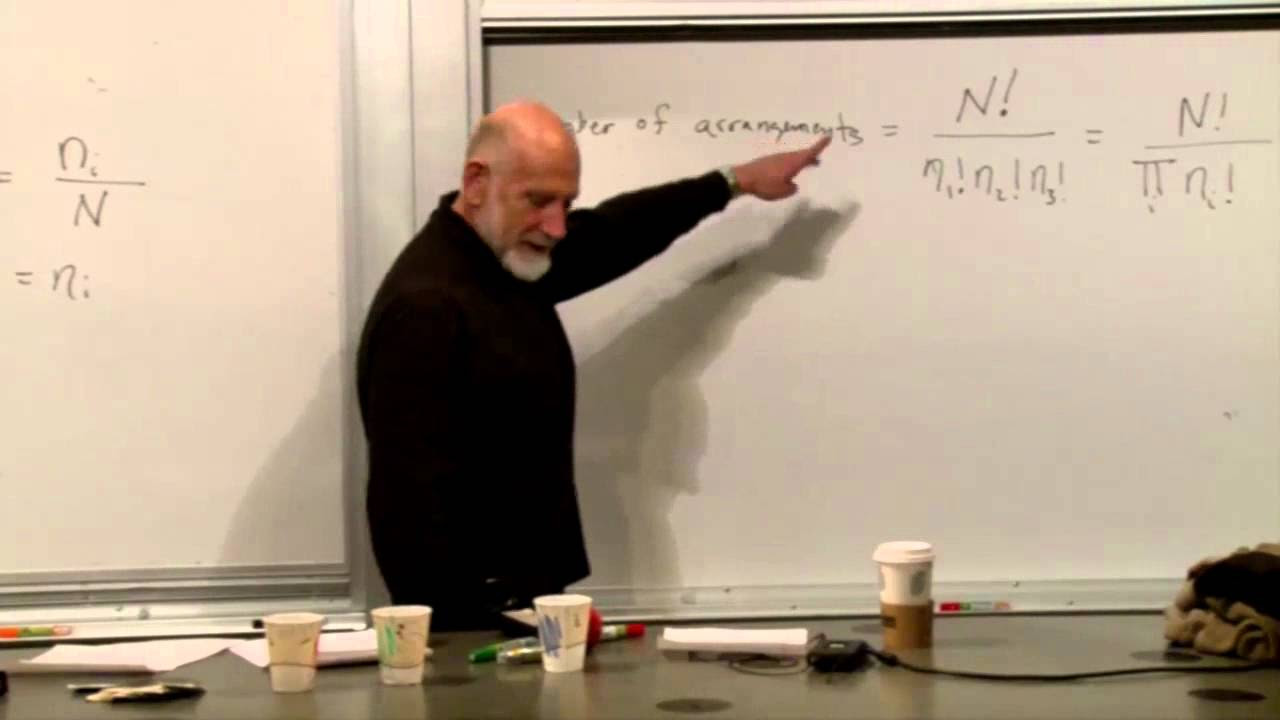Statistical Mechanics Lecture 7
TLDRThe video script is an engaging lecture that delves into the intricacies of statistical mechanics, with a particular focus on the second law of thermodynamics and the concept of entropy. The lecturer begins by discussing the speed of sound in gases and the statistical mechanics behind it, using this as a springboard to introduce the second law and its implications. The talk explores the relationship between temperature, pressure, and density, and how these factors influence the behavior of gases. The lecturer then transitions into a detailed analysis of the harmonic oscillator, highlighting the differences between classical and quantum mechanics in the context of energy distribution and temperature dependence. The script also touches on the historical confusion surrounding the energy of diatomic molecules and how quantum mechanics provided a resolution. The lecture concludes with a thought-provoking discussion on the nature of chaos in systems, the unpredictability inherent in chaotic systems, and the paradox of reversibility versus irreversibility in physics. The content is rich with scientific insight and historical context, making it a valuable resource for those interested in the foundational concepts of thermodynamics and quantum mechanics.
Takeaways
- 📚 The speed of sound in a gas can be estimated by considering the average velocity of the molecules in the gas.
- 📐 The formula for the speed of sound in a gas is related to the derivative of pressure with respect to mass density.
- 🎓 For an ideal gas, the energy of a molecule is directly proportional to the temperature (3/2 * k * T), where k is the Boltzmann constant.
- 🤔 The average energy of a harmonic oscillator in a heat bath is independent of its mass and spring constant, which was a puzzle that led to the development of quantum mechanics.
- 🧵 The phase space representation of a system's state is crucial for understanding the statistical mechanics of that system.
- ⚖️ Liouville's theorem states that the volume in phase space is conserved, which seems to imply that entropy remains constant, but this doesn't account for coarse-graining in entropy calculations.
- 🔄 The second law of thermodynamics, which states that entropy tends to increase, is a statistical law that arises from the unpredictability of particle states over time.
- 🌡️ Quantum mechanics introduces a minimum scale to the uncertainty in a system, which can help resolve the paradox between the reversibility of classical mechanics and the irreversibility observed in macroscopic systems.
- ⛄️ At low temperatures, quantum effects dominate and suppress the energy of oscillators, leading to behavior that is significantly different from classical predictions.
- 🌟 Einstein's work on the specific heat of solids was an early example of applying quantum mechanics to solve problems that classical physics could not.
- ⏳ The time it takes for a system to return to its initial state from a state of maximum entropy is extremely long, highlighting the unpredictability inherent in chaotic systems.
Q & A
What is the second law of thermodynamics?
-The second law of thermodynamics states that the entropy of a closed system can never decrease; it can only increase or stay the same. This is often interpreted as the law that governs the transfer of heat and the increase of entropy in a system.
How does the speed of sound in a gas relate to the average velocity of the molecules?
-The speed of sound in a gas is expected to be somewhat faster than the time it takes for a molecule to travel a certain distance. For a very dilute gas, the speed of sound might be close to the average velocity of the molecules, as it spreads out by the molecules moving out.
What is the relationship between the speed of sound squared and the derivative of pressure with respect to mass density?
-The speed of sound squared in a gas is equal to the derivative of pressure with respect to mass density. This relationship is more general than just for an ideal gas and is a fundamental formula in thermodynamics.
What is the quantum mechanical explanation for the energy levels of a harmonic oscillator?
-In quantum mechanics, the energy levels of a harmonic oscillator are quantized, meaning they come in discrete multiples of Planck's constant times the frequency (h-bar * Omega). Each energy level is an integer multiple of this quantum of energy.
How does the average energy of a quantum harmonic oscillator compare to its classical counterpart at high temperatures?
-At high temperatures, the quantum harmonic oscillator behaves similarly to its classical counterpart, with the average energy per oscillator being approximately equal to kT/2, where k is the Boltzmann constant and T is the temperature.
What is the significance of the crossover point where the behavior of an oscillator transitions from quantum to classical?
-The crossover point occurs when the beta times the h-bar times Omega (β * ħ * Ω) is about equal to 1. At this point, the oscillator starts to behave classically, meaning the energy of the oscillator is equal to one quantum's worth of energy, and the discreteness of the energy levels becomes less significant.
Why does the specific heat of a crystal pose a problem for classical physics?
-Classical physics overestimates the specific heat of a crystal because it does not account for the quantum mechanical suppression of high frequency oscillators at low temperatures. According to classical physics, the energy per oscillator is independent of the spring constant, which leads to an infinite amount of energy for an infinite number of oscillators, an unphysical result.
What is the role of quantum mechanics in explaining the specific heat of solids?
-Quantum mechanics helps explain the specific heat of solids by introducing the concept that energy levels are quantized. At low temperatures, only a few of these energy levels are populated, leading to a lower specific heat than what classical physics predicts.
How does the concept of coarse-graining in phase space relate to the increase of entropy?
-Coarse-graining in phase space is a process where individual points are replaced by blobs due to limited resolution. As the system evolves, these blobs spread out, leading to an increase in the perceived volume they occupy. This increase in volume corresponds to an increase in entropy due to the loss of precise information about the system's state.
What is the paradox associated with the reversibility of Newton's equations and the irreversibility observed in thermodynamic processes?
-The paradox lies in the fact that while Newton's equations of motion are reversible, implying that time can flow in both directions without changing the laws, the second law of thermodynamics suggests an arrow of time, where entropy always increases. This irreversibility is reconciled by considering the probabilistic nature of entropy increase and the sensitivity of chaotic systems to initial conditions.
What is the difference between a chaotic system and a non-chaotic system in terms of predictability?
-A chaotic system is one where nearby trajectories in phase space exponentially diverge over time, making long-term predictability impossible without infinite precision in initial conditions. A non-chaotic system, on the other hand, has trajectories that do not diverge significantly over time, allowing for more reliable long-term predictions.
Outlines
😀 Introduction to the Second Law of Thermodynamics
The speaker begins by expressing their intention to discuss the second law of thermodynamics, emphasizing its importance and complexity. They mention that understanding this law is crucial and challenging, suggesting that very few people have a deep grasp of it. Before diving into the law, the speaker wants to explore physical examples related to statistical mechanics, specifically focusing on the speed of sound in gases. They propose a thought experiment involving a dilute gas and ponder the speed at which sound travels through it, relating it to the average velocity of gas molecules.
🌬️ Speed of Sound and Gas Dynamics
The discussion shifts to the speed of sound in a gas, particularly a dilute one. Two formulas are alluded to, but the focus is on the behavior of sound in a very dilute gas. The speaker theorizes that the speed of sound might be close to the average velocity of the molecules. To support this claim, they derive a formula for the average velocity of molecules in a gas at thermal equilibrium at a given temperature T. The relationship between temperature, kinetic energy, and the mass of the molecules is explored, leading to an estimation of the speed of sound that is consistent with known values.
🔍 Puzzle of Sound Speed and Pressure Variation
The speaker poses a puzzle regarding the constancy of temperature in relation to the speed of sound. They assume that for small amplitude sound waves, the temperature remains fairly constant, which is a reasonable assumption for an ideal gas. They acknowledge some uncertainty about this assumption but suggest it's likely accurate. The conversation then turns to the relationship between pressure variation, density, and the speed of sound. It's noted that while pressure and density variations are small, their derivative with respect to each other is not necessarily small, hinting at a complex relationship in non-ideal conditions.
🎓 Harmonic Oscillator and Statistical Mechanics
The topic of a harmonic oscillator is introduced, moving from the study of gases to oscillatory systems. The speaker describes a harmonic oscillator as a system that oscillates when disturbed, such as a spring-mass system or an electromagnetic wave in a cavity. They aim to calculate the average energy of the oscillator and how it depends on mass and the spring constant. The energy of the oscillator is expressed in terms of its coordinate and the spring constant. The speaker then outlines the process of applying statistical mechanics to find the average energy of the oscillator by using the Boltzmann distribution.
🧮 Partition Function and Energy Calculation
The speaker delves into the calculation of the partition function for a quantum harmonic oscillator. They explain that the partition function is a crucial concept in statistical mechanics and express it as an integral over momentum and position space. The integration process is detailed, highlighting the separation of variables and the use of the Gaussian integral result. The partition function is simplified to an expression involving fundamental constants, the mass of the oscillator, and the spring constant. The energy of the oscillator is then derived from the partition function, revealing that the average energy depends on temperature but not on the mass or spring constant.
🔄 Classical vs. Quantum Mechanics
The speaker contrasts the classical and quantum mechanical treatment of a harmonic oscillator. They highlight the paradox that in the classical view, the energy of an oscillator is independent of its mass and spring constant, which seems counterintuitive. The speaker suggests that this paradox was a point of confusion historically and led to the realization that classical physics was incomplete. They introduce quantum mechanics as the missing ingredient, explaining that the energy levels of an oscillator are quantized in quantum mechanics. The quantum mechanical partition function is derived, and the energy calculation reflects the discrete energy levels of the oscillator.
🔧 Quantum Mechanics and Specific Heat of Solids
The discussion turns to the specific heat of solids, which was a problem that classical physics could not accurately explain. The speaker credits Einstein for proposing that quantum mechanics could resolve the discrepancy by showing that at low temperatures, the energy levels of oscillators in a crystal are suppressed, leading to lower specific heat than predicted by classical physics. The speaker emphasizes that quantum mechanics provides a correction to the classical view and that the transition from quantum to classical behavior in oscillators occurs when the temperature is high enough to provide more than one quantum's worth of energy.
🎰 Chaotic Systems and the Second Law of Thermodynamics
The speaker explores the concept of chaotic systems and their relation to the second law of thermodynamics. They describe how small differences in initial conditions can lead to vastly different outcomes in chaotic systems, making long-term predictions impossible without infinite precision. This sensitivity to initial conditions and the resulting unpredictability are central to the idea that entropy, or the degree of disorder in a system, tends to increase over time. The speaker also touches on the historical significance of understanding entropy and the second law, mentioning the contributions of Boltzmann and the concept of coarse-graining in phase space.
⛓️ Reversible Motion and the Paradox of Irreversibility
The speaker addresses the paradox between the reversibility of Newton's laws of motion and the apparent irreversibility of macroscopic phenomena, as described by the second law of thermodynamics. They discuss how the concept of coarse-graining in phase space helps to resolve this paradox by introducing a measure of uncertainty that aligns with real-world observations. The speaker suggests that while a system can return to its original configuration, the likelihood of this happening becomes increasingly small as the system size grows, reflecting the practical irreversibility observed in nature.
🤔 The Nature of Chaos and Predictability
The speaker concludes with a discussion on the nature of chaos in dynamical systems. They define chaos as a situation where nearby trajectories in phase space diverge exponentially over time, leading to a loss of predictability. They contrast this with non-chaotic systems, such as a simple pendulum or an orbit around the Sun, where nearby trajectories remain close. The speaker acknowledges the complexity of determining whether a given system is chaotic and notes that while most systems are unpredictable over time due to chaos, knowing the initial conditions with infinite precision would allow for perfect predictability.
Mindmap
Keywords
💡Second Law of Thermodynamics
💡Speed of Sound
💡Harmonic Oscillator
💡Quantum Mechanics
💡Coarse-Graining
💡Entropy
💡Phase Space
💡Chaos Theory
💡Statistical Mechanics
💡Boltzmann Distribution
💡Thermal Equilibrium
Highlights
The lecture begins with an introduction to the second law of thermodynamics, emphasizing its importance and the challenge it presents in terms of consistency with other physical laws.
The concept of the speed of sound in a gas is explored, with a focus on deriving its relationship with molecular velocity and the implications for a dilute gas.
An approximate formula for the speed of sound is discussed, highlighting the difference between ideal gas behavior and real gas behavior under various conditions.
The role of pressure and mass density in determining the speed of sound is examined through the derivation of the standard formula for the square of the speed of sound.
The lecturer provides a numerical example using the speed of sound in air to illustrate the application of the theoretical concepts discussed.
The harmonic oscillator is introduced as a system for studying statistical mechanics, with an emphasis on understanding its average energy and how it varies with mass and spring constant.
The partition function for a quantum mechanical oscillator is derived, revealing the quantization of energy levels and the role of Planck's constant in determining these levels.
The crossover between quantum and classical behavior in oscillators is discussed, with a focus on the temperature-dependent activation of oscillators.
The concept of coarse-graining in phase space is introduced to explain the increase in entropy and the limitations of resolution in observing system dynamics.
The second law of thermodynamics is discussed in the context of phase space, highlighting the tension between the reversibility of microscopic dynamics and the apparent irreversibility of macroscopic processes.
The idea of Liouville's theorem and its implications for the conservation of volume in phase space is presented, contrasting with the observed increase in entropy.
The lecturer addresses the paradox of reversibility versus irreversibility in systems, discussing the historical significance and the contributions of Boltzmann to resolving this paradox.
The role of chaos in systems is explored, explaining how small changes in initial conditions can lead to vastly different outcomes in chaotic systems.
The concept of predictability in systems is discussed, with a focus on the relationship between the desired prediction time and the precision required in initial conditions.
The double pendulum is used as an example of a chaotic system, demonstrating how seemingly small changes can lead to unpredictable and complex behavior.
The lecturer concludes with a teaser for the next lecture, promising to delve deeper into the second law of thermodynamics, reversibility, and the resolution of the paradox of irreversibility.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: