Calculus 1 Lecture 3.7: Optimization; Max/Min Application Problems
TLDRThe video script delves into the application of calculus to optimize various real-world scenarios. It begins with a discussion on maximizing and minimizing continuous functions, particularly over closed and open intervals. The concept of absolute maximum and minimum values and their occurrence at endpoints or relative extrema is introduced. The script then illustrates these ideas with practical examples, such as calculating the dimensions for a box to maximize volume with a given amount of cardboard, and determining the most cost-effective way to fence a rectangular region. It also explores the optimization of a pipeline's route to minimize construction costs, taking into account different costs for underwater and onshore pipes. The script concludes with a geometric problem of designing a cylindrical soda can that holds a liter of liquid while minimizing the surface area of material used, demonstrating the use of calculus to derive the dimensions for cost efficiency. The summary underscores the practical utility of calculus in everyday problem-solving and decision-making.
Takeaways
- 📈 Maximizing or minimizing continuous functions can be approached by considering closed or open intervals and applying calculus to find critical points.
- 💰 In real-life scenarios such as business, it's crucial to use calculus to find the minimum cost or maximum profit rather than guessing, which could lead to financial loss.
- 📦 For applied problems like creating the most volume with a given amount of material, calculus helps determine the optimal dimensions, such as for a box made from a square piece of cardboard.
- 📐 Understanding constraints is key in optimization problems; for example, the amount of fencing available limits the area that can be enclosed.
- 🔍 Identifying and applying the correct formula that incorporates all elements of the problem is essential for solving maximization or minimization problems.
- 🤔 To find the maximum or minimum of a function, one must find critical points where the derivative is zero and check endpoints, especially for closed intervals.
- 📏 For a rectangular region with a fixed perimeter, the area is maximized when the rectangle is a square, which is an intuitive result from calculus.
- 🧮 Taking the derivative of the area formula with respect to one variable, after using the constraint to express the other variable, allows for the application of calculus to find maxima or minima.
- 🛠️ In engineering problems like pipeline construction, calculus is used to determine the optimal points to minimize costs, considering different costs for underwater and onshore pipes.
- 📊 Setting up the problem with a clear picture and formulating it correctly is half the solution; the other half is using calculus to find the derivative and solve for critical points.
- 🔗 The process of optimization involves not just finding a solution but also verifying it as a maximum or minimum through methods like the second derivative test or first derivative analysis.
Q & A
What are the two scenarios when dealing with maximizing or minimizing continuous functions?
-The two scenarios are closed finite intervals and open infinite intervals. Closed finite intervals are guaranteed to have an absolute maximum and minimum, while open infinite intervals may not have these points.
How does calculus help in real-life business decisions such as cost minimization?
-Calculus can be used to determine the optimal production levels and costs, allowing businesses to maximize profits and minimize expenses. It provides a precise mathematical approach to decision-making rather than relying on guesswork.
What is the formula for the volume of a rectangular storage box?
-The formula for the volume of a rectangular box is length multiplied by width multiplied by height (V = lwh).
What is a constraint in applied problems?
-A constraint is a limitation or condition that restricts the solution of a problem. In the context of the script, it refers to the amount of fencing available to enclose a rectangular region.
How does the concept of relative maxima and minima apply to continuous functions?
-For continuous functions, absolute maxima and minima must occur at either relative maxima or minima or at endpoints of the function's domain. Relative extrema are points where the derivative is zero or undefined.
What is the process to find the maximum or minimum of a function with a constraint?
-The process involves first solving the constraint for one variable and substituting it into the function to be maximized or minimized. Then, taking the derivative of the resulting function and setting it to zero to find critical points. Finally, evaluating the endpoints and critical points to determine the absolute maximum or minimum.
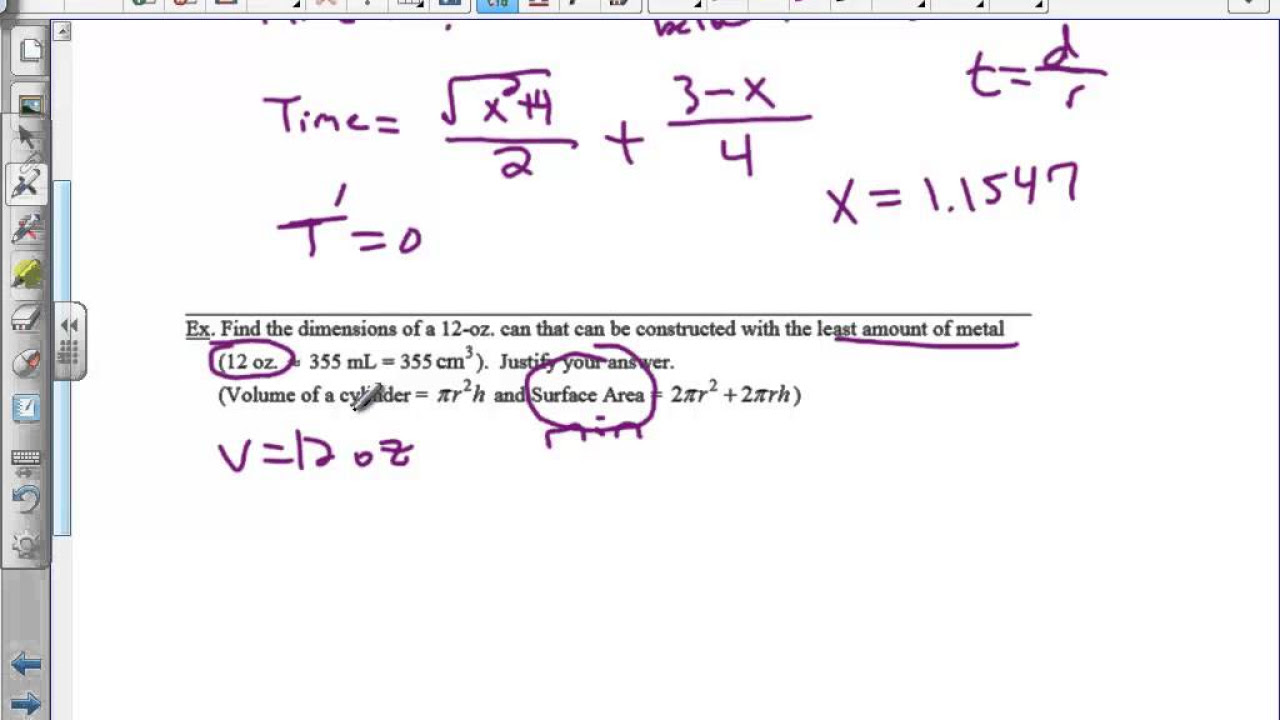
What is the formula for the surface area of a cylinder?
-The surface area of a cylinder is given by the sum of the areas of the two circular bases and the lateral surface area. The formula is A = 2πr² + 2πrh, where r is the radius and h is the height of the cylinder.
What is the volume formula of a cylinder and how is it used as a constraint?
-The volume formula of a cylinder is V = πr²h. It is used as a constraint when the volume of the cylinder is fixed, such as when the cylinder must hold a specific amount of liquid, like 1,000 cubic centimeters.
How does the Pythagorean theorem relate to the problem of minimizing the cost of an underwater pipeline?
-The Pythagorean theorem (a² + b² = c²) is used to calculate the length of the underwater pipeline segment, which is a right triangle's hypotenuse when the distances from the oil source to the shore and from the shore to the refinery are the other two sides.
What is the significance of the first derivative in solving optimization problems?
-The first derivative of a function gives the slope of the function at a particular point. In optimization problems, setting the first derivative to zero helps find critical points where the function may have a local maximum or minimum.
How can you determine if a critical point found by setting the first derivative to zero is a maximum, minimum, or neither?
-To determine the nature of a critical point, one can either use the second derivative test (checking if the second derivative is positive for a minimum or negative for a maximum) or analyze the behavior of the first derivative around the critical point to see if there is a change from increasing to decreasing or vice versa.
Outlines
📚 Introduction to Maximizing and Minimizing Continuous Functions
The paragraph introduces the topic of maximizing and minimizing continuous functions. It discusses two scenarios: closed intervals with finite endpoints and open intervals with infinite endpoints. The real-life application of these concepts is mentioned, particularly in business contexts where minimizing costs or maximizing profits are common goals. The importance of using calculus to determine optimal production quantities and costs is highlighted.
🏗️ Applied Problems and Constraints in Optimization
This section delves into applied problems involving optimization, specifically the example of fencing a rectangular region with limited materials. The concept of a 'constraint' is introduced, which refers to the limitations one must work within when trying to maximize or minimize something. The importance of visualizing problems through drawing and formulating them mathematically is emphasized, along with the strategy of using calculus to find the optimal solution.
🔍 Solving Optimization Problems Step by Step
The paragraph outlines the step-by-step process for solving optimization problems. It begins with solving for one variable using the given constraint and substituting it into the formula for the quantity to be maximized or minimized. The process involves reducing the problem to a single-variable calculus problem, which can then be solved using derivatives to find the critical points where the slope is zero, indicating potential maxima or minima.
🔢 Maximizing Area with a Given Amount of Fencing
The paragraph presents a specific problem of maximizing the area of a rectangular region using a fixed amount of fencing. It demonstrates how to find the dimensions of the rectangle that will maximize the area, given the constraint of 100 feet of fencing. The solution involves finding the critical point where the derivative of the area function with respect to one of the variables is zero and checking this against the endpoints of the domain to determine the absolute maximum.
📦 Maximizing Volume with a Cardboard Box
This section discusses the problem of maximizing the volume of an open box made from a piece of cardboard by cutting out squares from each corner and folding up the sides. The paragraph explains the need to visualize the problem, establish a formula for the volume, and identify constraints inherent in the problem. It emphasizes the importance of taking derivatives to find the critical points that could potentially be the solution to the maximization problem.
🛠️ Derivatives and Critical Points in Optimization
The paragraph focuses on the role of derivatives in finding critical points where the slope of the function is zero, which are potential locations for maxima or minima. It discusses the process of taking the first derivative of a volume function with respect to a variable, setting it equal to zero, and solving for the variable to find possible critical points. The paragraph also touches on the need to check these critical points against the endpoints of the domain to determine the actual maximum or minimum.
🌐 Cost Minimization for Underwater Pipelines
This section explores a real-world application of optimization by discussing the problem of minimizing the cost of building an underwater pipeline to transport oil. It introduces the concept of different costs for laying pipes underwater versus on land and presents a scenario where the goal is to find the optimal point to lay the pipeline to minimize costs. The paragraph outlines the need to formulate a cost function based on the distances involved and the costs associated with each section of the pipeline.
📏 Minimizing Material Usage for a Soda Can
The final paragraph presents the challenge of designing a soda can that holds a liter of soda while minimizing the amount of material used. It discusses the geometric aspects of a cylindrical can and the need to calculate the surface area, which is the material cost in this context. The paragraph emphasizes the importance of understanding the formula for the surface area of a cylinder and how to incorporate the volume constraint into the optimization problem.
Mindmap
Keywords
💡Maximization
💡Minimization
💡Continuous Functions
💡Derivatives
💡Constraints
💡Critical Points
💡End Points
💡Optimization
💡Volume
💡Surface Area
💡Cylinder
Highlights
The discussion focuses on maximizing and minimizing continuous functions, particularly in real-life scenarios such as business cost minimization and profit maximization.
The importance of calculus in determining optimal production quantities and costs is emphasized, as opposed to making guesses without mathematical backing.
The concept of constraints in optimization problems is introduced, showing how they can limit the area or possibilities in which a solution can be found.
An example of maximizing the area of a fenced rectangular region with a fixed amount of fencing material is presented to illustrate the application of calculus in optimization.
The use of derivatives in finding critical points, where the slope of a function is zero, is explained as a method to identify potential maximum or minimum values.
The relationship between absolute maxima and minima, relative maxima and minima, and end points of a function's domain is discussed in the context of closed and open intervals.
An applied problem involving the construction of a box from a piece of cardboard with a given surface area is used to demonstrate the use of calculus in maximizing volume.
The process of solving for one variable using a constraint and substituting it into the area or volume formula to simplify the optimization problem is outlined.
The significance of checking end points and critical points to ensure the identified solution corresponds to an absolute maximum or minimum is highlighted.
An economic application of calculus is shown through the problem of minimizing the cost of an underwater oil pipeline by optimizing the location of an intermediary point.
The use of the Pythagorean theorem in calculating the distances involved in the pipeline optimization problem is demonstrated.
The idea of setting up a cost function based on the distances and costs of different segments of the pipeline is introduced to formulate the optimization problem.
The minimization of the surface area of a cylindrical can to reduce material usage while maintaining a fixed volume is explored as a geometric optimization problem.
The volume of a cylinder is derived and used as a constraint to express the height of the cylinder in terms of the radius, which is then used to minimize the surface area.
The role of the second derivative in confirming the concavity and thus the nature of the critical point as a minimum is explained.
The final solution for the radius and height of the can that minimizes material usage for a fixed volume is presented, along with the interpretation of the results.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: