Business Calculus - More Optimization
TLDRThe video script is a comprehensive tutorial on optimization problems in the context of business calculus. It covers a variety of scenarios where calculus is used to find minimum or maximum values to optimize outcomes, such as minimizing fencing for a picnic park, deriving the cost-effective dimensions of a cylindrical can, determining the optimal pricing strategy for a manufacturer to maximize profit, and finding the most economical route for running a cable. The script also addresses a pollution problem, where the goal is to minimize the total pollutant exposure from two industrial plants, and concludes with a problem on inventory management for a bicycle manufacturer seeking to minimize costs associated with tire orders. Each problem is approached by setting up an equation or function, taking derivatives, and applying calculus concepts to find the optimal solution. The tutorial is designed to be accessible, with clear explanations and step-by-step calculations, making it a valuable resource for those looking to understand the practical applications of calculus in business and real-world scenarios.
Takeaways
- 📏 The video discusses various optimization problems in the context of business calculus, focusing on minimizing costs or maximizing profits through calculus.
- 🚧 For the picnic park problem, the goal is to minimize fencing needed for a rectangular park with a fixed area alongside a highway, leading to dimensions of 100 yards by 50 yards.
- 🧮 In the cylindrical can problem, calculus is used to find the relationship between the radius and height of the can that minimizes construction cost, based on fixed volume and different costs for materials.
- 📈 The profit maximization for video cassettes involves setting up a profit function based on the number of cassettes sold and the price per cassette, with the manufacturer looking to increase prices.
- 🏭 A manufacturer's pricing strategy is influenced by the relationship between price increases and the number of units sold, aiming to find the optimal price point for maximum profit.
- 🏠 The location of a house between two industrial plants is optimized to minimize the total pollution exposure, considering the reciprocal distance decay of pollutants.
- 💰 The cost of running a cable from a power plant to a factory is minimized by calculating the most economical route, balancing the cost of underwater and overland cable laying.
- 🚍 A bus company's revenue is maximized by determining the optimal group size for chartering a bus, based on the number of people and the fare per person.
- 🔄 A bicycle manufacturer minimizes costs by calculating the optimal number of tires to order per shipment, considering ordering fees, storage costs, and purchase costs.
- 📉 The concept of the average number of tires in storage is used to simplify the calculation of storage costs over time, assuming a constant usage rate.
- 🔍 The application of derivatives, specifically the first and second derivatives, is crucial in solving these optimization problems to find maximums, minimums, and the optimal conditions.
Q & A
What is the objective of the highway department in the picnic park optimization problem?
-The objective of the highway department is to determine the dimensions of the rectangular picnic park that would minimize the amount of fencing required while maintaining an area of 5,000 square yards.
What is the formula for the perimeter of the picnic park in terms of its length (x) and width (y)?
-The formula for the perimeter (P) of the picnic park is P = x + 2y, representing the length plus twice the width since the park is rectangular and only three sides need fencing.
How is the area of the picnic park related to its length and width?
-The area (A) of the picnic park is related to its length (x) and width (y) by the formula A = x * y, which in this case is given as 5,000 square yards.
What is the cost function for the cylindrical can in terms of its radius (r) and height (h)?
-The cost function (C) for the cylindrical can is given by C = 6πr^2 + 4πrh, where the first term represents the cost of the top and bottom and the second term represents the cost of the curved side.
How does the cost of the cylindrical can change with respect to its volume (V)?
-The cost of the cylindrical can is minimized when the radius (r) is equal to the cube root of the volume (V) divided by 3π, which ensures the most economical use of material for a given volume.
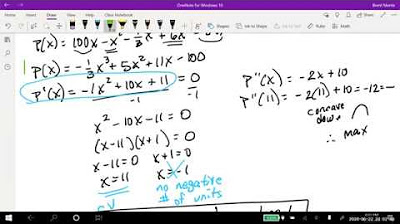
What is the profit function for the video cassette manufacturer in terms of the new price (x)?
-The profit function (P) for the video cassette manufacturer is P = (400 * (15 - x)) * (x - 2), where 400 represents the number of cassettes sold at the initial price minus 400 times the price increase.
What is the relationship between the number of cassettes sold and the price increase for the manufacturer?
-For each $1 increase in the price, 400 fewer cassettes are sold. This relationship is used to model the decrease in sales volume as the price increases.
What is the objective of the optimization problem regarding the location of a house between two industrial plants?
-The objective is to find the optimal location on the road joining the two plants where a house should be built to minimize the total pollutant arriving from both plants.
What is the cost function for running a cable from a power plant to a factory across a river?
-The cost function (C) for running the cable is C(x) = 5 * √(900^2 + x^2) + 4 * (3000 - x), where x is the distance run on land and 3000 - x is the distance under water.
What is the strategy for a bus company to maximize its revenue when charging different prices for different group sizes?
-The bus company should maximize its revenue by determining the size of the group that yields the highest revenue. For groups of exactly 35 people, the fare is $60 per person, and for larger groups, the fare is reduced by $1 for each person over 35.
What is the economic order quantity for a bicycle manufacturer buying tires from a distributor?
-The economic order quantity, which minimizes the total cost, is 500 tires per shipment for the bicycle manufacturer.
Outlines
🔍 Introduction to Optimization Problems
The video begins with an introduction to optimization problems in business calculus. The host explains that the video will cover more challenging problems than the previous one, focusing on how to find the minimum or maximum values in different scenarios, such as fencing requirements for a picnic park alongside a highway with a given area.
📏 Minimizing Fencing for a Picnic Park
The first problem involves calculating the minimum amount of fencing needed for a rectangular picnic park next to a highway. The area is fixed at 5,000 square yards, and the park is to be fenced on three sides. Using calculus, the host derives the dimensions that minimize the perimeter (fencing) required, resulting in a park measuring 100 yards by 50 yards.
🛍️ Cost Minimization for a Cylindrical Can
The second problem is about finding the dimensions of a cylindrical can that will minimize the cost of construction for a fixed volume of liquid. The cost varies for the top and bottom and the curved side. By taking the derivative of the cost function and setting it to zero, the host finds a relationship between the radius and height that minimizes the cost.
🏭 Profit Maximization for Video Cassettes
The third problem discusses how a manufacturer can maximize profit by adjusting the price of video cassettes. With a given cost per cassette and a fixed selling price, the manufacturer estimates a decrease in sales with each price increase. The host uses calculus to find the price that maximizes profit, considering the number of cassettes sold and the profit per cassette.
🏠 Minimizing Pollution Exposure for a House
The fourth problem involves finding the optimal location for a house between two industrial plants that emit pollutants. The concentration of pollutants decreases with the reciprocal of the distance from the plants. The host sets up a function to represent the total pollution at a house and uses calculus to find the position that minimizes the total pollution.
🚧 Economical Routing for a Cable
The fifth problem is about running a cable from a power plant to a factory across a river and downstream. The cost of running the cable under water is different from the cost over land. The host uses the Pythagorean theorem to express the distances and formulates a cost function. By finding the derivative and setting it to zero, the optimal route is determined to minimize cost.
🚍 Maximizing Revenue for Bus Charters
The sixth problem deals with a bus company that wants to maximize revenue by determining the optimal group size for a bus that holds 50 people. The host sets up a revenue function based on the number of people in excess of 35 and finds the number of additional people that maximizes the revenue, considering the different ticket prices.
🔄 Minimizing Cost for Tire Orders
The seventh problem involves a bicycle manufacturer that needs to minimize the total cost of ordering, storing, and purchasing 6,000 tires annually. The host formulates a cost function that includes ordering costs, purchasing costs, and storage costs. By taking the derivative and solving for the optimal number of tires per shipment, the host finds the order quantity that minimizes the total cost.
📚 Conclusion and Further Optimization Problems
The video concludes with a summary of the optimization problems solved and an invitation for viewers to check out more videos with additional optimization problems from business calculus. The host encourages viewers to like, share, subscribe, and comment, and teases more content to come.
Mindmap
Keywords
💡Optimization
💡Derivative
💡Perimeter
💡Area
💡Cost Function
💡Volume
💡Profit
💡Revenue
💡Storage Cost
💡Ordering Cost
💡Purchasing Cost
Highlights
The video introduces optimization problems in the context of business calculus, focusing on more complex scenarios.
A problem involving the construction of a picnic park along a highway is presented to minimize fencing costs.
The solution to the picnic park problem involves calculating the dimensions of the park to require the least amount of fencing.
A cylindrical can construction problem is discussed, aiming to find the relationship between the radius and height for the least costly can.
The cost function for the can is derived using calculus, considering material costs for the top, bottom, and curved side.
The manufacturer's pricing strategy for video cassettes is analyzed to maximize profit, considering the impact of price on sales volume.
The concept of marginal profit is applied to find the optimal price point for selling cassettes.
A pollution problem is tackled to determine the optimal location for a house to minimize exposure to pollutants from two industrial plants.
The reciprocal of distance is used as a factor in calculating the concentration of pollutants from each plant.
The Pythagorean theorem is applied to find the optimal route for running a cable from a power plant to a factory across a river.
The cost function for the cable routing problem includes variables for the cost of running cable under water and over land.
A bus company's revenue maximization problem is solved by calculating the optimal group size for a bus charter service.
The concept of marginal revenue is used to determine the number of people in a group that yields the highest revenue.
A bicycle manufacturer's inventory optimization problem is presented, focusing on minimizing the total cost of tire orders.
The economic order quantity model is used to find the optimal number of tires to order in each shipment.
The video concludes with a summary of the optimization problems and a teaser for more problems in subsequent videos.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: