Continuous Money Flow: Finding Revenue Stream when Future Value is Known

Sun Surfer Math
4 May 202204:44
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a financial problem involving the future value of a continuous money flow, known as a 'working backwards' problem. The goal is to determine the initial investment needed to reach a future value of $50,000 in three years, with an interest rate of 5%. The script guides through setting up the problem using the future value formula, integrating with a constant income stream 'a', and applying u-substitution to solve for 'a'. The process involves evaluating the integral from 0 to 3, solving for 'a', and then verifying the solution by calculating the future value of the continuous income stream. The result indicates an initial investment of approximately $15,447 to achieve the desired future value, providing a clear and engaging explanation of the mathematical process.

Takeaways
  • 🚗 The goal is to calculate the initial investment needed for a future value of $50,000 in 3 years for a car purchase.
  • 📈 The future value (FV) is calculated using the formula for continuous compounding: FV = ∫(a * e^(r*t) dt) from 'a' to 'b'.
  • 🔍 The interest rate (r) is given as 5%, and the time period (t) is 3 years.
  • 💡 The problem is approached by working backwards from the known future value to find the unknown continuous income stream (a).
  • 📊 The revenue stream is assumed to be constant, so 'a' is used to represent the constant deposit amount.
  • ✅ The integration is performed using u-substitution with u = e^(0.05t) and du = 0.05e^(0.05t) dt.
  • 🧮 After integration, the equation is simplified to solve for 'a', the initial deposit amount.
  • 📉 The equation is simplified to: 50,000 = a * (e^(0.15) - 1) / 0.05.
  • 🔢 By solving for 'a', the continuous income stream required is calculated to be approximately $15,447.
  • 🔄 To verify, the future value of the continuous income stream (15,447 * e^(0.05t) dt) from 0 to 3 is calculated and should equal $50,000.
  • 📝 The process demonstrates how to work backwards from a future value to find the required continuous investment over a given period.
Q & A

  • What is the future value formula used in the problem?

    -The future value formula used is the integral from 'a' to 'b' of the income stream or revenue stream, times e to the r t, dt.

  • What is the future value the individual is aiming for?

    -The future value is $50,000, which is the amount the individual wants to have in three years to buy a new car.

  • What is the interest rate for the investment?

    -The interest rate for the investment is 5 percent.

  • What does 'r sub t' represent in the context of the problem?

    -'r sub t' represents the unknown rate of the continuous income stream that needs to be determined.

  • How is the constant income stream assumed to behave in the problem?

    -The constant income stream is assumed to remain constant, not increasing over time.

  • What is the variable 'a' used to represent in the problem?

    -The variable 'a' is used to represent the constant income stream that is being deposited continuously over the time period.

  • What is the method used to integrate the future value formula?

    -The method used to integrate the future value formula is u-substitution.

  • What is the result of evaluating the integral from 3 to 0?

    -The result is e to the point zero five times three, minus e to the point zero five times zero, which simplifies to e^(0.15) - 1.

  • How is 'a' solved for in the equation?

    -To solve for 'a', both sides of the equation are multiplied by 0.05, and then 'a' is divided by e to the point one five minus one.

  • What is the calculated continuous income stream over the next three years?

    -The calculated continuous income stream is approximately $15,447.

  • How can the result be checked for accuracy?

    -The result can be checked by calculating the future value from 0 to 3 of a revenue stream of $15,447 times e to the point zero five t, dt, which should equal $50,000.

  • What is the significance of working backwards in this problem?

    -Working backwards allows the individual to determine the necessary continuous income stream to achieve a specific future value, given the interest rate and time period.

Outlines
00:00
🚗 Calculating Continuous Investment for a Future Car Purchase

The video script introduces a problem-solving approach for determining the continuous investment needed to reach a future value, specifically for purchasing a car. It outlines the use of the future value formula, which is an integral of an income stream multiplied by e^(rt) from time 'a' to 'b'. The scenario involves a future value of $50,000 for a car to be purchased in three years with an interest rate of 5%. The challenge is to find the constant deposit 'a' that needs to be invested continuously. The script guides through the steps of setting up the integral with the known future value, assuming a constant income stream, and performing u-substitution for integration. The final calculation results in a continuous income stream of approximately $15,447 to be invested over three years to achieve the desired future value. The script concludes with a verification step, showing that the calculated income stream, when invested continuously, indeed results in the future value of $50,000.

Mindmap
Keywords
💡Future Value
Future Value refers to the value of an asset or cash at a future date, based on an assumed rate of return. In the video, it is used to calculate the amount of money needed to accumulate to buy a car worth $50,000 in three years, which is the main goal of the problem discussed.
💡Continuous Money Flow
Continuous Money Flow describes a constant and uninterrupted stream of money being invested or deposited over a period of time. In the context of the video, it is the method of investment where money is deposited continuously at a rate 'r sub t', which is essential to find out to reach the future value.
💡Interest Rate
The Interest Rate is the percentage at which interest is paid by a borrower or earned by an investor for a loan or deposit of money. In the script, the interest rate is given as 5%, which is used to calculate the future value of the continuous money flow.
💡Integral
In mathematics, an Integral represents the area under a curve defined by a function, which in this video's context, is used to calculate the total amount of money accumulated over time from a continuous income stream. The integral formula is central to the problem-solving process described in the video.
💡Revenue Stream
A Revenue Stream is a source of income or the inflow of money from a particular business activity. In the video, the revenue stream is represented by a constant 'a', which is the continuous income deposited to reach the future value.
💡Working Backwards
Working Backwards is a problem-solving approach where one starts with the final goal and works towards the initial conditions. The video presents a 'working backwards problem' where the future value is known, and the goal is to find the initial investment rate.
💡U Substitution
U Substitution is a method used in calculus to evaluate integrals by substituting a new variable u for a function of the original variable. In the video, u substitution is used to simplify the integral and solve for the unknown continuous income stream 'a'.
💡e to the rt
e to the rt (where e is the base of the natural logarithm and r is the interest rate) is a mathematical expression used in the context of continuous compounding. In the video, it represents the exponential growth of the investment over time 't' at an interest rate 'r'.
💡Exponential Function
An Exponential Function is a mathematical function of the form y = a * b^x, where the variable x appears as an exponent. In the video, the function e^(.05t) is an example of an exponential function, showing how the investment grows exponentially over time.
💡Integration
Integration is a fundamental process in calculus that finds the accumulated value of a function over an interval. It is used in the video to find the total amount of money that needs to be invested continuously to achieve the future value of $50,000.
💡Checking the Solution
Checking the Solution involves verifying the correctness of the solution by re-evaluating the problem or using a different method. In the video, the presenter suggests recalculating the future value using the found continuous income stream to ensure it equals the desired future value of $50,000.
Highlights

The problem involves calculating the future value of a continuous money flow.

The future value formula is integral from a to b of the income stream times e to the r t, dt.

The future value is known to be $50,000 for a car to be bought in three years.

The investment has a 5% interest rate.

The goal is to find the total amount needed to invest with continuous deposits over the time period.

The variable r sub t is to be determined, representing the income stream.

An assumption is made that the income stream is constant and does not change over time.

The revenue stream is represented as a constant 'a' multiplied by e to the .05 t dt.

Integration is performed using u substitution with a divided by .05 as the substitution variable.

The evaluation of the integral results in an equation with 50,000 as the future value.

Solving for 'a' involves multiplying both sides by .05 and dividing by e to the .15 minus 1.

The continuous income stream required over three years is calculated to be approximately $15,447.

To verify the result, the future value of the revenue stream from 0 to 3 years is recalculated.

The recalculated future value matches the initial known future value of $50,000.

The problem demonstrates a method of working backwards from a known future value to determine the required investment.

The mathematical process involves setting up the integral, making assumptions, performing integration, and solving for the unknown.

The transcript provides a step-by-step guide to solving a continuous money flow problem.

The use of exponential functions and integration is key to finding the solution.

The final answer confirms the practical application of mathematical formulas in financial planning.

Transcripts

Browse More Related Video

Continuous Money Flow: Future Value with increasing rate of growth

Continuous Money Flow: Present Value Problems

MATH 1325 13 4 Lecture

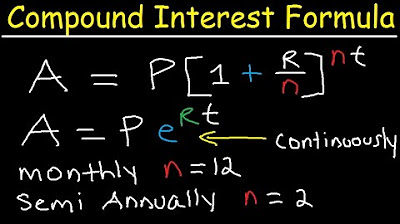
Compound Interest Formula Explained, Investment, Monthly & Continuously, Word Problems, Algebra

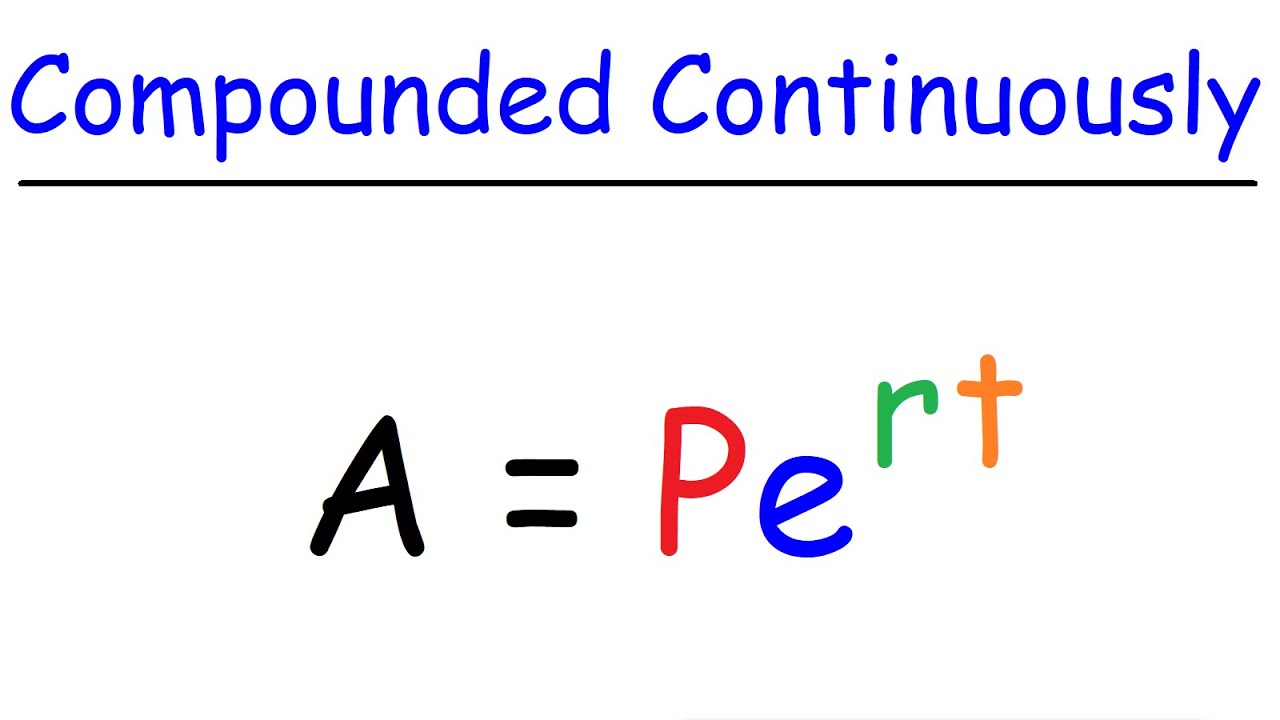
Interest Compounded Continuously

Compound Interest & Population Growth Word Problems - Logarithms

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Future ValueContinuous FlowInvestmentInterest RateIntegral CalculusFinancial PlanningMoney ManagementRetirement SavingsCompound InterestWorking BackwardsFinancial Goal