Speed, Velocity and Distance Time Graphs - Physics - Science - Get That C In your GCSE and IGCSE

Christopher Thornton
17 Sept 201305:06
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, Mr. Thornton introduces the concepts of speed and velocity, emphasizing their calculation through the formula speed = distance / time. He clarifies the difference between speed (a scalar quantity) and velocity (a vector quantity), highlighting that velocity includes direction. The video further explains how to interpret distance-time graphs, identifying constant velocity, stopped objects, and reversing motion through line slopes and directions. This lesson is tailored to help GCSE students understand and apply these fundamental physics concepts.

Takeaways
  • 📚 The lesson focuses on calculating speed and velocity, and representing them on a distance-time graph.
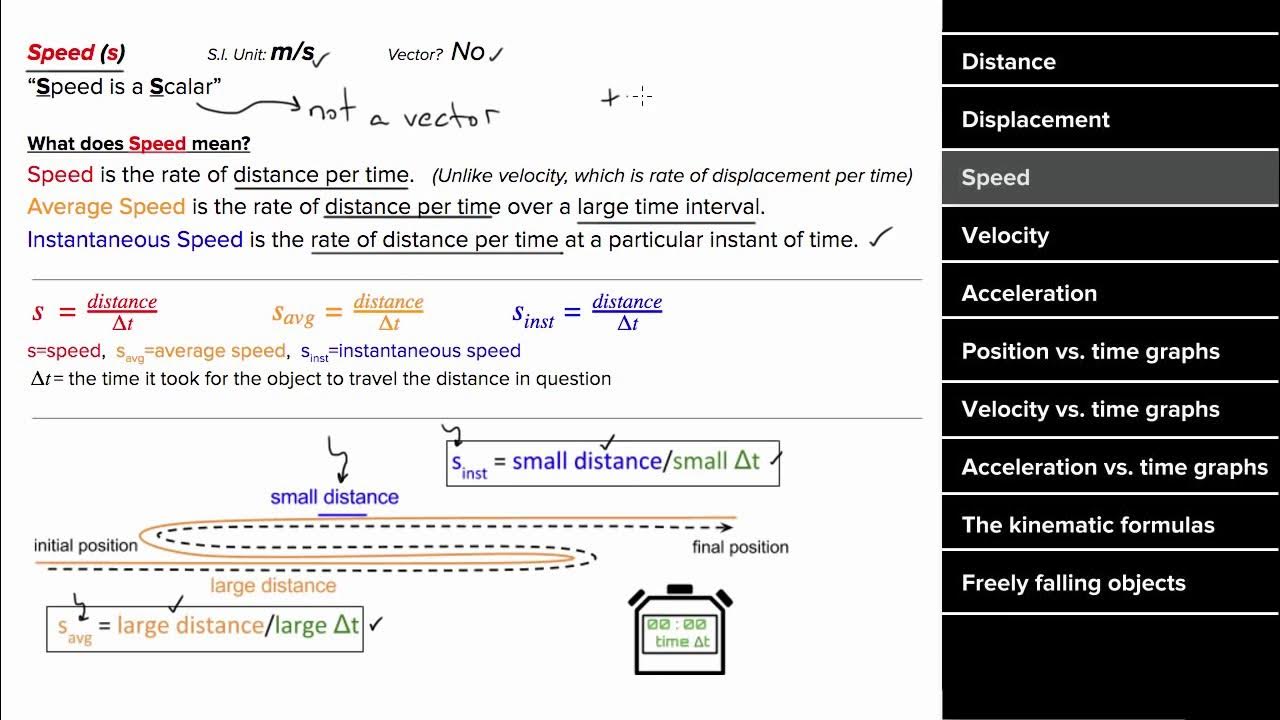
  • 🔢 Speed is calculated using the formula: speed = distance / time, which is a fundamental equation in GCSE.
  • 📱 Students are allowed to use a basic calculator during the GCSE exam for these calculations.
  • 🏃‍♂️ An example given is a 100-meter sprinter running the distance in 10 seconds, resulting in a speed of 10 meters per second.
  • 📌 Velocity is similar to speed but includes direction, making it a vector quantity.
  • ➡️ Velocity with a positive direction is represented by a positive number, while a negative direction is represented by a negative number.
  • 📈 A distance-time graph shows the progression of an object's distance from its starting point over time.
  • 🌟 A straight line in the graph indicates constant velocity, with the slope representing the speed.
  • 🚫 A horizontal line signifies that the object has stopped, with the speed being zero.
  • 🔄 A downward sloping line in the graph indicates the object is moving backward, representing negative velocity.
  • 👍 The video encourages students to engage with the content, like, share, and subscribe for more educational material.
Q & A

  • What is the main topic of the lesson?

    -The main topic of the lesson is to calculate speed and velocity and represent them on a distance-time graph.

  • What is the formula for calculating speed?

    -The formula for calculating speed is speed equals distance divided by time.

  • Is a calculator allowed in the GCSE exam for this topic?

    -Yes, a basic calculator is allowed in the GCSE exam for this topic.

  • How is velocity different from speed?

    -Velocity is different from speed in that velocity has direction, whereas speed does not.

  • What does a straight line on a distance-time graph indicate?

    -A straight line on a distance-time graph indicates that an object is moving at a constant velocity.

  • What does the gradient of a line on a distance-time graph represent?

    -The gradient of a line on a distance-time graph represents the velocity of the object. A steeper gradient indicates a higher velocity.

  • What does a horizontal line on a distance-time graph mean?

    -A horizontal line on a distance-time graph means that the object has stopped, as there is no change in distance over time.

  • What is indicated by a line sloping downwards on a distance-time graph?

    -A line sloping downwards on a distance-time graph indicates that the object is moving backwards, which is referred to as negative velocity.

  • How can you determine if an object is moving forward or backward from a distance-time graph?

    -An object is determined to be moving forward if the velocity is a positive number and backward if the velocity is a negative number.

  • What is the unit of measurement for both speed and velocity?

    -The unit of measurement for both speed and velocity is meters per second.

  • What should one do if they find this video useful for their GCSE studies?

    -If one finds the video useful, they should like it, share it, and subscribe for more content.

Outlines
00:00
📚 Introduction to Speed and Velocity Calculation

This paragraph introduces the concept of calculating speed and velocity, emphasizing their importance in GCSE examinations. Mr. Thornton explains the formula for speed (distance divided by time) and its application in a basic example involving a 100-meter sprinter. The distinction between speed and velocity is clarified, with velocity being described as speed with a direction. The paragraph also highlights the significance of understanding and using units correctly. Furthermore, the importance of using a calculator during the exam is mentioned, ensuring students are well-prepared for their assessments.

05:02
📈 Understanding Distance-Time Graphs

This paragraph delves into the interpretation of distance-time graphs, which are essential for comprehending the motion of objects as represented by their velocity. Mr. Thornton explains the significance of the slope in these graphs, where a constant slope indicates a constant velocity, and varying slopes reflect changes in speed. The paragraph also describes how to determine if an object is moving forward (positive velocity), backward (negative velocity), or has come to a stop (zero velocity). The explanation is supported by examples of different gradients on the graph, providing students with a clear understanding of how to analyze and interpret distance-time graphs in the context of motion and velocity.

Mindmap
Keywords
💡GCSE
GCSE stands for General Certificate of Secondary Education, which is a set of examinations taken by students in the United Kingdom. In the context of this video, Mr. Thornton is addressing students preparing for their GCSE exams, specifically focusing on the subject of physics and the concepts of speed and velocity.
💡Speed
Speed is a physical quantity that describes how fast an object is moving. It is calculated by dividing the distance traveled by the time taken to travel that distance. In the video, Mr. Thornton emphasizes the importance of understanding this basic equation: speed = distance / time, which is fundamental to the study of motion in GCSE physics.
💡Velocity
Velocity is similar to speed but includes the direction of motion. It is also calculated by dividing distance by time, but the result is a vector quantity that indicates both the rate and the direction of motion. Positive velocity indicates forward motion, while negative velocity indicates backward motion.
💡Distance-Time Graph
A distance-time graph is a graphical representation that shows the relationship between the distance an object has traveled and the time it has taken to travel that distance. The vertical axis represents distance, and the horizontal axis represents time. The slope of the line on the graph indicates the velocity of the object.
💡Calculator
A calculator is an electronic device used to perform mathematical calculations. In the context of the GCSE exam, students are allowed to bring a basic calculator into the examination hall to assist with calculations, including those related to speed and velocity.
💡Units
Units are standardized measurements used to express the magnitude of physical quantities. In the context of speed and velocity, the standard unit is meters per second (m/s). Understanding and using correct units is crucial in physics to ensure accurate calculations and interpretations.
💡Constant Velocity
Constant velocity refers to the state of an object moving at a steady, unchanging speed in a straight line. This is represented on a distance-time graph as a straight line with a constant slope, indicating that the object's speed does not vary over time.
💡Gradient
Gradient, in the context of a distance-time graph, refers to the slope of the line representing the motion of an object. A positive gradient indicates that the object is moving forward and accelerating, while a negative gradient indicates that the object is moving backward. A zero gradient means the object is at rest or moving at a constant speed.
💡Physics
Physics is the natural science that studies matter, its motion, and the forces that act upon it. In this video, physics is the subject being taught, with a focus on the concepts of speed and velocity, which are fundamental to understanding motion in the field of physics.
💡Motion
Motion refers to the change in position of an object. It is a fundamental concept in physics and is described by various quantities such as speed, velocity, and acceleration. Understanding motion is essential for solving problems related to the movement of objects.
💡Direction
Direction refers to the path or way in which something moves or is aimed. In physics, direction is a crucial aspect of velocity, as it not only considers the rate of motion (speed) but also the specific path the object is taking.
Highlights

Introduction to Mr. Thornton and the goal of achieving a C grade in GCSE.

Calculation of speed and velocity as the key topics for this lesson.

Emphasis on the use of distance-time graphs instead of velocity-time graphs for this lesson.

Explanation of the speed equation (distance divided by time) and its importance in GCSE.

Advice on being allowed to use a calculator in the GCSE exam.

Simple example calculation of speed for a 100-meter sprinter.

Explanation of the units for speed (meters per second) and their importance.

Difference between speed and velocity, highlighting that velocity includes direction.

Description of how to interpret a distance-time graph, focusing on the meaning of slopes and gradients.

Illustration of constant velocity with a straight line on the distance-time graph.

Explanation of varying velocities with different slopes on the graph.

Identification of when an object is stopped on the graph (zero gradient).

Explanation of negative velocity as represented by a line sloping downwards on the graph.

Definition of positive and negative gradients on a distance-time graph.

Summary of the four types of motion (constant velocity, faster constant velocity, stopped, and reversing) on the graph.

Encouragement for GCSE students and a call to like, share, and subscribe for more content.

Transcripts

Browse More Related Video

AP Physics 1 review of 1D motion

What Are Speed and Velocity? | Physics in Motion

Velocity and speed: Motion in One Dimension

What is Velocity? Physics

Distance, Displacement, Speed and Velocity

Distance vs. Displacement & Speed vs. Velocity | Kinematics Explained

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics EducationSpeed CalculationVelocity DirectionGCSE ScienceMathematics ApplicationDistance-Time GraphsEducational VideoInteractive LearningPhysics ConceptsMr. Thornton