Distance, Displacement, Speed and Velocity
TLDRThis lecture introduces fundamental concepts of kinematics, differentiating between distance and displacement, and speed and velocity. It explains that distance is the total path length traveled, while displacement is the shortest directed path. Speed is a scalar quantity, measuring the rate of distance covered over time, whereas velocity is a vector quantity, accounting for both rate and direction. The lecture also covers numerical problems involving these parameters, emphasizing their importance in understanding motion and providing examples to illustrate the concepts.
Takeaways
- 📏 Distance is the total path covered by a body in a given time and is a scalar quantity, denoted by letters like s, x, m, with the unit meter.
- 🔜 Displacement is the shortest and directed path traveled by a body, and is a vector quantity, denoted by s arrow, x arrow, rm arrow, with the unit meter.
- 🚗 The difference between distance and displacement is that distance has no direction (scalar) while displacement has a specific direction (vector).
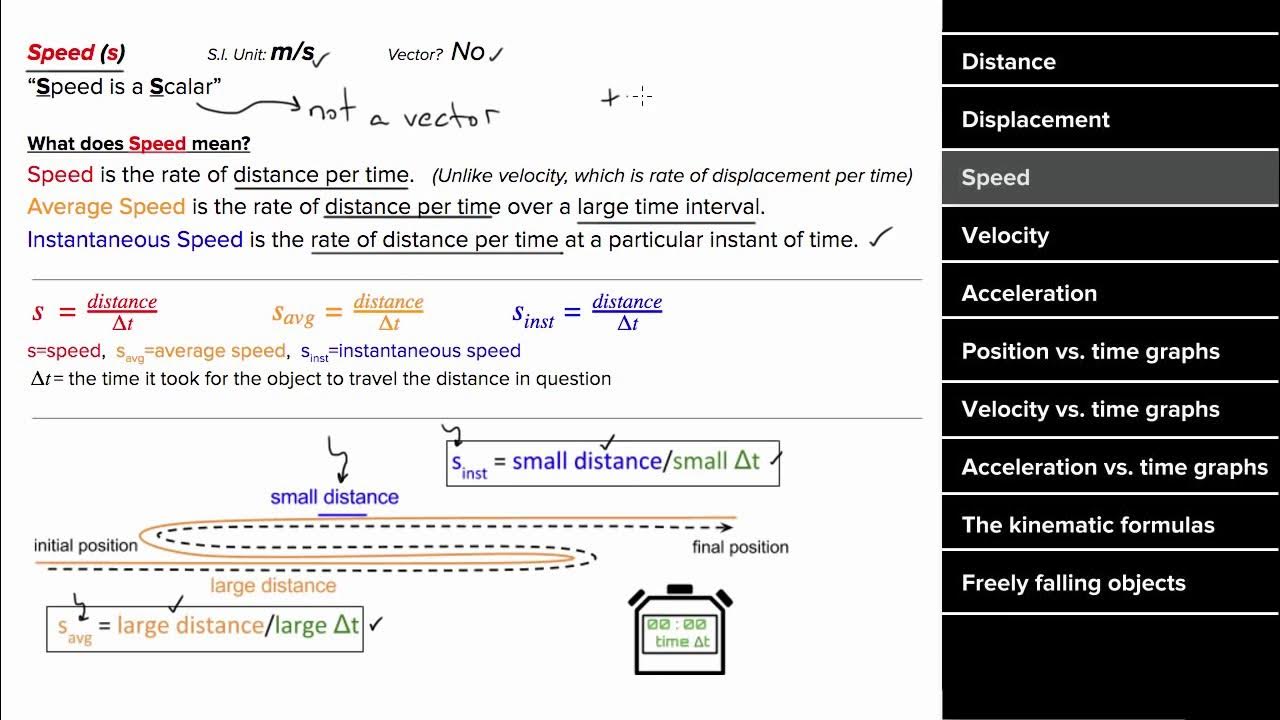
- 🏃 Speed is the rate at which an object covers distance, calculated as distance divided by time, and is a scalar quantity, denoted by v, with the unit meter per second.
- 🏁 Velocity is the rate at which an object covers displacement, calculated as displacement divided by time, and is a vector quantity, denoted by v arrow, with the unit meter per second in a specific direction.
- 🔄 When an object moves in a closed path, its distance is equal to the perimeter of the path, but its displacement is zero if the initial and final positions are the same.
- 📐 For numerical problems, understanding the perimeter of different shapes (circle, rectangle, triangle, etc.) is key to calculating distance, displacement, speed, and velocity.
- 🚗🕒 In calculating a car's speed and velocity, use the formula for the perimeter or distance traveled and divide by the time taken, and specify the direction for velocity.
- 🏊♂️🔢 For an object moving in multiple directions, use the Pythagorean theorem to calculate the resultant displacement and then find the velocity.
- 📌 It's important to note that displacement can be positive or negative indicating direction, but distance is always a positive value.
- 📈 Understanding the concepts of distance, displacement, speed, and velocity is crucial for solving various kinematic problems in physics.
Q & A
What is the basic concept of distance?
-Distance is defined as the total path covered by a body in a given time. It is a scalar quantity represented by letters like s, x, or m, and its unit is meter.
How is displacement different from distance?
-Displacement is the shortest and directed path traveled by a body. Unlike distance, displacement is a vector quantity, which means it has both magnitude and direction. It is represented by s arrow, x arrow, or rm arrow.
Why is distance considered a scalar quantity?
-Distance is considered a scalar quantity because it only has magnitude and no specific direction. It represents the total path length traveled, regardless of the route taken.
What is the definition of speed?
-Speed is defined as the rate at which an object covers distance. It is calculated as the distance divided by time and is represented by the symbol v. The unit of speed is meters per second.
How is velocity different from speed?
-Velocity is defined as the rate at which an object covers displacement. Unlike speed, which is a scalar quantity, velocity is a vector quantity because it includes both magnitude and direction. It is represented by v arrow and has units of meters per second in a proper direction.
Why do we need to study both speed and velocity?
-We study both speed and velocity because while speed tells us how fast an object is moving, velocity provides additional information about the direction of motion. This is crucial for accurately describing and analyzing motion in physics.
What is the perimeter of a circle?
-The perimeter of a circle, also known as the circumference, is given by the formula 2πr, where r is the radius of the circle.
If someone walks around a circle and returns to the starting point, what is their displacement?
-If someone walks around a circle and returns to the starting point, their initial and final positions are the same. Therefore, their displacement is zero, as displacement measures the change in position.
How can you calculate the speed and velocity of a car traveling along the perimeter of a semi-circle?
-To calculate the speed and velocity of a car traveling along the perimeter of a semi-circle, first determine the distance (half the circumference of the circle, which is πr) and the time taken. Speed is the distance divided by time. For velocity, consider the direction of travel, which in this case is along the semi-circle's perimeter.
What is the significance of the Pythagorean theorem in calculating the displacement of an object moving in two directions?
-The Pythagorean theorem is used to calculate the resultant displacement when an object moves in two perpendicular directions. It helps determine the hypotenuse (displacement) using the formula √(base² + perpendicular²), which represents the straight-line distance from the starting point to the final position.
How can you describe the velocity of a ball that traveled 4 meters east and 3 meters north in 2 seconds?
-To describe the velocity of the ball, calculate the magnitude of the displacement using the Pythagorean theorem (√(4² + 3²) = 5 meters) and determine the direction (eastward and northward components). The velocity is then the displacement divided by time, which is 5 meters in 2 seconds, resulting in a velocity of 2.5 meters per second.
Outlines
📏 Understanding Distance and Displacement
This paragraph introduces the fundamental concepts of distance and displacement in the context of kinematics. It explains that distance is the total path covered by an object in a given time, represented by scalar quantities such as s, x, or m, with meters as the unit. In contrast, displacement is defined as the shortest and directed path between two points, represented as a vector quantity with symbols like s arrow or x arrow rm, also having meters as its unit. The key distinction between the two is that distance lacks direction, making it a scalar quantity, while displacement includes direction, making it a vector quantity. The lecture emphasizes the importance of understanding these differences, using examples of individuals traveling between two houses via different routes to illustrate the concepts.
🚗 Distinguishing Speed and Velocity
This paragraph delves into the concepts of speed and velocity. Speed is described as the rate at which an object covers distance, calculated as distance divided by time, and is represented by the scalar quantity v. Its unit is meters per second. Velocity, on the other hand, is the rate at which an object covers displacement and is calculated as displacement divided by time, represented by the vector quantity v arrow. The key difference between speed and velocity is that speed only indicates how fast an object is moving, while velocity provides both the rate and the direction of motion. The lecture uses hypothetical examples of cars moving at different speeds to highlight the significance of velocity in conveying the direction of motion.
📐 Numerical Problems and Applications
The final paragraph focuses on applying the concepts of distance, displacement, speed, and velocity to solve numerical problems. It begins with a discussion on calculating the perimeters of various shapes, such as circles, rectangles, and triangles, and how these perimeters relate to distance in closed paths. The lecture then moves on to practical examples, including a person walking around a circle and a car traveling along a semi-circle, to demonstrate how to calculate distance, displacement, speed, and velocity in real-world scenarios. It concludes with a problem involving a ball traveling in two perpendicular directions, illustrating how to use the Pythagorean theorem to find the resultant displacement and subsequently the velocity. This section aims to reinforce the understanding of the concepts and their practical applications.
Mindmap
Keywords
💡distance
💡displacement
💡speed
💡velocity
💡scalar quantity
💡vector quantity
💡perimeter
💡circumference
💡numerical problems
💡pythagorean theorem
Highlights
The basic concept of distance and displacement is introduced, explaining the difference between the total path covered and the shortest directed path.
Distance is defined as the total path covered by a body in a given time and is represented by scalar quantities such as s, x, m, etc.
Displacement is defined as the shortest and directed path traveled by a body, represented by vector quantities like s arrow, x arrow, rm arrow.
The distinction between scalar and vector quantities is clarified, with distance being a scalar and displacement being a vector.
The reason why distance is a scalar quantity is explained, emphasizing the lack of direction in the total path covered.
The reason why displacement is a vector quantity is detailed, highlighting the constant direction of the shortest path.
Speed and velocity are defined, with speed being the rate at which an object covers distance and velocity being the rate at which an object covers displacement.
The formula for speed is given as v = s / t, with the unit being meters per second, indicating it's a scalar quantity.
The formula for velocity is provided as v arrow = s arrow / t, with the unit being meters per second in a proper direction, confirming it's a vector quantity.
The importance of studying speed and velocity is discussed, emphasizing that while speed shows how fast an object is moving, velocity includes direction.
A numerical problem involving a person walking around a circle is used to demonstrate the calculation of distance, displacement, speed, and velocity.
The concept of perimeter is introduced, with examples given for circle, rectangle, and triangle perimeters.
An exam question is presented, calculating a car's speed and velocity after traveling a semi-circle in one minute.
The calculation of a ball's velocity after traveling in eastern and northern directions for 2 seconds is explained using Pythagoras' theorem.
The significance of understanding the difference between distance, displacement, speed, and velocity is emphasized for practical problem-solving.
The use of diagrams and real-world examples helps to clarify abstract concepts, making the lecture engaging and informative.
The lecture provides a comprehensive understanding of kinematics parameters, beneficial for students and those interested in physics.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: