4. Binding Energy, the Semi-Empirical Liquid Drop Nuclear Model, and Mass Parabolas
TLDRThe lecture delves into the technical aspects of boron neutron capture therapy and nuclear reactions, focusing on the calculation of Q values, which indicate whether a reaction is exothermic or endothermic. It explains the use of the Q equation, the significance of kinetic and binding energies, and the concept of mass-energy conservation. The discussion progresses to cover the semi-empirical mass formula, which predicts the binding energy of a nucleus based on its proton and neutron numbers. The formula's success in predicting stability trends and the concept of magic numbers are highlighted. The lecture also touches on decay processes like beta decay and electron capture, illustrating how nuclei transition to more stable states.
Takeaways
- 📚 The lecture begins with a review of the boron neutron capture therapy (BNCT) reaction, highlighting the importance of understanding the Q value and the conservation of mass and energy in nuclear reactions.
- ⚛️ Boron-10 captures a neutron to become lithium-7 and helium-4, releasing energy in the form of kinetic energy of recoil products and gamma rays.
- 🔧 The Q equation is essential for determining whether a nuclear reaction is exothermic (releasing energy) or endothermic (absorbing energy), with Q > 0 indicating exothermic reactions.
- 🧮 The Q value can be calculated using the masses of the nuclei involved, their kinetic energies, or the binding energies, with the latter being the most straightforward method when values are given in MeV or keV.
- 💡 The binding energy of a nucleus is a measure of its stability, with higher binding energies indicating greater stability and a larger mass-to-energy conversion.
- 🧬 The concept of excess mass and its relation to the binding energy is introduced, emphasizing that excess mass does not have a physical significance but is useful for calculations.
- 📉 The lecture discusses the semi-empirical mass formula, which is a model that predicts the binding energy of a nucleus based on its number of protons and neutrons, accounting for volume, surface area, asymmetry, and pairing effects.
- 📊 A mass parabola is a graphical representation used to visualize the stability of nuclei with a given mass number (A) by plotting the mass as a function of the number of protons (Z).
- ✨ The most stable nucleus for a given mass number can be found by differentiating the mass formula with respect to the number of protons and setting it to zero, which gives the most tightly bound nucleus.
- ⚡ The stability of a nucleus can also be inferred from its position on the mass parabola, with the lowest point on the curve representing the most stable isotope for that mass number.
- 🚫 Certain elements, such as technetium and promethium, do not have any stable isotopes, which is reflected in the semi-empirical mass formula's predictions and can be observed in the chart of nuclides.
- 🔬 The lecture concludes with a discussion on decay modes, such as beta decay and electron capture, which are processes by which unstable nuclei can move towards a more stable state by changing their proton or neutron numbers.
Q & A
What is the focus of the lecture from the provided transcript?
-The focus of the lecture is on boron neutron capture therapy and the technical aspects related to nuclear reactions, specifically the calculation of Q values.
How does the reaction of boron-10 capturing a neutron result in energy release?
-When boron-10 captures a neutron, it transforms into lithium-7 and helium-4, releasing a gamma ray and energy in the form of kinetic energy of the recoil products.
What is the Q equation used for in the context of nuclear reactions?
-The Q equation is used to determine the energy change during a nuclear reaction, indicating whether the reaction is exothermic (releases energy) or endothermic (absorbs energy).
How can the Q value of a nuclear reaction be calculated?
-The Q value can be calculated using the masses of the nuclides involved (in atomic mass units, then multiplied by c squared), the difference in kinetic energies, or the binding energies (already given in MeV or keV) of the nuclides.
What is the significance of the binding energy in determining the stability of a nucleus?
-Binding energy is directly related to the stability of a nucleus. A higher binding energy indicates a more stable nucleus, as it would require more energy to break the nucleus apart.
How does the semi-empirical mass formula help in predicting the binding energy of a nucleus?
-The semi-empirical mass formula provides a universal approach to estimate the binding energy of a nucleus based on its number of protons and neutrons, taking into account factors like volume, surface area, Coulombic repulsion, asymmetry, and pairing terms.
What are 'magic numbers' in the context of nuclear physics?
-Magic numbers refer to specific numbers of nucleons (protons and neutrons) that result in a nucleus with exceptionally high stability, due to the complete filling of energy levels at a certain level.
Why are even-numbered nuclei generally more stable than odd-numbered ones?
-Even-numbered nuclei tend to have more stable configurations because they can achieve greater pairing stability with an equal number of protons and neutrons, which is reflected in the semi-empirical mass formula.
What is the relationship between the mass of a nucleus and its stability?
-The mass of a nucleus is related to its stability through the concept of binding energy. A nucleus with a lower mass (for a given atomic number) is more stable because it has a higher binding energy, indicating a more tightly bound system.
How can one determine the most stable isotope for a given mass number?
-To find the most stable isotope for a given mass number (A), one can use the semi-empirical mass formula and take the derivative with respect to the atomic number (Z), then set it equal to zero and solve for Z, which will give the most stable number of protons for that mass number.
Outlines
📚 Introduction to Nuclear Reactions and Q-Value
This paragraph introduces the concept of nuclear reactions, specifically focusing on boron neutron capture therapy. It explains the process of a boron-10 nucleus capturing a neutron and transforming into lithium-7 and helium-4, releasing a gamma ray and recoil products with kinetic energy. The Q-value, representing the energy change in the reaction, is discussed, with emphasis on its significance in determining whether a reaction is exothermic or endothermic. The equation for calculating Q-value is provided, highlighting the importance of mass and kinetic energy conservation in nuclear reactions.
🔢 Methods for Calculating Q-Value in Nuclear Reactions
This section delves into the three methods for calculating the Q-value in nuclear reactions: using masses in atomic mass units (amu), differences in kinetic energies, and binding energies. The discussion emphasizes the convenience of using binding energies, as they are readily available in MeV or keV. The process of calculating the Q-value for a specific reaction involving boron-10, lithium-7, and helium-4 is worked out as an example. The distribution of kinetic energies between the products is also explored, considering the conservation of momentum and energy principles.
🔄 Conservation Laws in Nuclear Reactions
The importance of conservation laws in nuclear reactions is highlighted, focusing on the conservation of mass-energy and momentum. The relationship between the kinetic energies of the reaction products and their masses is discussed, with an example illustrating how to calculate the distribution of kinetic energy between two products. The concept of alpha decay is introduced, explaining the energy release and the kinetic energy of the decay products, thorium and helium nuclei, and how they relate to the conservation principles.
📈 Understanding Binding Energy and Nuclear Stability
This paragraph introduces the concept of binding energy per nucleon and its significance in understanding nuclear stability. The semi-empirical mass formula is presented as a tool for predicting the binding energy of a nucleus. The formula's components, representing volume, surface area, Coulombic repulsion, asymmetry between protons and neutrons, and pairing effects, are explained. The paragraph also discusses the concept of magic numbers and their relation to exceptional stability in certain nuclei.
🌐 Trends in Nuclear Stability and Odd-Even Effects
The discussion continues with trends in nuclear stability, focusing on the odd-even effects for protons and neutrons. It is noted that even-numbered nuclei tend to be more stable, which is reflected in the semi-empirical mass formula. The magic numbers, associated with high stability, are identified, and the absence of stable isotopes for certain elements like technetium and promethium is discussed. The relationship between the number of stable nuclei and both proton and neutron numbers is examined, revealing insights into nuclear stability patterns.
📊 Mass Parabolas and Nuclear Decay
The concept of mass parabolas is introduced, illustrating how the mass of a nucleus varies with its proton number for a fixed mass number A. The paragraph explains how nuclei decay through beta decay or electron capture to reach the most stable configuration, as predicted by the semi-empirical mass formula. The decay of various isotopes leading to the stable isotope of niobium-93 is used as an example to demonstrate the construction and application of mass parabolas in understanding nuclear stability and decay processes.
🚀 Conclusion and Preview of Future Topics
In conclusion, the paragraph summarizes the key points discussed in the lecture, including the concepts of Q-value, conservation laws, binding energy, and nuclear stability. It also provides a preview of upcoming topics, such as positron decay, electron capture, and further exploration of nuclear reactions and decay modes. The lecture ends with a reminder of the importance of understanding these principles for future studies in nuclear physics.
Mindmap
Keywords
💡Boron Neutron Capture Therapy (BNCT)
💡Nuclear Reaction
💡Q Value
💡Binding Energy
💡Nucleon
💡Mass-Energy Equivalence
💡Liquid Drop Model
💡Semi-Empirical Mass Formula
💡Nuclear Stability
💡Magic Numbers
💡Beta Decay
Highlights
The lecture focuses on the concept of boron neutron capture therapy and its technical aspects.
The Q value, or energy change, in nuclear reactions is either released or consumed, and can be determined using the Q equation.
The Q value calculation can be done using masses in atomic mass units (amu), kinetic energies, or binding energies.
The difference in mass or energy in a nuclear reaction can be used to determine if the reaction is exothermic (Q > 0) or endothermic (Q < 0).
The table of nuclides is a valuable resource for finding information such as excess mass and binding energy.
The binding energy is directly related to the mass additively, allowing for the calculation of the Q value using binding energies.
The Q value for a given nuclear reaction can be calculated using the binding energies of the products and reactants.
The conservation of energy and momentum are key principles in nuclear reactions and decay processes.
The lecture introduces the semi-empirical mass formula, which predicts the binding energy of a nucleus based on its proton and neutron numbers.
The semi-empirical mass formula accounts for volume, surface area, Coulombic repulsion, asymmetry, and pairing terms.
The formula shows that nuclei with equal numbers of protons and neutrons tend to be more stable, especially for light nuclei.
Magic numbers, or numbers of nucleons that fill all energy levels at a certain level, result in exceptionally stable nuclei.
The most stable nuclei are found near the magic numbers 82, 50, 28, and repetition of this pattern with larger periodicity.
Elements with no stable isotopes, such as technetium and promethium, are found far from the magic numbers.
The lecture discusses the concept of mass parabolas, which show the relationship between the mass of a nucleus and its proton number.
Nuclei decay through beta decay or electron capture to reach the most stable configuration.
The mass parabolas can be constructed for any given mass number A to understand the stability of nuclei and their decay paths.
For large energy changes, nuclei can decay through positron decay or electron capture, while for small energy changes, only electron capture is possible.
Transcripts
Browse More Related Video
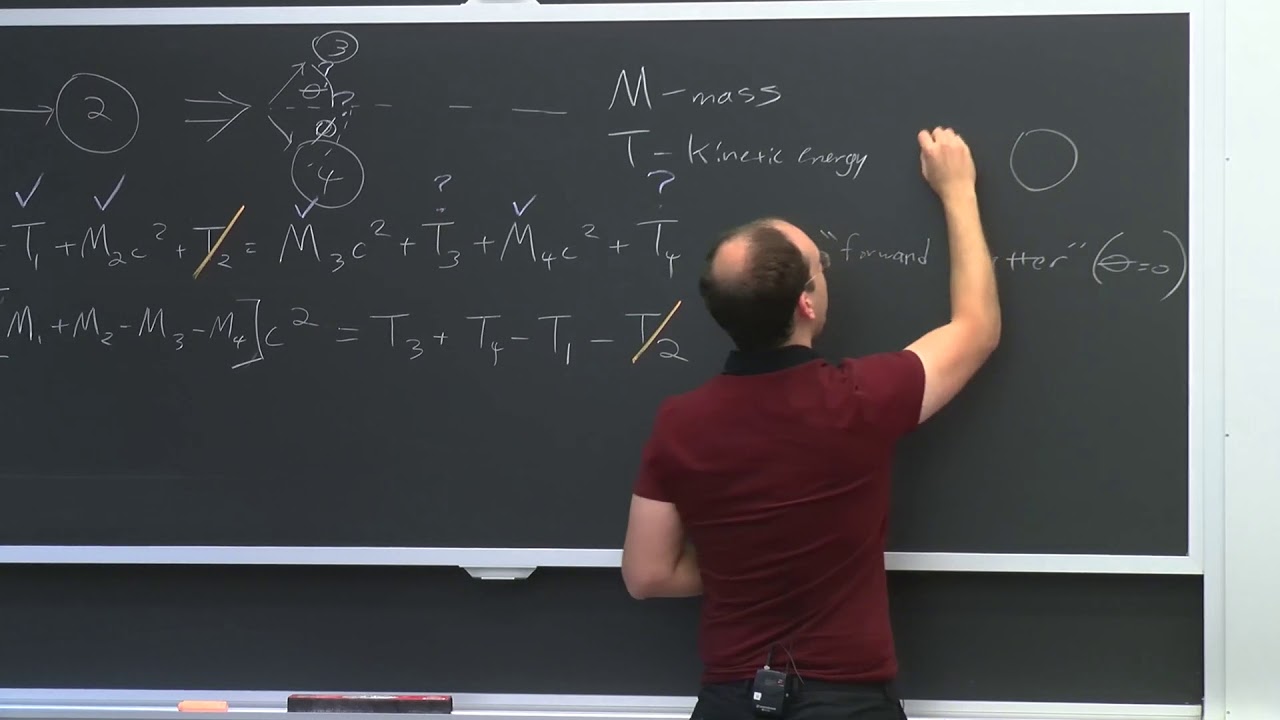
6. The Q-Equation — The Most General Nuclear Reaction

5. Mass Parabolas Continued, Stability, and Half Life

Nuclear Physics: Crash Course Physics #45

Alpha Particles, Beta Particles, Gamma Rays, Positrons, Electrons, Protons, and Neutrons

3. Nuclear Mass and Stability, Nuclear Reactions and Notation, Introduction to Cross Section

7. Q-Equation Continued and Examples
5.0 / 5 (0 votes)
Thanks for rating: