Essential & Practical Circuit Analysis: Part 1- DC Circuits
TLDRThis comprehensive video tutorial delves into the fundamentals of circuit analysis, exploring key concepts such as Ohm's law, series and parallel circuits, voltage and current dividers, and Kirchhoff's laws. The presenter introduces Thevenin and Norton equivalent circuits and demonstrates the powerful Superposition Theorem for simplifying complex circuits. Through practical examples, the video emphasizes the importance of practice and experimentation to master these techniques and enhance problem-solving skills in electronics.
Takeaways
- 📚 Circuit analysis is a fundamental toolkit for understanding and designing complex circuits, built upon layers of abstraction from physics to electronics systems.
- 🔌 The video covers a range of topics including linear circuit elements, Ohm's law, series and parallel circuits, voltage and current dividers, Kirchhoff's laws, source transformation, Thevenin and Norton equivalent circuits, and superposition theorem.
- 🔄 Linear circuit elements like resistors, capacitors, and inductors have a linear relationship between current and voltage, which is essential for simplification and analysis.
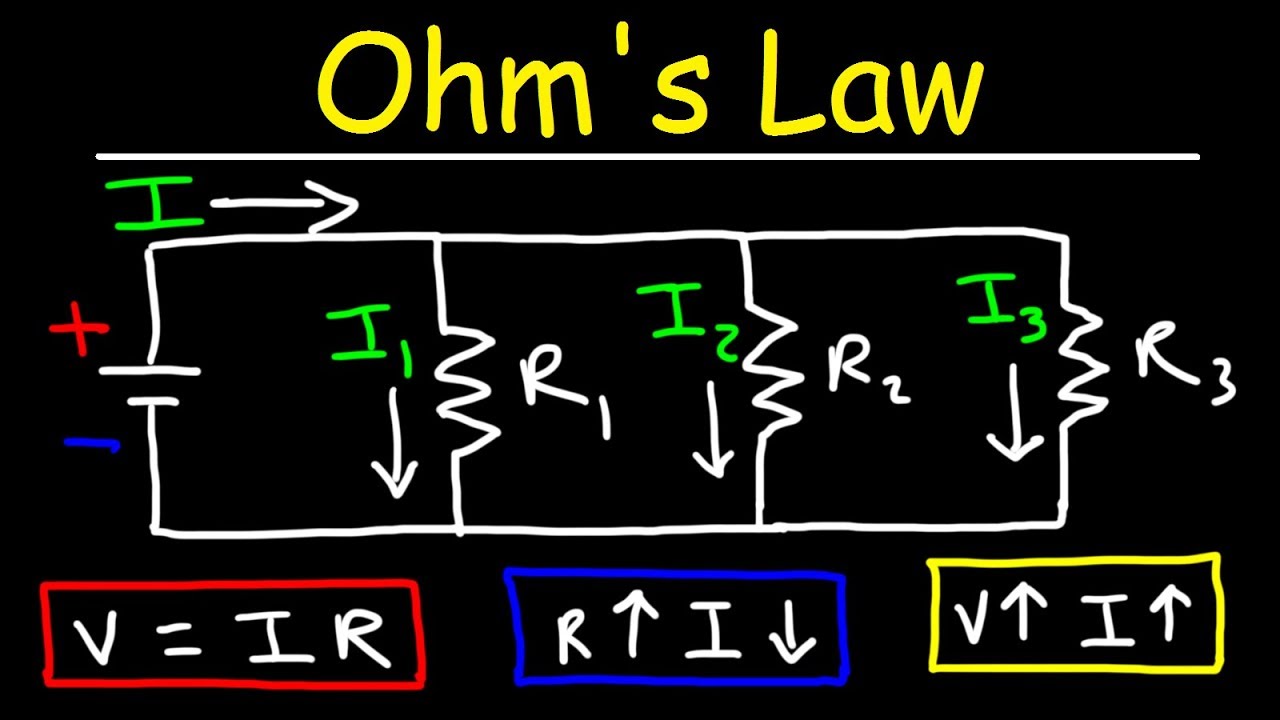
- ⚡ Ohm's law (V=IR) is the cornerstone of circuit analysis, allowing the calculation of unknown voltage, current, or resistance given two of the three elements.
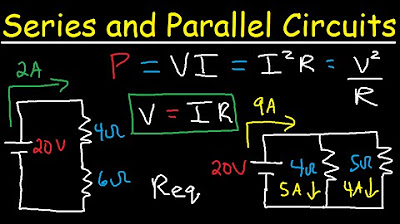
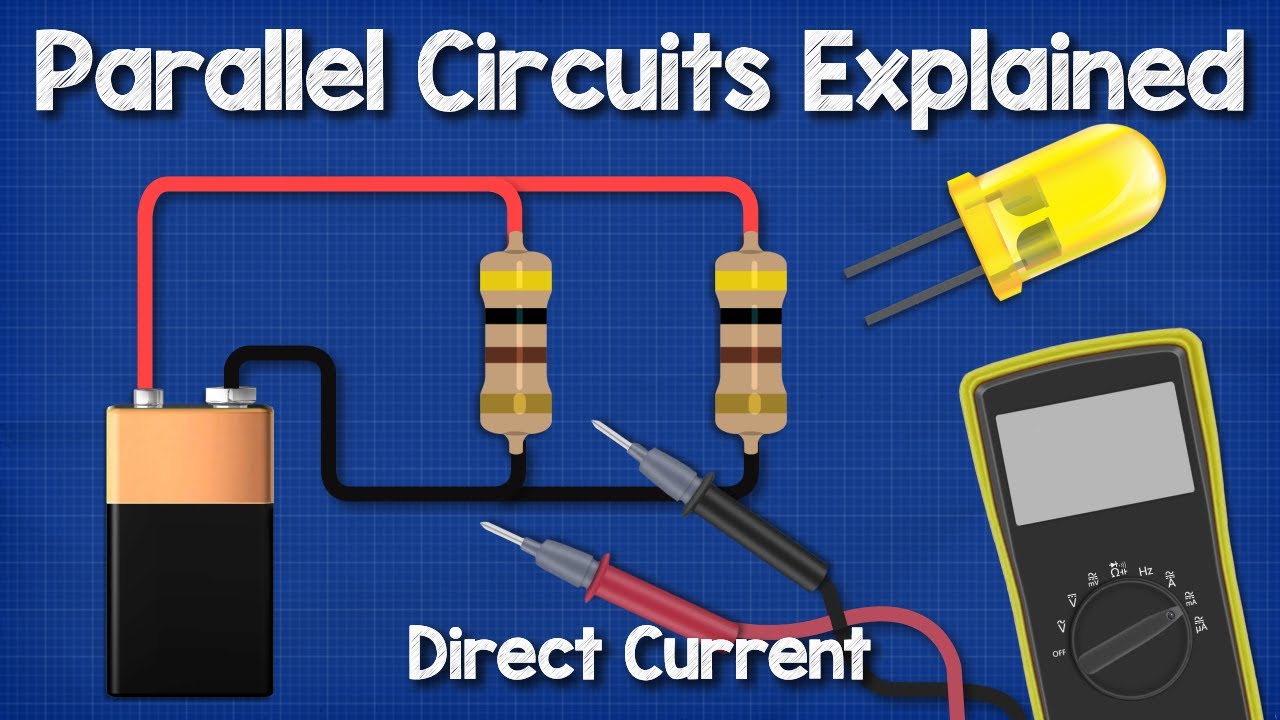
- 🔗 Series and parallel circuits are basic configurations where elements are connected. In series circuits, current is the same throughout, while in parallel circuits, voltage is shared among elements.
- 🔄 Voltage dividers and current dividers are circuits that create outputs which are fractions of their input voltage or current, using series and parallel combinations of resistors.
- 🔍 Kirchhoff's Current Law (KCL) and Kirchhoff's Voltage Law (KVL) are fundamental laws used in nodal and loop analysis to solve for unknown currents and voltages in a circuit.
- 🔌 Source transformation allows the conversion between Thevenin (voltage source in series with a resistor) and Norton (current source in parallel with a resistor) equivalent circuits for easier analysis.
- 📈 Superposition theorem is a powerful method to solve circuits with multiple sources by considering each source individually, then summing their effects to find the overall circuit behavior.
- 🛠️ Practicing circuit analysis involves identifying the appropriate technique for a given problem, experimenting with actual circuits, and using simulation software like LTspice for verification and further learning.
Q & A
What is the main purpose of circuit analysis?
-Circuit analysis is a toolkit for understanding and designing more complex circuits. It provides a layer of abstraction based on other abstractions and is essential for success in the areas of electronics and electronic systems.
What are the fundamental components of Ohm's law?
-Ohm's law is based on three variables: Voltage (V), Current (I), and Resistance (R). The law states that V equals I times R, or V/R equals I, allowing us to solve for an unknown current, voltage, or resistance given two of those elements.
How does a series circuit differ from a parallel circuit?
-In a series circuit, elements are connected one after another, forming a single path for current to flow. In contrast, a parallel circuit has elements connected across common points, creating multiple paths for current to flow.
What is a voltage divider and how does it function?
-A voltage divider is a circuit that creates a voltage which is a fraction of its voltage source. It typically consists of two resistors in series, and the output voltage is observed across the second resistor. The output voltage can be calculated using the formula Vout = Vs * R2 / (R1 + R2).
What is the difference between a voltage divider and a current divider?
-A voltage divider creates an output voltage that is a fraction of its source voltage, while a current divider creates an output current that is a fraction of its source current. Voltage dividers are more commonly used in practice because most real-world sources resemble voltage sources more closely.
What is Kirchhoff's Current Law (KCL) and how is it applied?
-Kirchhoff's Current Law, or KCL, states that the sum of all currents entering a node equals the sum of all currents exiting the node. It is used to solve for unknown currents in a circuit by setting up equations based on the conservation of charge at nodes (junctions) within the circuit.
What is Kirchhoff's Voltage Law (KVL) and how does it relate to voltage drops and rises in a circuit?
-Kirchhoff's Voltage Law, or KVL, states that the sum of all voltage drops and rises in a closed loop equals zero. It is used to solve for unknown voltages and currents in a circuit by considering the balance of potential energy changes as current flows through the circuit.
What is the significance of linear circuit elements in circuit analysis?
-Linear circuit elements, such as resistors, capacitors, and inductors, are significant in circuit analysis because their current-voltage relationships are linear, making them easier to analyze and predict. This linearity allows for the application of powerful mathematical techniques like superposition.
What is source transformation and why is it useful in circuit analysis?
-Source transformation is a method that allows the conversion of a circuit containing a voltage source in series with a resistor (Thevenin circuit) into an equivalent circuit with a current source in parallel with a resistor (Norton circuit), and vice versa. It is useful for simplifying complex circuits and making them easier to analyze using established techniques.
How does the concept of a loop and a node relate to circuit analysis?
-In circuit analysis, a loop is a closed path that begins and ends at the same node, and a node is a junction of connecting wires where circuit elements are joined. Understanding these concepts is crucial for applying KVL and KCL to analyze circuits, as they help in identifying the paths and points at which to apply these laws.
What is the role of practice in mastering circuit analysis?
-Practice is essential in mastering circuit analysis as it helps in applying the learned techniques to various scenarios and identifying the most appropriate method for a given circuit. Regular practice also aids in understanding the behavior of circuits and the effects of changing components.
Outlines
📚 Introduction to Circuit Analysis
The paragraph introduces the concept of circuit analysis as a fundamental toolkit for understanding and designing complex circuits. It emphasizes the importance of grasping the foundational concepts of circuit analysis before delving into electronics or electronic systems. The video aims to cover a range of topics starting from basic circuits to more complex ones, including linear circuit elements, Ohm's law, series and parallel circuits, voltage and current dividers, Kirchhoff's laws, and Thevenin and Norton equivalent circuits.
🔌 Understanding Circuit Components and Ohm's Law
This section focuses on the basic components of a circuit such as nodes, branches, and loops, and how they function within a circuit. It explains Ohm's law, which is a fundamental relationship in circuit analysis, and how it can be used to calculate unknown values of voltage, current, or resistance in a circuit. The paragraph also discusses the difference between series and parallel connections of circuit elements and how to calculate equivalent resistance in these configurations.
🔄 Voltage Dividers and Parallel Circuits
The discussion moves on to voltage dividers, explaining how they work in a series circuit and how to calculate the output voltage using resistor values. It also covers the effect of attaching a load to a voltage divider and how it changes the output voltage. The concept of current dividers is briefly introduced, highlighting the difference between voltage and current dividers and their applications in real-world circuits.
🔧 Kirchhoff's Current Law (KCL)
This part introduces Kirchhoff's Current Law (KCL), which states that the sum of all currents entering a node must equal the sum of all currents exiting the node. The paragraph provides examples and illustrations to help understand KCL and its application in solving for unknown currents in a circuit. It also sets the stage for further exploration into nodal analysis, which uses KCL to determine node voltages.
🔄 Nodal Analysis and Kirchhoff's Voltage Law (KVL)
The paragraph delves into nodal analysis, a technique for solving for node voltages using KCL. It explains the process of setting up reference nodes and writing KCL equations for each node in the circuit. The discussion then transitions to Kirchhoff's Voltage Law (KVL), which states that the sum of all voltage drops in a closed loop must equal the sum of all voltage rises. The paragraph provides an analogy of skiing to illustrate KVL and demonstrates how to apply it to solve for unknown voltages in a circuit.
🔌 Thevenin and Norton Equivalent Circuits
This section introduces Thevenin and Norton equivalent circuits, which are simplified representations of a complex circuit. It explains the process of source transformation, which allows the conversion between Thevenin and Norton circuits, and how to find the equivalent resistance and voltage or current for these representations. The paragraph emphasizes the usefulness of these equivalent circuits in simplifying circuit analysis and making it easier to understand the behavior of complex circuits.
📈 Superposition Theorem
The Superposition Theorem is introduced as a powerful tool for analyzing circuits with multiple sources. The theorem allows the consideration of one source at a time and the addition of their individual effects to find the total effect. The paragraph outlines the steps for applying the Superposition Theorem, including setting all but one source to zero, solving for the necessary currents and voltages, and summing the solutions to find the actual values. The theorem is demonstrated through an example that involves finding the voltage across a resistor using the theorem.
🛠️ Circuit Analysis Techniques and Practice
The conclusion of the video script emphasizes the importance of practice in mastering circuit analysis. It encourages viewers to experiment with real circuits and use simulation software like LT Spice to verify their findings and explore circuit behavior. The video creator shares personal insights on the challenges of identifying the appropriate technique for a given circuit and the value of hands-on experience. The script ends with a call to action for viewers to provide feedback and engage with the content by liking and subscribing to the channel.
Mindmap
Keywords
💡Circuit Analysis
💡Ohm's Law
💡Series and Parallel Circuits
💡Voltage Divider
💡Kirchhoff's Laws
💡Thevenin's Theorem
💡Norton's Theorem
💡Superposition Theorem
💡Linear Circuit Elements
💡Node
💡Branch
💡Loop
Highlights
Circuit analysis is a toolkit for understanding and designing complex circuits, based on layers of abstraction.
Ohm's law is a fundamental relationship in circuit analysis, allowing the calculation of unknown current, voltage, or resistance.
Series and parallel circuits are the two basic ways in which circuit elements can be connected.
Voltage dividers and current dividers are circuits that create fractions of their source voltage or current.
KCL (Kirchhoff's Current Law) states that the sum of all currents entering a node equals the sum of all currents exiting the node.
KVL (Kirchhoff's Voltage Law) asserts that all voltage drops must equal all voltage rises in a closed loop.
Source transformation allows the conversion between Thévenin (voltage source in series with a resistor) and Norton (current source in parallel with a resistor) equivalent circuits.
Superposition theorem enables the analysis of a circuit by considering one source at a time and then summing the contributions of each source.
The process of finding a Norton equivalent circuit involves short-circuiting the output terminals and finding the short-circuit current.
For Thévenin equivalent circuits, the open-circuit voltage across the output terminals is determined after detaching the load resistor and short-circuiting all sources.
Nodal analysis uses KCL to solve for node voltages in a circuit, which can then be used to find other unknowns.
Loop analysis, using KVL, involves assigning loop currents to independent loops and writing KVL equations to solve for those currents.
Understanding circuit analysis is crucial for success in electronics and electronic systems, as it provides a foundation for more advanced topics.
Linear circuit elements, such as resistors, capacitors, and inductors, have a linear relationship between current and voltage.
In a series circuit, the current is the same through all elements, while in a parallel circuit, each resistor can have a different current.
The voltage across each resistor in a series circuit can be found using Ohm's law, with the voltage divided proportionally based on resistance values.
When a load is attached to a voltage divider, the output voltage is reduced due to the equivalent resistance change caused by the parallel connection of the load.
Circuit analysis techniques can be applied to a wide range of electronic systems, from simple to complex.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: