High School Physics - Parallel Circuits

Dan Fullerton
3 Jan 201214:57
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative session, Mr. Fullerton delves into the intricacies of parallel circuits, emphasizing the application of Kirchhoff's laws, Ohm's law, and the concept of equivalent resistance. He illustrates how to calculate the power used in parallel circuits and provides a clear method for determining the equivalent resistance of resistors in parallel. Through practical examples and step-by-step problem-solving, the session aims to enhance the understanding of parallel circuit analysis, offering valuable insights for anyone interested in electronics.

Takeaways
  • 📌 Parallel circuits have multiple current paths with a constant voltage across each element.
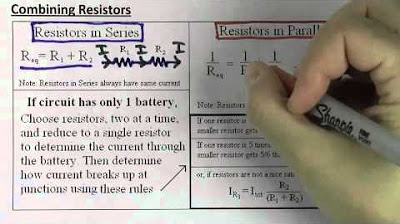
  • 🔧 The equivalent resistance in a parallel circuit can be calculated using the formula 1/R_equivalent = 1/R1 + 1/R2 + ... + 1/Rn.
  • 💡 A shortcut for two resistors in parallel is R_equivalent = (R1 * R2) / (R1 + R2).
  • 🌐 Kirchhoff's laws and Ohm's law are essential tools for solving parallel circuit problems.
  • ⚡ The total current in a parallel circuit is the sum of the individual currents through each resistor.
  • 🔌 The potential difference across each resistor in a parallel circuit is the same due to the constant voltage.
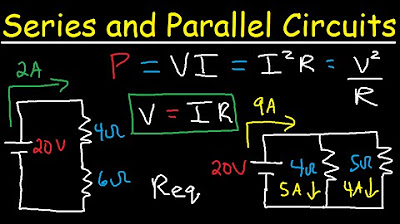
  • 📈 Power in a parallel circuit is the sum of the power dissipated in each resistor, calculated as P = VI.
  • 🛠️ When solving parallel circuits, a verb table can be used to organize and calculate unknown values.
  • 🎯 To find the current through an individual resistor, use Ohm's law: I = V/R.
  • 🔄 Currents split and recombine in a parallel circuit, maintaining the total current throughout.
  • 📊 In a parallel circuit, the equivalent resistance is always less than the smallest individual resistor involved.
Q & A

  • What is the main objective of the video?

    -The main objective of the video is to teach viewers how to solve parallel circuit problems using FERP tables, calculate the equivalent resistance for resistors in a parallel configuration, and calculate the power used in parallel circuits.

  • What is a characteristic feature of a parallel circuit?

    -A characteristic feature of a parallel circuit is that it has multiple current paths, and the voltage is constant across the circuit elements that are in parallel.

  • How does Kirchhoff's current law relate to parallel circuits?

    -Kirchhoff's current law states that the sum of all currents entering a circuit element is the same as the sum of all currents leaving that circuit element. This law is important for analyzing parallel circuits because it helps to determine how the current is divided among the parallel branches.

  • What is the formula for calculating the equivalent resistance of two resistors in parallel?

    -The formula for calculating the equivalent resistance of two resistors in parallel is R_equivalent = (r1 * r2) / (r1 + r2).

  • How does the equivalent resistance of resistors in parallel compare to the individual resistances?

    -The equivalent resistance of resistors in parallel is always less than the smallest individual resistor in the configuration.

  • What is the purpose of a verb table in circuit analysis?

    -A verb table is used in circuit analysis to organize and summarize the known and unknown values in a circuit, which helps in solving for the remaining unknown quantities in a systematic manner.

  • How does the current behave in a parallel circuit?

    -In a parallel circuit, the total current splits among the parallel branches, with each branch carrying a portion of the current. The currents through each branch add up to equal the total current in the circuit.

  • What happens to the power dissipated in resistors in a parallel circuit?

    -The power dissipated in each resistor in a parallel circuit adds up to equal the total power dissipated in the circuit.

  • How can you determine the potential difference across a resistor in a parallel circuit?

    -In a parallel circuit, the potential difference across each resistor is the same as the potential difference across the entire circuit, which is determined by the voltage source.

  • What is the relationship between the total resistance and the individual resistances in a parallel circuit?

    -The total resistance in a parallel circuit is less than the smallest individual resistance, and it can be calculated using the reciprocal formula for equivalent resistance.

  • How can you calculate the current through a specific resistor in a parallel circuit?

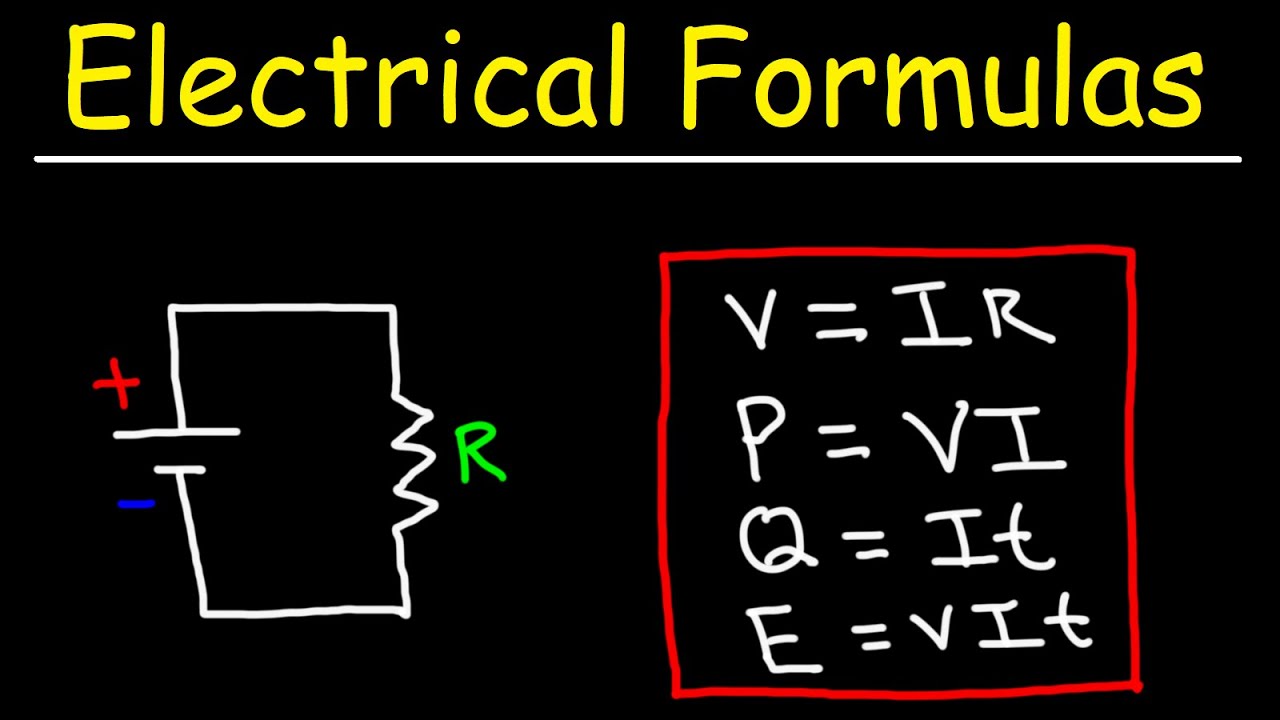
    -You can calculate the current through a specific resistor in a parallel circuit using Ohm's law, which states that the current (I) is equal to the voltage (V) divided by the resistance (R).

Outlines
00:00
🔌 Introduction to Parallel Circuits and Analysis

This paragraph introduces the concept of parallel circuits and their analysis. It outlines the objectives of understanding and solving parallel circuit problems using FEERP tables, calculating equivalent resistance for resistors in parallel configurations, and determining power usage in parallel circuits. The content explains that in a parallel circuit, the voltage is constant across all elements and provides an example of how resistors are connected in parallel. It also briefly reviews Kirchhoff's laws and Ohm's law, which are essential tools for circuit analysis, and explains how to calculate equivalent resistance in parallel circuits with examples.

05:03
📊 Parallel Circuit Analysis using a VERB Table

This paragraph delves into the method of analyzing parallel circuits using a Voltage, Current, Resistance, and Power (VERB) table. It describes the process of labeling and filling out the table with known values, such as voltage sources and resistances, to find unknowns like total current and power dissipation. The paragraph provides a detailed example of completing a VERB table for a circuit with three resistors and explains how to use the table to determine the potential difference and current through each resistor. It also emphasizes the characteristics of parallel circuits, such as the splitting and recombining of current and the additive nature of power dissipation.

10:06
🖌️ Drawing and Solving Parallel Circuit Problems

The final paragraph focuses on drawing diagrams of operating parallel circuits and solving related problems. It provides a step-by-step guide on how to represent a parallel circuit with a battery, resistors, and an ammeter in a diagram. The paragraph then presents a sample problem involving a circuit with a known ammeter reading and an unknown resistor. It explains how to use a VERB table to determine the equivalent resistance, the current through a known resistor, and the resistance of the unknown resistor. The summary highlights the importance of understanding the potential drop across parallel elements and the methods to calculate currents and power in such circuits.

Mindmap
Keywords
💡Parallel Circuits
Parallel circuits are a type of electrical circuit configuration where multiple components, such as resistors, are connected side by side. This allows for multiple paths for the current to flow. In the context of the video, parallel circuits are the main focus for analysis, and the properties of these circuits, such as constant voltage across components, are essential for solving circuit problems.
💡Equivalent Resistance
Equivalent resistance is a single value that represents the combined resistance of multiple resistors connected in parallel. It is calculated using the reciprocal formula of the individual resistances. In the video, the concept of equivalent resistance is crucial for analyzing parallel circuits, as it simplifies the circuit and allows for easier calculation of total current and power dissipation.
💡Kirchhoff's Laws
Kirchhoff's laws are fundamental principles used in electrical circuit analysis. Kirchhoff's current law states that the total current entering a junction equals the total current leaving it, while Kirchhoff's voltage law states that the sum of potential differences around any closed loop in a circuit is zero. These laws are essential for solving complex circuits, including parallel circuits, as they help in determining the flow of current and potential differences in the circuit.
💡Ohm's Law
Ohm's law is a vital relationship in electrical engineering that states that the current through a conductor between two points is directly proportional to the voltage across the two points and inversely proportional to the resistance between them. It is used to calculate the current flowing through a resistor in a parallel circuit when the voltage and resistance are known.
💡Power Calculation
Power calculation in electrical circuits is the process of determining the rate at which electrical energy is converted into another form of energy. It is calculated using the formula P=VI, where P is power in watts, V is voltage in volts, and I is current in amps. In the context of the video, power calculation is important for understanding the energy dissipation in parallel circuits.
💡Circuit Analysis
Circuit analysis is the process of determining the relationships between the various quantities within a circuit, such as voltage, current, and resistance. It involves using mathematical models and physical laws to predict the behavior of the circuit. In the video, circuit analysis is the main activity, with a focus on parallel circuits and the use of tools like Kirchhoff's laws and Ohm's law to solve for unknown values.
💡FERP
FERP is an acronym for Fill, Equivalent Resistance, Rule, and Power, which is a method for solving parallel circuit problems. It is a systematic approach that helps in remembering the steps to analyze and solve such circuits. The video emphasizes using FERP to calculate equivalent resistance and power in parallel circuits.
💡Verb Table
A verb table is a tool used in circuit analysis to organize known and unknown values within a circuit, making it easier to solve for the missing parameters. It typically includes columns for Voltage (V), Current (I), Resistance (R), and Power (P), with rows for each component and a total or summary row. The video script demonstrates creating and using a verb table to analyze parallel circuits.
💡Current Splitting
Current splitting is a phenomenon in parallel circuits where the total current entering a junction splits into smaller currents that flow through each parallel branch. These individual currents then recombine at the junction on the other side of the parallel branches. The concept is important for understanding how current behaves in parallel circuits and is used to solve for currents through individual components.
💡Voltage Drop
Voltage drop is the decrease in electric potential (voltage) across a component in a circuit. In a parallel circuit, the voltage drop across each component is the same because they are all directly connected to the same two points, which have the same potential. Understanding voltage drop is crucial for analyzing the behavior of components in a parallel circuit and calculating power and current.
Highlights

Introduction to parallel circuits and their analysis using FERP tables.

Objective to solve parallel circuit problems and calculate equivalent resistance and power used.

Description of a parallel circuit with multiple current paths and constant voltage across elements.

Explanation of Kirchhoff's laws as main tools for solving circuits, alongside Ohm's law.

Calculation method for equivalent resistance in parallel circuits with the formula 1/R_equivalent = 1/R1 + 1/R2 + ....

Cheat sheet formula for two resistors in parallel: R_equivalent = (R1 * R2) / (R1 + R2).

Principle that equivalent resistance in parallel is always less than the smallest resistor.

Example calculation of equivalent resistance with three 2000-ohm resistors in parallel.

Construction of a verb table for parallel circuit analysis with resistors labeled and column labels defined.

Determination of total current and power dissipation in a circuit using the equivalent resistance.

Use of a ground sign to symbolize the zero reference point in potential.

Addition of currents through parallel elements to equal the total current.

Addition of power dissipated in parallel elements to equal the total power dissipated.

Sample problem of finding equivalent resistance with resistors of 4 ohms, 6 ohms, and 8 ohms in parallel.

Illustration of a circuit diagram with resistors in parallel and an ammeter measuring total current.

Solution to a sample problem involving a 3-ohm resistor, an unknown resistor R, and ammeters.

Use of a verb table to determine equivalent resistance, current through a meter, and resistance of an unknown resistor.

Conclusion and encouragement for further analysis of parallel circuits with additional problems provided.

Transcripts

Browse More Related Video

High School Physics - Series Circuits

Essential & Practical Circuit Analysis: Part 1- DC Circuits

Resistors in parallel | Circuits | Physics | Khan Academy

Circuits (AP Physics SuperCram Review)

Series and Parallel Circuits

Electrical Formulas - Basic Electricity For Beginners

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Circuit AnalysisParallel CircuitsEquivalent ResistanceKirchhoff's LawsOhm's LawPhysics EducationElectrical EngineeringProblem SolvingTutorial