Related Rates - Inflated Balloon & Melting Snowball Problem - Surface Area & Volume
TLDRThe video script presents a comprehensive guide to solving related rate problems involving circles and spheres. It begins with calculating the rate of change of a circle's circumference and area, given the rate of change of its radius. The script then moves on to discuss the rate of change of a balloon's radius when air is pumped in at a constant rate, and finally, it tackles the rate of change of a snowball's diameter as its surface area decreases. The examples are rich in detail, providing step-by-step mathematical differentiation and clear explanations, making the concepts accessible and engaging for viewers.
Takeaways
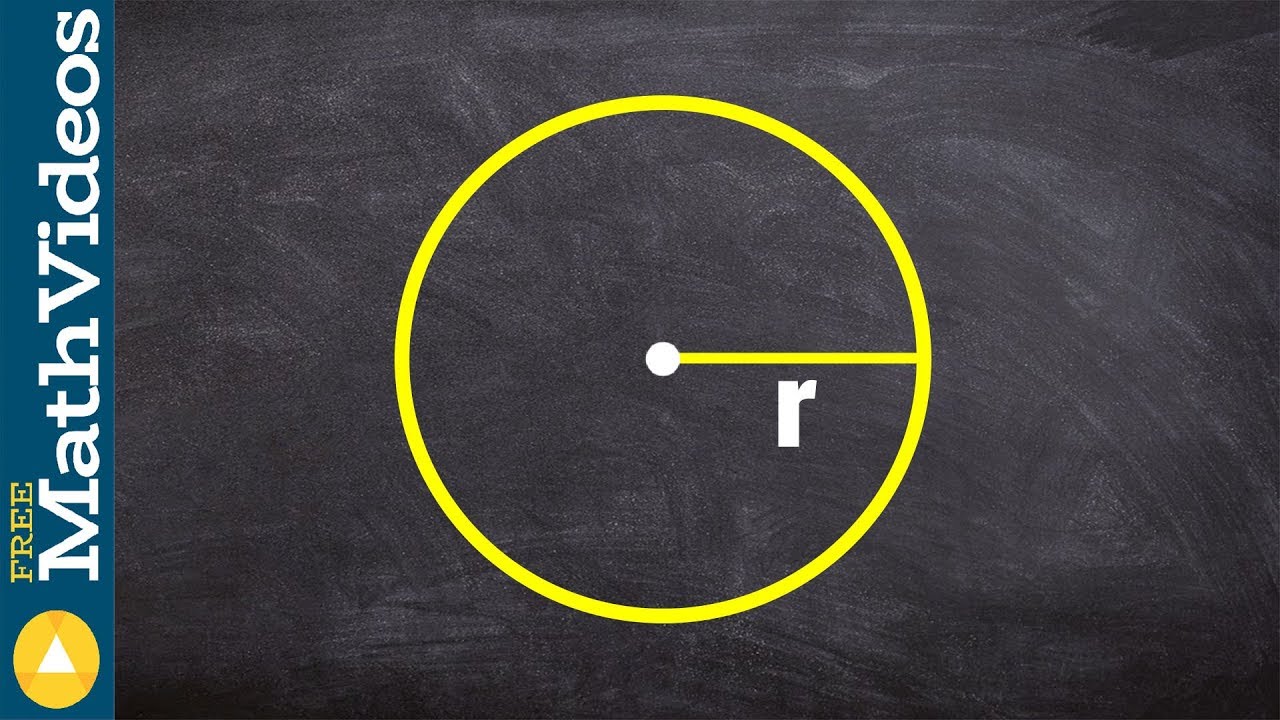
- 📐 The radius of a circle increasing at a rate of 5 cm/min leads to the circumference changing at a rate of 10π cm/min.
- 📐 When the radius of a circle is 8 cm, the area of the circle changes at a rate of 80π sq cm/min.
- 📐 The volume of a balloon being inflated at 450 cm³/min corresponds to the rate of change of the balloon's volume.
- 📐 The radius of a balloon changes at a rate of 9/8π cm/min when the volume inflation rate is 450 cm³/min and the radius is 10 cm.
- 📐 The surface area of a sphere is given by the formula 4πr², which can be used to relate the rate of change of the surface area to the radius.
- 📐 The diameter of a snowball, which is twice the radius, can be related to the surface area and its rate of change.
- 📐 When the surface area of a snowball decreases at a rate of 2 sq cm/min and the radius is 5 cm, the diameter changes at a rate of -1/10π cm/min.
- 📐 Differentiating equations with respect to time is a fundamental method for solving related rate problems involving circles and spheres.
- 📐 Constants like π and the formulas for circumference and area of circles and volume of spheres are critical in these calculations.
- 📐 Positive and negative rates of change indicate whether a quantity is increasing or decreasing, respectively.
Q & A
What is the main topic of the video?
-The main topic of the video is related rate problems involving circles, spheres, and the concept of an inflating balloon and a melting snowball.
How is the rate of change of a circle's circumference calculated?
-The rate of change of a circle's circumference (dc/dt) is calculated using the formula C = 2πr, where C is the circumference and r is the radius. By differentiating this formula with respect to time (t), we get dc/dt = 2π(dr/dt). When the radius is increasing at 5 cm per minute, the circumference is changing at a rate of 10π centimeters per minute.
What is the relationship between the radius and the area of a circle?
-The area (A) of a circle is related to its radius (r) by the formula A = πr². This relationship is quadratic, meaning that the area of the circle increases with the square of the radius.
How is the rate of change of a circle's area calculated?
-The rate of change of a circle's area (dA/dt) is calculated by differentiating the area formula A = πr² with respect to time (t). This gives us dA/dt = 2πr(dr/dt). When the radius is 8 cm and changing at a rate of 5 cm per minute, the area is changing at a rate of 80π square centimeters per minute.
What is the volume formula for a sphere?
-The volume (V) of a sphere is given by the formula V = (4/3)πr³, where r is the radius of the sphere.
How can we find the rate at which the radius of a balloon is changing?
-The rate at which the radius of a balloon is changing (dr/dt) can be found by differentiating the volume formula for a sphere V = (4/3)πr³ with respect to time (t) and using the given rate of volume change (dv/dt). When the volume is increasing at 450 cubic centimeters per minute and the radius is 10 cm, the radius is changing at a rate of (9/8)π centimeters per minute.
What is the surface area formula for a sphere?
-The surface area (SA) of a sphere is given by the formula SA = 4πr², where r is the radius of the sphere.
How can we determine the rate at which the diameter of a sphere is changing?
-The rate at which the diameter of a sphere (dd/dt) is changing can be determined by differentiating the surface area formula SA = 4πr² with respect to time (t) and using the given rate of surface area change (dsa/dt). When the radius is 5 cm and the surface area is decreasing at 2 square centimeters per minute, the diameter is changing at a rate of -1/(10π) centimeters per minute.
What is the significance of the negative sign in the rate of change of the snowball's surface area?
-The negative sign in the rate of change of the snowball's surface area (dsa/dt) indicates that the surface area is decreasing over time, which is consistent with the melting snowball scenario presented in the video.
How do the concepts of calculus apply to the problems discussed in the video?
-Calculus, specifically differentiation, is used to find the rates of change for various properties of geometric shapes like circles and spheres. By differentiating equations relating quantities such as radius, circumference, area, and volume with respect to time, we can determine how these quantities change as the shape evolves.
What is the role of constants in the differentiation process?
-Constants, such as π in the formulas for the circumference and area of a circle or the volume and surface area of a sphere, do not change when taking the derivative with respect to time. They can often be factored out of the differentiation process, simplifying the calculations.
How does the video script demonstrate the application of related rates in real-world scenarios?
-The video script demonstrates the application of related rates by using real-world scenarios such as an inflating balloon and a melting snowball. It shows how the principles of calculus can be used to model and solve problems involving changing dimensions and properties of physical objects over time.
Outlines
📐 Circumference and Area Change Rates
This paragraph discusses the concept of related rates in the context of circles and spheres. It begins with a problem involving the rate of change of a circle's radius and how it affects the circumference. The radius is increasing at a rate of 5 cm/min, and the task is to find the rate of change of the circumference when the radius is 10 cm. The explanation involves using the formula for the circumference of a circle (C = 2πr) and differentiating it with respect to time to find dC/dt. The result is 10π cm/min. The paragraph then moves on to discuss the rate of change of the area of a circle when the radius is 8 cm. The area formula (A = πr^2) is used in a similar fashion to find dA/dt, resulting in 80π square cm/min.
🎈 Rate of Change for Spherical Balloon
The second paragraph focuses on the rate of change of the radius of a spherical balloon. The balloon's volume is increasing at a rate of 450 cubic cm/min, and the goal is to find the rate of change of the radius when it is 10 cm. The volume of a sphere is given by the formula (V = (4/3)πr^3), and differentiating both sides with respect to time yields an equation involving the derivative of r. By setting up and solving the equation with the given values, the paragraph concludes that the rate of change of the radius (dr/dt) is positive 9/8π cm/min.
❄️ Melting Snowball Diameter Change Rate
The final paragraph addresses a problem involving a melting snowball. The surface area of the snowball is decreasing at a rate of 2 square cm/min, and the task is to find the rate of change of the diameter when the radius is 5 cm. The surface area of a sphere is expressed as (SA = 4πr^2), and by relating the diameter to the radius (d = 2r), an equation is derived to solve for the rate of change of the diameter (dd/dt). The detailed calculation leads to the conclusion that the diameter is decreasing at a rate of negative 1/10π cm/min.
Mindmap
Keywords
💡Related Rate Problems
💡Circle
💡Circumference
💡Differentiation
💡Radius
💡Sphere
💡Volume
💡Rate of Change
💡Surface Area
💡Pi (π)
💡Balloon
Highlights
The video discusses related rate problems involving circles and spheres.
The radius of a circle is increasing at a rate of 5 cm/min.
The circumference of a circle changes at a rate of 10π cm/min when the radius is 10 cm.
The area of a circle changes at a rate of 80π cm²/min when the radius is 8 cm.
Air is pumped into a spherical balloon at a rate of 450 cm³/min.
The radius of a balloon changes at a rate of 9/8π cm/min when the radius is 10 cm.
The surface area of a spherical snowball decreases at a rate of 2 cm²/min.
The diameter of a snowball changes at a rate of -1/10π cm/min when the radius is 5 cm.
The circumference formula of a circle is C = 2πr.
The area formula of a circle is A = πr².
The volume formula of a sphere is V = (4/3)πr³.
The surface area formula of a sphere is SA = 4πr².
The diameter of a circle or sphere is twice the radius (d = 2r).
Differentiating equations with respect to time is a key method to solve related rate problems.
Constants like π do not change when differentiating, which simplifies the process.
Positive and negative rates indicate increase and decrease respectively.
Units of measurement are crucial in determining the type of change (linear vs. area).
The video provides a comprehensive guide on solving basic related rates problems.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: