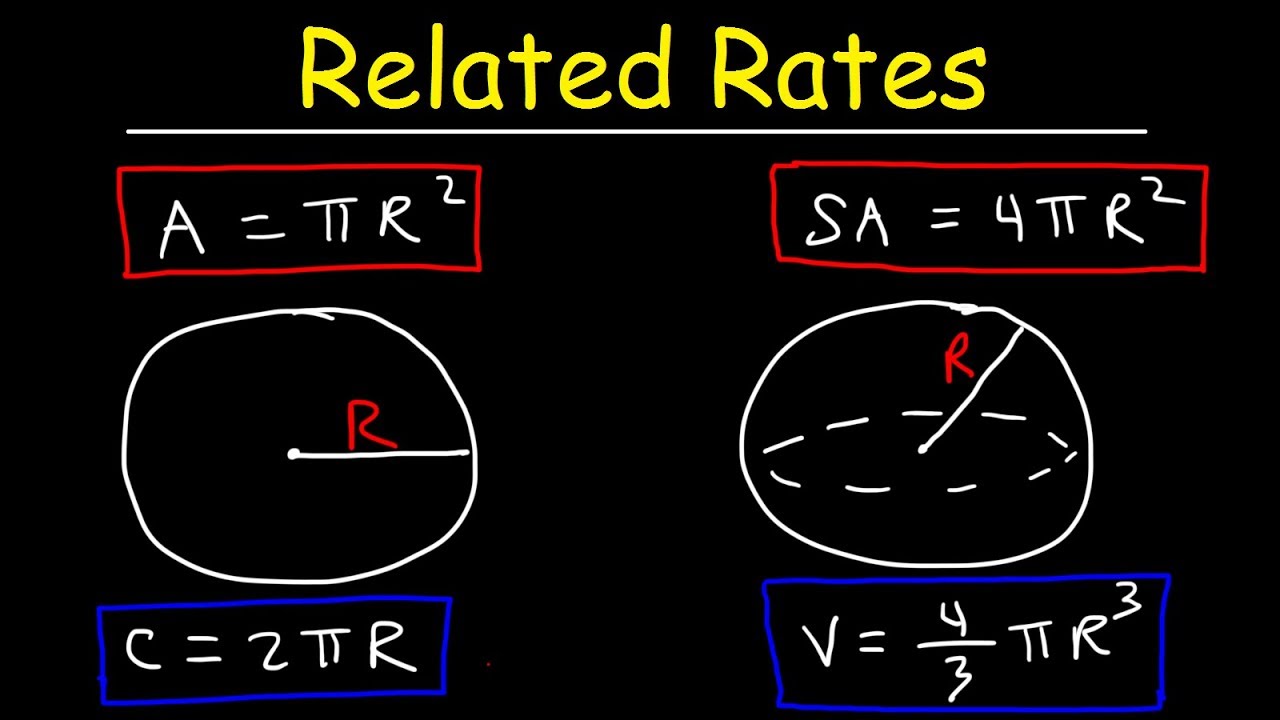
Related Rate Problems - The Cube - Volume, Surface Area & Diagonal Length
TLDRThe video script discusses the mathematical problem of calculating the rate of change of a cube's volume, surface area, and diagonal length. It begins by explaining how to find the rate of volume change (dv/dt) when an edge is 5 meters, using the formula for volume and differentiation. The script then moves on to calculate the rate of change of the surface area (ds/dt) when an edge is 8 meters. Lastly, it tackles the rate of change of the cube's diagonal (dz/dt) when an edge is 12 meters, using the Pythagorean theorem and differentiation. The video provides step-by-step calculations and explanations, making complex mathematical concepts accessible.
Takeaways
- 📈 The edge of a cube is increasing at a rate of 10 meters per hour.
- 🧱 To find the rate of change of volume (dv/dt), we use the formula for the volume of a cube (V = x^3) and differentiate with respect to time.
- 🔢 When x is 5 meters, the rate of change of volume (dv/dt) is 750 cubic meters per hour.
- 🌐 The units for dv/dt are cubic meters per hour, indicating the volume is increasing at a positive rate.
- 📐 For part b, the problem shifts to finding the rate of change of the surface area (dsa/dt) when the edge length is 8 meters.
- 🏠 The surface area of a cube is calculated as 6 times the square of the edge length (SA = 6x^2).
- 🔄 Differentiating the surface area with respect to time gives us a formula involving 2x and dx/dt.
- 📊 At x equals 8 meters, dsa/dt is calculated to be 960 square meters per hour.
- 🛤️ In part c, the focus is on the rate of change of the diagonal length (dz/dt) of the cube when the edge length is 12 meters.
- 📐 The diagonal of the cube is related to the edge length by the equation z^2 = 3x^2, derived from two right triangles formed within the cube.
- 🌐 By differentiating and solving for dz/dt at x equals 12 meters, the result is 10 times the square root of 3 meters per hour.
Q & A
What is the rate of increase of the cube's edges?
-The edges of the cube are increasing at a rate of 10 meters per hour.
How is the volume of a cube related to its side length?
-The volume of a cube is related to its side length by the formula V = x^3, where V is the volume and x is the side length.
What is the derivative of the volume with respect to time?
-The derivative of the volume with respect to time, denoted as dv/dt, is calculated using the formula 3x^2 * dx/dt.
What is the rate of change of the cube's volume when the side length is 5 meters?
-When the side length is 5 meters, the rate of change of the volume (dv/dt) is 750 cubic meters per hour.
How is the surface area of a cube related to its side length?
-The surface area of a cube is related to its side length by the formula SA = 6x^2, where SA is the surface area and x is the side length.
What is the derivative of the surface area with respect to time?
-The derivative of the surface area with respect to time, denoted as dSA/dt, is calculated using the formula 12x * dx/dt.
What is the rate of change of the cube's surface area when the side length is 8 meters?
-When the side length is 8 meters, the rate of change of the surface area (dSA/dt) is 960 square meters per hour.
How can you find the relationship between the diagonal length of a cube and its side length?
-The relationship between the diagonal length (z) and the side length (x) of a cube is given by the equation z^2 = 3x^2, derived from the Pythagorean theorem applied to the right triangles formed within the cube.
What is the rate of change of the cube's diagonal length with respect to time when the side length is 12 meters?
-When the side length is 12 meters, the rate of change of the diagonal length (dz/dt) is 10 times the square root of 3 meters per hour.
What is the unit of measurement for the rate of change of volume and surface area?
-The unit of measurement for the rate of change of volume is cubic meters per hour, and for the surface area, it is square meters per hour.
How does the rate of change of the cube's volume and surface area depend on the rate of increase of its edges?
-The rate of change of the cube's volume (dv/dt) depends on the square of the side length (x^2) multiplied by the rate of increase of the edges (dx/dt). Similarly, the rate of change of the surface area (dSA/dt) depends on the side length (x) multiplied by the rate of increase of the edges (dx/dt).
Outlines
📈 Calculating the Rate of Change of a Cube's Volume
This paragraph discusses the problem of finding the rate at which the volume of a cube is changing. The cube's edges are growing at a rate of 10 meters per hour, and we are interested in the volume change when the side length is 5 meters. The solution involves differentiating the volume formula (V = x^3) with respect to time (t) to find dV/dt. By substituting x = 5 and dx/dt = 10 into the derivative, the calculation yields dV/dt = 750 cubic meters per hour, indicating that the volume is increasing at this rate when the side length is 5 meters.
🔄 Determining the Rate of Change of a Cube's Surface Area
The second paragraph focuses on calculating the rate of change of the surface area of a cube when the edge length is 8 meters. The surface area (SA) of a cube is given by the formula SA = 6x^2. By differentiating this formula with respect to time, we obtain dSA/dt = 12x(dx/dt). Substituting x = 8 and dx/dt = 10 into the derivative, the result is dSA/dt = 960 square meters per hour. This means that the surface area is increasing at this rate when the edge length is 8 meters.
📐 Finding the Rate of Change of a Cube's Diagonal Length
The final paragraph addresses the problem of finding how fast the diagonal length of a cube is changing when the edge length is 12 meters. The diagonal (d) of a cube relates to the edge length (x) by the equation d^2 = 3x^2. Differentiating both sides with respect to time gives 2zd zdt = 3(2x)(dx/dt). After substituting x = 12 and dx/dt = 10, and simplifying the expression, we find that dz/dt = (10√3) meters per hour. This is the rate at which the diagonal length is changing when the edge length is 12 meters.
Mindmap
Keywords
💡Cube
💡Volume
💡Surface Area
💡Diagonal
💡Rate of Change
💡Differentiation
💡Power Rule
💡Pythagorean Theorem
💡Edge Length
💡Derivative
💡Calculus
Highlights
The cube's edges are increasing at a rate of 10 meters per hour.
The problem involves calculating the rate of change of the cube's volume and surface area, as well as the length of the diagonal.
The volume of the cube is given by the formula V = x^3, where x is the side length.
The derivative of the volume with respect to time is dV/dt = 3x^2 * dx/dt, which is used to find the rate of volume change.
When the side length x is 5 meters, the rate of volume change dV/dt is calculated to be 750 cubic meters per hour.
The surface area of the cube is given by the formula SA = 6x^2, where x is the side length.
The derivative of the surface area with respect to time is dSA/dt = 12x * dx/dt, which is used to find the rate of surface area change.
When the side length x is 8 meters, the rate of surface area change dSA/dt is calculated to be 960 square meters per hour.
The length of the cube's diagonal is related to the side length x by the equation z^2 = 3x^2, derived from two right triangles formed within the cube.
The derivative of the diagonal length with respect to time is dz/dt = (30/√3) * dx/dt, which is used to find the rate of change of the diagonal.
When the side length x is 12 meters, the rate of change of the diagonal length dz/dt is calculated to be 10√3 meters per hour.
The problem-solving approach involves visualizing the cube, identifying relationships between dimensions, and applying calculus to find rates of change.
The use of the Pythagorean theorem is crucial in deriving the relationship between the cube's diagonal and side length.
The problem demonstrates the practical application of calculus in understanding the dynamics of geometric shapes.
The process of differentiating equations with respect to time is a key method for finding rates of change in various physical quantities.
The problem requires unit consistency, ensuring that the units of measurement are correctly applied throughout the calculations.
The problem showcases the integration of mathematical concepts such as geometry, algebra, and calculus.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: