Related rates of volume and area of a cube

Prime Newtons
17 Oct 202308:35
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a problem from the field of related rates, focusing on the changing dimensions of a cube. It begins by establishing that the volume of a cube is increasing at a rate of 4 cm³ per minute and asks for the rate at which the surface area is increasing. The solution involves understanding the formulas for the volume and surface area of a cube, and applying differentiation to connect the two rates. The presenter uses the cube root and square root to establish a relationship between the volume (V) and surface area (A) of the cube, leading to the equation A^3 = 216V^2. By differentiating both sides with respect to time, the rate of change of the surface area with respect to time (dA/dt) is found to be 4/x * dV/dt, where x is the side length of the cube. With dV/dt given as 4 cm³/min and the side length x as 3 cm, the rate of change of the surface area is calculated to be 16 cm²/min. The video concludes with an inspirational message about the importance of continuous learning.

Takeaways
  • 📚 The topic is related rates, which involves finding the rate at which one quantity changes with respect to another.
  • 🔍 Given the rate of change of volume, the task is to find the rate at which the surface area of a cube changes.
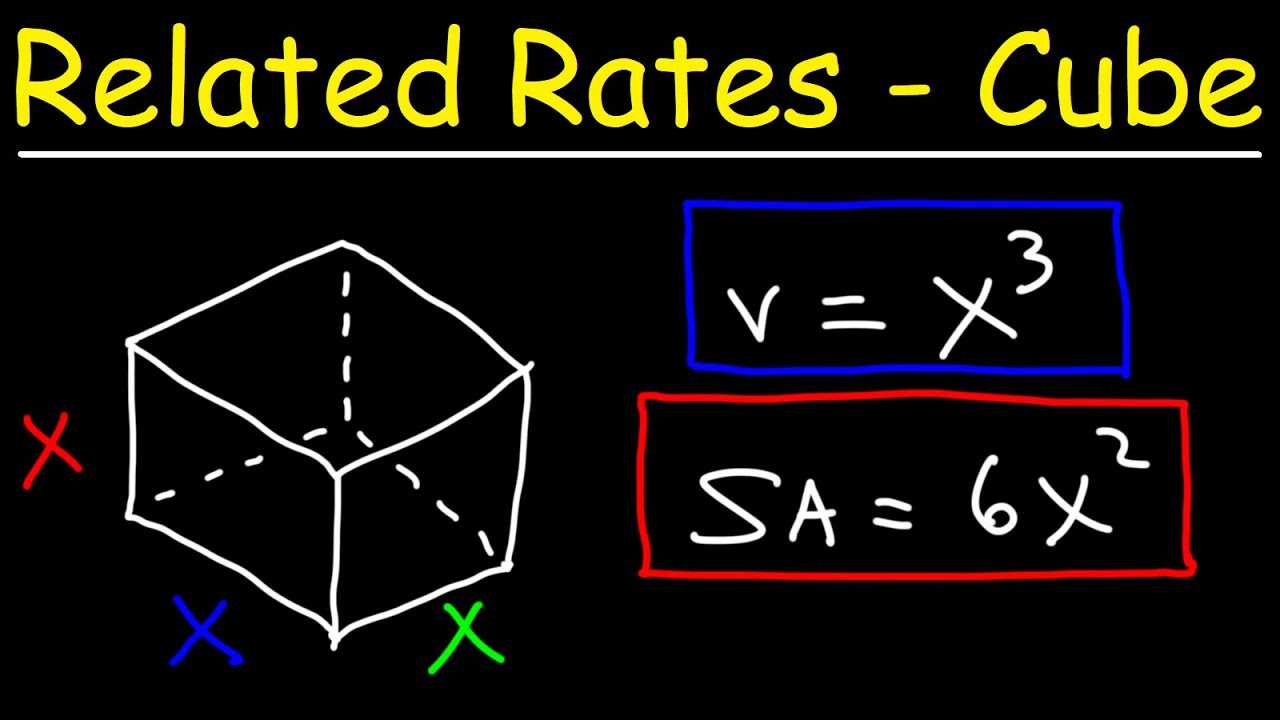
  • 🧮 The volume of a cube is calculated as \( V = X^3 \) cubic centimeters, where \( X \) is the length of a side.
  • 📏 The surface area of a cube is the sum of the areas of its six faces, given by \( A = 6X^2 \) square centimeters.
  • 🔗 To connect volume and surface area, express \( X \) in terms of \( V \) and \( A \), resulting in \( X = \sqrt[3]{V} \) and \( X = \sqrt{\frac{A}{6}} \).
  • ⚖️ By equating the two expressions for \( X \), derive the relationship \( V^{\frac{2}{3}} = \frac{A}{6^{\frac{2}{3}}} \).
  • 💡 To simplify, raise both sides to the sixth power to get rid of the roots, resulting in \( V^2 = \frac{A^3}{216} \).
  • 📌 Differentiate both sides of the equation with respect to time to find \( \frac{dA}{dt} \) in terms of \( \frac{dV}{dt} \).
  • 🔑 Use the chain rule to find \( \frac{dA}{dV} \), which simplifies to \( \frac{4}{X} \) when considering the cube root relationship.
  • ⏱ Given \( \frac{dV}{dt} = 4 \) cm³/min, substitute \( X = 3 \) to find the rate of change of surface area, \( \frac{dA}{dt} = \frac{4}{3} \) cm²/min.
  • 📝 The final answer to the problem is that the surface area of the cube increases at a rate of \( \frac{16}{3} \) cm²/min when \( X = 3 \).
  • 🌟 The video script emphasizes the importance of continuous learning, suggesting that those who stop learning cease to live.
Q & A

  • What is the topic of the video?

    -The video is about related rates, a topic in calculus that deals with the rate at which one quantity changes in relation to another.

  • What is the rate at which the volume of a cube is increasing in the given problem?

    -The volume of the cube is increasing at a rate of 4 cm³ per minute.

  • What is the formula for the volume of a cube?

    -The volume (V) of a cube is given by the formula V = X³, where X is the length of a side of the cube.

  • How is the surface area of a cube calculated?

    -The surface area (A) of a cube is the sum of the areas of its six faces, which is given by the formula A = 6X², where X is the length of a side of the cube.

  • What mathematical concept is used to connect the rate of change of volume with the rate of change of surface area?

    -Differentiation is used to connect the rate of change of volume with the rate of change of surface area.

  • What is the relationship between the volume and the surface area of a cube?

    -The relationship between the volume (V) and the surface area (A) of a cube is given by the equation A³ = 216V², after eliminating the cube root and square root by raising both sides of the initial equation to the sixth power.

  • What does the rate of change of the surface area with respect to time (da/dt) represent?

    -The rate of change of the surface area with respect to time (da/dt) represents how fast the surface area of the cube is increasing per minute.

  • How is the rate of change of the surface area with respect to the volume (da/dv) derived?

    -The rate of change of the surface area with respect to the volume (da/dv) is derived by differentiating the volume-surface area relationship with respect to V, which results in da/dv = 4/X.

  • What is the final expression for the rate of change of the surface area with respect to time?

    -The final expression for the rate of change of the surface area with respect to time is da/dt = (da/dv) * (dv/dt) = (4/X) * (4 cm³/min).

  • What is the rate at which the surface area of the cube is increasing when the side length is 3 cm?

    -When the side length (X) is 3 cm, the rate at which the surface area is increasing is da/dt = 16/3 cm²/min.

  • What is the significance of using the cube root and square root in this problem?

    -The cube root and square root are used to express the side length (X) in terms of the volume (V) and the surface area (A), which allows for the creation of an equation that can be differentiated to find the rates of change.

  • Why is it important to consider the positive value for the side length of the cube?

    -The side length of a cube must be positive because a cube, being a three-dimensional geometric shape, cannot have negative side lengths.

Outlines
00:00
📐 Introduction to Related Rates and Calculating Changes in Cube Dimensions

This paragraph introduces the concept of related rates, a calculus principle used to find the rate at which one quantity changes in relation to another. The scenario presented involves a cube whose volume is increasing at a rate of 4 cm³ per minute, and the task is to find the rate at which the surface area of the cube is increasing. Key concepts needed to solve this problem include understanding the formulas for the volume and surface area of a cube, basic differentiation, and setting up relations between different rate changes using these formulas.

05:01
🔢 Solving for Surface Area Change Rate Using Calculus

This paragraph provides a detailed step-by-step calculation to determine how the surface area of the cube changes as its volume increases. The process involves expressing the side length of the cube in terms of volume and area, then relating these expressions to find a common variable. The final calculation involves differentiating to find the rate of change of the surface area with respect to time, given the rate of change of the volume. The example concludes with a practical application of these concepts to find the specific rate at which the surface area changes when the side of the cube is 3 cm, leading to a solution of 16/3 cm² per minute.

Mindmap
Keywords
💡Related Rates
Related rates is a topic in calculus that deals with finding the rate at which one quantity changes with respect to another. In the context of the video, it is used to determine the rate of change of the surface area of a cube when the rate of change of its volume is given. The concept is central to the video's theme as it guides the entire problem-solving process.
💡Volume of a Cube
The volume of a cube is calculated by raising the length of one of its sides to the third power (V = X^3). In the video, the volume is used as a starting point to establish a relationship with the surface area. It is mentioned that the volume is increasing at a rate of 4 cm³ per minute, which is a key piece of information for solving the related rates problem.
💡Surface Area of a Cube
The surface area of a cube is the sum of the areas of all six faces, with each face having an area of X^2 (since all sides are equal in a cube). The total surface area is thus 6 * X^2. In the video, the surface area is the quantity whose rate of change we are trying to find. It is directly connected to the volume through mathematical relationships and differentiation.
💡Differentiation
Differentiation is a mathematical process used to find the rate at which a quantity changes. In the context of the video, differentiation is used to connect the rate of change of the volume with the rate of change of the surface area of the cube. It is a fundamental operation in calculus that allows us to find the derivative of an equation, which in this case helps us solve for the rate of change of the surface area.
💡Cube Root
The cube root of a number is a value that, when multiplied by itself three times, gives the original number. In the video, the cube root is used to express the side length of the cube in terms of its volume (X = cube root of V). This mathematical operation is crucial for establishing the relationship between the volume and the side length of the cube.
💡Square Root
The square root of a number is a value that, when multiplied by itself, gives the original number. In the script, the square root is used in the transformation of the area equation to express the side length of the cube (X = square root of A/6). It is used to simplify the relationship between the surface area and the volume of the cube.
💡Linearity
Linearity in this context refers to the property that the rate of change of one quantity with respect to another is directly proportional. The video uses linearity in the sense that the rate of change of the surface area (dA/dt) is directly related to the rate of change of the volume (dV/dt) through a constant multiplier, which is derived from the cube root relationship between the volume and the side length of the cube.
💡Differential Equation
A differential equation is a mathematical equation that involves derivatives, or rates of change. In the video, the relationship between the volume and surface area of the cube is expressed as a differential equation (dA/dV = 4/X). This equation is then used to find the rate at which the surface area is changing with respect to time (dA/dt).
💡Least Common Multiple (LCM)
The least common multiple of two or more numbers is the smallest number that is a multiple of all of them. In the video, the LCM is used to eliminate the cube root and square root when connecting the volume and surface area equations by raising both sides to the sixth power. This simplifies the equation and allows for easier differentiation and solution.
💡Substitution
Substitution is a method used in mathematics to replace a complex expression with a simpler variable or symbol. In the video, substitution is used to replace the expressions for the side length of the cube in terms of volume and area with a single variable (X), which simplifies the process of finding the rate of change of the surface area.
💡Rate of Change
The rate of change is the speed at which one quantity changes with respect to another. In the video, the rate of change is a central concept, as the problem is to find the rate at which the surface area of the cube increases as the volume increases at a known rate. The rate of change is represented by the derivative with respect to time (dA/dt).
Highlights

The topic is related rates, where you're given the rate at which one quantity is changing and asked to find the rate at which another is changing

For this problem, the volume of a cube is increasing at a rate of 4 cm³/min and we need to find the rate at which the surface area is increasing

Key concepts needed are computing the volume and surface area of a cube, and differentiation

The volume of a cube is given by X³ cm³ where X is the side length

The surface area of a cube is the sum of the areas of its 6 faces, which is 6X² cm²

To connect volume and surface area, express X in terms of both V (volume) and A (surface area)

X = cube root of V and X = sqrt(A/6)

Set the two expressions for X equal to each other to relate V and A: cube root of V = sqrt(A/6)

Raise both sides to the 6th power to eliminate roots: V² = (A/6)³

Solve for A in terms of V: A = (216)^(1/3) * V^(2/3)

Differentiate the equation relating A and V with respect to time to find dA/dt in terms of dV/dt

dA/dV = 4/X, and given dV/dt = 4 cm³/min, we can find dA/dt = (4/X) * (4 cm³/min)

Substitute X = 3 cm (given in the problem) to find dA/dt = 16/3 cm²/min

So the rate at which the surface area of the cube is increasing is 16/3 cm²/min

Key takeaway: Use related rates to find rates of change for different quantities that are functions of each other

Differentiate the equation relating the quantities with respect to the changing variable to find the rate of change

Always express everything in terms of the changing variable, then differentiate and solve for the desired rate

This technique is useful in many real-world applications involving rates of change

Never stop learning - those who stop learning have stopped living

Transcripts

Browse More Related Video

AP CALCULUS AB 2022 Exam Full Solution FRQ#4d

Related Rate Problems - The Cube - Volume, Surface Area & Diagonal Length

Solve with changing area using related rates

AP CALCULUS AB 2022 Exam Full Solution FRQ#2d

Optimization Example: Minimizing Surface Area Given a Fixed Volume

Learn how to find the change in theta for a triangle, related rates

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Related RatesCalculusCube VolumeSurface AreaRate of ChangeMathematicsDifferentiationLearningEducational ContentSTEMMath Problem