AP Physics Workbook 5.E Conservation of momentum in Inelastic Collisions
TLDRThe video script discusses the concept of conservation of momentum in physics, using the example of a toy car and a truck. It explains the difference between elastic and inelastic collisions, and how momentum is represented graphically. The scenario involves calculating the final momentum after a collision, emphasizing that in a perfect inelastic collision, momentum is conserved but kinetic energy is lost. The script also explores a hypothetical situation where two identical toy cars collide and stick together, resulting in zero final momentum. The explanation is clear, detailed, and educational, providing a solid understanding of the principles of momentum conservation.
Takeaways
- 🌟 The concept of conservation of momentum is crucial in understanding how momentum behaves in different collision scenarios.
- 🚗 In the given scenario, two objects - a big container car and a small toy car - are considered as one system for analysis.
- 📈 The initial momentum of the system is determined by the product of mass and velocity for each object, represented visually as areas on a diagram.
- 🔄 The final momentum of the system after a collision depends on the type of collision - elastic or inelastic.
- 💥 In an elastic collision, objects bounce off each other with momentum conserved but kinetic energy lost.
- 🧱 In an inelastic collision, objects stick together, conserving momentum while losing kinetic energy and potentially converting it into other forms.
- 🎯 The final velocity and momentum of the system can be calculated by considering the direction and magnitude of the initial momenta.
- 🔽 When identical toy cars collide at identical speeds in opposite directions, the total initial momentum is zero, leading to a final momentum of zero post-collision.
- 📊 The script uses diagrams and mathematical calculations to illustrate the changes in momentum and energy before and after collisions.
- 📝 The example provided in the script helps to understand the practical application of the conservation of momentum principle in real-world scenarios.
- 🌐 The principles discussed are fundamental to the study of physics, particularly in the area of mechanics and collision dynamics.
Q & A
What is the main topic of the video?
-The main topic of the video is the concept of conservation of momentum in physics, specifically focusing on collisions between objects.
How many objects are mentioned in the scenario?
-There are two objects mentioned in the scenario: a big container car and a toy car (M).
What is the positive direction defined as in the video?
-In the video, the positive direction is defined as to the right and up.
What is the mass and velocity of the toy car?
-The mass of the toy car is 1, and its velocity is given as 5, in the negative direction (left).
How is momentum represented in the video?
-Momentum is represented by the area under a velocity-time graph, which is calculated as mass times velocity.
What are the two types of collisions explained in the video?
-The two types of collisions explained in the video are elastic collisions, where objects rebound away from each other, and inelastic collisions, where objects stick together after colliding.
What happens to kinetic energy in a perfectly inelastic collision?
-In a perfectly inelastic collision, kinetic energy is conserved, but there is a loss of thermal energy.
What is the initial momentum of the system with the toy car and the truck?
-The initial momentum of the system with the toy car and the truck is zero because they are not moving before the collision.
What is the final momentum of the system after the toy car and the truck collide and stick together?
-The final momentum of the system after the collision is in the positive direction to the right, with a value of 10 kilogram meter second.
How does the video explain the outcome if two identical toy cars collide at identical speeds in opposite directions?
-If two identical toy cars collide at identical speeds in opposite directions, the total momentum before and after the collision remains zero, as the cars will stick together and stop moving.
What principle is used to explain the final velocities of the cars in the perfectly inelastic collision scenario?
-The principle used to explain the final velocities of the cars is Newton's first law, which states that an object will remain at rest or in uniform motion unless acted upon by an external force.
Outlines
📚 Introduction to Momentum Conservation
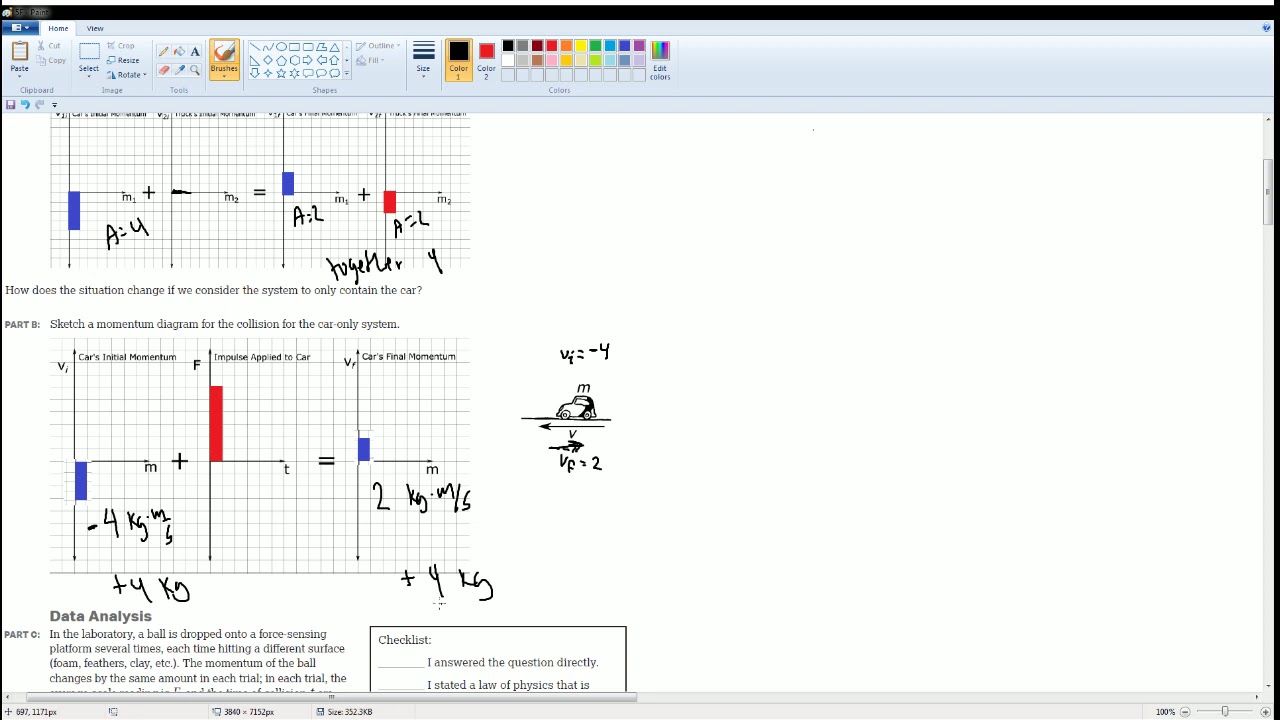
This paragraph introduces the concept of momentum conservation in the context of a physics workbook, specifically focusing on unit 5, section 5.8. It explains the scenario presented in Part B, where two objects - a big container car and a toy car (M) - are considered as a single system. The paragraph details the process of identifying the system, drawing the situation with designated positive directions, and calculating the momentum of the toy car with given mass and velocity. It also touches upon the concept of mass and velocity in determining momentum, as well as the types of collisions (elastic and inelastic) and the conservation of momentum in inelastic collisions where objects stick together. The importance of understanding the conservation of momentum and the loss of kinetic energy in inelastic collisions is emphasized.
🚗 Analysis of Collision Outcomes
This paragraph delves into the analysis of the outcomes of collisions, particularly focusing on the change in kinetic energy and the direction of travel after a collision. It explains how the total momentum (P_total) is represented and how it changes from an initial state to a final state after a collision. The paragraph clarifies that in the absence of external forces, the total momentum after a collision must be equal to the initial momentum. It also discusses the scenario where a toy car and a truck collide and stick together, resulting in a change in kinetic energy and a shift in the direction of travel. The paragraph further illustrates the initial and final states of momentum using a diagram and explains the energy dynamics before and after the collision, highlighting the conservation of momentum and the absence of external work in an ideal spring scenario.
🔄 Collision of Identical Objects
This paragraph discusses a hypothetical scenario where two identical toy cars collide. It explains how the momentum would be represented in a diagram and how it changes before and after the collision. The paragraph emphasizes that since the cars have equal mass and opposite velocities, the total initial momentum of the system is zero. It then describes the outcome of the collision, where the cars stick together and come to a stop, resulting in a final momentum of zero. The explanation includes a mathematical breakdown of how the momentum of the two cars cancels each other out, leading to a final velocity of zero. The paragraph concludes by reinforcing the concept of momentum conservation in collisions involving identical objects with opposite velocities.
Mindmap
Keywords
💡Momentum
💡Conservation of Momentum
💡Elastic Collision
💡Inelastic Collision
💡Positive and Negative Direction
💡Velocity
💡Mass
💡Collision
💡Kinetic Energy
💡Area Representation
💡Final Momentum
💡Explosive Scenario
Highlights
The introduction of the concept of momentum in the context of AP Physics workbook (Unit 5, Section 5.8).
Identification of the system as the combination of a big container car and a toy car.
Explanation of labeling the right direction as positive and up as positive for the scenario.
Description of the small car's negative velocity direction and its representation in the problem.
Calculation of the small car's momentum using its mass and velocity.
Discussion of the big car's mass and velocity in the context of momentum.
Explanation of the conservation of momentum in the system after the collision where the cars stick together.
Differentiation between elastic and inelastic collisions, and the loss of thermal kinetic energy in inelastic collisions.
Illustration of how momentum is conserved in a perfect inelastic collision with an example.
Explanation of the direction of travel after the collision based on the conservation of momentum.
Presentation of a detailed analysis of the momentum before and after the collision between the toy car and the truck.
Clarification that the initial momentum of the system is zero due to the car and truck being at rest.
Description of the energy before and after the explosion when the toy car and truck are compressed by an ideal spring.
Explanation of the final energy state after the toy car and truck break apart from the spring potential energy.
Discussion of the impact of mass on kinetic energy and velocity in the context of the two identical toy cars collision.
Demonstration of how the momentum in the diagram would change if two identical toy cars were traveling at identical speeds in opposite directions.
Conclusion that the momentum after the collision between two identical cars would be zero due to the cancellation of their momenta.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: