Differentiating Between Elastic and Inelastic Collisions | Physics in Motion
TLDRThis engaging video segment from 'Physics In Motion' explores the physics behind collisions, focusing on the critical concepts of momentum and kinetic energy transfer. It distinguishes between elastic and inelastic collisions, emphasizing that inelastic collisions involve energy conversion into heat, sound, and deformation, while elastic collisions conserve both momentum and kinetic energy without energy loss. Through practical examples, such as a paintball hitting a barrier and an elastic collision with exercise balls, the video illustrates how to calculate final velocities and the conservation of momentum. The segment concludes with a problem-solving demonstration, reinforcing the principles discussed and providing a deeper understanding of collision dynamics.
Takeaways
- 🚨 Collisions in physics involve the transfer of momentum or kinetic energy from one object to another.
- 🌟 Kinetic energy is the energy of motion, while momentum is the quantity of that motion.
- 🔍 Collisions are categorized as either elastic or inelastic based on the conservation of kinetic energy.
- 🔄 In an inelastic collision, momentum is conserved but kinetic energy is not, with some energy converted into heat, sound, and deformation.
- 📌 In a perfectly inelastic collision, objects stick together and move with the same final velocity.
- 🎯 For the paintball and barrier example, the final velocity is calculated by dividing the total momentum by the combined mass of the system.
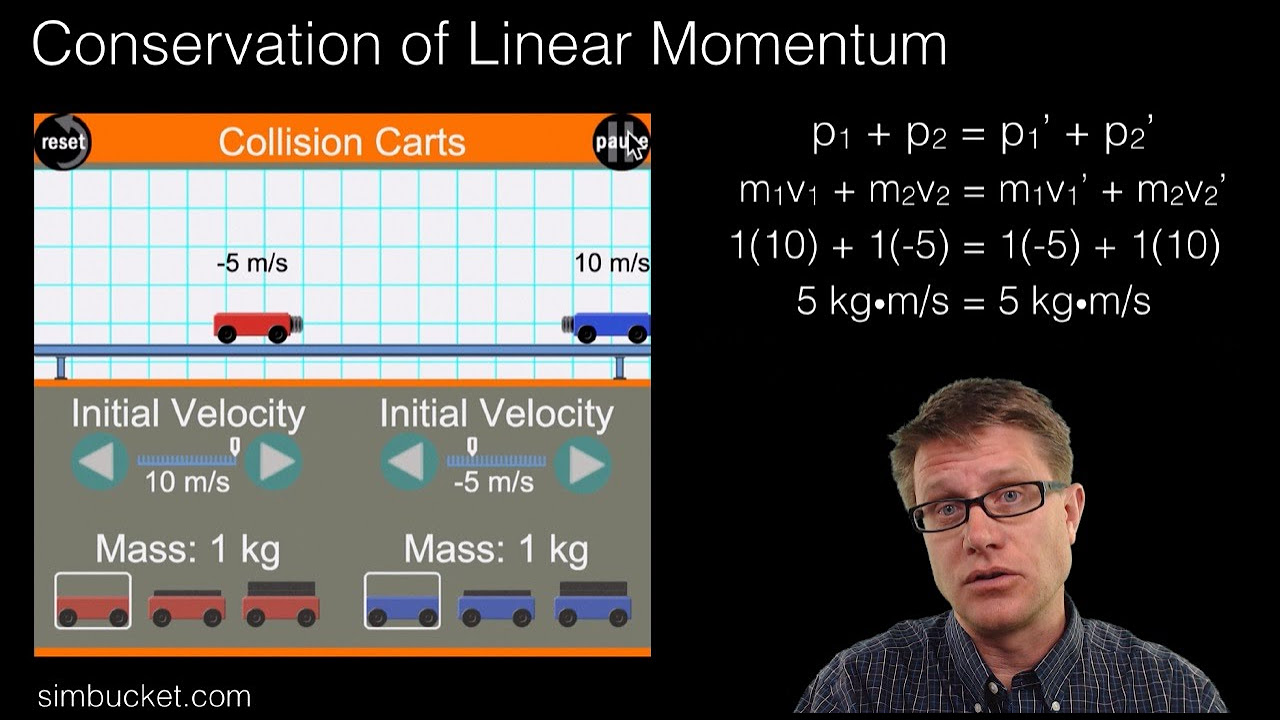
- 🤹 In an elastic collision, both momentum and kinetic energy are conserved, with no energy lost to sound, heat, or deformation.
- 🏋️♂️ An example of an elastic collision involves Summer and Tom with rubber exercise balls, where they bounce off each other.
- 📐 The momentum before the collision equals the momentum after the collision, which is used to solve for final velocities in elastic collision problems.
- ⚖️ The final velocity of objects in an elastic collision can be found by applying the conservation of momentum principle.
- 🔢 The mass of objects involved in a collision, along with their velocities before and after the collision, are crucial for calculating momentum.
- ⏹ The final velocities in collisions indicate the direction and magnitude of motion after the impact has occurred.
Q & A
What is a collision in the context of physics?
-In physics, a collision occurs when momentum or kinetic energy is transferred from one object to another.
What are the two types of collisions?
-The two types of collisions are elastic and inelastic.
What happens to kinetic energy in an inelastic collision?
-In an inelastic collision, kinetic energy is not conserved. Some of it is converted into heat, sound, and deformation of the objects.
What is the final velocity of the paintball and the barrier after the inelastic collision in the example?
-The final velocity of the paintball and the barrier after the inelastic collision is 0.03 meters per second.
How is momentum calculated in an object?
-Momentum is calculated as the mass of the object multiplied by its velocity.
What is conserved in an elastic collision?
-In an elastic collision, both momentum and kinetic energy are conserved.
What is the condition for objects after a perfectly inelastic collision?
-In a perfectly inelastic collision, the objects stick together, resulting in the same final velocities.
What is the initial momentum of the system in the example with Summer and Tom?
-The initial momentum of the system is 125 kilograms meters per second.
What is Tom's final velocity after the elastic collision with Summer?
-Tom's final velocity after the elastic collision is 3 meters per second.
Why is the velocity of the paintball and the barrier eventually at rest?
-After the collision, the paintball and the barrier slow down due to external forces like friction and air resistance until they come to a stop at 0 meters per second.
How does the conservation of momentum principle apply to the problem with Summer and Tom?
-The conservation of momentum principle states that the total momentum before the collision (P_sub_I) is equal to the total momentum after the collision (P_sub_F). This principle is used to solve for the final velocities of Summer and Tom after the collision.
What is the significance of the negative sign in the calculation of Tom's velocity?
-The negative sign indicates that Tom's direction of motion is opposite to Summer's. This is important in calculating the total momentum of the system and in determining the direction of motion after the collision.
Outlines
🚨 Understanding Collision Dynamics 🚨
This paragraph delves into the physics of collisions, explaining the concepts of momentum and kinetic energy transfer during an impact. It distinguishes between elastic and inelastic collisions, with the latter involving no bounce and energy conversion into heat, sound, and deformation. An example of an inelastic collision is provided using a paintball and a barrier, with a step-by-step calculation of the final velocity of the system post-collision. The paragraph also touches on perfectly inelastic collisions where objects stick together, sharing the same final velocity.
🏋️♂️ Elastic Collisions with Summer and Tom 🏋️♀️
The second paragraph focuses on elastic collisions, where both momentum and kinetic energy are conserved, and no energy is lost to sound, heat, or deformation. Using an example with Summer and Tom running and colliding while holding rubber exercise balls, the paragraph illustrates how to calculate final velocities in an elastic collision scenario. The conservation of momentum is applied to find Tom's final velocity after the collision, with detailed calculations provided. The outcome demonstrates the principles of an elastic collision, where objects bounce off each other, and the system's kinetic energy remains unchanged.
Mindmap
Keywords
💡Collision
💡Momentum
💡Kinetic Energy
💡Elastic Collision
💡Inelastic Collision
💡Conservation Laws
💡Mass
💡Velocity
💡Deformation
💡Heat
💡Sound
Highlights
Safety test crashes are used to understand the power of collisions involving tons of metal at high speeds.
Collisions in physics involve the transfer of momentum or kinetic energy from one object to another.
Kinetic energy is the energy of motion, and momentum is the quantity of that motion.
Collisions are categorized as either elastic or inelastic based on the conservation of kinetic energy and momentum.
In an inelastic collision, objects do not bounce away, momentum is conserved but kinetic energy is not.
Some kinetic energy in an inelastic collision is converted into heat, sound, and deformation.
In a perfectly inelastic collision, objects stick together and share the same final velocity.
An example of an inelastic collision is a paintball hitting a barrier and sticking to it.
The final velocity of objects in an inelastic collision can be calculated using the conservation of momentum.
The momentum of a system before and after a collision remains the same, allowing for the calculation of final velocities.
In an elastic collision, both momentum and kinetic energy are conserved, with no energy loss due to sound, heat, or deformation.
An elastic collision is characterized by objects bouncing apart, with no permanent change in shape or size.
An example of an elastic collision is demonstrated with two people holding rubber exercise balls running towards each other.
Conservation of momentum is applied to calculate the final velocities of objects in an elastic collision.
The final velocities in an elastic collision indicate the direction and magnitude of motion after the collision.
The segment concludes with a clear understanding of the definition of a collision and the differences between elastic and inelastic collisions.
The 'Physics In Motion' toolkit offers additional resources for practice problems, lab activities, and note-taking guides.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: