Fundamental Theorem of Calculus Parts 1&2
TLDRThe video script provides an in-depth explanation of the Fundamental Theorem of Calculus, highlighting its two key parts. Part one establishes the connection between continuous functions and their integrals, showing that the derivative of an integral is the upper limit of the function. Part two addresses the integration of a function over a constant interval, stating that the result is the function evaluated at the upper limit minus the function at the lower limit. The video uses examples to clarify these concepts, emphasizing the importance of understanding the relationship between integration and differentiation.
Takeaways
- 📚 The Fundamental Theorem of Calculus (FTC) establishes a connection between differentiation and integration, also known as anti-differentiation.
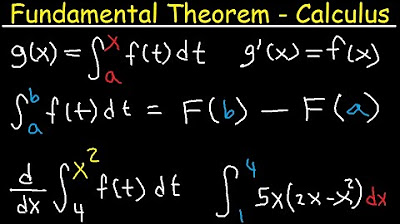
- 🔄 FTC Part 1 states that if a function f is continuous on a closed interval [a, b], then the derivative of the integral from a to x is f(x).
- 📈 FTC Part 2 indicates that the integral of a function f from a to b is F(b) - F(a), where F(x) is the antiderivative of f(x).
- 🌟 Anti-differentiation is the process of finding a function whose derivative is a given function, essentially the reverse of differentiation.
- 🔢 The first part of the FTC is often overlooked, but understanding it is crucial for solving complex calculus problems.
- 🎓 When differentiating the integral, the constant lower limit is replaced by zero to simplify the expression.
- 🔄 Swapping the limits of integration requires a negative sign to be introduced, as subtraction is not commutative.
- 📊 If the upper limit is a function, the chain rule or u-substitution must be applied to find the derivative of the integral.
- 🧠 Understanding the FTC is essential for grasping the relationship between rates of change and accumulation of quantities in calculus.
- 🌐 The FTC is a cornerstone of calculus, with wide applications in various fields such as physics, engineering, and economics.
- 🚀 Continuous learning is encouraged, as it is the key to understanding complex concepts like the FTC and its applications.
Q & A
What is the main topic of the video?
-The main topic of the video is the Fundamental Theorem of Calculus, specifically Part 1 and Part 2, and how they establish the connection between integration and differentiation.
What does the term 'anti-differentiation' imply?
-Anti-differentiation refers to the process that is reverse to differentiation. It is used to describe the act of finding the integral of a function, which, when differentiated, should return the original function.
What is the condition for a function to be integrable according to the Fundamental Theorem of Calculus Part 1?
-For a function to be integrable, it must be continuous on a closed interval [a, b]. Continuity implies that the function is differentiable over that interval.
How does the Fundamental Theorem of Calculus Part 1 relate to the derivative of an integral?
-The Fundamental Theorem of Calculus Part 1 states that if a function is continuous on [a, b], then the derivative of the integral of that function with respect to x is simply the function evaluated at x, which is f(x).
What does the Fundamental Theorem of Calculus Part 2 state?
-Part 2 states that if you integrate a function between two constants a and b, the value of the integral is the function evaluated at the upper limit minus the function evaluated at the lower limit, i.e., ∫[a to b] f(x) dx = f(b) - f(a).
How does the concept of 'variable on top and constant under' apply to the first part of the FTC?
-For the first part of the FTC to be relevant, there must be a constant under the integral and a variable on top. This means that the lower limit of integration is a constant, and the upper limit is the variable with respect to which we are taking the derivative.
What is the significance of the chain rule when the upper limit is a function?
-When the upper limit is a function, the chain rule is applied to find the derivative of the integral. This involves taking the derivative of the outer function and multiplying it by the derivative of the inner function with respect to the variable.
How does the video script illustrate the application of the Fundamental Theorem of Calculus in solving problems?
-The video script provides several examples where the Fundamental Theorem of Calculus is used to find the derivative of an integral. It shows how to apply the theorem in various scenarios, including when the upper and lower limits are variables, constants, or functions.
What is the role of the substitution method in the context of the FTC?
-The substitution method is used when the upper limit is a function of the variable. It involves replacing the function with a new variable (u-substitution), and then using the chain rule to find the derivative of the integral.
Why is it important to understand the Fundamental Theorem of Calculus?
-Understanding the Fundamental Theorem of Calculus is crucial because it reveals the deep connection between differentiation and integration, two fundamental operations in calculus. It provides a powerful tool for solving a wide range of problems in mathematics and its applications.
How does the video emphasize the importance of continuous learning in the context of calculus?
-The video ends with the statement 'never stop learning those who stop learning have stopped living,' emphasizing that continuous learning is essential for mastery and understanding of complex subjects like calculus.
Outlines
📚 Introduction to the Fundamental Theorem of Calculus
This paragraph introduces the topic of the Fundamental Theorem of Calculus (FTC), emphasizing its importance in understanding the relationship between integration and differentiation. The speaker explains that the FTC reveals a connection between these two concepts, often referred to as anti-differentiation, which is essentially the reverse process of differentiation. The explanation begins with the basic premise that if a function f is continuous on a closed interval [a, b], it is differentiable over that interval, and thus, one can define an integral function F(x) that represents the area under the curve from a to x. The speaker then delves into the specifics of the FTC, part one, which states that the derivative of the integral function F(x) with respect to x is the original function f(x). This is a foundational concept that is crucial for comprehending the rest of the discussion.
📈 Explanation of FTC Part 1 and Part 2
The paragraph continues with a deeper exploration of the FTC, focusing on both its first and second parts. The first part is clarified as stating that the derivative of an integral from a lower limit to an upper limit (x) of a function f(t) dt, with respect to x, is simply the function evaluated at the upper limit, which in this case is f(x). The second part of the FTC is introduced as a practical tool for integration, stating that the integral of a function f(x) between two constants a and b is the value of the function at the upper limit minus its value at the lower limit, f(b) - f(a). The speaker uses examples to illustrate how these two parts of the FTC simplify the process of finding derivatives and integrals, respectively, and emphasizes the importance of understanding these concepts to solve calculus problems effectively.
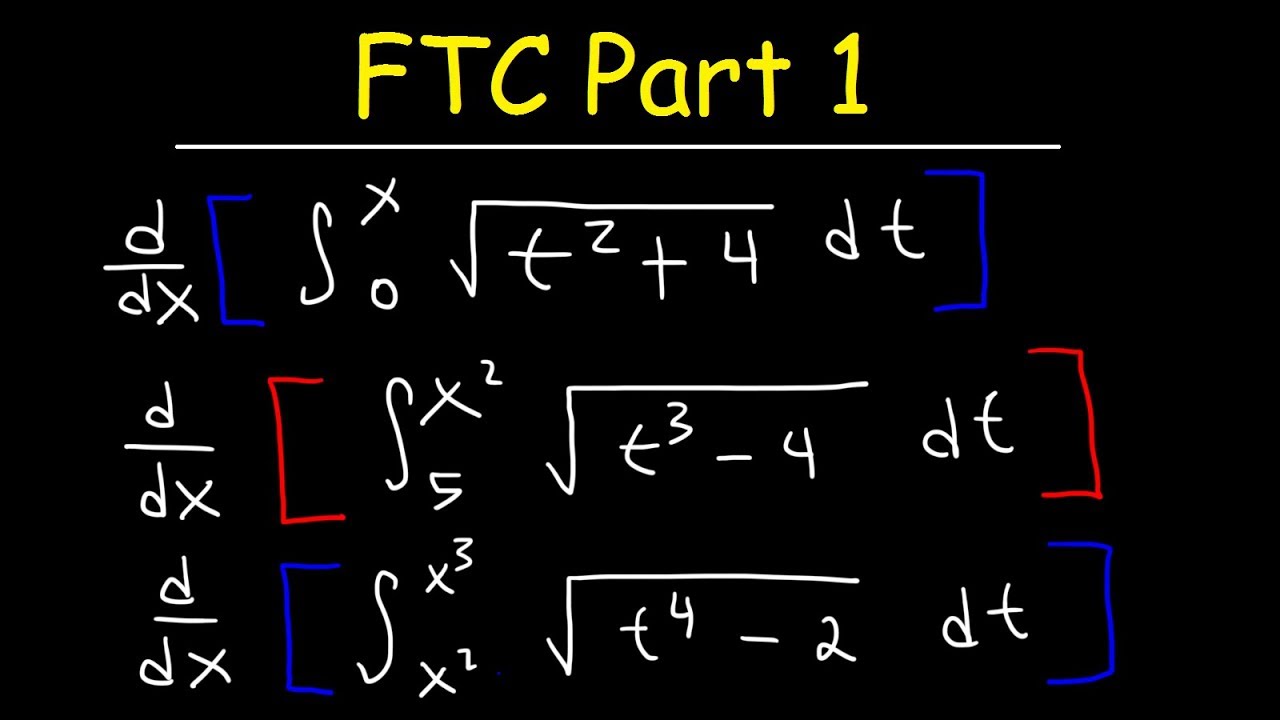
🔢 Application of FTC with Variable and Function Limits
This section discusses the application of the FTC in scenarios where the limits of integration are a variable and a function. The speaker explains how to handle these situations by applying the chain rule and the concept of u-substitution. The explanation includes a detailed walkthrough of how to transform the integral expression to isolate the variable and how to apply the FTC to find the derivative of the integral. The speaker also addresses the importance of recognizing when to apply the chain rule, particularly when the upper limit is a function of the variable. The examples provided in this paragraph serve to reinforce the application of the FTC in more complex integral problems and demonstrate the versatility of the theorem in various mathematical contexts.
🎓 Advanced FTC Applications and Conceptual Understanding
The final paragraph of the script focuses on advanced applications of the FTC, including handling integrals with functions as both the upper and lower limits. The speaker presents a complex example involving the function cosine(x) and demonstrates how to break down the problem into manageable parts using the FTC. The explanation includes the use of negative signs and the application of the chain rule when the upper limit is a function of x. The speaker emphasizes the importance of continuous learning in mathematics and encourages viewers to keep exploring and understanding new concepts. The summary concludes with a final expression derived from the application of the FTC, encapsulating the essence of the theorem's utility in solving calculus problems.
Mindmap
Keywords
💡Fundamental Theorem of Calculus
💡Integration
💡Differentiation
💡Anti-differentiation
💡Continuous Function
💡Derivative
💡Closed Interval
💡Upper Limit
💡Lower Limit
💡Chain Rule
Highlights
The video discusses the Fundamental Theorem of Calculus, which connects integration and differentiation.
Part one of the theorem states that if a function is continuous on a closed interval, it can be integrated from the start point to any point within the interval.
Differentiating the integral of a continuous function on a closed interval returns the original function.
The derivative of an integral is the upper limit of the function, as per the first part of the theorem.
The second part of the theorem explains that the integral of a function between two constants is the value of the function at the upper limit minus its value at the lower limit.
The video demonstrates the application of the Fundamental Theorem of Calculus to solve four different integration problems.
In the first example, the integral of t squared from one to x results in f(x), and its derivative is x squared.
The second example involves flipping variables to apply the first part of the theorem and results in 3x.
The third example uses the chain rule and u-substitution to handle an upper limit that is a function of x, yielding 2x^7.
The fourth example involves integrating a function with a variable in the bottom and a function on top, resulting in -x minus sine x times cosine x.
The video emphasizes the importance of understanding the first part of the theorem to solve a variety of integration problems.
The chain rule is applied when the upper limit is a function of the variable, as shown in the third example.
The video provides a clear explanation of how to apply the Fundamental Theorem of Calculus in practical problem-solving.
The concept of anti-differentiation is introduced as the reverse process of differentiation.
The video clarifies that the derivative of a constant is zero, which simplifies the process of finding derivatives of integrals.
The video uses a step-by-step approach to explain complex calculus concepts, making them accessible to learners.
The video concludes with a motivational message encouraging continuous learning in the field of mathematics.
Transcripts
Browse More Related Video

02 - Fundamental Theorem of Calculus, Part 1 - Learn Antiderivatives & Derivatives
Fundamental Theorem of Calculus Part 1
Monday Night Calculus: FTC (Fundamental Theorem of Calculus)
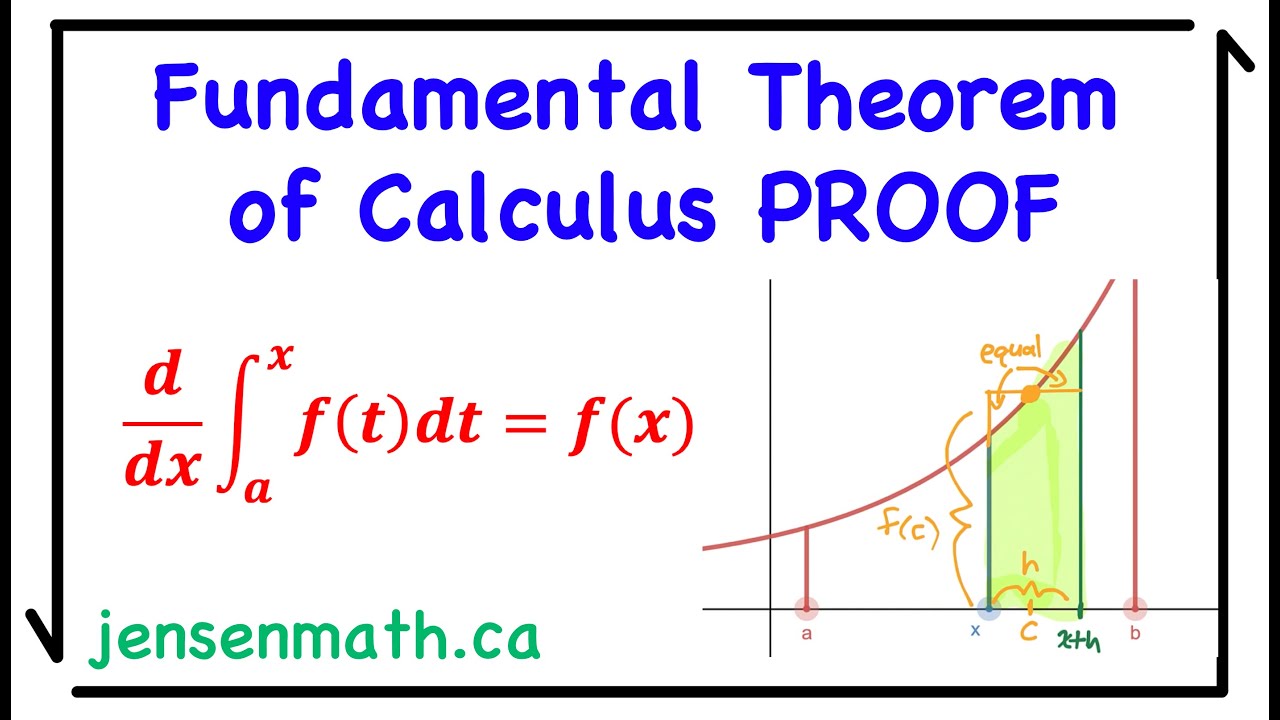
1st Fundamental Theorem of Calculus PROOF | Calculus 1 | jensenmath.ca
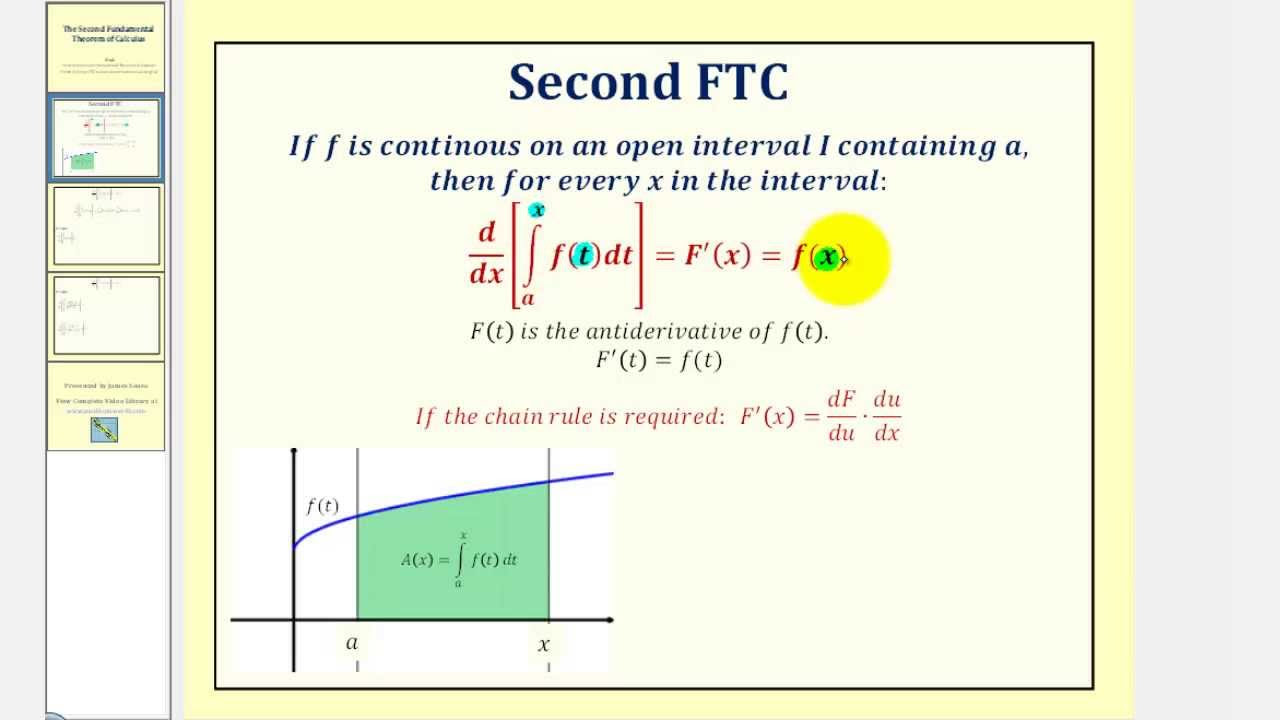
The Second Fundamental Theorem of Calculus
Fundamental Thereom of Calculus Explained - Part 1 & 2 Examples - Definite Integral
5.0 / 5 (0 votes)
Thanks for rating: