Monday Night Calculus: FTC (Fundamental Theorem of Calculus)
TLDRIn this informative session of 'Monday Night Calculus,' host Curtis Brown is joined by Steve and Tom to delve into the Fundamental Theorem of Calculus (FTC). The discussion is centered around the importance of the FTC in AP Calculus and its two parts, which connect differential and integral calculus. The first part is about the derivative of the definite integral with respect to its upper limit being the integrand evaluated at that limit, while the second part focuses on evaluating definite integrals using antiderivatives. The session includes visual aids, examples, and a Q&A format to clarify common misconceptions and emphasize the theorem's significance in understanding calculus concepts.
Takeaways
- 📚 The Fundamental Theorem of Calculus (FTC) is a central concept in AP Calculus and all of calculus, connecting differential and integral calculus.
- 🔢 FTC分为两部分:第一部分指出,如果一个函数g(x)是由定积分定义的,那么它的导数g'(x)就等于被积函数f(x)。
- 📈 利用FTC第一部分,可以直接求出由定积分得到的函数的导数,无需找到原函数的反导数。
- 🌟 FTC第二部分说明,如果函数f在闭区间[a, b]上连续,那么定积分∫[a, x] f(t) dt的导数就是f(x)。
- 📊 在讲解FTC时,可以通过图形化的方式帮助理解,例如将定积分的函数视为面积函数。
- 👀 通过图形化解释,可以帮助学生更好地理解定积分函数的导数实际上就是原函数在积分上限处的值。
- 📝 在解决涉及定积分的导数问题时,学生常见的错误包括试图找到原函数的反导数,或在导数中加入额外的求导步骤。
- 🤔 学生有时会错误地用变量t代替x来表达定积分的导数,但在考试中应使用x来表示。
- 🔄 FTC的应用不仅限于基本的定积分求导问题,还可以解决更复杂的问题,如带有变量上下限的定积分问题。
- 📱 通过实际例子(如手机电池充电问题)讲解FTC的应用,可以帮助学生更好地理解其在现实情境中的作用。
- 🚀 利用图形计算器可以直观展示定积分函数的图形,帮助学生理解这些函数的斜率和形状。
Q & A
What is the main topic of the discussion in the transcript?
-The main topic of the discussion is the Fundamental Theorem of Calculus (FTC), its importance in calculus, and its applications in various problems.
What are the two parts of the Fundamental Theorem of Calculus?
-The two parts of the Fundamental Theorem of Calculus are Part 1, which relates the derivative of the definite integral to the integrand function, and Part 2, which allows the evaluation of definite integrals using antiderivatives.
How does the Fundamental Theorem of Calculus connect differential and integral calculus?
-The FTC establishes a precise relationship between the derivative and integral, showing that differentiation and integration are inverse operations. This allows for the computation of definite integrals and finding antiderivatives of continuous functions more easily.
What is the significance of the Fundamental Theorem of Calculus in the AP Calculus course and exam?
-The FTC is a central idea in AP Calculus, as it is mentioned in the course and exam description booklet. It is considered one of the most important concepts in calculus, and students are expected to understand and apply it in various problems, including those on the AP exam.
How does the speaker visualize the function g(x) in the context of the FTC Part 1?
-The speaker visualizes g(x) as an 'area so far' function, where g(x) represents the area under the graph of the integrand function f(t) from a to x, above the x-axis.
What is a common mistake students make when applying FTC Part 1?
-A common mistake is that students may try to find the definite integral first, attempting to find an antiderivative of the function, instead of directly evaluating the integrand at the upper limit, as stated by the FTC Part 1.
What is the net change theorem mentioned in the transcript?
-The net change theorem is an interpretation of the FTC Part 2, stating that the definite integral of a rate of change function is equal to the net change in the original function as x changes from a to b.
How does the speaker use a graphing calculator to demonstrate the concept of antiderivatives?
-The speaker inputs the integrand function into the graphing calculator and uses the integral function to plot the antiderivative. By adjusting the lower and upper limits of integration, the speaker shows how the antiderivative changes and how it relates to the original function.
What is the main takeaway from the discussion about the Fundamental Theorem of Calculus in the transcript?
-The main takeaway is the importance and utility of the FTC in understanding and solving calculus problems. It highlights the connection between differentiation and integration, and how the FTC can be applied to find antiderivatives and evaluate definite integrals without needing a closed-form expression.
How does the discussion help in preparing for the AP Calculus exam?
-The discussion provides insights into the key concepts of the FTC, common mistakes to avoid, and strategies for solving problems. It also emphasizes the significance of the FTC in the AP Calculus curriculum and exam, helping students understand what to expect and how to approach related problems.
Outlines
🎓 Introduction to Monday Night Calculus
The video begins with host Curtis Brown welcoming viewers to another edition of Monday Night Calculus. He introduces the guests, Steve and Tom, and mentions the opportunity for viewers to download the questions and examples from the TI website. Curtis emphasizes the importance of feedback and the continuation of the series in the spring. The topic for the night is the Fundamental Theorem of Calculus, which Steve describes as one of his favorites and crucial to AP Calculus. The theorem's significance in connecting differential and integral calculus is highlighted, along with its ability to simplify the computation of definite integrals and to find antiderivatives of continuous functions.
📚 Explanation of the Fundamental Theorem of Calculus (Part 1)
Steve delves into the first part of the Fundamental Theorem of Calculus (FTC), providing a background and interpretation of the theorem. He explains that the theorem can be visualized by considering a continuous function g defined as the definite integral from a to x of f(t) dt. Steve clarifies that g is a function of x, with a as a constant and x varying. He uses a graphical approach to demonstrate that the derivative of g is f, explaining the concept of 'area so far' and how it relates to the function g. The graphical and analytical justification for g' being f is also discussed, along with common student errors when dealing with such problems.
📈 Application of the Fundamental Theorem of Calculus (Part 1)
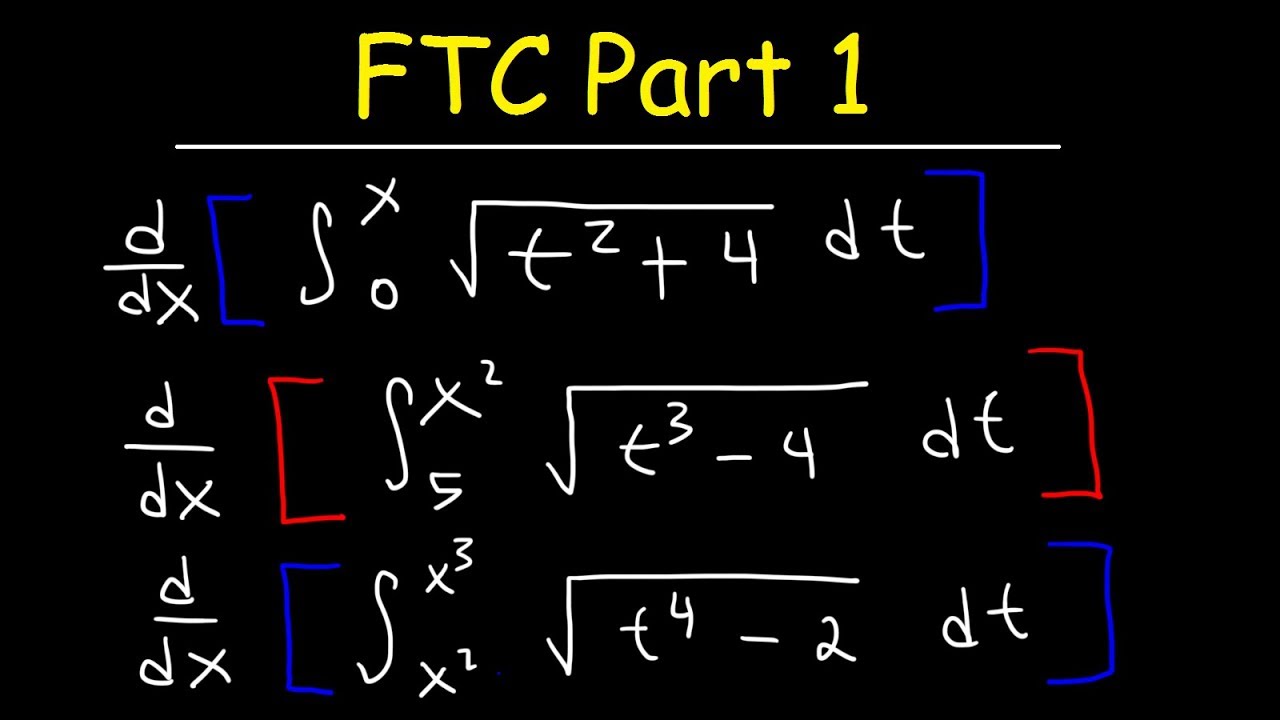
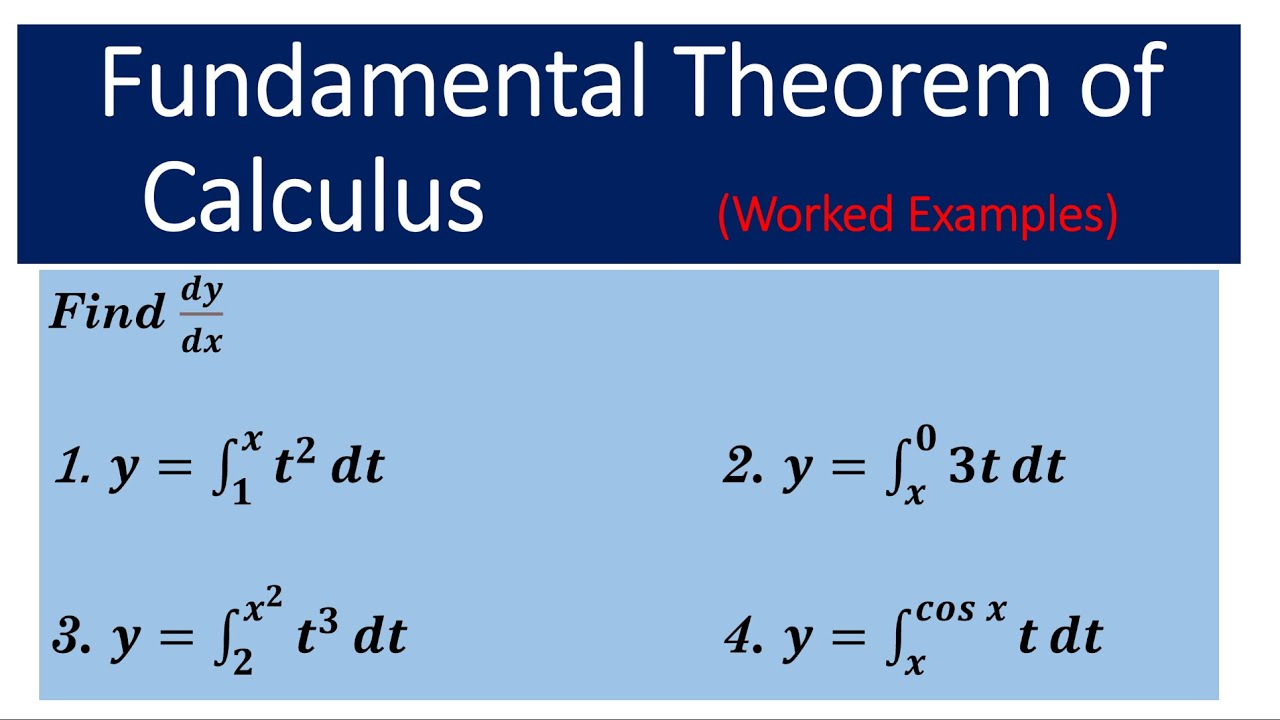
The paragraph continues with Steve applying the Fundamental Theorem of Calculus, Part 1, to find the derivatives of various functions. He emphasizes the common mistake of students trying to find the definite integral first, instead of directly applying the theorem. Curtis and Steve discuss the importance of recognizing the application of the theorem and the simplicity of substituting the variable x into the integrand. They also touch on the importance of writing answers in terms of x, especially for exam situations. The discussion concludes with a look at how the FTC can be applied to more complex problems involving functions of x as the upper limit of integration.
🌟 The Power of the Fundamental Theorem of Calculus (Part 2)
Steve introduces the second part of the Fundamental Theorem of Calculus, explaining its powerful implications. He states that if a function f is continuous on a closed interval, then the definite integral can be evaluated by finding any antiderivative of f. Steve illustrates this with an example involving the velocity and position functions, showing how the theorem can be used to calculate distance traveled. He also discusses the concept of net change and how the definite integral of a rate of change represents the net change in the original function. The paragraph ends with a mention of a cell phone battery scenario to demonstrate the practical application of the theorem.
📝 Solving Problems Using the Fundamental Theorem of Calculus
The paragraph showcases a variety of problems that utilize the Fundamental Theorem of Calculus. Steve and Curtis discuss the process of solving these problems, emphasizing the importance of practice and understanding of the theorem. They cover topics such as change of variables, definite integrals, and the application of the theorem to real-world scenarios like cell phone battery charging. The paragraph also includes a discussion on the historical discovery of the theorem, mentioning the dispute between Newton and Leibniz. The solutions to the problems are mentioned to be posted later, and the importance of the theorem in AP Calculus exams is highlighted.
🤔 Challenging Problems and Extensions
The discussion moves to more challenging problems that involve the Fundamental Theorem of Calculus. Steve presents a problem involving the derivative of an integral function and its analytical solution. He also introduces a problem with a function h defined in terms of another function g, and demonstrates how to find h(4) using g(2). The paragraph concludes with a look at a problem involving the function k(x), which is an integral from the sine of x to the cosine of x. The solution involves breaking the integral into two parts and using the FTC to find k'(x). The problem is left as a challenge for viewers to solve.
📊 Graphing Definite Integral Functions
Tom discusses the use of a graphing calculator, specifically the TI-84, to visualize definite integral functions. He demonstrates how to graph a function that is the antiderivative of a given integrand, using the fundamental theorem of calculus. Tom emphasizes the educational value of this process, as it allows students to see the graph of an antiderivative even when a closed-form expression is not available. He also shows how to adjust the graphing settings to speed up the plotting process and discusses the visual comparison between the derivative and the antiderivative graphs.
🌐 Final Thoughts and Future Plans
The video concludes with Curtis thanking Steve and Tom for their insights and expertise. He mentions that the show will continue in the spring, with plans to start in January and return to a biweekly schedule. Curtis encourages viewers to sign up for emails to stay informed about the show's return and expresses gratitude for the engagement during the fall season. He wishes everyone a good holiday season and looks forward to the next session of Monday Night Calculus.
Mindmap
Keywords
💡Fundamental Theorem of Calculus
💡Derivative
💡Integral Calculus
💡Antiderivative
💡Chain Rule
💡Definite Integral
💡Rate of Change
💡Net Change
💡Graphing Calculator
💡AP Calculus Exam
Highlights
Introduction to the fundamental theorem of calculus and its two parts, emphasizing its importance in AP calculus.
Explanation of how the fundamental theorem of calculus connects differential and integral calculus, providing a precise relationship between the derivative and integral.
Discussion on the ability to compute definite integrals easily and find antiderivatives of continuous functions using the fundamental theorem of calculus.
Illustration of the concept of the fundamental theorem of calculus Part 1 using a positive continuous function and its interpretation as an area under the curve.
Presentation of a formula for the area so far function and its derivation using graphical and analytical justification.
Formal statement of the fundamental theorem of calculus Part 1, highlighting the derivative of the definite integral with respect to its upper limit.
Common mistakes students make when applying the fundamental theorem of calculus, such as trying to find the definite integral first or not recognizing the application of the theorem.
Explanation of the fundamental theorem of calculus Part 2, which allows evaluating a definite integral by finding any antiderivative of the integrand and evaluating it at the interval endpoints.
Application of the fundamental theorem of calculus in the context of particle motion, relating velocity functions to position functions and the distance traveled.
Demonstration of using the fundamental theorem of calculus to find the derivative of functions defined by definite integrals, with examples involving different upper limits.
Challenge question involving the derivative of a function with both upper and lower bounds as functions of x, requiring the application of the fundamental theorem of calculus.
Discussion on the net change theorem, an alternate interpretation of the fundamental theorem of calculus, relating the definite integral of a rate of change to the net change in the original function.
Practical example of applying the fundamental theorem of calculus to a cell phone battery charging scenario, demonstrating the concept of net change.
Presentation of homework problems related to the fundamental theorem of calculus, showcasing various applications and challenges for students.
Solutions to the homework problems using the fundamental theorem of calculus, with detailed steps and explanations.
Discussion on the historical discovery of the fundamental theorem of calculus, the dispute between Newton and Leibniz, and the importance of publishing mathematical discoveries.
Demonstration of graphing definite integral functions using a TI-84 calculator, emphasizing the visual connection between the derivative and antiderivative.
Explanation of how to speed up graphing definite integral functions on a TI-84 calculator by adjusting the x resolution.
Illustration of the effect of changing the lower limit of integration on the graph of the antiderivative, showing a vertical shift in the graph.
Transcripts
Browse More Related Video
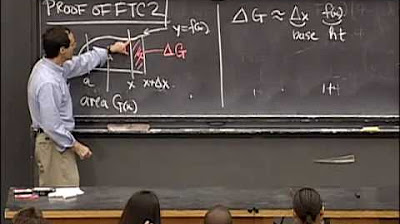
Lec 20 | MIT 18.01 Single Variable Calculus, Fall 2007

Fall 2023 MNC: Slice thin and add - Riemann sums and definite integrals
Fundamental Theorem of Calculus Part 1

Monday Night Calculus: Substitution
Fundamental Theorem of Calculus Parts 1&2

Fall 2023 MNC: Up close and personal with differentiability and local linearity
5.0 / 5 (0 votes)
Thanks for rating: