Center of Mass
TLDRIn this AP Physics essentials video, Mr. Andersen explores the concept of the center of mass, demonstrating its significance in understanding motion and balance. Through various examples, including a tire, a hammer, and a Saturn 5 rocket, he illustrates how the center of mass affects an object's movement and how it remains constant even when objects are separated. He introduces a simulation tool for visualizing the center of mass and explains the practical hang test method. The video concludes with a formula for calculating the center of mass in both simple and complex systems, emphasizing its importance in physics and problem-solving.
Takeaways
- 🎯 The center of mass is a crucial concept in physics, determining the point around which an object rotates and where it balances.
- 🔴 A regular motion of an object, like a tire, indicates that its center of mass is at the geometric center.
- 🛠️ When the center of mass is off-center, like in a hammer, the motion appears irregular and tricky to the eye.
- 🚀 For complex systems like the Saturn 5 rocket, the center of mass can be estimated and remains constant even when the system is broken apart, assuming no external forces.
- 📐 Newton's second law states that without a net external force, the center of mass maintains a constant velocity, either staying still or moving uniformly.
- 🔍 Identifying the center of mass can be done through simulations, like the center of mass builder from simbucket, which visually demonstrates the concept.
- 📊 The hang test is a simple experimental method to find the center of mass, where the object balances on a pivot point, revealing the center of mass location.
- 🧮 The center of mass position can be calculated using the formula: (mass1 * distance1 + mass2 * distance2) / (mass1 + mass2), and extended for more masses and dimensions.
- 🌟 Even in complex or chaotic motion, the center of mass moves with constant velocity, which can be confusing to observe but is consistent with the lack of external forces.
- 📈 In multi-dimensional problems, like 3D systems, the center of mass can be calculated by extending the formula to include z-axis components.
- 📚 As students progress to AP Physics 2, understanding and calculating the center of mass becomes an essential skill for solving more complex problems.
Q & A
What is the significance of the center of mass in the context of the tire and hammer examples?
-The center of mass is significant because it is the point around which an object rotates and determines the motion's uniformity. For the tire, the center of mass is in the middle, leading to regular motion. In contrast, the hammer's off-center center of mass results in trickier, non-uniform motion.
How does the center of mass affect the motion of the Saturn 5 rocket stages?
-The center of mass remains constant for the rocket stages unless an external force is applied. Even when the rocket breaks apart, the center of mass stays in the same location, maintaining constant velocity in the absence of net external force.
What is the formula to calculate the center of mass for a system with two masses?
-The formula to calculate the center of mass for two masses is Xcm = (mass1 * x1 + mass2 * x2) / (mass1 + mass2), where Xcm is the x-coordinate of the center of mass, mass1 and mass2 are the masses, and x1 and x2 are their respective distances from the reference point.
How can you experimentally determine the center of mass of an object?
-You can experimentally determine the center of mass by using the hang test. By hanging the object and letting it settle, the vertical line through the hanging point will pass through the center of mass.
What happens to the motion of an object when an external force is applied?
-When an external force is applied to an object, it results in acceleration, changing the object's velocity. However, without an external force, the object maintains constant velocity, either remaining still or moving uniformly.
How does the motion of the two ice blocks connected by a spring illustrate the concept of center of mass?
-The motion of the ice blocks connected by a spring illustrates that despite the complex, caterpillar-like motion of the blocks, the center of mass remains constant and moves with uniform velocity. This demonstrates that the apparent irregular motion does not affect the center of mass's constant velocity.
What would happen to the center of mass if the rocket were to break apart during flight?
-If the rocket were to break apart during flight, the center of mass would continue to follow the same parabolic path as if the rocket were intact, assuming no external forces are acting on the system.
How can the concept of center of mass simplify complex physics problems?
-The concept of center of mass simplifies complex physics problems by allowing us to focus on the overall motion of a system rather than the individual motions of its components. By understanding where the center of mass is, we can predict the system's behavior more easily.
What is the importance of understanding the center of mass in AP Physics 2?
-In AP Physics 2, understanding the center of mass is crucial for calculating and predicting the motion of complex systems. It helps in analyzing the translational and rotational dynamics of objects without getting overwhelmed by the intricacies of their individual parts.
How does the center of mass change when an object is also moving in the y-axis?
-When an object is moving in the y-axis, you would calculate the y-coordinate of the center of mass using a similar formula to the x-coordinate, but for the distances in the y-direction. This extends the calculation to two dimensions, allowing you to find the center of mass in both x and y.
What is the role of the center of mass in the motion of a system with multiple masses connected by springs?
-In a system with multiple masses connected by springs, the center of mass is the point that balances the system. Despite the complex motion of the individual masses, the center of mass moves with a constant velocity in the absence of external forces, providing a consistent reference point for analyzing the system's motion.
Outlines
🌪️ Center of Mass and Object Motion
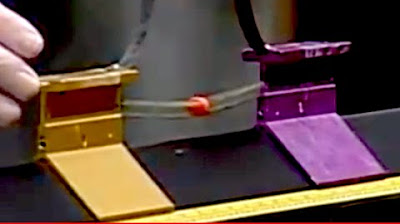
This paragraph introduces the concept of the center of mass and its impact on the motion of objects. Mr. Andersen uses a tire and a hammer to demonstrate how the regularity of motion is related to the position of the center of mass. He explains that the center of mass's location remains constant in the absence of external forces, even during rotational motion. The paragraph also touches on the application of Newton's second law in understanding constant velocity and the lack of acceleration when no net external force is applied. Furthermore, Mr. Andersen introduces a simulation tool, the center of mass builder from simbucket, to visually understand the center of mass and its calculation. The hang test method is also mentioned as a way to experimentally determine the center of mass.
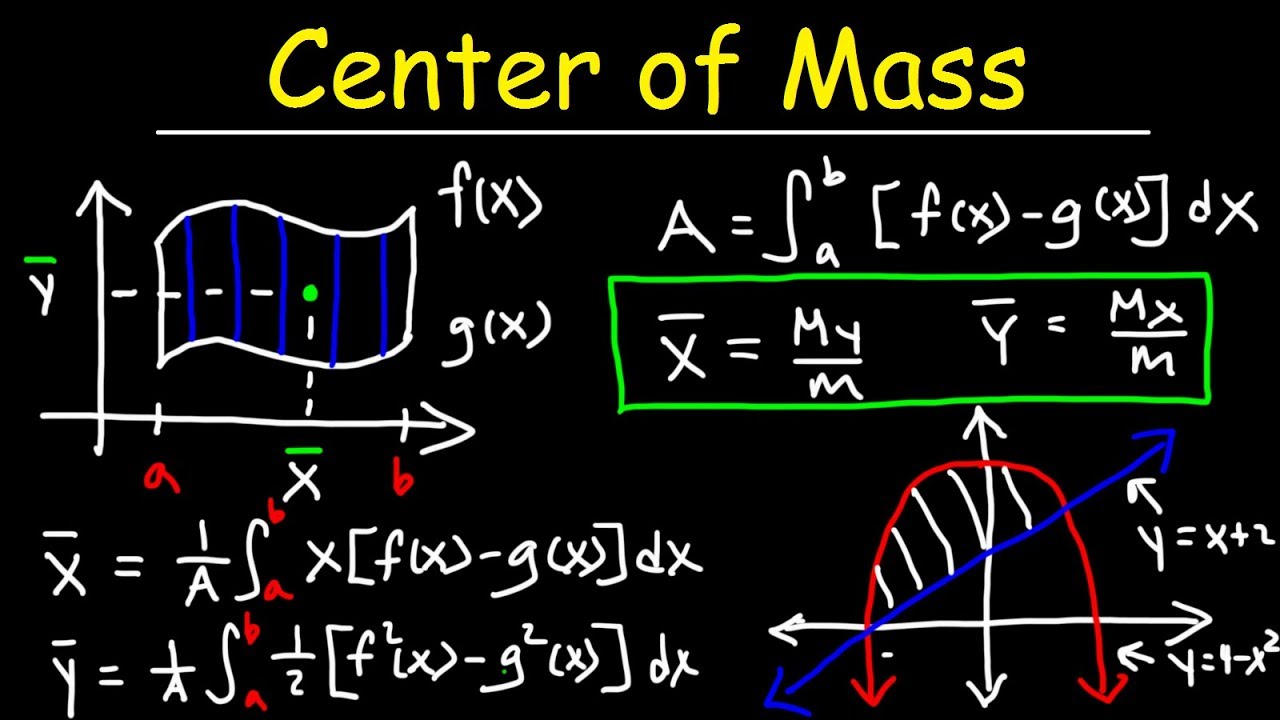
📐 Calculating the Center of Mass
This paragraph delves into the mathematical aspect of determining the center of mass for a system of objects. Mr. Andersen explains the formula for calculating the center of mass when objects are aligned along a straight line (1D). He provides a clear example using two masses connected by a bar and demonstrates how to apply the formula to find the x-coordinate of the center of mass. The concept is extended to include a third mass, showing how to incorporate additional masses into the calculation. The paragraph also briefly mentions the extension of these principles to two-dimensional (2D) and three-dimensional (3D) scenarios, which will be covered in more depth in AP Physics 2. The importance of understanding the center of mass for solving complex problems in physics is emphasized, highlighting its practical applications.
Mindmap
Keywords
💡Center of Mass
💡Uniform Motion
💡Newton's Second Law
💡Simulation
💡Hang Test
💡Constant Velocity
💡Acceleration
💡Parabolic Path
💡Calculation
💡Complex Systems
Highlights
The concept of center of mass and its importance in understanding the motion of objects is introduced.
A tire is used as an example to demonstrate how its center of mass being in the middle results in uniform rotation.
The motion of a hammer is shown to be less uniform due to its off-center center of mass.
The center of mass of a system remains constant even when the system is broken apart, as long as there is no external force.
The Saturn 5 rocket is used as an example to discuss the center of mass in complex systems.
A simulation tool called 'center of mass builder' is introduced for visualizing the center of mass.
The hang test is explained as a method to experimentally determine the center of mass.
The illusion of irregular motion in objects is discussed, emphasizing the constant velocity of the center of mass.
A video demonstration of two ice blocks connected by a spring is used to illustrate the concept of center of mass.
The caterpillar-like motion of the ice blocks on a spring is shown, with the center of mass remaining constant.
The importance of visualizing the center of mass in complex systems, such as a rocket flying and breaking apart, is highlighted.
The formula for calculating the center of mass for a system with two masses is provided.
An example calculation of the center of mass for two masses is worked out.
The concept is extended to systems with three or more masses and in multiple dimensions.
The practical application of center of mass in AP Physics 2 is mentioned, emphasizing the need to calculate it in both one and two dimensions.
The video concludes with a recap of the importance of understanding and calculating the center of mass for solving complex physics problems.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: