Linear Momentum
TLDRIn this enlightening AP Physics essentials video, Mr. Andersen demonstrates the concept of linear momentum through a series of experiments. By dropping a basketball and an apple simultaneously, he visually illustrates the transfer of momentum, which is mass times velocity. The video further explains how to calculate momentum using video analysis to determine an object's velocity and subsequently its momentum. The principle of conservation of momentum is also highlighted, showing how it applies during collisions between two spheres. The lesson aims to equip viewers with the knowledge to perform similar calculations and analyses.
Takeaways
- 🏀 Momentum is the product of an object's mass and its velocity.
- 🍏 When a basketball and an apple are dropped simultaneously, the apple gains significant velocity due to the momentum transfer from the more massive basketball.
- 📈 Momentum can be calculated using video analysis to track the position of an object's center of mass over time.
- 🤹♂️ The velocity of an object can be determined by the slope of its position-time graph, indicating constant velocity if the slope is linear.
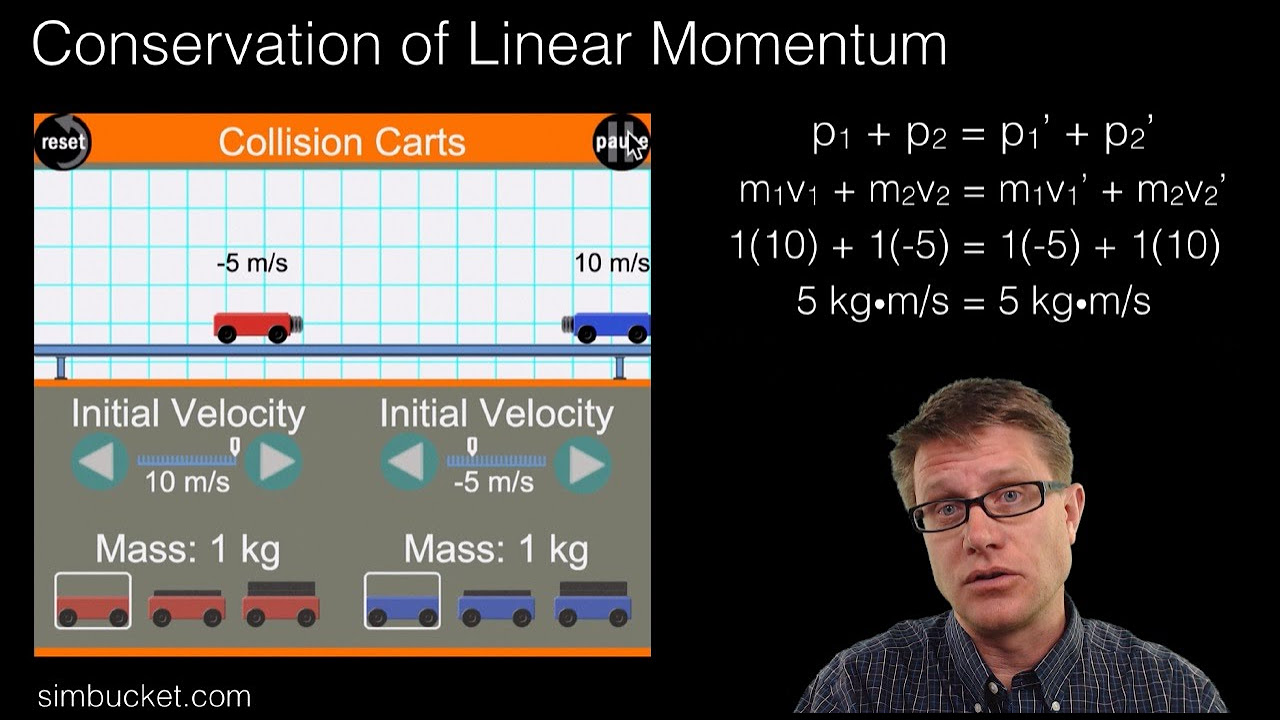
- 🎾 In a collision, momentum is conserved; the total momentum before the collision equals the total momentum after the collision.
- 🔵🟠 When two spheres collide, the change in momentum of one sphere is equal and opposite to the change in momentum of the other.
- 📊 The graph of an object's position over time can be used to calculate its velocity and consequently its momentum.
- 🥎 The momentum of a stationary object (before collision) is zero, as its velocity is zero.
- 🚀 After a collision, the velocities and hence the momenta of the objects involved change, but the total momentum remains constant.
- 🍏🏀 Momentum transfer can be observed and quantified by comparing the velocities of objects before and after an interaction, such as dropping an apple and a basketball.
Q & A
What is the main topic of the video?
-The main topic of the video is linear momentum, and it includes a demonstration involving a basketball and an apple.
How does the video demonstrate the concept of momentum?
-The video demonstrates momentum by dropping a basketball and an apple simultaneously and observing their velocities, highlighting that the basketball's greater mass transfers more momentum to the apple, causing the apple to move faster.
What is the formula for calculating momentum?
-The formula for calculating momentum is the product of an object's mass and its velocity, represented as momentum (p) = mass (m) × velocity (v).
How does the video use video analysis to calculate velocity?
-The video uses video analysis by observing the change in the position of an object's center of mass over time, graphing this data, and calculating the slope of the line to determine the constant velocity of the object.
What happens to the basketball and apple when they collide in the video?
-When the basketball and apple collide, some of the basketball's momentum is transferred to the apple, causing the apple to move faster, while the basketball's velocity decreases as it did not transfer all of its momentum.
How does the conservation of momentum principle apply in the collision between the two spheres in the video?
-The conservation of momentum principle states that the total momentum before the collision is equal to the total momentum after the collision. In the video, the orange sphere transfers some of its momentum to the green sphere upon collision, resulting in both spheres having a different, but algebraically sum-equivalent, total momentum post-collision.
What is the momentum of the green sphere before the collision?
-The momentum of the green sphere before the collision is 0 kilogram meters per second because it is not moving and its velocity is zero.
How is the velocity of the green sphere after the collision calculated?
-The velocity of the green sphere after the collision is calculated by determining the slope of the graph that represents the constant change in position over time post-collision, which is found to be approximately 1.5 meters per second.
What is the momentum of the orange sphere before and after the collision?
-Before the collision, the orange sphere's momentum is 10 kilogram meters per second (5 kg × 2 m/s). After the collision, it retains 2.5 kilogram meters per second (5 kg × 0.5 m/s), as some of its momentum is transferred to the green sphere.
How does the video demonstrate the transfer of momentum from the basketball to the apple?
-The video demonstrates the transfer of momentum from the basketball to the apple by showing the change in their velocities after the collision. The basketball, having more mass and thus more momentum, transfers some of its momentum to the apple, which has less mass and gains a higher velocity as a result.
What additional tool does the video suggest for calculating an object's momentum?
-The video suggests using video analysis as an additional tool for calculating an object's momentum by tracking the position of the object's center of mass over time and then calculating its velocity from the graphed data, which can then be used to determine the momentum.
Outlines
🏀 Demonstration of Linear Momentum and Momentum Calculation
This paragraph introduces the concept of linear momentum through a demonstration where a basketball and an apple are dropped simultaneously to illustrate the transfer of momentum. It explains that momentum (mass times velocity) is the reason behind the apple's increased speed due to the basketball's larger mass. The video script also covers how to calculate momentum using video analysis, which involves graphing the position of an object over time to determine its velocity and subsequently its momentum. The demonstration continues with an example of two spheres (5 kg each) colliding in a gravity-free environment, showing the transfer of momentum and how to calculate it before and after the collision using video analysis.
📊 Understanding Momentum Analysis and Data Calculation
The second paragraph emphasizes the importance of understanding how to calculate momentum by multiplying an object's mass with its velocity. It also highlights the application of video analysis in determining an object's velocity and consequently its momentum. The paragraph concludes by reinforcing the concept that the total momentum before a collision is equal to the total momentum after the collision, as demonstrated in the sphere collision example. The video aims to ensure that viewers have grasped the concept of calculating an object's momentum and analyzing data effectively.
Mindmap
Keywords
💡Linear Momentum
💡Mass
💡Velocity
💡Momentum Transfer
💡Video Analysis
💡Center of Mass
💡Collision
💡Conservation of Momentum
💡Graphing
💡Slope
Highlights
Introduction to the concept of linear momentum in physics.
Demonstration of dropping a basketball and an apple to illustrate momentum transfer.
Explanation that momentum is the product of an object's mass and velocity.
Observation that a more massive object (basketball) transfers momentum to a less massive object (apple), resulting in higher velocity for the apple.
Replay of the demonstration with a focus on the basketball's motion to show momentum transfer.
Discussion on how the direction of velocity and momentum are aligned for an object in motion.
Introduction to video analysis as a method for calculating an object's velocity and consequently its momentum.
Experiment with two 5-kilogram spheres to demonstrate momentum transfer during a collision, with gravity removed.
Explanation of how to calculate the momentum before and after a collision using video analysis and constant time intervals.
Illustration of the green sphere gaining momentum from the collision, showing a change from zero velocity to a constant velocity.
Calculation of the green sphere's momentum after the collision as 7.5 kilogram meters per second.
Analysis of the orange sphere's momentum before and after the collision, using video analysis to determine its velocity and momentum.
Explanation of the conservation of momentum principle, where the sum of momentum before collision equals the sum after collision.
Application of the principles learned to calculate the momentum transferred from the basketball to the apple in the initial demonstration.
Emphasis on the importance of understanding how to calculate an object's momentum by multiplying its mass and velocity.
Encouragement for viewers to apply the concepts learned to analyze data and calculate velocities and momenta in real-world scenarios.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: