Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (71 of 92) R=? T=? V0=(1/4)E (Ex. 1 of 4)

Michel van Biezen
30 Apr 201804:12
EducationalLearning
32 Likes 10 Comments

TLDRThis lecture explores the quantum behavior of particles encountering a potential step, where even a potential energy equal to a quarter of a particle's energy results in a surprisingly low reflection coefficient of 0.52%. The transmission coefficient is found to be 99.48%. The discussion delves into the mathematical derivation of these coefficients and hints at further analysis with different potential energies to understand how the percentages change.

Takeaways
  • 📚 The lecture discusses the quantum mechanical reflection and transmission of particles at a potential step.
  • 🌟 The potential step's energy is given as 1/4 the energy of the particle.
  • 🔢 Even at a 1/4 energy potential step, only a small percentage of particles will be reflected.
  • 📈 The calculation involves replacing the potential energy (V) with 1/4 of the particle's energy (E) in the equation.
  • 🧮 The mathematical process simplifies to finding the square root of E minus the square root of 3/4 E over the square root of E plus the square root of 3/4 E.
  • 🤔 The reflection coefficient is calculated to be 0.0052 or 0.52%, indicating a small fraction of particles will be reflected.
  • 📊 The transmission coefficient is the complement of the reflection coefficient, calculated as 1 minus the reflection coefficient.
  • 🌈 For the given potential step, 99.48% of the particles will be transmitted.
  • 🔧 The lecture suggests exploring how the percentages change with different potential step values (1/2, 3/4, etc.)
  • 💡 The example illustrates the counterintuitive nature of quantum mechanics where a significant potential step does not necessarily lead to a high reflection rate.
  • 📖 The process is demonstrated step-by-step, emphasizing the importance of mathematical analysis in understanding quantum phenomena.
Q & A

  • What is the potential step in the given example?

    -The potential step in the example is 1/4 the energy of the particle.

  • What was the initial assumption about the number of particles reflected and transmitted?

    -The initial assumption was that if the potential step is 1/4 the energy of the particle, a fair number of particles would be reflected and a greater number transmitted.

  • Why does the actual percentage of reflected particles differ from the initial assumption?

    -The actual percentage of reflected particles differs because the nature of the equation results in a very small percentage of particles being reflected, even when the potential step is 1/4 the energy of the particle.

  • How is the reflection coefficient calculated?

    -The reflection coefficient is calculated by squaring the difference between the square root of the particle's energy (E) and the square root of 3/4E, and then dividing by the square of the sum of the square root of E and the square root of 3/4E.

  • What is the numerical value of the reflection coefficient in the example?

    -The numerical value of the reflection coefficient in the example is 0.0052, or 0.52%.

  • What percentage of particles are transmitted when the potential step is 1/4 the energy of the particle?

    -When the potential step is 1/4 the energy of the particle, 99.48% of the particles are transmitted.

  • How does the potential step affect the reflection and transmission coefficients?

    -The potential step affects the reflection and transmission coefficients by determining the fraction of particles that will be reflected or transmitted. As the potential step increases, the reflection coefficient may increase and the transmission coefficient may decrease.

  • What happens to the reflection coefficient when the potential step is 1/2 the energy of the particle?

    -The script does not provide specific details for when the potential step is 1/2 the energy of the particle, but it implies that the percentage of reflected particles would change, likely increasing from the 0.52% when the potential step is 1/4 the energy.

  • What is the significance of the square root of E in the calculation?

    -The square root of E is significant as it represents the base energy level of the particle. It is used in the numerator and denominator of the reflection coefficient formula, indicating its fundamental role in determining the outcome.

  • How does the transmission coefficient relate to the reflection coefficient?

    -The transmission coefficient is calculated as 1 minus the reflection coefficient. This means that as the reflection coefficient increases, the transmission coefficient decreases, and vice versa.

  • What can we infer about the relationship between the potential step and the energy of the particle?

    -We can infer that even a relatively large potential step, such as 1/4 the energy of the particle, does not result in a high percentage of particles being reflected. This suggests that the energy of the particle has a more significant impact on the transmission and reflection outcomes than the size of the potential step alone.

Outlines
00:00
📚 Quantum Mechanics: Reflection and Transmission at a Potential Step

This paragraph introduces a lecture on quantum mechanics, focusing on the concept of particle reflection and transmission at a potential step. The discussion begins with an example where the potential step's energy is 1/4 of the particle's energy. It explains that despite the potential step being significant (1/4 of the particle's energy), the percentage of particles reflected is surprisingly small. The lecturer then walks through the mathematical derivation, starting with the substitution of V by 1/4 of the energy, leading to a complex equation. After simplification, the reflection coefficient is calculated to be 0.0052 or 0.52%, indicating that only a small fraction of particles will be reflected. The transmission coefficient is deduced as 99.48%, showing that the majority of particles will be transmitted. The paragraph concludes by hinting at further exploration of how the percentages change with different potential step values.

Mindmap
Keywords
💡potential step
The term 'potential step' refers to the energy difference or barrier that a particle encounters, which is a central concept in the discussion of particle transmission and reflection. In the video, the potential step is given as 1/4 the energy of the particle, which sets the stage for the calculation of reflection and transmission coefficients. This concept is crucial for understanding how particles interact with energy barriers, such as in quantum mechanics or wave dynamics.
💡reflection coefficient
The 'reflection coefficient' is a measure of the probability that a particle will be reflected off an energy barrier, such as a potential step. It is a key result derived from the mathematical equations presented in the video, indicating the fraction of particles that will not pass through the barrier. In the context of the video, the reflection coefficient is calculated to be 0.0052, meaning only 0.52% of the particles will be reflected, even when facing a potential step equal to a quarter of their energy.
💡transmission coefficient
The 'transmission coefficient' is the complementary concept to the reflection coefficient, representing the probability that a particle will pass through an energy barrier rather than being reflected. It is calculated as one minus the reflection coefficient and is used to understand how many particles will successfully traverse the potential step. In the video, when the potential step is 1/4 the energy of the particle, the transmission coefficient is found to be 0.9948, indicating that 99.48% of the particles will be transmitted.
💡energy of the particle
The 'energy of the particle' is a fundamental property that determines the behavior of a particle when it interacts with potential barriers. In the context of the video, the energy of the particle is used as a reference to define the potential step and is central to the calculations of reflection and transmission coefficients. It is the initial energy that the particle possesses before encountering the potential step.
💡quantum mechanics
While not explicitly mentioned in the script, 'quantum mechanics' is an underlying field of physics that deals with the behavior of particles at the atomic and subatomic level. The concepts of potential steps, reflection, and transmission coefficients are relevant in quantum mechanics when describing phenomena such as tunneling or particle interactions with potential energy surfaces. The calculations in the video are reminiscent of quantum mechanical problems involving wave functions and energy barriers.
💡particle
A 'particle' in the context of the video refers to an entity that possesses mass and energy, which is the subject of the transmission and reflection calculations. The behavior of particles when they encounter potential steps is the main focus of the lecture, with the particle's energy being a critical factor in determining the outcomes.
💡mathematical equations
The 'mathematical equations' mentioned in the script are the tools used to calculate the reflection and transmission coefficients. They are essential for understanding the quantitative aspects of how particles interact with potential steps. The equations involve square roots, fractions, and other mathematical operations that lead to the final results.
💡wave dynamics
Although not directly stated in the script, 'wave dynamics' is a relevant field in physics that studies the behavior of waves, including their interactions with obstacles and barriers. The concepts of reflection and transmission coefficients are also applicable in wave dynamics, where waves encounter changes in medium or obstacles, similar to particles encountering potential steps.
💡potential energy
In the context of the video, 'potential energy' refers to the stored energy that a system possesses due to the position of its particles relative to each other or to an external force. The potential step represents a form of potential energy barrier that particles must overcome. The script discusses how particles interact with this potential energy in terms of reflection and transmission.
💡calculation
The term 'calculation' refers to the process of performing mathematical operations to find the results or solutions to given problems. In the video, calculations are used to determine the reflection and transmission coefficients based on the energy of the particle and the potential step. These calculations are essential for understanding the quantitative outcomes of particle interactions with potential barriers.
💡interaction
The 'interaction' in the context of the video refers to the encounter between particles and a potential energy barrier, specifically the potential step. This interaction is crucial for determining the behavior of particles, whether they will be reflected, transmitted, or both. The interaction is the fundamental process that the mathematical equations and coefficients describe.
Highlights

The potential step is 1/4 the energy of the particle.

A fair number of particles would be reflected at this energy ratio.

The actual percentage of reflected particles is very small despite the potential step being 1/4 the energy.

The equation used in the example is fundamental to understanding particle behavior.

V is replaced by 1/4 the energy to calculate the reflection coefficient.

The reflection coefficient is calculated as the square root of (E - (1/4)E) over the square root of (E + (1/4)E), squared.

The square root of E can be factored out for simplification.

The reflection coefficient simplifies to (1 - (3/4)^0.5) / (1 + (3/4)^0.5)^2.

The final result for the reflection coefficient is 0.0052 or 0.52%.

The transmission coefficient is 1 minus the reflection coefficient, which equals 0.9948 or 99.48%.

Even with a potential step of 1/4 the energy, only slightly over 1/2 of 1% of particles are reflected.

The lecture discusses how the reflection and transmission percentages change with different potential step values.

The example demonstrates the impact of potential energy on particle transmission and reflection.

The mathematical process is detailed to show how the coefficients are derived and calculated.

The lecture provides a practical application of quantum mechanics principles.

The calculation shows that a higher potential step leads to a smaller reflection coefficient.

The lecture is an example of applying theoretical physics to real-world scenarios.

The results have implications for understanding particle behavior in various energy fields.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (72 of 92) R=? T=? V0=(1/4)E (Ex. 2 of 4)

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (67 of 92) Finding R=? T=? Coefficients

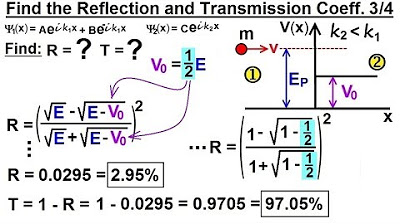
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (73 of 92) R=? T=? V0=(1/2)E (Ex. 3 of 4)

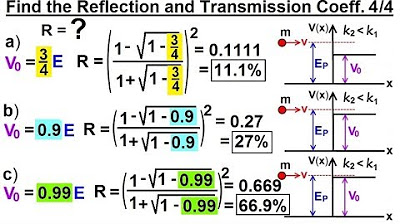
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (74 of 92) R=? T=? V0=(3/4)E,.(Ex. 4 of 4)

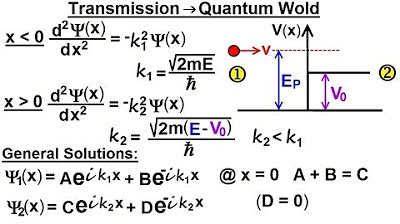
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (64 of 92) Transmission-Reflection (Q.M.)

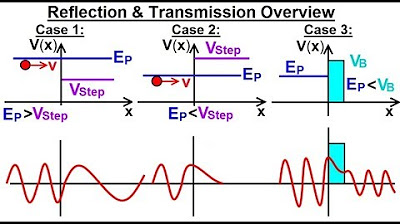
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (61 of 92) Reflection & Transmission

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Quantum MechanicsParticle PhysicsBarrier AnalysisReflection CoefficientTransmission CoefficientEnergy DynamicsScientific LectureEducational ContentMathematical ModelingPhysics ExperimentsPotential Energy