Particle in a Box Part 1: Solving the Schrödinger Equation
TLDRThis tutorial delves into solving the quantum problem of a particle in an infinite square well, also known as the particle in a box. By applying the Schrodinger equation and boundary conditions, the video explains how to determine the particle's allowed energy states and wavefunctions. The solution involves a mathematical exploration that leads to the quantization of energy levels, highlighting the wavefunction's form as a sine function and the normalization process to find the constant A. The result is a set of eigenfunctions and eigenenergies, providing insight into the quantized nature of quantum systems.
Takeaways
- 📝 The tutorial focuses on solving the quantum problem of a particle in an infinite square well, also known as the particle in a box model.
- 🌐 The potential V(x) is defined as infinite outside the well (x < 0 or x > a) and zero inside the well (0 ≤ x ≤ a).
- 🧪 The Schrodinger equation is applied to find the wavefunctions (ψ(x)) and eigenenergies for the particle within the well.
- 🚫 The boundary conditions require that the wavefunction ψ(x) equals zero at the boundaries (x = 0 and x = a).
- 📈 The time-independent Schrodinger equation is rearranged to simplify the process of finding solutions.
- 📊 The general solution to the differential equation is given by ψ(x) = A sin(kx) + B cos(kx), where A and B are constants to be determined.
- 🌟 The wavefunction ψ(x) is zero for all x outside the well, and non-zero inside the well, satisfying the boundary conditions.
- 🔄 The trigonometric functions' properties are used to determine that B must be zero, simplifying the wavefunction to ψ(x) = A sin(kx).
- 🔢 The wavefunction must be normalized, leading to the integral of ψ*ψ from 0 to a equaling one, which helps solve for the constant A.
- 💡 The quantization of energy levels is a result of the wavefunction satisfying the boundary conditions, with n being an integer.
- 🌈 The allowed energies (eigenenergies) for the particle are given by E_n = (hbar^2 * n^2 * π^2) / (2m * a^2), where n is an integer.
Q & A
What is the Schrodinger equation and why is it significant in quantum mechanics?
-The Schrodinger equation is a fundamental equation in quantum mechanics that describes how quantum systems evolve over time. It is significant because it provides a way to calculate the probability amplitude of a particle's state, which in turn allows us to predict the behavior of quantum systems.
What is the particle in an infinite square well model, and how does it simplify the quantum problem?
-The particle in an infinite square well model, also known as the particle in a box, is a theoretical model where a particle is confined to move within a one-dimensional box with infinite potential walls. This simplification allows for the analytical solution of the Schrodinger equation, making it a foundational problem in understanding quantum systems.
How does the potential function V(x) describe the environment of the particle in the box model?
-In the particle in a box model, the potential function V(x) is defined as infinity outside the interval from x=0 to x=a, and zero within this interval. This represents an impenetrable barrier outside the box and a region of zero potential within the box where the particle is confined to move.
What are the boundary conditions for the wavefunction of the particle in the box model?
-The boundary conditions for the wavefunction psi(x) in the particle in the box model are that psi(x) must equal zero at the boundaries (x=0 and x=a), indicating that the particle cannot be found outside the box, and that psi(x) is non-zero and determined by the Schrodinger equation within the box (0<x<a).
How does the time-independent form of the Schrodinger equation simplify the problem?
-The time-independent form of the Schrodinger equation simplifies the problem by allowing us to focus on the spatial part of the wavefunction. By rearranging the equation and isolating the second derivative, we can solve for the wavefunction psi(x) as a function of x, which describes the particle's state in the box.
What is the general solution to the time-independent Schrodinger equation for the particle in the box model?
-The general solution to the time-independent Schrodinger equation for the particle in the box model is psi(x) = A sin(kx) + B cos(kx), where A and B are constants to be determined by the boundary conditions, and k is defined as the square root of 2mE/hbar^2.
Why does the constant B have to be zero in the solution for the particle in the box model?
-The constant B has to be zero because, at the boundary x=0, the cosine term would otherwise not be zero, violating the boundary condition that requires the wavefunction psi(x) to be zero at the boundaries.
How does the wavefunction satisfy the boundary condition at x=a?
-The wavefunction satisfies the boundary condition at x=a by having sine(ka) equal to zero, which occurs when ka equals nπ, where n is an integer. This means that k equals nπ/a, imposing quantization on the system as n must be an integer.
What is the physical interpretation of the quantization in the particle in the box model?
-The quantization in the particle in the box model means that the energy levels and wavefunctions of the particle are discrete and can only take on specific values. This is a direct consequence of the boundary conditions and the nature of the sine function, which can only be zero at integer multiples of π.
How is the normalization constant A determined in the particle in the box model?
-The normalization constant A is determined by requiring that the wavefunction psi(x) is normalized from 0 to a, meaning that the integral of psi*psi dx from 0 to a equals one. This leads to the solution A = sqrt(2/a).
What is the expression for the eigenfunctions and eigenergies of the particle in the box model?
-The eigenfunctions psi_n are given by psi_n(x) = (sqrt(2/a)) sin(nπx/a), and the associated eigenergies E_n are given by E_n = (hbar^2 n^2 π^2)/(2m a^2), where n is an integer representing the quantum number.
Outlines
🌟 Introduction to Solving the Quantum Problem of a Particle in a Box
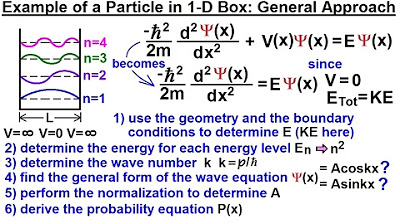
This paragraph introduces the task of solving the quantum problem of a particle confined in an infinite square well, also known as the particle in a box. It explains the concept of the potential and how it affects the particle's presence within the well. The goal is to find the eigenenergies and eigenfunctions of the particle using the Schrodinger equation, which will reveal the allowed states and probability density function of the particle within the confines of the well. The paragraph sets the stage for applying the previously learned forms of the Schrodinger equation to a practical quantum problem.
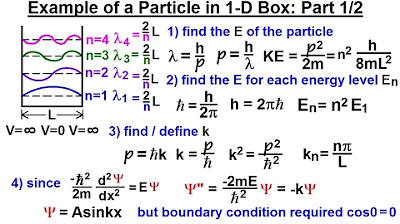
📚 Derivation and Solution of the Time-Independent Schrodinger Equation
The paragraph delves into the process of solving the time-independent Schrodinger equation for the particle in a box. It discusses the general solution involving sine and cosine functions and the boundary conditions that must be met for the wavefunction to be valid. The explanation includes the mathematical manipulation of the Schrodinger equation, the introduction of the constant k, and the derivation of the general solution psi of x as A sine(kx) + B cosine(kx). It also highlights the importance of the wavefunction being zero at the boundaries and the implications of this condition on the constants A and B.
📈 Graphical Interpretation and Quantization of the Wavefunction
This section provides a graphical interpretation of the wavefunction and its behavior at the boundaries. It explains how the sine and cosine functions contribute to the wavefunction and why the constant B must be zero. The paragraph also discusses the quantization of the system, as the wavefunction can only have non-zero values for integer values of n, which corresponds to the allowed states of the particle. The graphical representation of the wavefunction for different integer values of n is used to illustrate the quantized nature of the particle's energy levels.
🔍 Normalization of the Wavefunction and Calculation of Eigenenergies
The final paragraph focuses on the normalization of the wavefunction to determine the constant A and the calculation of the eigenenergies for each allowed state. It explains the normalization process, the integral involved, and the use of trigonometric identities to simplify the calculation. The paragraph concludes with the expression for the eigenenergies as a function of the integer n, providing a complete solution to the quantum problem of a particle in an infinite square well, including the mathematical representation of the wavefunctions and their associated energies.
Mindmap
Keywords
💡Schrodinger Equation
💡Eigenenergies
💡Eigenfunctions
💡Infinite Square Well
💡Boundary Conditions
💡Quantization
💡Wavefunction
💡Probability Density Function
💡Potential Energy
💡Normalization
Highlights
The tutorial focuses on solving the quantum problem of a particle in an infinite square well, also known as the particle in a box.
The potential V(x) is defined as infinity everywhere except between x=0 and x=a, where it equals zero.
The particle is confined to the square well, and the Schrodinger equation is used to determine its behavior within this potential.
The wavefunctions psi(x) represent the particle and must satisfy the Schrodinger equation to find the allowed states and energies.
Boundary conditions dictate that psi(x) must be zero where the potential is infinity, hence at x=0 and x=a.
The time-independent form of the Schrodinger equation is used to simplify the problem and find the solution.
The general solution is given by psi(x) = A sine(kx) + B cosine(kx), where A and B are constants to be determined.
The wavefunction must be zero at the boundaries, leading to the conclusion that B must be zero and the wavefunction simplifies to psi(x) = A sine(kx).
The quantization condition arises from the requirement that sine(kx) must be zero at x=a, leading to k = nπ/a where n is an integer.
Each integer value of n provides a different eigenfunction and eigenenergy, representing the allowed states for the particle.
The wavefunction is normalized from 0 to a, leading to the determination of the constant A as √(2/a).
The eigenfunctions are given by psi_n = √(2/a) sine(k_n x) where k_n = nπ/a, and each describes a different allowed state of the particle.
The eigenenergies are calculated as E_n = ħ²k_n²/2m, which depend on the integer value of n, indicating quantized energy levels.
The tutorial successfully solves the first quantum problem, determining the allowed energies of a particle in an infinite square well under given boundary conditions.
The implications of the solution are significant and require further discussion, indicating the depth and complexity of quantum mechanics.
The problem of the particle in a box serves as a fundamental example to understand the principles of quantum confinement and quantization.
The tutorial provides a comprehensive walkthrough of the mathematical process involved in solving the Schrodinger equation for a specific quantum system.
Transcripts
Browse More Related Video
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.

22. Quantum mechanics IV: Measurement theory, states of definite energy

23. Quantum Mechanics V: Particle in a Box
What is The Schrödinger Equation, Exactly?
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (19 of 92) Particle in 1-D Box: Example 1/2

Lecture 6: Time Evolution and the Schrödinger Equation
5.0 / 5 (0 votes)
Thanks for rating: