What is The Schrödinger Equation, Exactly?

Up and Atom
6 Jul 201809:28
EducationalLearning
32 Likes 10 Comments

TLDRThe Schrodinger equation, often encountered in quantum science, is the quantum world's equivalent of Newton's F=ma, revealing everything about a quantum system. It involves the wave function, which predicts the probability of a particle's location, and quantized energy levels, reflecting the particle's allowed energy states. The equation's solutions provide insights into a quantum system's potential and kinetic energy, offering a comprehensive understanding of quantum behavior.

Takeaways
  • 🌟 The Schrödinger equation is a fundamental equation in quantum mechanics, akin to F=ma in classical physics, providing comprehensive information about a quantum system.
  • 📚 It is a complex topic often mentioned in quantum science articles but not always explained in detail.
  • 🧬 The equation involves the Greek letter psi (ψ), representing the wave function which describes the probability distribution of a quantum object's location.
  • 🚫 Quantum objects, unlike classical objects, cannot have their exact position and momentum known simultaneously due to the Heisenberg uncertainty principle.
  • 📍 The wave function is a key concept, illustrating the probabilities of where a quantum object might be, rather than an exact location.
  • 🌊 The wave function represents a superposition of all possible states of a quantum object until it is measured, at which point the wave function collapses.
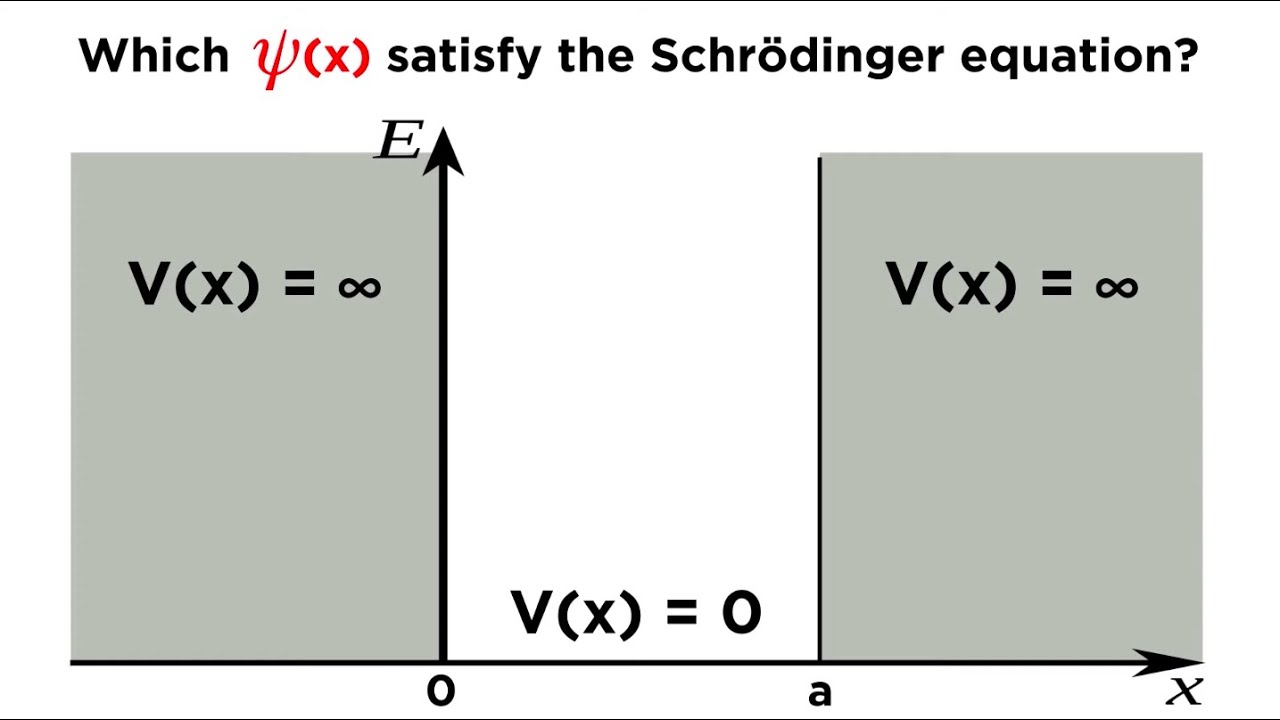
  • ⚡️ Energy in quantum systems is quantized, meaning electrons can only occupy certain energy levels, which is a result of the wave-like nature of probability distributions.
  • 🔧 The Schrödinger equation helps determine both the energy levels and the wave functions, which are crucial for understanding the behavior of quantum particles.
  • 🛠️ Solving the Schrödinger equation requires advanced mathematics and a strong intuitive understanding of quantum concepts.
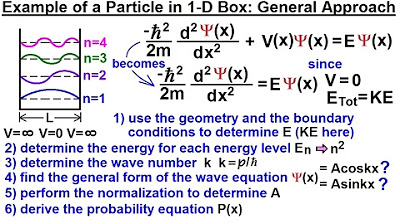
  • 📈 The solutions to the Schrödinger equation for a particle in a box scenario result in quantized energy levels and characteristic wave functions for each state.
  • 💻 Further study and practice with quantum mechanics problems can enhance understanding of the Schrödinger equation and its implications.
Q & A

  • What is the significance of the Schrodinger equation in quantum science?

    -The Schrodinger equation is fundamental in quantum science as it provides a way to calculate the probable behavior of quantum systems, essentially telling us everything we can know about a quantum system. It's akin to Newton's F=ma in classical physics, but for the quantum realm.

  • Why doesn't Newton's F=ma work for quantum objects?

    -Newton's F=ma doesn't apply to quantum objects because of the Heisenberg uncertainty principle, which states that we cannot know both the exact position and momentum of quantum objects simultaneously. Quantum objects exhibit probabilistic behavior that cannot be fully described by classical mechanics.

  • What is the wave function in the context of the Schrodinger equation?

    -The wave function, represented by the Greek letter psi (ψ), is a mathematical function that describes the probability distribution of a quantum object's location. It tells us where the object is likely to be, but not exactly where it will be, as the act of measurement collapses the wave function, localizing the quantum object.

  • How does the Heisenberg uncertainty principle relate to the Schrodinger equation?

    -The Heisenberg uncertainty principle is a fundamental concept in quantum mechanics that the Schrodinger equation accounts for. It implies that certain pairs of physical properties, like position and momentum, cannot be simultaneously measured with arbitrary precision. The Schrodinger equation incorporates this principle by dealing with wave functions that represent probabilities rather than definite positions or momenta.

  • What does the energy term (E) in the Schrodinger equation represent?

    -In the Schrodinger equation, the energy term (E) represents the allowed energy levels that a quantum object, like an electron, can have. These energy levels are quantized, meaning the electron can only occupy certain discrete energy states, which is a key aspect of quantum mechanics.

  • What is the relationship between energy and frequency in quantum mechanics?

    -In quantum mechanics, energy (E) is proportional to frequency (f), as described by the equation E=hf, where h is Planck's constant. This relationship indicates that the energy associated with a quantum object can only take on certain discrete values that correspond to specific frequencies of its wave function.

  • How does the potential energy (V) affect the solutions of the Schrodinger equation?

    -The potential energy (V) in the Schrodinger equation influences the allowed energy levels and the shape of the wave functions. It is a factor that helps determine the quantized energy levels within a quantum system and affects the probability distribution of where a quantum object can be found.

  • What are the typical solutions to the Schrodinger equation for an electron in a box?

    -The typical solutions to the Schrodinger equation for an electron in a box are sine wave-like functions for the wave function, and the energy equation is quantized, meaning the electron can only have certain discrete energy levels. These solutions are mathematical expressions that describe the quantized energy states and probability distributions of the electron within the box.

  • How does the act of measurement affect the wave function?

    -The act of measurement causes the wave function to collapse. Prior to measurement, the quantum object exists in a superposition of all possible states. However, when a measurement is made, the wave function collapses to a specific state, localizing the object at a particular point in space with a definite energy.

  • What is the significance of the quantization of energy levels in quantum mechanics?

    -The quantization of energy levels is a key feature of quantum mechanics that distinguishes it from classical physics. It means that quantum objects, such as electrons, can only occupy certain discrete energy states within a system. This quantization arises from the wave-like nature of quantum objects and their probability distributions, leading to the concept of quantized values in the quantum realm.

  • How can one gain a deeper understanding of the Schrodinger equation and quantum mechanics?

    -To gain a deeper understanding of the Schrodinger equation and quantum mechanics, one can study courses dedicated to these topics, such as those offered on Brilliant.org, which start with foundational concepts and lead up to the derivation of key equations. Working through problems and building a strong intuition is also crucial for understanding the complex nature of quantum systems.

Outlines
00:00
🌟 Introduction to the Schrodinger Equation

This paragraph introduces the Schrodinger equation as a fundamental concept in quantum science, often encountered in articles and journals but seldom explained in detail. Jade, the speaker, aims to clarify what the equation means, comparing it to the F=ma equation in classical physics. The Schrodinger equation is described as a tool that reveals everything knowable about a quantum system, including its position, momentum, velocity, and energy. The paragraph also touches on the Heisenberg uncertainty principle and the transition from classical to quantum mechanics, highlighting the limitations of Newton's equations at the quantum level.

05:02
📚 Quantum Systems and Energy Levels

The second paragraph delves into the specifics of quantum systems, using the example of an electron in a box to illustrate the concepts. It explains the role of the Greek letter psi (ψ) as the wave function, which provides probabilities of an electron's location rather than an exact position. The paragraph discusses the superposition of states and the famous thought experiment of Schrödinger's cat to illustrate this concept. It then moves on to explain the allowed energy levels (E) within the box, introducing the quantization of energy levels in quantum mechanics. The distinction between kinetic and potential energy in quantum systems is also clarified, along with the mathematical representation of these energies in the Schrodinger equation.

Mindmap
Keywords
💡Schrodinger Equation
The Schrodinger Equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. It is considered the 'F=ma' of the quantum world, as it allows us to predict the behavior of quantum objects. In the video, it is explained as containing all the information about a quantum system, such as the probabilities of where an electron might be found in a box.
💡Quantum System
A quantum system refers to a physical system that is described by the laws of quantum mechanics. These systems can be particles like electrons, photons, or larger entities behaving quantumly under certain conditions. The video focuses on an electron in a box as an example of a quantum system, aiming to explain its behavior using the Schrodinger Equation.
💡Wave Function
The wave function, represented by the Greek letter psi (ψ), is a mathematical description of the quantum state of a system. It provides information about the probabilities of finding a particle in different locations. The wave function is central to the interpretation of quantum mechanics and is used to calculate the probabilities of various outcomes when a measurement is made.
💡Heisenberg Uncertainty Principle
The Heisenberg Uncertainty Principle is a fundamental concept in quantum mechanics that states you cannot simultaneously know the exact position and momentum of a quantum object. The more precisely one property is measured, the less precisely the other can be known. This principle challenges the classical view of particles and introduces the probabilistic nature of quantum mechanics.
💡Energy Levels
Energy levels refer to the quantized, or discrete, amounts of energy that a quantum system can have. In quantum mechanics, particles do not have continuous energy values but can only occupy certain allowed energy states. This quantization is a key feature of the quantum world and is directly related to the wave-like nature of particles.
💡Superposition
Superposition is a principle in quantum mechanics where a quantum system exists simultaneously in all of its possible states until it is measured. At the moment of measurement, the system 'collapses' into one of the possible states. This concept is central to understanding the behavior of quantum objects and their wave-particle duality.
💡Probability Distribution
Probability distribution is a statistical concept that describes the likelihood of different outcomes in an experiment. In quantum mechanics, it is used to describe the likelihood of finding a particle in various locations, as given by the wave function. The probability distribution is spread out over space, much like a wave, hence the term 'wave function'.
💡Kinetic Energy
Kinetic energy is the energy of motion possessed by an object. In classical mechanics, it is given by the equation KE = 1/2 mv^2, where m is the mass and v is the velocity of the object. In quantum mechanics, the concept of kinetic energy is more complex and is related to the wave-like properties of particles.
💡Potential Energy
Potential energy is the stored energy of an object based on its position in a force field, such as a gravitational or electric field. It has the potential to be converted into kinetic energy. In quantum mechanics, potential energy is related to the forces acting on a particle and is a key component of the total energy in a quantum system.
💡Quantum Mechanics
Quantum mechanics is a fundamental theory in physics that describes the behavior of matter and energy at the atomic and subatomic scales. It introduces concepts like wave-particle duality, superposition, and quantization, which are essential for understanding the microscopic world.
💡Brilliant.org
Brilliant.org is an online learning platform that offers courses in various subjects, including physics, math, and computer science. The platform provides interactive lessons and problems for learners to practice at their own pace, with a focus on developing a strong intuition for complex concepts.
Highlights

The Schrodinger equation is a fundamental equation in quantum science that reveals everything we can know about a quantum system.

It is often referred to as the 'F=ma' of the quantum world, providing a framework to predict properties like position and momentum.

Unlike Newton's equations, the Schrodinger equation is necessary for understanding particle behavior at the quantum level.

The Heisenberg uncertainty principle states that we cannot know both the exact position and momentum of quantum objects simultaneously.

The wave function, represented by the Greek letter psi (ψ), is central to the Schrodinger equation and describes the probability distribution of a quantum object.

Quantum objects exist in a superposition of all possible states until measured, at which point the wave function collapses to a single state.

The Schrodinger equation allows us to solve for the energy levels and wave functions, which in turn lets us derive all possible knowledge about a particle.

Energy levels in quantum systems are quantized, meaning particles can only have certain discrete energy values, unlike the continuous range in classical physics.

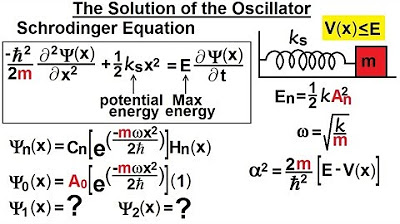
The equation's solutions involve mathematical forms that represent the allowed wave functions and energy states of the quantum system.

For an electron in a box, the wave function takes specific forms, and the probability distribution changes with different energy states.

The Schrodinger equation is time-independent in this discussion, focusing on the system's stationary states.

The potential and kinetic energy components of the total energy are crucial in solving the Schrodinger equation.

The solutions to the Schrodinger equation involve constants and whole numbers, reflecting the quantized nature of quantum states.

Understanding the Schrodinger equation requires building a strong intuition, often through problem-solving and engagement with quantum concepts.

Resources like Brilliant.org offer courses on quantum mechanics, providing a structured way to learn and understand the Schrodinger equation and related concepts.

The speaker suggests that engaging with the material and seeking feedback can improve one's understanding of complex quantum physics topics.

Transcripts

Browse More Related Video

Particle in a Box Part 1: Solving the Schrödinger Equation

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.

23. Quantum Mechanics V: Particle in a Box

Lecture 6: Time Evolution and the Schrödinger Equation

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (55 of 92) Solution of the Oscillator

22. Quantum mechanics IV: Measurement theory, states of definite energy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsSchrodingerEquationWaveFunctionQuantizedEnergyHeisenbergUncertaintyPhysicsExplainedEducationalContentJadeTutorialsQuantumBehaviorMathematics