AP Calculus BC Lesson 10.15

Elizabeth Fein

10 Apr 202325:21

EducationalLearning

32 Likes 10 Comments

TLDRThis video script covers advanced concepts in AP Calculus BC, focusing on differentiating and integrating series. The lesson begins with writing the first three terms and the general term for a given series, then moves on to finding the derivative of the series. It continues with integrating the series to find the antiderivative and concludes with applying Taylor series and Maclaurin series to functions, including finding the general term and working with initial conditions. The script provides detailed examples and explanations, making complex calculus topics accessible.

Takeaways

📝 The lesson focuses on differentiating and integrating series within the context of AP Calculus BC.
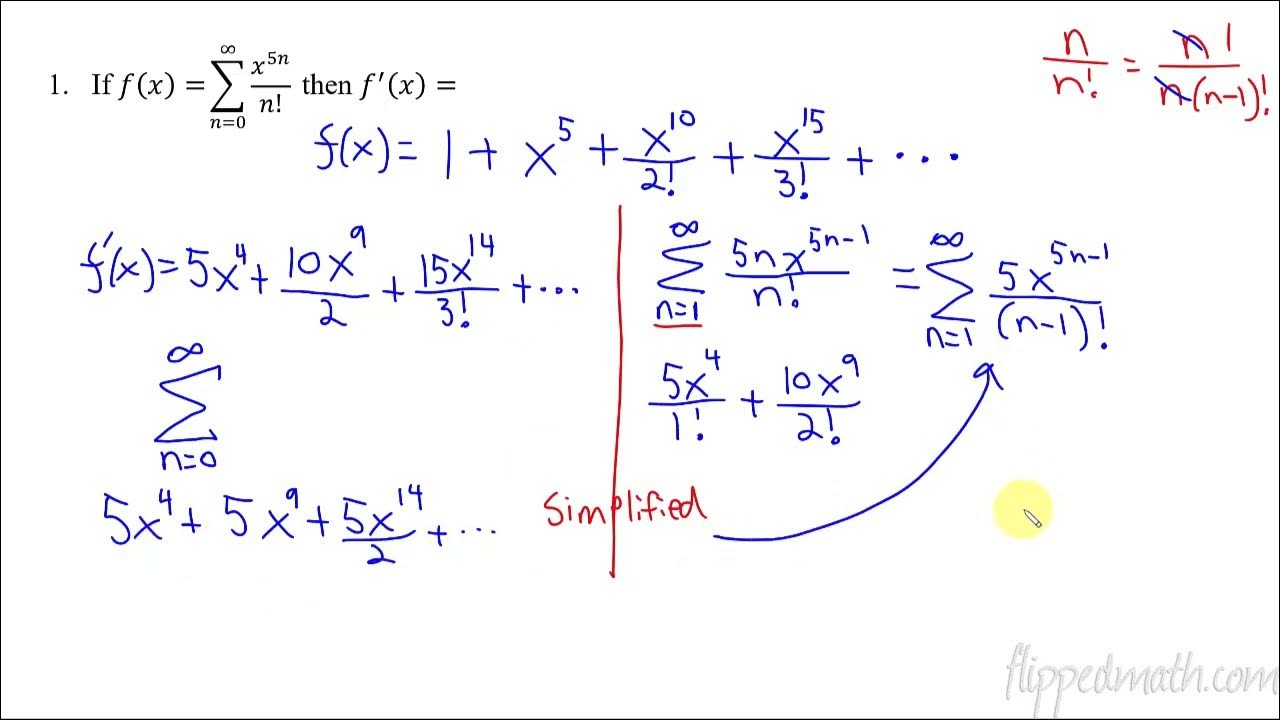
🌟 The function f(x) is represented by a series from n=1 to infinity of (2x^n)/n^3.
🔍 Part A involves writing the first three terms and the general term of the series for f(x).
📈 For Part B, the task is to find the first three terms and the general term for the derivative of f(x), f'(x).
🧠 Understanding the general term of a series is crucial for simplifying and differentiating series.
🤔 Part C requires integrating the function f(t) from 0 to x to find the series for G(x).
🌐 The script also discusses the Taylor series for functions f(x) and f'(x) about x=0.
📚 The Maclaurin series for sine of x is provided as a basis for finding the series for f'(x) when f(x) = sin(x^3).
🔧 The process of converting a function into a geometric series is demonstrated using the function F(x) with given initial conditions.
🧮 The script concludes with a free response question that involves finding the Maclaurin series for a function based on given derivative values and conditions.
📈 The general term formula for the Maclaurin series is derived by analyzing the pattern of the series and applying the rules for derivatives and integrals.

Q & A

What is the function f(x) represented by in the series form in the transcript?
-The function f(x) is represented by the series from n equals 1 to Infinity of (2x to the power of n) / (n cubed).
What are the first three terms of the series for f(x)?
-The first three terms of the series for f(x) are 2x, 8 (which is 2x squared over 2 cubed), and 27 (which is 2x cubed over 3 cubed).
What is the general term of the series for f(x)?
-The general term of the series for f(x) is 2x to the power of n / n cubed.
How is the derivative of the series for f(x), denoted as f'(x), calculated?
-The derivative of the series for f'(x) is calculated by taking the derivative of each term, including the general term. For example, the derivative of 2x is 2, the derivative of x squared is 0.5x, and the derivative of x cubed is 3x squared, and so on.
What is the general term for the series of f'(x)?
-The general term for the series of f'(x) is 2 times x to the power of n minus 1 over n squared.
How is the integral of the function f(x) from 0 to x, denoted as G(x), approached?
-G(x) is approached by integrating each term of the original series for f(x) with respect to t from 0 to x. The bounds of integration are applied by plugging in x and subtracting the result of plugging in 0.
What is the first three terms and the general term of the series for G(x)?
-The first three terms for G(x) are x squared, x cubed / 12, and 2x to the fourth / 27 times 4. The general term is 2 times x to the power of n plus 1 over n cubed times (n plus 1).
What is the Taylor series for f'(x) about x equals zero?
-The Taylor series for f'(x) about x equals zero is 3x squared, minus 9x to the power of 8 / 3!, plus 15x to the power of 14 / 5!, and so on.
What is the Maclaurin series for the function f(x) given its derivative f'(x) and initial condition?
-Given f'(x) = 1 / (3 - x) with f(0) = 0, the Maclaurin series for f(x) is x / 3, plus x squared / 18, plus x cubed / 81, plus x to the fourth / 324, and so on.
How is the Maclaurin series for f(x) derived from the given information?
-The Maclaurin series for f(x) is derived by integrating the Maclaurin series for f'(x), which is obtained by transforming the given function f'(x) into a geometric series and then integrating each term with respect to x.
What is the general rule for developing a Maclaurin series?
-The general rule for developing a Maclaurin series is f(x) = f(0) plus f'(0) times x plus f''(0) times x squared / 2! plus f'''(0) times x cubed / 3! and so on, where f(n)(0) represents the nth derivative of f evaluated at x equals zero.
How does the Maclaurin series for f(x) converge?
-The Maclaurin series for f(x) converges to f(x) for absolute values of x less than one, given that the derivatives of all orders exist for negative one less than x less than one.

Outlines

00:00

📚 Introduction to Differentiating and Integrating Series

This paragraph introduces the topic of differentiating and integrating series within the AP Calculus BC course. It presents an example of a function f(x) represented by an infinite series and explains how to find the first three terms and the general term of the series. The process of simplifying the series and finding the derivative (f'(x)) of the function is also detailed, including the method for differentiating each term and the general term. The paragraph concludes with the series for f'(x) after simplification.

05:00

📈 Deriving the Series for f'(x)

The focus of this paragraph is on deriving the series for f'(x) by taking the derivative of each term in the series for f(x). It explains the process of differentiating the general term and simplifies the series for f'(x). The paragraph then transitions to integrating, with the introduction of G(x) as the integral of f(t) from 0 to x, and outlines the steps to find the first three terms and the general term of G(x). The integration process is detailed, including the antiderivative of each term and the handling of bounds.

10:02

🌟 Taylor Series for f'(x) and Maclaurin Series

This paragraph delves into the Taylor series for f'(x) and the Maclaurin series. It starts by finding the Taylor series for f'(x) about x equals zero using the sine function and its derivatives. The process of finding the Maclaurin series for f(x) from the given f'(x) is explained, including the integration of the series. The paragraph also addresses a multiple-choice question regarding the Maclaurin series for f(x) given certain initial conditions and the derivative f'(x).

15:03

🧮 Constructing the Maclaurin Series for a Rational Function

The paragraph discusses the construction of the Maclaurin series for a rational function, given the function f(x) and its derivative f'(x). It explains the process of transforming the function into a geometric series format and finding the series for f'(x). The integral of f'(x) is then taken to find the Maclaurin series for f(x), and the constant term is determined using the initial condition that f(0) equals zero. The final Maclaurin series for f(x) is presented, matching a given answer choice.

20:05

🔄 Integrating the Maclaurin Series

This paragraph involves integrating the Maclaurin series of a function f(x) to find another function G(x). The process starts with the integral of f(t) from 0 to x, and the general term for G(x) is derived by integrating each term of f(t). The paragraph then explains how to evaluate the antiderivative at the bounds and simplify the series for G(x). The explanation includes the steps for integrating the general term and handling the bounds for the integral.

25:08

🎓 Additional Practice with Series FRQs

The final paragraph briefly mentions the intention to create a separate video for additional practice on Free Response Questions (FRQs) related to series, including Taylor series and Maclaurin series. It suggests that more content from chapter 10 will be covered in the upcoming video to provide further practice for exam-style questions.

Mindmap

Keywords

💡Differentiation

Differentiation is a fundamental operation in calculus that involves finding the derivative of a function. In the context of this video, it refers to the process of finding the derivative of a series represented by a function, which is a key step in analyzing the function's behavior and properties. For example, the video discusses finding the derivative of the series ∑(2x^n/n^3) and simplifies it to a more manageable form for further analysis.

💡Integration

Integration is the reverse process of differentiation and is used to find the antiderivative or the integral of a function. In the video, integration is used to find the function G(x) which is the integral of the function f(t) from 0 to x. This process is essential for understanding the accumulated effect of the function's behavior over an interval.

💡Series

A series in mathematics is the sum of the terms of a sequence, often used to represent functions. In this video, series are central to the discussion as they are used to define functions and to analyze their derivatives and integrals. The video focuses on power series and their applications in calculus, particularly in the context of Taylor and Maclaurin series.

💡Taylor Series

A Taylor series is a representation of a function as an infinite sum of terms calculated from the values of its derivatives at a single point. It is a powerful tool for approximating functions and is widely used in mathematical analysis. The video discusses Taylor series in the context of finding the series representation for the derivative of a given function.

💡Maclaurin Series

A Maclaurin series is a special case of a Taylor series where the expansion point is zero. It is used to approximate functions around the origin and is particularly useful for functions that are easier to differentiate and integrate when expanded in this way. The video uses Maclaurin series to find the antiderivative of a function and to determine its series representation.

💡Power Rule

The power rule is a fundamental rule in calculus that states the derivative of x^n, where n is any real number, is n*x^(n-1). This rule is essential for differentiating functions that involve monomials and is used extensively in the video when finding the derivative of the series terms.

💡Antidifferentiation

Antidifferentiation, also known as integration, is the process of finding a function whose derivative is a given function. It is the inverse operation to differentiation and is crucial for finding the original function from its derivative. The video discusses antidifferentiation in the context of finding the integral of a series to determine the function G(x).

💡Bounds of Integration

The bounds of integration specify the limits between which the integration is performed. These bounds are crucial in determining the definite integral of a function and in finding the accumulated effect of the function over a specific interval. In the video, the bounds are used to evaluate the integral of the series from 0 to x.

💡General Term

The general term of a series is a formula that expresses the nth term of the series in terms of n. It is a way to describe the pattern of the series without having to write out each individual term. The general term is essential for understanding the structure of the series and for performing operations like differentiation and integration.

💡Simplifying

Simplifying in mathematics refers to the process of making a mathematical expression or equation easier to understand or work with. In the context of this video, simplifying is used to reduce complex series expressions into more manageable forms, which facilitates further calculations like differentiation and integration.

Highlights

Introduction to differentiating and integrating series in AP Calculus BC course.

Explanation of writing the first three terms and the general term of a series.

Derivation of the series for f'(x) from the given function f(x).

Integration of the series to find the function G(x).

Discussion on the Taylor series for f(x) and f'(x) about x=0.

Procedure for finding the Maclaurin series for a function given its derivatives.

Illustration of converting a rational function into a geometric series.

Derivation of the Maclaurin series for f(x) from the given Maclaurin series for f'(x).

Solution for a multiple-choice question involving initial conditions and integration.

Process of finding the Maclaurin series for a function with given derivatives and conditions.

Explanation of how to find the first four non-zero terms and the general term of the Maclaurin series for a function.

Integration of a function to find its Maclaurin series and application of term evaluation at bounds.

Demonstration of the application of series in solving calculus problems, including Taylor and Maclaurin series.

Explanation of the convergence of the Maclaurin series to the function for a specific range of x values.

Introduction to a separate video for further practice on series-related free response questions.

Transcripts

Browse More Related Video

AP Calculus BC Lesson 10.14 Part 2

Taylor Series Day1

Integration of Taylor's Series | MIT 18.01SC Single Variable Calculus, Fall 2010

Calculus BC – 10.14 Finding Taylor or Maclaurin Series for a Function

Calculus BC – 10.15 Representing Functions as a Power Series

AP Calculus BC Multiple Choice Practice Test (2016 AP CED Problems)

Related Tags

Calculus Series Analysis Derivatives Integration AP Calculus Taylor Series Math Education Advanced Math Video Lesson Mathematics