Calculus BC – 10.14 Finding Taylor or Maclaurin Series for a Function
TLDRIn this calculus lesson, Mr. Bean introduces the concept of Taylor and Maclaurin series, focusing on memorizing the series for e^x, sine x, cosine x, and 1/(1+x). He explains how these series can represent functions and demonstrates how to manipulate them for different problems, including finding the first few terms and converting to summation notation.
Takeaways
- 📚 The lesson focuses on finding Taylor and Maclaurin series, which are polynomial series representations of functions.
- 🔍 A Taylor series is a representation of a function as an infinite sum of terms calculated from the values of the function's derivatives at a single point, while a Maclaurin series is a special case of a Taylor series centered at zero.
- 📈 The Maclaurin series for \( e^x \) is \( 1 + x + \frac{x^2}{2!} + \frac{x^3}{3!} + \cdots \), which is a sum from \( n = 0 \) to infinity of \( \frac{x^n}{n!} \).
- 📉 The Maclaurin series for \( \sin(x) \) is \( x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \cdots \), with terms involving odd powers of \( x \) and alternating signs.
- 🌐 The interval of convergence for the Maclaurin series of \( e^x \) is from negative infinity to infinity, indicating that the series converges for all real numbers.
- 🔄 The derivatives of \( \sin(x) \) and \( \cos(x) \) alternate between sine and cosine, which helps in determining the pattern of their Maclaurin series.
- 📌 Memorizing the Maclaurin series for \( e^x \) and \( \sin(x) \) is crucial for manipulating and applying Taylor series in various problems.
- 🔢 The series for \( \frac{1}{1+x} \) is a geometric series and can be represented as \( 1 - x + x^2 - x^3 + \cdots \), which is valid for \( |x| < 1 \).
- 📘 The Maclaurin series for \( \cos(x) \) is similar to that of \( \sin(x) \) but involves even powers of \( x \) and alternating signs, reflecting the alphabetical order of cosine and sine.
- 📚 The lesson emphasizes the importance of understanding and memorizing these series for solving calculus problems involving function approximations and manipulations.
Q & A
What is the main focus of the lesson?
-The main focus of the lesson is on how to find a Taylor series or a Maclaurin series.
What is the relationship between a Taylor series and a polynomial series?
-A Taylor series is similar to a polynomial series, and it follows the same pattern as a Taylor polynomial.
What is the difference between a Taylor series and a Maclaurin series?
-A Taylor series can be centered at any point 'c', while a Maclaurin series is specifically centered at 'c = 0', simplifying the formula by eliminating the 'minus c' part.
What are the four common functions whose Maclaurin series are important to memorize?
-The four common functions are e^x, sine x, cosine x, and 1/(1 + x).
How is the Maclaurin series for e^x derived?
-The Maclaurin series for e^x is derived by recognizing that all derivatives of e^x at x=0 are equal to e^0, which is 1. This results in the series 1 + x + x^2/2! + x^3/3! + ..., which is the sum of x^n/n! from n=0 to infinity.
What is the pattern of the derivatives of sine x in the Maclaurin series?
-The derivatives of sine x alternate between sine and cosine, with signs changing between positive and negative, and the pattern repeats every four derivatives.
How does the Maclaurin series for sine x look like?
-The Maclaurin series for sine x is -x + x^3/3! - x^5/5! + x^7/7! - ..., which is the sum of (-1)^n * x^(2n+1)/(2n+1)! from n=0 to infinity.
What is the interval of convergence for the Maclaurin series of e^x?
-The interval of convergence for the Maclaurin series of e^x is from negative infinity to infinity.
How is the Maclaurin series for 1/(1 + x) related to a geometric series?
-The Maclaurin series for 1/(1 + x) is equivalent to the sum of x^n from n=0 to infinity, which is a geometric series with a common ratio of x.
What is the significance of memorizing the Maclaurin series for these four functions?
-Memorizing the Maclaurin series for these four functions allows for easier manipulation and application of Taylor series in various mathematical problems.
Outlines
📚 Introduction to Taylor and Maclaurin Series
This paragraph introduces the topic of the lesson, focusing on Taylor and Maclaurin series in calculus. The instructor, Mr. Bean, explains that a Taylor series is akin to a polynomial series and is used to approximate functions. The Maclaurin series is a special case of the Taylor series centered at c=0, simplifying the formula. The lesson aims to teach students how to derive and memorize the Maclaurin series for four common functions: e^x, sine x, cosine x, and 1/(1+x). The instructor emphasizes the importance of memorization for ease of use in future problems and provides a brief overview of the process to find the Maclaurin series for e^x, highlighting the steps and the resulting formula.
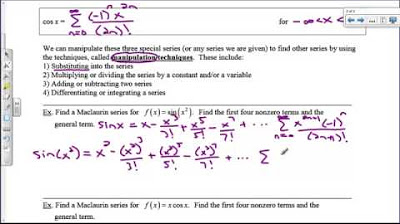
🔍 Deriving the Maclaurin Series for Sine and Cosine
In this paragraph, the instructor continues the lesson by deriving the Maclaurin series for sine x. The process involves identifying the derivatives of the sine function and their values at x=0, which alternate between 0 and 1, and then 0 again. The resulting series for sine x is presented with alternating signs and odd powers of x, divided by the factorial of the exponent. The instructor also discusses memorization techniques, comparing the patterns of sine and cosine series and using alphabetical order as a mnemonic. Additionally, the paragraph touches on the series for 1/(1+x), noting its relationship to a geometric series and its interval of convergence.
📘 Applying Maclaurin Series to Functions and Memorization Tips
The final paragraph of the script discusses applying the Maclaurin series to various functions, specifically showing how to modify the series for e^x and sine x when the function is squared or involves a different power of x. The instructor demonstrates how to adjust the series by replacing x with x^2 and explains the resulting changes in the series terms. Tips for memorizing the series are reiterated, and the instructor provides a method to remember the pattern of sine and cosine series by associating them with odd and even numbers. The paragraph concludes with a brief mention of the next lesson and a sign-off from Mr. Bean.
Mindmap
Keywords
💡Taylor Series
💡Maclaurin Series
💡Polynomial
💡Derivative
💡Summation
💡Factorial
💡Convergence
💡Ratio Test
💡Geometric Series
💡Series Manipulation
Highlights
Introduction to the topic of Taylor and Maclaurin series in calculus.
Explanation of the similarity between Taylor series and polynomial series.
The concept that the Taylor series equals the function for many common functions.
Focus on Maclaurin series, which is a special case of Taylor series centered at c=0.
Learning to find the Maclaurin series for four specific functions.
Memorization of the Maclaurin series for e^x, sine x, cosine x, and 1/(1+x).
Derivation of the Maclaurin series for e^x using the properties of the exponential function.
The summation notation for the Maclaurin series of e^x and its pattern.
Understanding the interval of convergence for the Maclaurin series of e^x.
Derivation of the Maclaurin series for sine x using its derivatives.
Pattern recognition in the Maclaurin series for sine x with alternating signs and odd powers.
Summation notation for the Maclaurin series of sine x and its nth term.
A mnemonic device for remembering the series of cosine and sine based on alphabetical order.
Explanation of the Maclaurin series for 1/(1+x) and its alternating pattern.
The connection between the series 1/(1-x) and the geometric series formula.
Application of the Maclaurin series to find the series for sine(x) squared.
Adjusting the summation notation for modified functions, such as sine(x) squared.
Example problem solving using the Maclaurin series for e^(x^2).
Final review and mastery check preparation for the upcoming lessons.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: