AP Calculus BC Lesson 10.6 Part 1

Elizabeth Fein

14 Jan 202320:53

EducationalLearning

32 Likes 10 Comments

TLDRThe video script discusses the direct comparison test for determining the convergence or divergence of series. It explains that if a larger series converges, a smaller series must also converge, but the converse is not necessarily true. The script provides examples of comparing series with different functions, such as 1/n^2 plus a constant, and using known series like the harmonic series and geometric series to draw conclusions. It also introduces the concept of the limit comparison test, which is further explored in a subsequent video.

Takeaways

📈 The Direct Comparison Test is a method used to determine the convergence or divergence of a series by comparing it to another known series.
🔄 If a series with larger terms converges, then a series with smaller terms must also converge, but the converse is not necessarily true.
🔢 The test states that if 0 < a_n ≤ b_n, and the series Σb_n converges, then the series Σa_n also converges.
🚫 If the smaller series diverges, the larger series must also diverge, but we cannot determine the status of the larger series if the smaller one converges.
🌰 Examples are provided to illustrate the Direct Comparison Test, including comparisons with series like 1/4^n and 1/n^2.
📊 The script explains that the behavior of the series can be inferred by comparing their growth rates and using known properties of standard series.
🔍 The nth term test is mentioned as a preliminary method to check for convergence, but it may be inconclusive and require further testing.
🔄 The script discusses the use of the Limit Comparison Test for series that do not have simple convergence properties.
📈 The geometric series test is referenced as a tool for determining the convergence of specific series, such as 3^n/4^n.
🚫 The script concludes that out of several series discussed, only one (Series 2) converges, with the others either diverging or inconclusive with the given tests.

Q & A

What is the direct comparison test used for in calculus?
-The direct comparison test is used to determine the convergence or divergence of a series by comparing it to another series with a known convergence or divergence behavior.
How does the direct comparison test work?
-The direct comparison test works by comparing the terms of the series under investigation (a_n) with the terms of a known series (b_n). If the series b_n converges and a_n ≤ b_n for all n, then a_n also converges. If b_n diverges and a_n ≤ b_n for all n, then a_n also diverges.
What is the significance of the series 1/4^n in the script?
-The series 1/4^n is used as a comparison series for the direct comparison test. It is a converging geometric series, which helps to determine the convergence of the series 1/4^n + 6/4^n.
What is the role of the P-series test in the examples provided?
-The P-series test is used to determine the convergence of the series 1/n^2 and 1/n, which are then used for comparison in the direct comparison test to assess the convergence of other series.
How does the script handle the case where the smaller series diverges?
-If the smaller series (a_n) diverges, the script indicates that the larger series (b_n) must also diverge, as a diverging series cannot be smaller than a converging one.
What is the conclusion for the series 1/n^2 + 9 in the script?
-The script concludes that the series 1/n^2 + 9 converges by comparing it with the series 1/n^3 and using the direct comparison test, as the latter is known to converge.
Why is the nth term test inconclusive for the series 1/(n*ln(n) + 3)?
-The nth term test is inconclusive for this series because the limit as n approaches infinity of the term a_n (1/(n*ln(n) + 3)) does not tend to zero or infinity, but instead tends to an indeterminate form, requiring a different test for convergence.
What is the limit comparison test, and how is it different from the direct comparison test?
-The limit comparison test is used to determine the convergence of a series by comparing it to another series with known convergence properties, but instead of direct inequality comparison, it involves taking the limit of the ratio of the two series' terms as n approaches infinity. It differs from the direct comparison test in that it can handle cases where the terms do not have a simple inequality relationship.
What is the significance of the limit comparison test result for the series 1/(4n)?
-The limit comparison test result for the series 1/(4n) is significant because it shows that the series diverges, as the limit as n approaches infinity of the ratio of 1/(4n) to 1/n is 1/4, which is a positive finite number, indicating that the series behaves similarly to the divergent harmonic series.
Which series in the script is the only one that converges?
-The only series that converges in the script is the series 3^n/(4^n), as determined by the direct comparison test with the converging geometric series 3^n/(4^n).
What is the conclusion for the series 1/(n^3)?
-The conclusion for the series 1/(n^3) is that it diverges, as it is compared to the harmonic series 1/n, which is known to diverge.

Outlines

00:00

📚 Introduction to the Direct Comparison Test

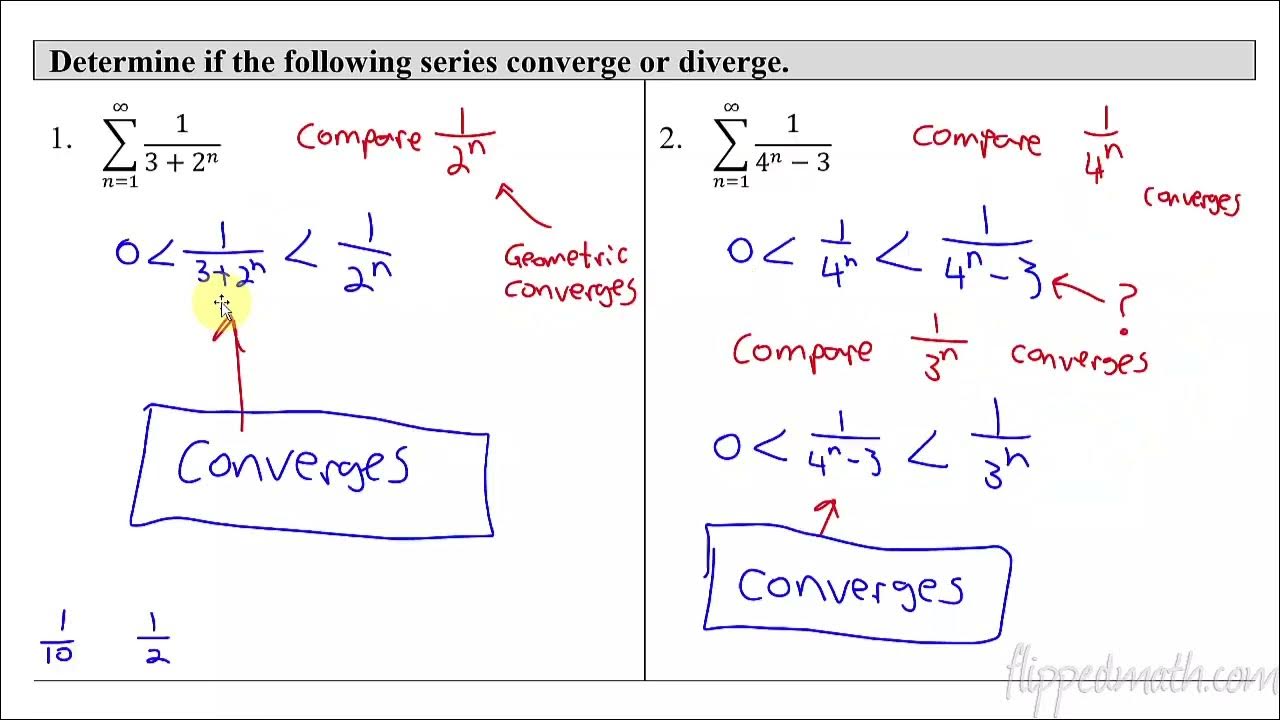
This paragraph introduces the concept of the Direct Comparison Test, a method used to determine the convergence or divergence of a series by comparing it to another known series. It explains the fundamental principle that if a larger series converges, the smaller one must also converge, and vice versa. The paragraph provides examples to illustrate how to apply the test and discusses the implications of the results, highlighting that convergence of the smaller series is guaranteed if the larger one converges, but divergence of the smaller series does not necessarily imply the same for the larger one.

05:01

🔍 Analyzing Series Convergence with Direct Comparison

This paragraph delves into the application of the Direct Comparison Test through specific examples. It demonstrates how to select a comparable series and set up the inequality to analyze the convergence of the given series. The paragraph explains that if the known series converges, it can be inferred that the series being compared also converges, and if the known series diverges, it provides limited information about the unknown series. The examples given illustrate the process of comparing terms and determining the behavior of the series under examination.

10:02

🤔 Complex Cases in Direct Comparison Test

The paragraph discusses more complex scenarios where the Direct Comparison Test is applied. It explains that sometimes the comparison does not provide a definitive answer about the convergence or divergence of the series, especially when the series being compared converges to a larger value or diverges. The paragraph emphasizes the need to choose an appropriate series for comparison and the challenges that arise when the series terms do not straightforwardly relate to each other.

15:03

🧠 Advanced Series Comparisons and Limit Comparison Test

This paragraph presents advanced concepts in series comparison, including the Limit Comparison Test. It explains how to determine if two series behave similarly by taking the limit as n approaches infinity of the ratio of their terms. The paragraph also discusses the implications of the limit result, stating that if the limit is a positive finite number, the series behave similarly. It concludes by applying this concept to determine the convergence or divergence of a series, highlighting that if the compared series diverges, the series under examination also diverges.

20:03

📈nth Term Test and Direct Comparison for Specific Series

The paragraph explores the use of the nth Term Test and Direct Comparison Test for specific series. It explains the process of using the nth Term Test to determine if a series diverges and then applies the Direct Comparison Test to compare the series with known diverging or converging series. The paragraph concludes that only the second series in the given examples converges, while the others do not, providing a clear conclusion based on the application of these tests.

Mindmap

Keywords

💡Direct Comparison Test

The Direct Comparison Test is a method used to determine the convergence or divergence of a series by comparing it to another series with a known convergence or divergence behavior. In the context of the video, this test is used to analyze various series and deduce whether they converge or diverge based on the comparison with other series. For example, if a series is smaller than a known converging series, it must also converge.

💡Convergence

Convergence in mathematics, particularly in the context of series, refers to the property of the series where the sum of its terms approaches a finite value as the number of terms increases indefinitely. The video discusses the conditions under which a series converges, such as when comparing a series to a known converging series using the Direct Comparison Test.

💡Divergence

Divergence in the context of mathematical series indicates that the series does not converge; instead, the sum of its terms increases without bound as more terms are added. The video explains that if a series is larger than a known diverging series, it must also diverge.

💡Series

A series in mathematics is the sum of the terms of a sequence, often approaching a limit or sum as the number of terms increases. The video focuses on various types of series, such as geometric and harmonic series, and their convergence or divergence properties.

💡Geometric Series

A geometric series is a type of mathematical series where each term is obtained by multiplying the previous term by a fixed non-zero number called the common ratio. The video mentions the geometric series as an example of a series that converges if the absolute value of the common ratio is less than one.

💡Harmonic Series

The harmonic series is the sum of the reciprocals of the natural numbers. It is a well-known divergent series in mathematics. In the video, the harmonic series is used as a reference point to compare other series and determine their divergence.

💡nth Term Test

The nth Term Test is a method used to determine the convergence or divergence of a series by examining the limit of the sequence's nth term as n approaches infinity. If the limit is zero, the series may converge; if the limit is a non-zero constant or if it does not exist, the series diverges.

💡Limit Comparison Test

The Limit Comparison Test is a technique used to determine the convergence or divergence of a series by comparing it to another series with a known convergence or divergence behavior and taking the limit of the ratio of the corresponding terms as n approaches infinity. If the limit is a finite non-zero number, the series have the same convergence or divergence behavior.

💡Natural Logarithm

The natural logarithm, denoted as ln or log_e, is the logarithm to the base e (where e is the mathematical constant approximately equal to 2.71828). It is a fundamental concept in calculus and is used in various mathematical applications, including the analysis of series in the video.

💡P-Series Test

The P-Series Test, also known as the p-test, is a method for determining the convergence of certain series where the terms are of the form 1/n^p. The series converges if p > 1 and diverges if p ≤ 1. This test is applied to series with terms that are powers of the natural number n.

💡Inequality

In mathematics, an inequality is a mathematical relation that shows the relationship between two expressions, indicating that one expression is less than, greater than, or equal to another. In the context of the video, inequalities are set up to compare different series and apply convergence tests.

Highlights

The direct comparison test is introduced, which is sometimes also called the comparison test.

The direct comparison test states that if 0 is less than a sub n is less than or equal to B sub n, and the series of B sub n converges, then the series of a sub n also converges.

If the smaller series a sub n diverges, then the larger series B sub n must also be divergent.

Conversely, if the larger series B sub n diverges, it does not provide conclusive information about the convergence or divergence of the smaller series a sub n.

The series 1 over 4 to the power of n is used as a comparison series because it converges, allowing the conclusion that the series 1 over 4 to the power of n plus 6 also converges.

The P series test is mentioned as a known method to determine the convergence of a series like 1 over N squared.

The series 1 over 3 N squared plus 5 is compared to 1 over N squared, and it is concluded that it converges by direct comparison since the latter is known to converge.

For the series 1 over 3 to the power of n minus 2, the comparison with 1 over 2 to the power of n leads to the conclusion that it converges, as the latter series is known to converge.

The concept of using the comparison test to determine the convergence or divergence of a series is illustrated with various examples.

The direct comparison test is distinguished from the limit comparison test, which will be discussed in a future video.

A series can only be determined to converge or diverge by comparison if the compared series has a known convergence or divergence status.

The convergence of a series is demonstrated by comparing it with a series that is smaller and known to converge.

The divergence of a series is shown by comparing it with a series that is larger and known to diverge.

The nth term test is mentioned as a potential method to determine the convergence or divergence of a series.

The limit comparison test is introduced as a method to compare two series by taking the limit as n approaches infinity of the ratio of their terms.

The series 1 over the natural log of n plus 1 is analyzed for convergence or divergence by comparison with other series.

The conclusion is reached that the series 1 over the natural log of n plus 1 diverges by comparison with the harmonic series, which is known to diverge.

The series 3 to the power of n over 4 to the power of n plus 5 is shown to converge by direct comparison with the known converging series 3 to the power of n over 4 to the power of n.

The series 1 over 4 n is determined to diverge by the limit comparison test, as it is compared to the harmonic series.

Only the second series, 3 to the power of n over 4 to the power of n plus 5, is found to converge among the given options.

Transcripts

Browse More Related Video

Direct comparison test | Series | AP Calculus BC | Khan Academy

Calculus BC – 10.6 Comparison Tests for Convergence

Worked example: limit comparison test | Series | AP Calculus BC | Khan Academy

Series Tests - Limit Comparison Test

Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test

AP Calculus BC Lesson 10.6 Part 2

Related Tags

Mathematics Calculus Series Convergence Direct Comparison Infinite Series Geometric Series Harmonic Series Limit Comparison Educational Content Math Tutorial