Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test
TLDRThis video script offers a comprehensive review of various convergence tests for infinite series, essential for determining whether a series converges or diverges. It covers the divergence test, geometric series test, p-series test, telescoping series, integral test, ratio test, root test, direct comparison test, limit comparison test, alternating series test, and absolute convergence test. Each test is explained with its conditions and application, providing clear guidelines on when to use them. The script also includes examples and step-by-step solutions to illustrate the process of testing series for convergence, making it an informative resource for understanding series analysis.
Takeaways
- 🚫 The Divergence Test: Take the limit of the sequence as n approaches infinity. If the limit does not equal zero, the series diverges.
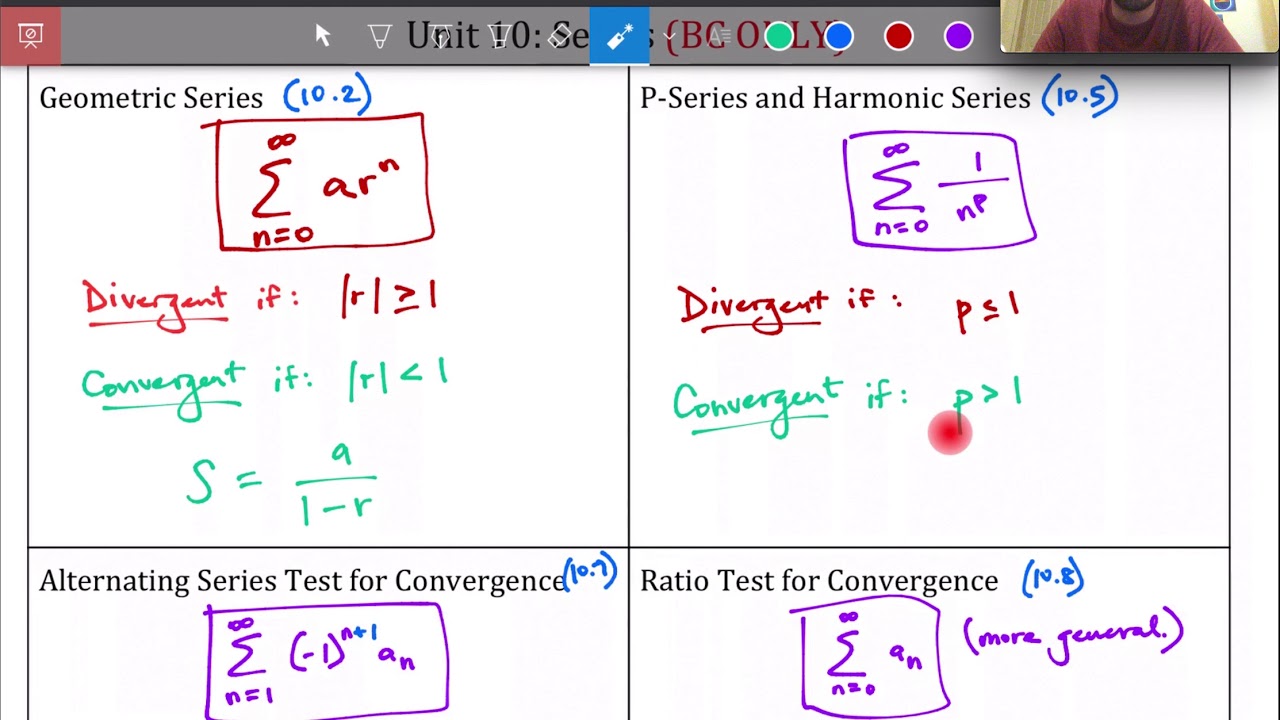
- 📉 Geometric Series: Identify the common ratio r. If |r| < 1, the series converges; if |r| ≥ 1, the series diverges.
- 🔢 P-Series Test: For a series in the form 1/n^p, if p > 1, the series converges; if p ≤ 1, the series diverges.
- 🔍 Telescoping Series: Write the partial sum and evaluate its limit as n approaches infinity. If the limit is finite, the series converges.
- ∫ Integral Test: Convert the sequence to a function and integrate from 1 to infinity. If the integral is finite, the series converges; otherwise, it diverges.
- 📈 Ratio Test: Take the limit of |a_(n+1)/a_n| as n approaches infinity. If the limit is less than 1, the series converges; if greater than 1, it diverges; if equal to 1, the test is inconclusive.
- √ Root Test: Take the limit of the nth root of |a_n| as n approaches infinity. If the limit is less than 1, the series converges; if greater than 1, it diverges; if equal to 1, the test is inconclusive.
- 🔄 Direct Comparison Test: Compare the given series to a known convergent or divergent series. If a larger series converges, so does the smaller one; if a smaller series diverges, so does the larger one.
- 🔗 Limit Comparison Test: Compare the limit of a_n/b_n to a positive finite number. If one series converges or diverges, so does the other.
- ➖ Alternating Series Test: For series with alternating signs, ensure the limit of a_n as n approaches infinity is zero and that a_n is decreasing. If both conditions are met, the series converges.
Q & A
What is the Divergence Test and how is it used to determine the convergence of a series?
-The Divergence Test involves taking the limit of the sequence a_sub_n as n approaches infinity. If this limit does not equal zero, the series diverges. If it equals zero, the series may converge or may diverge, and another test is needed to determine the convergence.
How can you identify a Geometric Series and what conditions cause it to converge?
-A Geometric Series is identified by its form, which typically includes a constant 'a' and a common ratio 'r' raised to the power of n or n-1. The series converges if the absolute value of 'r' is less than one. If the absolute value of 'r' is greater than or equal to one, the series diverges.
What is the p-Series Test and under what condition does it indicate convergence?
-The p-Series Test applies to series in the form of 1/n^p. The series converges if 'p' is greater than 1. If 'p' is less than or equal to 1, the series diverges.
Can you explain the concept of a Telescoping Series and how to determine its convergence?
-A Telescoping Series is one where terms cancel out when written out, often in the form of a_sub_n = 1 - 1/(n+1) + 1/(n+1) - 1/(n+2), and so on. To determine convergence, write a general formula for the partial sum and evaluate the limit as n approaches infinity. If a finite value 'l' is obtained, the series converges.
What is the Integral Test and how does it relate to the convergence of a series?
-The Integral Test involves comparing the series to the integral from 1 to infinity of a positive, continuous, and decreasing function f(x). If the integral converges to a finite value, the series also converges. If the integral diverges to infinity, the series diverges.
How does the Ratio Test work and what does the limit result indicate about the series?
-The Ratio Test involves taking the limit as n approaches infinity of the absolute value of (a_(n+1)/a_n). If the result is less than 1, the series converges. If the result is greater than 1 or goes to infinity, the series diverges. If the limit equals 1, the test is inconclusive.
What is the Root Test and how does it help in determining the convergence of a series?
-The Root Test involves taking the limit as n approaches infinity of the nth root of the absolute value of a_sub_n. If the result is less than 1, the series converges. If the result is greater than 1 or goes to infinity, the series diverges. If the result equals 1, the test is inconclusive.
What is the Direct Comparison Test and how does it relate to the convergence of two sequences?
-The Direct Comparison Test compares two sequences, a_sub_n (small sequence) and b_sub_n (big sequence). If the big series converges, the small series will also converge. Likewise, if the small series diverges, the big series diverges as well.
Can you describe the Limit Comparison Test and its use in determining the convergence of two series?
-The Limit Comparison Test involves taking the limit as n approaches infinity of a_sub_n divided by b_sub_n for two different sequences. If this limit is a finite positive number, both series will either converge or diverge together.
What is the Alternating Series Test and what conditions must be met for a series to be considered convergent?
-The Alternating Series Test applies to series with alternating signs, typically in the form of (-1)^(n+1) * a_sub_n. Two conditions must be met: the series must pass the divergence test by having the limit of a_sub_n as n approaches infinity equal to zero, and the sequence must be decreasing (a_sub_n >= a_(n+1)).
How can you determine if a series is absolutely convergent, conditionally convergent, or divergent?
-To determine the type of convergence: (1) Check if the original series converges. (2) Check if the series of absolute values converges. If both are convergent, the series is absolutely convergent. If the original series converges but the series of absolute values diverges, the series is conditionally convergent. If either the original series or the series of absolute values diverges, the series is divergent.
Outlines
📚 Series Convergence Tests Overview
This paragraph introduces a variety of mathematical tests used to determine whether a given series will converge or diverge. It begins with the Divergence Test, which involves taking the limit of the sequence's term as n approaches infinity; if the limit is non-zero, the series diverges. The paragraph continues with the Geometric Series Test, emphasizing the importance of identifying the common ratio 'r' and its absolute value; if less than one, the series converges, otherwise, it diverges. The p-Series Test is also discussed, highlighting that for the series in the form of 1/n^p to converge, 'p' must be greater than one. The Telescoping Series Test is then introduced, explaining how to identify and sum series with canceling terms. Lastly, the Integral Test is briefly mentioned, suggesting that if the integral from one to infinity of a positive, continuous, and decreasing function 'f(x)' has a finite value, the series converges.
🔍 Deep Dive into Series Convergence Tests
In this paragraph, the discussion on series convergence tests continues with a detailed explanation of the Integral Test. It explains that for a series with a positive, continuous, and decreasing function 'f(n)', if the integral from one to infinity of 'f(x)dx' yields a finite value, the series converges; otherwise, it diverges. The Ratio Test is then described, stating that the limit of the absolute value of the ratio of consecutive terms (a_(n+1)/a_n) as n approaches infinity, if less than one, indicates convergence, while greater than one or infinity indicates divergence. The Root Test is also outlined, where the limit of the nth root of the absolute value of the term (a_n) as n approaches infinity, if less than one, results in convergence, and greater than one or infinity in divergence. The Direct Comparison Test and Limit Comparison Test are introduced, explaining how the convergence or divergence of one series can be used to infer the behavior of another series. The paragraph concludes with the Alternating Series Test, which requires two conditions: passing the divergence test with a limit of zero and the sequence being decreasing.
📉 Application of Convergence Tests to Specific Series
This paragraph applies the previously discussed convergence tests to specific series problems. It starts with a series involving '2n^2 + 5' over '7n^2 - 4', suggesting the use of the Divergence Test and L'Hôpital's Rule to determine divergence. The paragraph then examines a series with 'cube root of n' divided by 'n to the fifth power', identifying it as a p-series and concluding convergence due to the exponent being greater than one. A geometric series example with 'five times one fourth raised to the power of n minus one' is provided, with the sum calculated using the formula for geometric series when the common ratio's absolute value is less than one. The paragraph also discusses an alternating series with 'negative 1 to the power of n' divided by the square root of 'n', confirming convergence using the Alternating Series Test and determining conditional convergence by examining the absolute value of the series.
📌 Further Exploration of Series Convergence
The paragraph delves deeper into series convergence with examples and tests. It begins with a series '1/n * (n + 1)', suggesting partial fraction decomposition to identify it as a telescoping series, which converges to a finite value. Another series '1/n^2 + 4' is discussed, recommending the Direct Comparison Test to establish convergence by comparing it to a known p-series. The paragraph then considers a series '1/sqrt(n - 2)', using the Divergence Test and Direct Comparison Test to conclude divergence. The Integral Test is also applied to this series, confirming divergence due to the integral's result of infinity. The paragraph continues with a series 'sqrt(n) / (n^3 + 2)', recommending the Limit Comparison Test, which shows convergence by comparing it to a simplified p-series. The Root Test is applied to '3n^2 - 9 / (7n^2 + 4)^n', leading to the conclusion of convergence as the limit is less than one.
🔢 Convergence Tests for Complex Series
In this paragraph, complex series are analyzed using various convergence tests. A series '2^n / n!' is introduced, and the Ratio Test is applied to determine its convergence. The limit of the ratio of consecutive terms, when simplified, shows that the series converges as the limit approaches zero. This final example wraps up the discussion by demonstrating the application of the Ratio Test to a series with a factorial in the denominator, a common scenario in convergence analysis.
Mindmap
Keywords
💡Divergence Test
💡Geometric Series
💡P Series Test
💡Telescoping Series
💡Integral Test
💡Ratio Test
💡Root Test
💡Direct Comparison Test
💡Limit Comparison Test
💡Alternating Series Test
Highlights
Introduction to different tests for determining convergence or divergence of a series.
Explanation of the Divergence Test and its application.
Identification of the form and value of 'r' in a Geometric Series.
Convergence criteria for a series based on the value of 'p' in a p-Series Test.
Understanding Telescoping Series and the method to find their sum.
The Integral Test and its conditions for determining series convergence.
The Ratio Test and how to apply it to series for convergence assessment.
The Root Test and its use in series convergence analysis.
Direct Comparison Test and its principle for series comparison.
Limit Comparison Test and its method to compare two series.
Alternating Series Test and its two conditions for convergence.
Absolute Value Test to determine if a series is absolutely convergent or conditionally convergent.
Example problem solving using Divergence Test and L'Hôpital's Rule.
Analysis of a series with p-Series form and its convergence criteria.
Geometric Series convergence determination and sum calculation.
Alternating Series Test application with an example problem.
Conditional and Absolute Convergence analysis in series.
Telescoping Series problem-solving approach using partial fraction decomposition.
Direct Comparison Test application with an example series.
Application of the Integral Test to determine series divergence.
Limit Comparison Test recommendation and its application.
Root Test application for series convergence with an example.
Ratio Test application to determine convergence of a series.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: