AP Calculus BC Lesson 10.6 Part 2
TLDRThe video script delves into the limit comparison test, a method for determining the convergence or divergence of series. It contrasts this with the direct comparison test and illustrates the process with examples. The key concept is that if the limit of the ratio of two series' terms approaches a finite positive value, the series either both converge or both diverge. The examples demonstrate how to identify comparable series and calculate the critical limit to ascertain the series' behavior. The script also highlights the importance of accurate limit computation and the implications of non-positive finite results in series comparison.
Takeaways
- 📚 The limit comparison test is a method to determine the convergence or divergence of two series by comparing their behavior as n approaches infinity.
- 🔍 To apply the test, we need to identify two series, a_n and b_n, where a_n > 0 and b_n > 0, and then evaluate the limit of a_n/b_n as n approaches infinity.
- 🌟 If the limit is a finite positive number, both series converge or diverge together; otherwise, the test does not apply or they have different behaviors.
- 📈 The direct comparison test is different from the limit comparison test as it compares the actual sizes of the series terms, not their limits.
- 🌰 An example in the script compares the series 1/(n^3 - 2n^2 + 2n) with 1/n^3, showing that both series converge since the limit comparison yields a finite positive number (1).
- 🔢 For the series 2/(√(5n) - 4), the limit comparison with 1/√n results in a finite positive number (2/√5), indicating both series diverge as the compared series diverges by the p-series test.
- 🔍 When comparing series, it's crucial to choose a b_n that behaves similarly to a_n, focusing on the highest powers in the numerator and denominator.
- 📊 The script also discusses a shortcut method for multiple-choice questions, using the nth term test to quickly determine divergence without a full limit comparison.
- 🌟 The limit comparison test is a powerful tool for series analysis but requires careful selection of comparable series and accurate limit calculations.
- 📝 It's important to double-check the results, especially when using shortcuts, to ensure the validity of the comparison and the accuracy of the conclusion.
Q & A
What is the limit comparison test and how does it relate to the direct comparison test?
-The limit comparison test is a method for determining the convergence or divergence of two series by comparing their behavior as n approaches infinity. It states that if the limit of the ratio of the nth terms of two series is a finite positive number, then both series either converge or diverge together. This is different from the direct comparison test, which compares the actual sizes of the terms in two series, rather than their limit behavior.
How do you identify a_n and b_n for the limit comparison test?
-In the limit comparison test, a_n is the given series whose convergence or divergence is being investigated, and b_n is a series that behaves similarly to a_n. The choice of b_n should be such that it is easy to determine its convergence or divergence and that it reflects the dominant behavior of a_n.
What is the significance of the limit being a finite positive number in the limit comparison test?
-If the limit as n approaches infinity of the ratio a_n/ b_n is a finite positive number, it indicates that the series a_n and b_n have the same behavior in terms of convergence or divergence. If b_n converges, then a_n also converges, and if b_n diverges, a_n also diverges.
How does the limit comparison test determine the convergence or divergence of a series?
-The limit comparison test determines the convergence or divergence of a series by comparing it to another series whose behavior is known. If the limit of the ratio of their corresponding terms as n approaches infinity is a finite positive number, the series will converge or diverge together.
What is the role of the limit in the limit comparison test?
-The limit in the limit comparison test is crucial as it provides information about the long-term behavior of the series. By taking the limit of the ratio of the nth terms of the given series and a comparison series as n approaches infinity, one can determine if the series will have the same convergence or divergence behavior.
What happens if the limit comparison test yields an infinite or undefined result?
-If the limit comparison test yields an infinite or undefined result, it means that the behavior of the two series being compared is not similar enough for the test to be applicable. In such cases, the test cannot be used to determine the convergence or divergence of the series, and another method must be employed.
Can the limit comparison test be used to compare series with non-positive terms?
-The limit comparison test is generally not applicable to series with non-positive terms because the test relies on the assumption that both series have positive terms. If the terms are non-positive, other tests such as the integral test or the comparison with an integral may be more appropriate.
What is the difference between the limit comparison test and the ratio test?
-The limit comparison test compares the limit of the ratio of the nth terms of two series, while the ratio test compares the ratio of successive terms of a single series. The limit comparison test is useful when comparing two series with similar behavior, whereas the ratio test is used to determine the convergence or divergence of a single geometric series.
How does the limit comparison test handle series with alternating signs?
-The limit comparison test may not be directly applicable to series with alternating signs, as the behavior of such series can be more complex. For alternating series, other tests like the alternating series test or the Leibniz test might be more suitable.
What is the relationship between the limit comparison test and the p-series test?
-The limit comparison test can be used to compare a given series with a p-series, which is a series of the form 1/n^p. If the limit of the ratio of the nth terms of the given series and the p-series is a finite positive number, and the p-series converges (p > 1), then the given series also converges. If the p-series diverges (p ≤ 1), then the given series also diverges.
Can the limit comparison test be used to compare series with different growth rates?
-The limit comparison test is most effective when the series being compared have similar growth rates. If the growth rates are significantly different, the test may not provide a clear conclusion about the convergence or divergence of the series. In such cases, it might be necessary to find a more appropriate comparison series or use a different convergence test.
Outlines
📚 Introduction to Limit Comparison Test
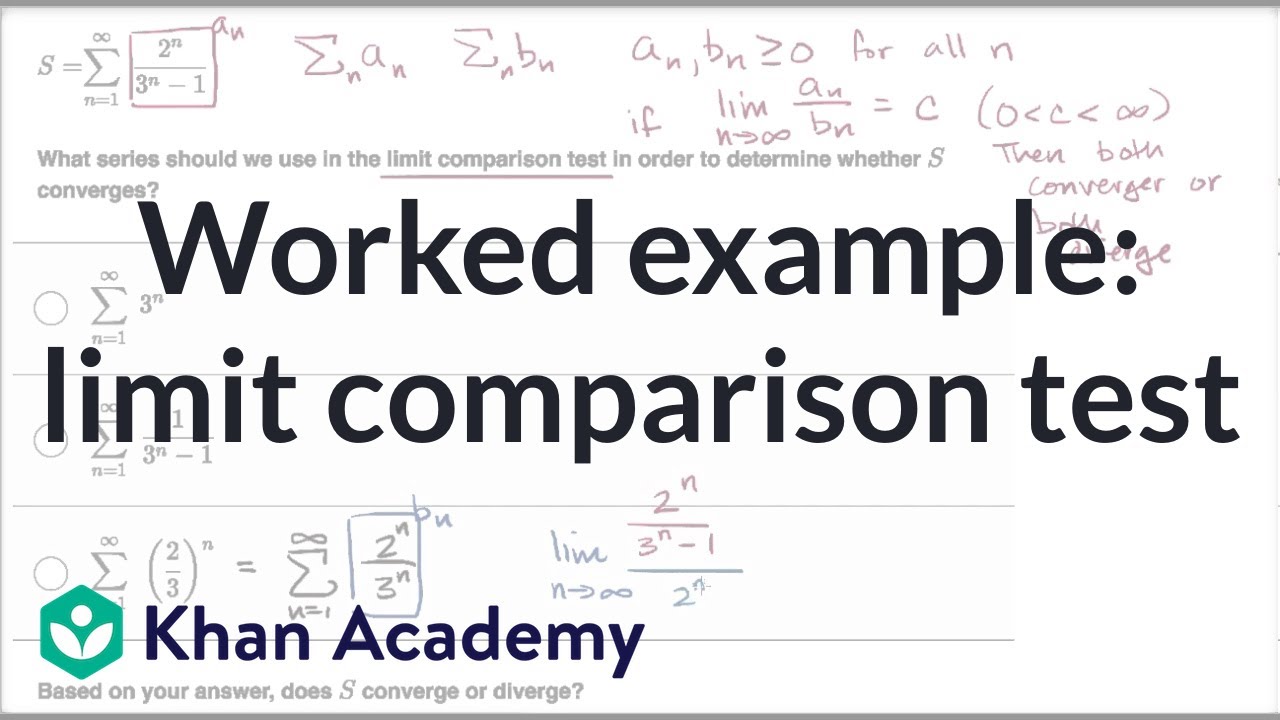
This paragraph introduces the concept of the limit comparison test, a method for determining the convergence or divergence of two series. It explains that if the limit of the ratio of the terms of two series approaches a finite positive number as n tends to infinity, then both series either converge or diverge together. The paragraph contrasts this approach with the direct comparison test from a previous video and provides an example to illustrate the process of identifying the functions a_n and B_n for the test.
🔍 Applying the Limit Comparison Test
The paragraph delves into the application of the limit comparison test with a specific example. It guides through the process of identifying a_n and B_n for the series, setting up the limit comparison, and simplifying the expression to find the limit. The example demonstrates how to conclude the convergence or divergence of the series based on the limit's value. The paragraph also highlights the importance of choosing the right series for comparison and notes the implications of obtaining a non-positive finite number.
📈 Multiple Choice Approach and Double-Checking
This section discusses a shortcut method for multiple-choice questions related to the limit comparison test. It provides a quick way to predict the divergence of series by comparing terms without the full limit comparison process. The paragraph then emphasizes the importance of double-checking the predictions by actually performing the limit comparison test to ensure accuracy, especially when time is not a constraint.
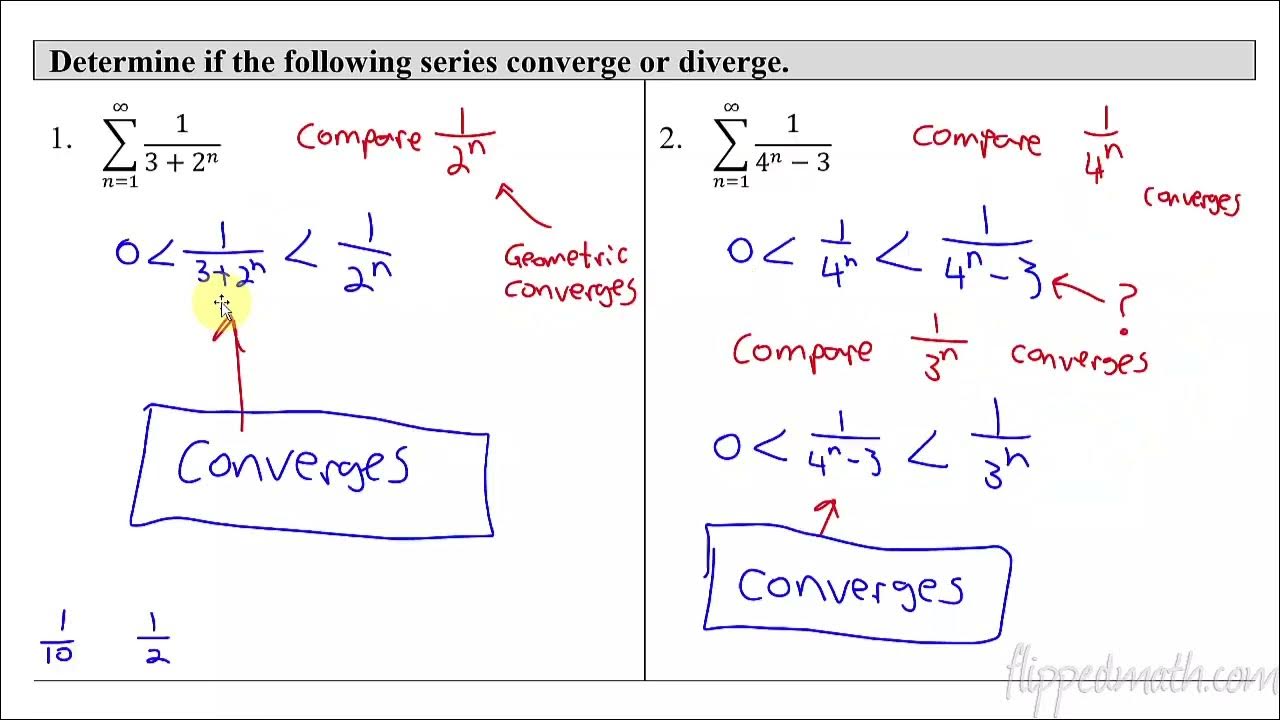
🤔 Determining Convergence Through Limit Comparison
The paragraph focuses on using the limit comparison test to determine the convergence or divergence of specific series. It explains the process of selecting a comparable series and verifying that the limit comparison is valid by ensuring a positive finite number. The paragraph provides examples of series that can and cannot be compared using the limit comparison test, and it illustrates how to find the correct series for comparison by examining the degrees of the terms.
🌟 Convergence of e^n Series via Limit Comparison
This paragraph examines the convergence of a series involving e^n through limit comparison. It discusses the inconclusiveness of the nth term test and the application of the limit comparison test with a series of e^n over 3^n. The paragraph explains how to simplify the comparison, verify the limit for validity, and determine the convergence of the series based on the limit's value. It concludes that the series converges, as the limit comparison yields a finite positive number.
Mindmap
Keywords
💡Limit Comparison Test
💡Direct Comparison Test
💡Convergence
💡Divergence
💡p-Series
💡nth Term Test
💡Greatest Power
💡Horizontal Asymptote
💡Geometric Series
💡Harmonic Series
Highlights
Exploring the limit comparison test for determining the convergence or divergence of series.
The limit comparison test states that if the limit of a_sub_n over b_sub_n approaches a finite positive number, then both series have the same behavior.
The difference between the direct comparison test and the limit comparison test is that the former compares the size of two series, while the latter compares their behavior.
Example given: Determine the convergence or divergence of the series 1/(n^3 - 2n^2 + 2n).
In the example, a_sub_n is chosen as 1/(n^3 - 2n^2 + 2n) and b_sub_n is chosen as 1/n^3 for comparison.
The limit comparison test is applied by taking the limit as n approaches infinity of a_sub_n divided by b_sub_n.
The limit calculation results in a finite positive number (1), indicating both series have the same behavior.
The series 1/n^3 is a p-series with p=3, which converges, so the given series also converges by the limit comparison test.
Another example: Determine the convergence or divergence of the series 2/(sqrt(5n) - 4).
For this series, the comparison is made with the series 1/(rad n), and the limit comparison test is applied.
The limit comparison shows that the series diverges, as the comparable series diverges by the p-series test.
A third example involves the series 6n^4 + 3n^2 / (n + 4n^5) and is compared to 1/n.
The limit comparison test indicates that this series diverges, as the comparable series diverges by the harmonic series test.
A shortcut method for multiple-choice questions is described, which is not always 100% accurate but can be useful when time is limited.
The shortcut method is applied to quickly determine the divergence of series based on the p-series and nth term tests.
The importance of double-checking the results using the limit comparison test when time allows is emphasized.
A final example examines the series 2*sqrt(n) / (5n^2 - n + 1) and its comparison with the series 1/(sqrt(n))
The series is determined to converge by the limit comparison test, as the comparable series is found to converge.
The limit comparison test is confirmed to be the correct method for comparing series by ensuring a positive finite limit.
The series e^(n+4) / (3^n) is discussed, and its convergence is examined using both the nth term test and the limit comparison test.
The series e^(n+4) / (3^n) is found to converge by the limit comparison test, as the comparable series converges.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: