Total Energy

Bozeman Science
21 Nov 201406:14
EducationalLearning
32 Likes 10 Comments

TLDRIn this AP Physics essentials video, Mr. Andersen explains the concept of total energy in a system, covering kinetic, gravitational potential, and elastic potential energy. He uses a simulation of a red sphere to demonstrate how these energies convert from one form to another, emphasizing that total energy remains constant despite these conversions. The video also touches on internal energy and illustrates how to calculate different forms of energy with examples, highlighting the interrelation between energy and work.

Takeaways
  • 🌟 Total energy of a system is the sum of kinetic, potential, and internal energies.
  • 🏀 Kinetic energy is the energy of motion, calculated using mass and velocity (KE = 0.5 * m * v²).
  • 🌐 Potential energy can be gravitational (PE = m * g * h) or elastic, depending on the object's position or deformation.
  • 🔄 In a perfect system without friction, energy would be conserved and could be converted between forms indefinitely.
  • 💥 Friction and other forces can convert energy into other forms, leading to a loss of mechanical energy over time.
  • 🔋 Internal energy is the energy associated with the movement of particles within an object, which is difficult to calculate and typically not included in AP Physics calculations.
  • 🚀 Work is related to energy; an object with energy has the ability to do work, defined as a force applied over a distance.
  • 📈 The conservation of energy principle states that the total energy in a closed system remains constant, despite energy transformations.
  • 🔄 The video demonstrates the conversion of elastic potential energy to kinetic energy and vice versa in a simulation.
  • 📊 The script provides examples of calculating kinetic and potential energy for a baseball, emphasizing the importance of understanding these concepts for AP Physics.
  • 🔧 The concept of rotational kinetic energy is introduced, hinting at its calculation involving rotational inertia and angular velocity.
Q & A

  • What is the total energy of a system?

    -The total energy of a system is the sum of all the energies within that system, including kinetic energy, potential energy, and internal energy.

  • What types of energy does a moving red sphere with given velocity and stretched spring possess?

    -The red sphere possesses kinetic energy due to its motion and elastic potential energy due to the stretched spring. It also has potential gravitational energy because of its position.

  • What happens to the energies when the red sphere is released?

    -When the red sphere is released, the energies convert back and forth between kinetic and potential energies. Elastic potential energy becomes kinetic energy, and vice versa.

  • How does friction affect the total energy of a system?

    -Friction converts some of the mechanical energy (kinetic and potential) into other forms of energy, such as heat. In a real-world scenario with friction, the system will eventually lose energy and come to rest.

  • What is internal energy?

    -Internal energy is the energy associated with the random motion of particles within an object. For example, the molecules in a glass of water have internal energy due to their movement.

  • How is kinetic energy calculated?

    -Kinetic energy is calculated using the formula KE = 0.5 * m * v^2, where m is the mass of the object and v is its velocity.

  • What factors affect the amount of kinetic energy an object has?

    -The amount of kinetic energy an object has depends on its mass and the square of its velocity. Doubling the mass or velocity will double or quadruple the kinetic energy, respectively.

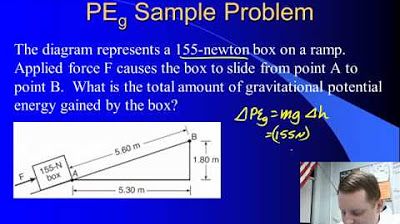
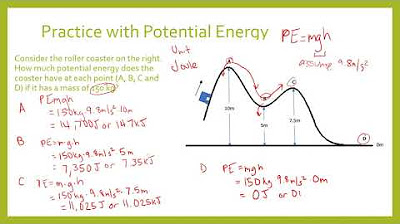
  • How is gravitational potential energy calculated?

    -Gravitational potential energy is calculated using the formula PE = m * g * h, where m is the mass, g is the acceleration due to gravity, and h is the height above the reference point.

  • What is elastic potential energy and how is it calculated?

    -Elastic potential energy is the energy stored in a deformed elastic object, such as a spring. It is calculated using the formula PE = 0.5 * k * x^2, where k is the spring constant and x is the displacement from the equilibrium position.

  • How can changes in position and frictional interactions affect the total energy of a system?

    -Changes in position can lead to conversions between different forms of energy, while frictional interactions can convert mechanical energy into other forms like heat, leading to a decrease in the total mechanical energy of the system over time.

  • What is the relationship between energy and work?

    -Energy is the ability to do work. Work is the application of a force over a distance, and an object with energy has the potential to perform work on another object.

  • How can you predict the behavior of a system over time?

    -By understanding the types of energy present in a system and the interactions between them, one can predict how the total energy will change over time due to factors like position changes and friction.

Outlines
00:00
🌟 Introduction to Total Energy Concepts

This paragraph introduces the concept of total energy in a system, explaining the different forms of energy such as kinetic, gravitational potential, and elastic potential energy. It uses a simulation of a red sphere to illustrate the conversion between these energy forms. The paragraph also touches on the idea of internal energy and the expectation in AP Physics to calculate only kinetic and potential energy. The importance of understanding energy conversion and the relationship between energy and work is emphasized, with an example of a car applying force over a distance to demonstrate work.

05:01
📚 Calculation of Kinetic and Gravitational Potential Energy

This paragraph delves into the specifics of calculating kinetic and gravitational potential energy. It provides a step-by-step explanation of how to calculate the kinetic energy of a baseball, including the necessary formula and an example calculation. The concept of doubling the mass or velocity and its impact on kinetic energy is also discussed. The calculation of gravitational potential energy is explained with another example involving a baseball at a certain height, and the formula for elastic potential energy is introduced with an example involving a spring and a baseball.

Mindmap
Keywords
💡Total Energy
Total energy refers to the combined energy of all forms within a system, including kinetic, potential, and internal energy. It is a fundamental concept in physics that illustrates the conservation of energy, meaning the total energy in an isolated system remains constant despite the transformation between different forms. In the video, the red sphere's total energy is demonstrated by its kinetic energy due to velocity, potential gravitational energy due to its position, and elastic potential energy from the stretched spring.
💡Kinetic Energy
Kinetic energy is the energy of motion, possessed by an object due to its movement. It is directly proportional to the mass of the object and the square of its velocity. In the context of the video, the red sphere has kinetic energy because it is given a certain velocity. The kinetic energy can be calculated using the formula KE = 0.5 * m * v^2, where m is the mass and v is the velocity of the object.
💡Potential Gravitational Energy
Potential gravitational energy is the energy an object has due to its position in a gravitational field, typically related to its height above a reference point like the ground. It is calculated using the formula PE = m * g * h, where m is the mass, g is the acceleration due to gravity, and h is the height. In the video, the red sphere has potential gravitational energy because of its position in the simulation, which is converted to kinetic energy when it is released.
💡Elastic Potential Energy
Elastic potential energy is the energy stored in an object when it is stretched, compressed, or deformed elastically. This type of energy is related to the deformation of materials and can be released when the object returns to its original shape. The formula for calculating elastic potential energy is PE = 0.5 * k * x^2, where k is the spring constant and x is the displacement from the equilibrium position. In the video, the stretched spring has elastic potential energy, which can be converted to kinetic energy when the system is released.
💡Internal Energy
Internal energy is the energy associated with the random, chaotic motion of particles within a system, such as molecules or atoms. It is not typically calculated in the context of AP Physics but is an important concept in thermodynamics. The video mentions internal energy as the energy of particles within an object, like the molecules in a glass of water, which move and possess energy due to their thermal motion.
💡Energy Conversion
Energy conversion refers to the process of transforming energy from one form to another within a system. The video demonstrates this concept by showing how the red sphere's elastic potential energy is converted to kinetic energy and how friction can convert energy into other forms, leading to a loss of mechanical energy. The conservation of energy principle states that energy cannot be created or destroyed, only transformed from one form to another.
💡Friction
Friction is the force that opposes the relative motion or tendency of such motion of two surfaces in contact. It plays a crucial role in energy conversion, often transforming mechanical energy into thermal energy. In the video, friction is mentioned as a factor that causes the loss of mechanical energy in the system, eventually bringing the red sphere to rest.
💡Conservation of Energy
The principle of conservation of energy states that the total amount of energy in an isolated system remains constant. Energy can neither be created nor destroyed; it can only be transformed from one form to another or transferred from one object to another. This fundamental principle is central to the discussion in the video, as it explains why the total energy of the system does not change despite the conversion between kinetic, potential, and other forms of energy.
💡Work
Work, in the context of physics, is a measure of energy transfer that occurs when a force is applied over a distance. It is calculated as the product of the force applied and the displacement in the direction of the force. In the video, work is related to the concept of energy, as an object with energy has the potential to do work. The example given is a car with kinetic energy that can apply a force over a distance, demonstrating its ability to do work.
💡Gravitational Field Strength
Gravitational field strength, often denoted by 'g', is the acceleration due to gravity at a particular location, typically near the Earth's surface. It is a constant value used in the calculation of potential gravitational energy. The video script uses the standard value of 9.8 m/s^2 for g when calculating the gravitational potential energy of the baseball held at head level.
💡Spring Constant
The spring constant, symbolized by 'k', is a measure of the stiffness of a spring. It is a proportionality constant that relates the force exerted by the spring to the displacement from its equilibrium position, as described by Hooke's Law (F = -k * x). In the video, the spring constant is used to calculate the elastic potential energy stored in a spring when it is compressed or stretched, with a given value of 120 N/m used for the example.
Highlights

Total energy of a system includes kinetic, gravitational potential, and elastic potential energy.

Kinetic energy is the energy of motion and can be converted to other forms of energy.

Gravitational potential energy is due to an object's position in a gravitational field.

Elastic potential energy is stored in a spring when it is stretched or compressed.

Friction and other forces can convert energy from one form to another, affecting the total energy of a system.

In a perfect system without friction, energy would be conserved and could theoretically last forever.

Internal energy refers to the energy of particles within an object as they move around.

AP Physics expects students to calculate kinetic and potential energy, not internal energy.

Kinetic energy can be calculated using the formula KE = 0.5 * m * v^2.

Doubling the mass of an object doubles its kinetic energy; doubling the velocity quadruples it.

Rotational kinetic energy is different from translational and involves the object's mass, rotational inertia, and angular velocity.

Gravitational potential energy is calculated with the formula m * g * h.

Elastic potential energy is calculated using the formula 0.5 * k * x^2, where k is the spring constant and x is the displacement.

Energy conservation is key in understanding the dynamics of physical systems.

Predicting changes in a system's total energy involves considering position changes and frictional interactions.

Understanding energy calculations is crucial for practical applications in physics.

Transcripts

Browse More Related Video

Kinetic and Potential Energy

High School Physics - Types of Energy

Kinetic Energy

Elastic and Inelastic Collisions

Potential and Kinetic Energy with Practice Problems

AP Physics 8-5 Potential Energy Curves

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Energy ConservationPhysics ConceptsKinetic EnergyPotential EnergyMechanical EnergySimulation AnalysisFrictional ForcesWork and EnergyAP PhysicsEducational Content