Tensor Calculus 22: Riemann Curvature Tensor Geometric Meaning (Holonomy + Geodesic Deviation)
TLDRThis video delves into the Riemann curvature tensor, a mathematical instrument for gauging the curvature of space. It explains the tensor's derivation and geometric significance, emphasizing its importance beyond visual intuition. The script discusses the limitations of connection coefficients in detecting curvature and introduces the concept of parallel transport to define curvature through vector twisting. It also covers the tensor's linearity and its role in geodesic deviation, offering a deeper understanding of space's intrinsic properties.
Takeaways
- 📚 The Riemann curvature tensor is a mathematical tool used to determine if a space is curved or flat.
- 🔍 The script assumes prior knowledge of covariant derivatives and Lie brackets, which are foundational for understanding the Riemann tensor.
- 📉 The Riemann tensor is derived abstractly, with a focus on its geometric meaning rather than specific components or symmetries, which are covered in a subsequent video.
- 🌐 The concept of 'flat space' is explored, with the metric tensor and connection coefficients playing crucial roles in its definition.
- 📏 The script explains that a space is considered flat if, in a certain coordinate system, the connection coefficients are zero, although this is not a definitive test for flatness.
- 🧭 Riemann normal coordinates or local inertial frames are introduced as a special coordinate system where the metric tensor is the identity matrix and connection coefficients are zero at a specific point.
- 🔄 The script describes the process of parallel transport of vectors and how it can be used to detect curvature through the holonomy, or twisting, of vectors around a loop.
- 📐 A formula for the Riemann curvature tensor is developed, illustrating how it measures the change in a vector field when parallel transported around a small parallelogram.
- 🔢 The Riemann tensor is shown to be linear in all of its inputs, which is important for its role as a tensor in differential geometry.
- 🌍 Geodesic deviation is introduced as another method to detect space curvature, where the rate of change of the separation vector between geodesics indicates flatness or curvature.
- 📘 The video script concludes with an overview of the Riemann tensor's role in detecting curvature and a teaser for the next video, which will delve into the tensor's components and symmetries.
Q & A
What is the Riemann curvature tensor?
-The Riemann curvature tensor is a mathematical tool used to determine whether a space is curved or flat. It is a once contravariant and three times covariant tensor that measures the amount of curvature in a space by examining the change in a vector as it is parallel transported around a small parallelogram formed by two vector fields.
Why can't we rely solely on the covariant derivative and the Lie bracket to understand space curvature?
-While the covariant derivative and the Lie bracket are important for understanding the behavior of vectors in a space, they are not sufficient to determine curvature. The covariant derivative measures changes in vectors along a path, and the Lie bracket measures the failure of two vector fields to commute, but neither directly quantifies the curvature of the space itself.
What is the significance of the metric tensor in determining space curvature?
-The metric tensor measures lengths and angles in a coordinate system. It is used in conjunction with the connection coefficients to determine whether a space is curved or flat. However, the metric tensor alone is not enough to detect curvature; it must be used with other tools like the Riemann curvature tensor.
What are Riemann normal coordinates or local inertial frames?
-Riemann normal coordinates or local inertial frames are special coordinate systems at a point where the metric tensor is the identity matrix and the connection coefficients are all zero. They provide a local reference frame where the space appears flat at that specific point, but this does not necessarily mean the entire space is flat.
How does the Riemann curvature tensor relate to the concept of parallel transport?
-The Riemann curvature tensor is derived from the concept of parallel transport. It measures the change in a vector as it is parallel transported around a small parallelogram. If the vector returns to its original state after parallel transport, the space is flat; if it does not, the space is curved.
What is the geometric meaning of the Riemann curvature tensor?
-The geometric meaning of the Riemann curvature tensor is related to the holonomy or the twisting of a vector when it is transported around a loop in the space. If the vector ends up pointing in a different direction after the transport, it indicates that the space is curved.
How is the Riemann curvature tensor used to detect geodesic deviation?
-Geodesic deviation refers to the change in the separation vector between nearby geodesics. The Riemann curvature tensor is used to compute the second covariant derivative of this separation vector. If this derivative is nonzero, it indicates that the geodesics are accelerating relative to each other, suggesting a curved space.
What is the role of the connection coefficients in the context of the Riemann curvature tensor?
-Connection coefficients are used in the calculation of the Riemann curvature tensor. They are involved in the process of parallel transport and are calculated using the derivatives of the metric tensor. The connection coefficients being zero in a specific coordinate system does not necessarily imply that the space is flat; the Riemann curvature tensor provides a more definitive measure of curvature.
Why is it necessary to consider the limit as the size of the parallelogram approaches zero in the definition of the Riemann curvature tensor?
-Taking the limit as the size of the parallelogram approaches zero allows us to focus on the curvature information at a single point rather than across a finite area. This provides a more precise measure of the space's curvature at that point.
How does the linearity of the Riemann curvature tensor in its inputs affect its computation?
-The linearity of the Riemann curvature tensor in its inputs means that the tensor's action on any vector combination can be computed by knowing its action on a basis of vectors. This simplifies the computation because it reduces the problem to evaluating the tensor on a set of basis vectors.
Outlines
📚 Introduction to the Riemann Curvature Tensor
This paragraph introduces the Riemann curvature tensor, a fundamental concept in differential geometry used to determine the curvature of a space. The video aims to derive the tensor's abstract formula and explain its geometric significance. It presupposes the viewer's understanding of the covariant derivative and the Lie bracket, and references previous videos for further information. The script also discusses the limitations of using the connection coefficients to define flatness, highlighting the need for a more robust mathematical tool like the Riemann tensor, which can detect curvature through helanome and geodesic deviation.
🔍 Geometric Interpretation of Curvature via Parallel Transport
The second paragraph delves into the concept of parallel transport, which is a method to move vectors along a surface in a way that keeps them as unchanged as possible. It uses the analogy of transporting a vector from the North Pole to the equator on a sphere and back to illustrate how the vector's direction changes due to the curvature of the space. This change in direction, known as holonomy, is a key indicator of curvature. The paragraph then sets up a mathematical framework to quantify this change using the Riemann curvature tensor, focusing on the helanome definition and its relation to parallel transport around a parallelogram formed by two vector fields.
📘 Derivation of the Riemann Curvature Tensor Formula
The third paragraph presents a detailed mathematical derivation of the Riemann curvature tensor formula. It starts by considering a small parallelogram defined by two vector fields and the parallel transport of a third vector around this parallelogram. The paragraph explains the process of taking limits and using covariant derivatives to express the change in the transported vector. It also addresses the complications that arise when the Lie bracket of the two vector fields is non-zero, necessitating an additional term in the tensor formula to account for the lack of closure in the parallelogram.
🔗 Linearity and Tensor Properties of the Riemann Curvature Tensor
This paragraph discusses the tensorial properties of the Riemann curvature tensor, emphasizing its linearity in all of its inputs. The script provides a mathematical proof to show that the tensor behaves linearly when the input vectors are scaled or when the input vectors are combined linearly. This property is crucial because it ensures that the Riemann curvature tensor is a geometric object that is invariant under coordinate transformations, making it a reliable tool for analyzing space curvature.
🌐 Riemann Normal Coordinates and Geodesic Deviation
The fifth paragraph introduces Riemann normal coordinates, a special coordinate system where the metric tensor is the identity matrix and all connection coefficients are zero at a specific point. It also explores the concept of geodesic deviation, which is another way to detect space curvature by examining the acceleration of geodesics. The paragraph explains how the separation between geodesics can indicate whether space is flat or curved, with constant separation rates suggesting flatness and variable rates indicating curvature.
📐 Conclusion and Future Exploration of the Riemann Tensor
The final paragraph summarizes the video's exploration of the Riemann curvature tensor, its derivation, and its geometric interpretations. It mentions the next steps, which include computing the Riemann tensor components for a sphere and examining the tensor's symmetries in future videos. The script concludes by inviting viewers to support the content creator and check the description for additional resources.
Mindmap
Keywords
💡Riemann curvature tensor
💡Covariant derivative
💡Lie bracket
💡Metric tensor
💡Connection coefficients
💡Geodesic
💡Holonomy
💡Geodesic deviation
💡Riemann normal coordinates
💡Multi-linear map
Highlights
Introduction to the Riemann curvature tensor as a mathematical tool for determining if a space is curved or flat.
Assumption that the audience understands covariant derivatives and Lie brackets, with references to previous videos for further information.
Derivation of the abstract formula for the Riemann tensor and an explanation of its geometric meaning.
Discussion on the limitations of using connection coefficients to determine if a space is flat due to coordinate system dependencies.
Introduction of Riemann normal coordinates or local inertial frames where the metric tensor and connection coefficients are zero at a specific point.
Explanation of the concept of parallel transport of vectors and its relation to the curvature of space.
Geometric interpretation of the Riemann tensor through the holonomy or twisting of vectors when transported around a loop.
Development of a formula for the Riemann curvature tensor using the concept of parallel transport around a parallelogram formed by two vector fields.
Clarification on the necessity of the third term in the Riemann tensor formula when the Lie bracket is non-zero.
Demonstration of the Riemann tensor's linearity in all of its inputs, which simplifies computation to knowing its action on basis vectors.
Geodesic deviation as another method to detect space curvature through the acceleration of geodesics.
Explanation of how the second derivative of the separation vector between geodesics can indicate flat or curved space.
The significance of the Riemann tensor being zero for indicating a flat space through geodesic deviation.
Upcoming content预告 on computing the Riemann tensor components for a sphere and exploring the tensor's symmetries in the next video.
A call to action for viewers to check links in the description and consider supporting the channel.
Transcripts
Browse More Related Video
Tensor Calculus Lecture 8e: The Riemann Christoffel Tensor & Gauss's Remarkable Theorem
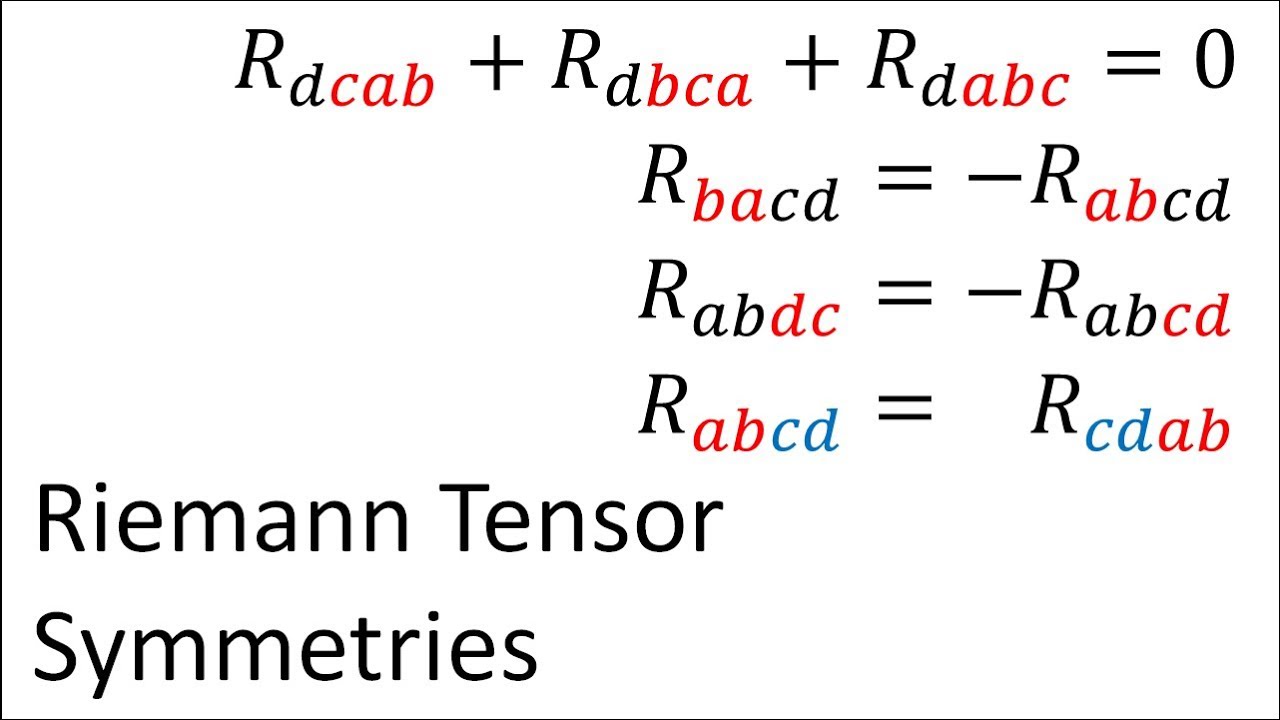
Tensor Calculus 23: Riemann Curvature Tensor Components and Symmetries
Video 82 - Riemann Christoffel Tensor
Tensor Calculus Lecture 6c: The Covariant Derivative 2

Video 93 - Geodesic Curvature Tensor
Tensor Calculus Lecture 8: Embedded Surfaces and the Curvature Tensor
5.0 / 5 (0 votes)
Thanks for rating: