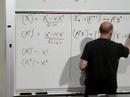International Physics Olympiad: Large Hadron Collider

ZPhysics
15 Dec 202204:32
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores the magnetic field strength required to keep protons on a circular path in the Large Hadron Collider (LHC). It corrects the classical solution of 9 Tesla and applies Einstein's special theory of relativity due to protons' near-light speed. The script derives a relativistic formula for the magnetic flux density, using the kinetic energy relation and the relativistic factor gamma. By assuming the speed of protons is nearly the speed of light, the video simplifies the formula and calculates the magnetic field strength to be approximately 5.5 Tesla, highlighting the significant difference from the classical result.

Takeaways
  • 🧲 The classical solution for the magnetic field strength is incorrect for protons in the Large Hadron Collider due to their near-light speed.
  • 🌀 Einstein's special theory of relativity is required to accurately calculate the centripetal force acting on protons moving at relativistic speeds.
  • 🎯 The relativistic factor, gamma, is crucial and is given by the equation \( \gamma = \frac{e}{mc^2} \) when the kinetic energy is negligible compared to the rest energy.
  • 🔗 The centripetal force formula is modified for relativistic speeds to \( \frac{\gamma mv^2}{R} \), which is then equated to the magnetic force \( qvB \).
  • ⚛ The charge of a proton is equal in magnitude but opposite in sign to the charge of an electron, and can be represented by \( e \).
  • 🔄 The kinetic energy equation from classical physics is not applicable in the relativistic scenario; instead, the total energy minus rest energy is used.
  • 📉 The mass-energy equivalence principle simplifies the gamma factor approximation when the proton's speed is close to the speed of light.
  • 🔄 The magnetic field \( B \) can be derived by rearranging the force equations and considering the speed of light \( c \) and the circular path's circumference \( L \).
  • 📏 The circumference of the Large Hadron Collider's ring is used to express the radius in terms of \( L/2\pi \).
  • 🔢 The actual calculation of the magnetic field strength involves plugging in the energy of the protons in TeV, converting it to joules, and using the given length of the LHC.
  • 📉 The result from the relativistic calculation shows a significant difference from the classical prediction, highlighting the importance of relativistic effects at high speeds.
Q & A

  • What is the Large Hadron Collider (LHC)?

    -The Large Hadron Collider is the world's largest and most powerful particle accelerator, designed to collide particles at extremely high speeds to study the fundamental particles of the universe.

  • Why is the classical solution for the magnetic field strength incorrect for the LHC?

    -The classical solution is incorrect because the protons in the LHC are orbiting very close to the speed of light, requiring the use of Einstein's special theory of relativity for an accurate calculation.

  • What is the relativistic factor (gamma) and why is it used?

    -The relativistic factor, denoted as gamma, accounts for the increase in mass of particles moving at relativistic speeds. It is used to calculate the correct centripetal force needed to keep the proton beam on a circular track at near-light speeds.

  • What is the relationship between the kinetic energy and the relativistic factor (gamma)?

    -In the relativistic case, the kinetic energy is given by the equation gamma - 1 times m c squared, where m is the mass of the proton, c is the speed of light, and gamma is the relativistic factor.

  • How is the charge of a proton related to the electric charge?

    -The charge of a proton is equal in magnitude but opposite in sign to the elementary charge (e), which is the fundamental unit of electric charge.

  • What is the significance of the equation e = gamma m c squared - m c squared in the context of the LHC?

    -This equation shows that the charge e of a proton can be approximated as gamma m c squared when the proton is moving close to the speed of light, which simplifies the calculation of the magnetic field needed to maintain the proton's circular path.

  • Why can the mass term (m c squared) be neglected in the approximation for gamma?

    -The mass term can be neglected because when the proton is moving at speeds close to the speed of light, the product of the mass of the proton and the speed of light squared becomes negligible compared to the kinetic energy.

  • How is the magnetic field strength related to the speed of the proton and the radius of the LHC's circular track?

    -The magnetic field strength is derived from the equation e/(c^2) * (v/c) = B, where v is the speed of the proton, c is the speed of light, and B is the magnetic field strength. Since v is approximately equal to c, the equation simplifies to B ≈ e/(c^2).

  • What is the significance of the circumference of the LHC's circular track in the calculation of the magnetic field strength?

    -The circumference of the LHC's track is used to relate the magnetic field strength to the radius and to simplify the expression for B in terms of the track's length (L) and the fundamental constants.

  • How does the energy of the proton beam in the LHC affect the required magnetic field strength?

    -The energy of the proton beam, given in tera electron volts (TeV), is a key factor in determining the required magnetic field strength to maintain the beam's circular path. Higher energy beams require stronger magnetic fields.

  • What is the difference between the classical and relativistic results for the magnetic field strength in the LHC?

    -The classical result underestimates the magnetic field strength needed because it does not account for the relativistic effects at near-light speeds. The relativistic calculation provides a more accurate value, which is approximately 5.5 Tesla for the given conditions.

Outlines
00:00
🔬 Magnetic Field Strength in the Large Hadron Collider

This paragraph discusses the calculation of the magnetic field required to keep protons on a circular path in the Large Hadron Collider (LHC). It clarifies that the classical solution of 9 Tesla is incorrect due to the protons traveling near the speed of light, necessitating the use of Einstein's special theory of relativity. The paragraph explains the relativistic formula for centripetal force, introduces the relativistic factor gamma, and sets it equal to the magnetic force qvB. It also highlights the trick of simplifying the kinetic energy equation using the relativistic approximation, leading to an expression for the magnetic field B in terms of the LHC's circumference and the energy of the protons.

Mindmap
Keywords
💡Magnetic Field
A magnetic field is a vector field that describes the magnetic influence on moving electric charges, electric currents, and magnetic materials. In the context of the video, the strength of the magnetic field is crucial for keeping protons on a circular track within the Large Hadron Collider (LHC). The script mentions that the classical prediction of a magnetic field is 'no point North 9 Tesla,' which is incorrect due to the relativistic speeds of the protons.
💡Large Hadron Collider (LHC)
The LHC is the world's largest and most powerful particle accelerator, designed to test the predictions of different theories of high-energy physics and to increase our understanding of the smallest building blocks of the universe. The video discusses the LHC's role in maintaining a proton beam on a circular path using a magnetic field, emphasizing the need for a relativistic approach to calculate the necessary field strength.
💡Uniform Magnetic Flux Density
Uniform magnetic flux density refers to the constant magnetic field strength throughout a given region. The script asks to derive an expression for this density to keep the proton beam on a circular track, which is a fundamental concept in understanding how particles are controlled within the LHC.
💡Einstein's Special Theory of Relativity
This theory, proposed by Albert Einstein, addresses the behavior of objects in high-velocity motion, particularly as they approach the speed of light. In the script, it is mentioned that relativistic effects must be considered because the protons in the LHC are orbiting very close to the speed of light, affecting the calculations for the magnetic field required.
💡Relativistic Factor (Gamma)
The relativistic factor, often denoted as 'gamma,' is a dimensionless quantity that relates the observed time interval in a moving frame to that in the rest frame. In the script, gamma is used to calculate the centripetal force required to keep the protons in a circular path at near-light speeds, as opposed to the classical formula which does not account for relativistic effects.
💡Centripetal Force
Centripetal force is the inward force required to keep an object moving in a circular path. The script explains that in the classical solution, the centripetal force is calculated as 'm v^2 / R,' but this must be modified with the gamma factor for relativistic speeds in the LHC.
💡Charge of a Proton
The charge of a proton is a fundamental property of the particle, which is equal in magnitude but opposite in sign to the charge of an electron. In the script, it is mentioned that the force on the protons can be expressed as 'e v B,' where 'e' is the charge of the proton and 'B' is the magnetic field.
💡Kinetic Energy
Kinetic energy is the energy that an object possesses due to its motion. The script contrasts the classical kinetic energy equation ('1/2 m v^2') with the relativistic case, where the kinetic energy is expressed as '(gamma - 1) m c^2,' accounting for the increase in mass at high speeds.
💡Elementary Charge
The elementary charge is the electric charge carried by a single proton or the same magnitude but opposite in sign for an electron. In the script, the conversion of energy from electron volts to joules involves the elementary charge, which is a fundamental constant in physics.
💡Circumference
Circumference is the total distance around a circle or circular path. The script uses the circumference of the LHC ring to express the magnetic field strength in terms of the length of the track, rather than the radius, to simplify the calculation.
💡Tera Electron Volts
Tera electron volts (TeV) is a unit of energy commonly used in particle physics to describe the energy of particles. The script mentions an energy of '7 TeV' for the protons in the LHC, which is a significant amount that requires a strong magnetic field to maintain their circular path.
Highlights

The classical solution for magnetic field strength is incorrect for protons orbiting near the speed of light in the Large Hadron Collider.

Einstein's special theory of relativity is required to accurately calculate the centripetal force on protons in the LHC.

The relativistic factor gamma is introduced to account for the high velocities of protons.

The force on protons is expressed as qvB, where q is the charge and v is the velocity.

The charge of a proton is equivalent but opposite to that of an electron.

A trick is used to simplify the equation by approximating the kinetic energy in the relativistic case.

The gamma factor is derived from the kinetic energy equation, considering the mass-energy equivalence.

The approximation simplifies the equation by neglecting the mass-energy term when protons are moving close to the speed of light.

The magnetic field B is calculated using the simplified equation involving the velocity of light and the circumference of the LHC.

The speed of the protons is assumed to be approximately equal to the speed of light for simplification.

The circumference of the LHC is used to express the radius and simplify the magnetic field equation.

The magnetic flux density is calculated by substituting the energy of 7 TeV into the derived equation.

Conversion from electron volts to joules is necessary for the calculation, involving the elementary charge.

The length of the LHC is provided and used in the calculation of the magnetic flux density.

The calculated magnetic field strength is 5.5 Tesla, contrasting with the classical result.

The significance of the relativistic result over the classical one is highlighted in the context of the LHC.

The physics Olympiad problem and its solution are presented as an engaging and educational derivation.

Transcripts

Browse More Related Video

Magnetic flux explained in 3 minutes

Lecture 7 | Quantum Entanglements, Part 3 (Stanford)

Magnetic (AP Physics SuperCram Review)

Why moving charges produce magnetic field?

Magnetic Flux and Magnetic Flux Density

Special Relativity | Lecture 1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Magnetic FieldsRelativityProton BeamsPhysics OlympiadEinstein TheorySpecial RelativityCentrifugal ForceMagnetic Flux DensityParticle AccelerationScientific Derivation