Magnetic flux explained in 3 minutes

PhysicsHigh
12 Jan 202303:22
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, Paul from Physics High explains the concept of magnetic flux, which is the measure of the magnetic field in a specific area. He uses a visual representation to demonstrate how the number of magnetic field lines passing through a loop changes with the loop's size and orientation. Paul also covers how to calculate magnetic flux mathematically, introducing the formula Φ = B * A * cos(θ), where Φ is the magnetic flux, B is the magnetic field strength, A is the area perpendicular to the field, and θ is the angle between the normal to the surface and the magnetic field. The unit for magnetic flux is the Weber (Wb), equated to Tesla meters squared.

Takeaways
  • 🧲 Magnetic flux is a measure of the magnetic field in a specific area, represented by the symbol B.
  • 🔍 The strength of the magnetic field can be visualized by the number of magnetic field lines passing through a given area.
  • 🔄 The magnetic flux can be altered by changing the area or the orientation of the loop within the magnetic field.
  • 📏 Reducing the area of the loop decreases the number of magnetic field lines passing through, thus reducing the flux.
  • 🔄 Rotating the loop can also reduce the number of field lines passing through, reaching zero when perpendicular to the field.
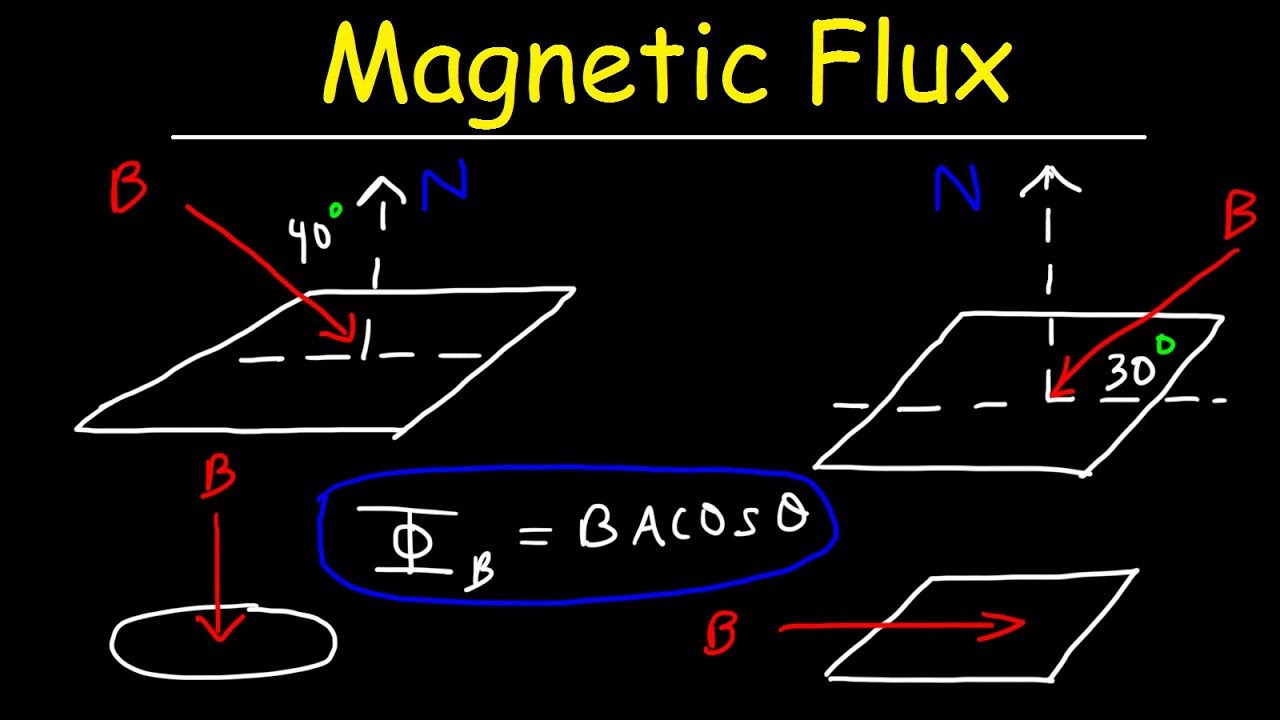
  • 📚 The mathematical expression for magnetic flux, denoted by Φ, involves the product of the magnetic field strength, the area, and the cosine of the angle (θ) between the field and the area.
  • 📐 The area considered in the calculation of magnetic flux is only the component that is parallel to the magnetic field.
  • 📈 The cosine function determines the contribution of the area to the flux, being 1 when parallel (θ=0) and 0 when perpendicular (θ=90°).
  • 🔢 The unit of magnetic flux is the Weber (Wb), which is equivalent to Tesla meters squared (Tm²).
  • 👋 The script is part of a quick review series by Paul from Physics High, who encourages viewers to subscribe, share, and support his content.
  • ☕️ The video includes a call to action for viewers to support the creator by buying him a coffee through a provided link.
Q & A

  • What is magnetic flux?

    -Magnetic flux is the measurement of the magnetic field in a particular area, represented by the symbol B, and it quantifies the number of magnetic field lines passing through a given area.

  • How can you visualize magnetic flux?

    -Magnetic flux can be visualized as magnetic field lines entering or leaving a surface area, with the number of lines indicating the strength of the magnetic field in that area.

  • What factors can change the magnetic flux through a loop?

    -The magnetic flux can be changed by altering the area of the loop, the strength of the magnetic field, or the orientation of the loop relative to the magnetic field.

  • What happens to the magnetic flux when the loop is rotated?

    -When the loop is rotated, the number of magnetic field lines passing through it can decrease, potentially reaching zero if the loop is perpendicular to the magnetic field lines.

  • What is the mathematical formula for calculating magnetic flux?

    -The mathematical formula for magnetic flux is Φ = B * A * cos(θ), where Φ is the magnetic flux, B is the magnetic field strength, A is the area perpendicular to the magnetic field, and θ is the angle between the normal to the area and the direction of the magnetic field.

  • What is the unit of measurement for magnetic flux?

    -The unit of measurement for magnetic flux is the Weber (Wb), which is equivalent to one Tesla meter squared.

  • How does the direction of the loop affect the magnetic flux?

    -The direction of the loop, represented by the normal vector, affects the magnetic flux because only the component of the area that is parallel to the magnetic field contributes to the flux.

  • What is the relationship between the angle θ and the magnetic flux?

    -The relationship between the angle θ and the magnetic flux is given by the cosine function in the formula Φ = B * A * cos(θ). When θ is 0 degrees, cos(θ) is 1, and the magnetic flux is at its maximum. When θ is 90 degrees, cos(θ) is 0, and the magnetic flux is zero.

  • What does it mean when the magnetic flux is zero?

    -A magnetic flux of zero indicates that there are no magnetic field lines passing through the loop or surface area, which can occur when the loop is oriented perpendicular to the magnetic field lines.

  • Who is the presenter of the video and what is the purpose of the series?

    -The presenter of the video is Paul from Physics High, and the purpose of the series is to provide quick reviews on various physics topics, including the calculation of magnetic flux.

Outlines
00:00
🧲 Understanding Magnetic Flux

This paragraph introduces the concept of magnetic flux as part of a quick review series. The speaker explains that magnetic flux is a measure of the magnetic field in a specific area, represented by the symbol B. They use a visual representation to illustrate how the number of magnetic field lines passing through a loop can indicate the flux. The speaker then discusses how changing the area or the orientation of the loop can affect the number of field lines passing through, and hence the magnetic flux. The paragraph concludes with a brief introduction to the mathematical representation of magnetic flux, involving the normal to the surface area and the direction of the magnetic field.

Mindmap
Keywords
💡Magnetic Flux
Magnetic flux is a measure of the total magnetic field that passes through a given area. It is a fundamental concept in electromagnetism, denoted by the symbol 'Φ' (Phi). In the video, the concept is introduced as the measurement of magnetic field lines in a particular area, with the example of a loop through which magnetic field lines pass, illustrating how the number of lines can change the flux.
💡Magnetic Field
The magnetic field, represented by the symbol 'B', is a vector field that describes the magnetic influence on moving electric charges, magnetic materials, and other magnetic fields. The script uses the concept to explain how the strength and direction of the magnetic field affect the magnetic flux through a given area.
💡Magnetic Field Lines
Magnetic field lines are a visual representation of the direction and strength of a magnetic field. In the script, the presenter uses these lines to demonstrate how the magnetic flux changes with the number of lines passing through a loop, showing that the flux is directly related to the number of these lines.
💡Area
In the context of magnetic flux, 'area' refers to the surface through which the magnetic field lines pass. The script explains that the amount of flux can be altered by changing the area of the loop, which in turn changes the number of magnetic field lines passing through it.
💡Flux Density
Flux density, or magnetic field strength, is the measure of the magnitude of the magnetic field at a particular point. The script mentions that the flux is calculated by multiplying the flux density by the area of the loop that is parallel to the magnetic field.
💡Loop
In the script, a 'loop' is a physical representation used to demonstrate the concept of magnetic flux. The number of magnetic field lines passing through the loop changes as the area or orientation of the loop changes, affecting the magnetic flux.
💡Rotation
Rotation is the act of turning an object around an axis. In the video, the presenter rotates the loop to show how the orientation of the loop affects the number of magnetic field lines passing through it, and consequently, the magnetic flux.
💡Normal
The 'normal' is a vector that is perpendicular to a surface. The script explains that the direction of the normal to the surface of the loop is important in determining the component of the area that contributes to the magnetic flux.
💡Cosine Theta
Cosine Theta (cos θ) is a trigonometric function used in the formula for magnetic flux to account for the angle between the magnetic field and the normal to the surface area. The script uses the example of a loop rotated to be perpendicular to the magnetic field, where cos 90° equals zero, indicating no flux.
💡Weber
The Weber (Wb) is the unit of measurement for magnetic flux in the International System of Units (SI). The script mentions that one Weber is equivalent to one Tesla meter squared, providing a concrete measure for the magnetic flux discussed.
💡Tesla
The Tesla is the SI derived unit for measuring magnetic flux density or magnetic induction. It is used in the script to describe the strength of the magnetic field and is part of the unit for measuring magnetic flux (Weber).
Highlights

Magnetic flux is the measurement of the magnetic field in a particular area.

The symbol B is used to represent the magnetic field strength.

Magnetic flux can be visualized by the number of magnetic field lines passing through a loop.

Changing the area of the loop can alter the magnetic flux.

Rotation of the loop can reduce the number of magnetic field lines passing through, affecting the flux.

Magnetic flux can be zero when the loop is positioned such that no magnetic field lines pass through it.

The direction of the loop is important and is represented by a normal vector.

The mathematical formula for magnetic flux involves the magnetic field strength, area, and the cosine of the angle between them.

The Greek letter Phi (Φ) is used to denote magnetic flux.

Only the component of the area parallel to the magnetic field contributes to the magnetic flux.

The unit for magnetic flux is the Weber (Wb), equivalent to Tesla meters squared.

The video is part of a quick review series on physics concepts.

The speaker encourages viewers to subscribe and support the channel.

A coffee support link is provided in the video description for viewers to contribute.

The video features a visual representation of magnetic field lines and their interaction with a loop.

The impact of the loop's orientation on the calculation of magnetic flux is discussed.

The video concludes with a reminder to like, share, and subscribe for more physics content.

Transcripts

Browse More Related Video

Magnetic Flux, Basic Introduction - Physics Problems

Magnetic Flux and Magnetic Flux Density

Magnetic Torque | Physics with Professor Matt Anderson | M23-08

Magnetic (AP Physics SuperCram Review)

Electromagnetic Induction

Magnetic Flux | Physics with Professor Matt Anderson | M24-01

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Magnetic FluxPhysicsEducationalCalculationMagnetic FieldWeberTeslaMeters SquaredPaulPhysics HighSubscription