Single Slit Interference | Physics with Professor Matt Anderson | M28-19

Physics with Professor Matt Anderson

16 Mar 202206:07

EducationalLearning

32 Likes 10 Comments

TLDRIn this educational video, the concept of single-slit diffraction is explored through a problem-solving approach. The script discusses how to determine the wavelength of light using the formula for dark fringes in a diffraction pattern. With a given slit width of 11 microns and the first-order dark fringe at 4.31 degrees, the formula d * sin(θ) = m * λ is applied, where d is the slit width, θ is the angle, m is the order of the fringe (1 for the first dark fringe), and λ is the wavelength. The calculation leads to an approximate wavelength of 826 nanometers, aligning with the given answer of 827 nanometers.

Takeaways

🔬 The problem involves calculating the wavelength of light using a single-slit diffraction pattern.
📏 The width of the slit (d) is given as 11 microns, which is equivalent to 11 * 10^-6 meters.
📐 The angle (theta) to the first dark fringe from the center line is 4.31 degrees.
🌀 The formula for the dark fringe in single-slit diffraction is the same as for the bright fringe in double-slit diffraction: d * sin(theta) = m * lambda.
📘 The formula sheet confirms that the equation d * sin(theta) = m * lambda applies to both scenarios.
🔢 The integer m represents the order of the fringe, and for the first dark fringe, m is equal to 1.
🧮 To find the wavelength (lambda), rearrange the formula to lambda = d * sin(theta) / m.
📲 Use a calculator to compute the sine of the angle and divide the product of the slit width and sine by m.
📉 The initial calculation yields a wavelength in meters, which needs to be converted to nanometers.
🔍 Convert meters to nanometers by moving the decimal point two places to the right and adjusting the exponent accordingly.
🎯 The calculated wavelength is approximately 826 nanometers, close to the provided answer of 827 nanometers.

Q & A

What is the width of the slit used in the single-slit diffraction experiment described in the transcript?
-The width of the slit, denoted as 'd', is 11 microns, which is equivalent to 11 times 10 to the power of negative 6 meters.
What is the angle of the first order dark fringe from the center line in the diffraction pattern?
-The angle of the first order dark fringe from the center line is given as 4.31 degrees.
What formula is used to relate the width of the slit, the angle of the fringe, and the wavelength of light in single-slit diffraction?
-The formula used is d * sin(θ) = m * λ, where d is the width of the slit, θ is the angle of the fringe, m is an integer representing the order of the fringe, and λ is the wavelength of light.
What is the significance of the integer 'm' in the formula for single-slit diffraction?
-The integer 'm' represents the order of the fringe in the diffraction pattern. For the first dark fringe, m is equal to 1.
How does the formula for bright fringes in a double-slit experiment compare to the formula for dark fringes in a single-slit experiment?
-Surprisingly, the formula for bright fringes in a double-slit experiment (d * sin(θ) = m * λ) is the same as that for dark fringes in a single-slit experiment, with 'm' being the order of the fringe.
What is the calculated wavelength of the light if the first dark fringe is at an angle of 4.31 degrees and the slit width is 11 microns?
-The calculated wavelength of the light is approximately 8.26 times 10 to the power of negative 7 meters, which converts to 826 nanometers.
How does the wavelength calculated from the single-slit diffraction experiment compare to the provided answer choices?
-The calculated wavelength of 826 nanometers is closest to answer choice A, which is 827 nanometers.
Why is it important to refer to the formula sheet when solving physics problems like this one?
-Referring to the formula sheet ensures that you are using the correct formula for the specific type of problem, avoiding confusion between similar but distinct physical phenomena.
What is the unit conversion that needs to be done to match the calculated wavelength with the answer choices?
-The calculated wavelength in meters needs to be converted to nanometers by moving the decimal point two places to the right, resulting in a unit of nanometers.
What is the role of the sine function in the formula for single-slit diffraction?
-The sine function relates the angle of the diffracted light to the width of the slit and the wavelength of the light, indicating the position of the fringes in the diffraction pattern.
What insight does this problem provide about the relationship between physical phenomena and mathematical representation?
-This problem illustrates how the same mathematical formula can describe different physical phenomena, such as bright fringes in a double-slit experiment and dark fringes in a single-slit experiment, highlighting the universality of mathematical expressions in physics.

Outlines

00:00

🔬 Single Slit Diffraction and Wavelength Calculation

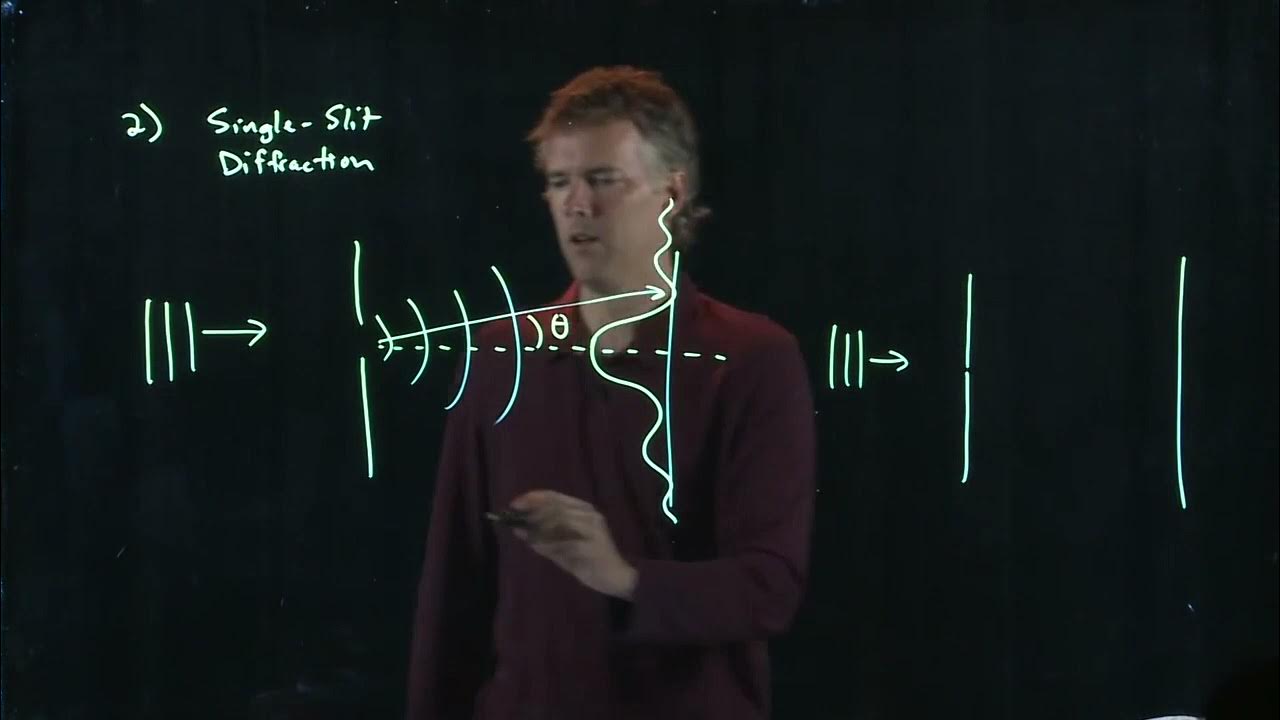
This paragraph discusses a physics problem involving single-slit diffraction of monochromatic light. The scenario describes a beam of light passing through a 11-micron-wide slit and the formation of a diffraction pattern with a first-order dark fringe at an angle of 4.31 degrees from the centerline. The goal is to calculate the wavelength of the light. The instructor guides the students through the process, clarifying the use of the formula d * sin(θ) = m * λ, where d is the slit width, θ is the angle to the dark fringe, m is the order of the fringe, and λ is the wavelength. The students are encouraged to refer to the formula sheet for the correct equation, which is indeed the same as that for bright fringes in a double-slit experiment. The summary ends with the formula to solve for the wavelength, λ = d * sin(θ) / m, with the values for d and θ provided, and m assumed to be 1 for the first dark fringe.

05:01

📏 Calculation of Wavelength with Given Data

The second paragraph continues the physics problem-solving process, focusing on the calculation of the wavelength of light using the previously mentioned formula. The instructor provides the value for the slit width (d = 11 microns or 11 * 10^-6 meters) and the angle to the first dark fringe (θ = 4.31 degrees). The order of the fringe (m) is identified as 1, corresponding to the first dark fringe. The students are instructed to use a calculator to find the sine of the angle and then apply the formula to solve for the wavelength, λ. The calculated result is 8.26 * 10^-7 meters, which is then converted to nanometers, yielding 826 nanometers. The instructor notes a discrepancy with the provided answers, suggesting a possible error in the calculation or the answers, and ends with a note to check the calculation after the decimal point.

Mindmap

Keywords

💡Monochromatic Light

Monochromatic light refers to light consisting of a single wavelength, which is necessary for certain optical experiments because it produces a consistent and predictable pattern. In the video, the use of monochromatic light is crucial for the single-slit diffraction experiment, as it allows for the observation of a clear diffraction pattern with distinct fringes.

💡Slit Width

The slit width, denoted as 'd' in the script, is the physical dimension of the opening through which the light passes in a diffraction experiment. It is a critical parameter because it affects the spacing and intensity of the resulting diffraction pattern. In the context of the video, the slit width is given as 11 microns, which is a key piece of information for calculating the wavelength of the light.

💡Diffraction Pattern

A diffraction pattern is the distribution of light intensity as it bends around an obstacle or through an aperture. It is the observable outcome of wave interference and is central to understanding wave behavior in physics. In the video, the diffraction pattern is produced by the monochromatic light passing through a single slit and is analyzed to determine the wavelength of the light.

💡First Order Dark Fringe

The first order dark fringe in a diffraction pattern is the first location where no light is observable, occurring at a specific angle from the central bright fringe. It is significant in the video because the angle at which this dark fringe appears is used to calculate the wavelength of the light, with the formula involving the sine of this angle.

💡Wavelength

Wavelength is the distance between two consecutive points in a wave that are in the same phase, such as from crest to crest or trough to trough. It is a fundamental property of light and is the quantity being sought in the video's experiment. The script describes a method to calculate the wavelength of light based on the dimensions of the slit and the angle of the dark fringe.

💡Single Slit Diffraction

Single slit diffraction is a phenomenon that occurs when light passes through a single, narrow opening and spreads out into a pattern of bright and dark fringes on a screen. The video discusses this concept as it is the basis for the experiment being analyzed, where the properties of light are deduced from the observed pattern.

💡Bright Fringe

A bright fringe in a diffraction pattern is a region of increased light intensity, typically appearing as a band of light. In the context of the video, the formula for the position of bright fringes in a double-slit experiment is mentioned, which is similar to that used for dark fringes in single-slit diffraction, highlighting the interconnectedness of optical phenomena.

💡Formula Sheet

The formula sheet mentioned in the video is a reference guide that students are encouraged to consult during exams. It contains key equations and formulas relevant to the subject, such as the relationship between slit width, angle, and wavelength in diffraction patterns, which is essential for solving the problem presented in the video.

💡Sine Function

The sine function is a trigonometric function that relates the angle of a right triangle to the ratio of the length of the side opposite the angle to the length of the hypotenuse. In the video, the sine function is used in the formula to calculate the wavelength of light from the angle of the dark fringe and the width of the slit.

💡Units Conversion

Units conversion is the process of changing a physical quantity from one unit of measurement to another. In the script, the calculated wavelength is initially in meters, which is then converted to nanometers to match the units provided in the answer choices. This step is crucial for ensuring the answer is presented in the correct format.

💡Optical Experiment

An optical experiment is a scientific procedure that investigates the behavior of light, often involving the manipulation of light through various apparatus to observe phenomena such as refraction, reflection, or diffraction. The video script describes a specific optical experiment involving a single-slit diffraction setup to determine the wavelength of light.

Highlights

The problem involves calculating the wavelength of light using single-slit diffraction.

A monochromatic light beam passes through a 11-micron wide slit.

The first-order dark fringe in the diffraction pattern is at 4.31 degrees from the center line.

The slit width is given as d = 11 microns or 11 x 10^-6 meters.

The angle to the first dark fringe is θ = 4.31 degrees.

The formula d * sin(θ) = m * λ is used to calculate the wavelength λ.

The formula is the same for bright fringes in double-slit diffraction and dark fringes in single-slit diffraction.

The integer m represents the order of the fringe, with m=1 for the first dark fringe.

Solving for λ gives λ = (d * sin(θ)) / m.

Substituting the given values: λ = (11 x 10^-6 * sin(4.31)) / 1.

Calculating λ results in 8.26 x 10^-7 meters.

Converting to nanometers gives λ = 826 nm.

The closest answer option is 827 nm.

The problem demonstrates the application of single-slit diffraction formula in calculating wavelengths.

The example illustrates the process of solving physics problems using given formulas and values.

The importance of referring to formula sheets during exams is emphasized.

The solution involves unit conversion from meters to nanometers.

The problem highlights the connection between double-slit and single-slit diffraction equations.

Transcripts

Browse More Related Video

Two Slit Interference | Physics with Professor Matt Anderson | M28-18

Physics - Diffraction of Light (1 of 4) The Thin Slit

Physics 60 Interference of Light (4 of 8) Young's Double Slit

Resolving Power of a Telescope | Physics with Professor Matt Anderson | M28-14

Single Slit Diffraction | Physics with Professor Matt Anderson | M28-16

Wavelength-Frequency equation

Single Slit Interference | Physics with Professor Matt Anderson | M28-19

Takeaways

Q & A

What is the width of the slit used in the single-slit diffraction experiment described in the transcript?

What is the angle of the first order dark fringe from the center line in the diffraction pattern?

What formula is used to relate the width of the slit, the angle of the fringe, and the wavelength of light in single-slit diffraction?

What is the significance of the integer 'm' in the formula for single-slit diffraction?

How does the formula for bright fringes in a double-slit experiment compare to the formula for dark fringes in a single-slit experiment?

What is the calculated wavelength of the light if the first dark fringe is at an angle of 4.31 degrees and the slit width is 11 microns?

How does the wavelength calculated from the single-slit diffraction experiment compare to the provided answer choices?

Why is it important to refer to the formula sheet when solving physics problems like this one?

What is the unit conversion that needs to be done to match the calculated wavelength with the answer choices?

What is the role of the sine function in the formula for single-slit diffraction?

What insight does this problem provide about the relationship between physical phenomena and mathematical representation?