AP Calculus AB - 1.4 Estimating Limit Values from Tables

In this segment, Mr. Bortnick delves into the topic of estimating limit values from tables within the realm of AP Calculus AB. He introduces the lesson on finding limits from tables and emphasizes the importance of using a graphing calculator or an online graphing calculator for this section. The instructor provides a step-by-step guide on how to interpret graphical and tabular data to estimate limits, using the function f(x) as an example. He visually demonstrates that the limit as x approaches 3 of f(x) is four, both from a graphical and a tabular perspective. The lesson also touches on the importance of understanding different mathematical representations, such as graphical, tabular, and algebraic, which are all interconnected and will be further explored in future lessons.

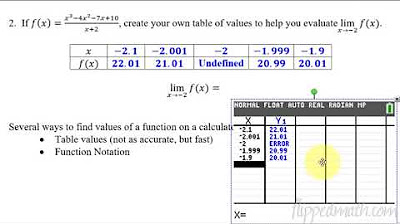
This paragraph focuses on employing advanced calculator techniques to evaluate limits, specifically when dealing with functions that may result in undefined values at certain points. Mr. Bortnick demonstrates how to input equations into a TI-84 Plus CE calculator and create a table of values to approximate limits. He highlights a 'table set' feature that allows for the input of non-integer x-values, which is crucial for estimating limits as x approaches values that make the function undefined, such as negative two in the provided example. The instructor also introduces function notation on the calculator to obtain more precise values and decimal places, which is particularly useful for closer approximations of limits.

In this part of the lesson, the concept of continuity and increasing functions is explored. The instructor discusses how to use a table of values for a function that is continuous and increasing for x greater than or equal to one. He explains the process of selecting x-values close to the point of interest (x=2 in this case) to approximate the limit of the cosine of the function as x approaches two. The approach involves observing the values from both sides of the point and inferring the limit based on the trend of the values. The instructor also provides a calculator tip, emphasizing the importance of using radian mode for accurate trigonometric calculations in AP Calculus.

The instructor wraps up the lesson by guiding students through the process of creating their own table of values to evaluate the limit of a function as x approaches a specific value. He advises on the selection of x-values that are very close to the point of interest to ensure a more accurate approximation of the limit. Mr. Bortnick also discusses the importance of rounding to three decimal places in AP Calculus, as it is a standard requirement for the AP exam. The lesson concludes with a reminder to practice the problems and to seek clarification for any doubts, fostering an environment of continuous learning and improvement.

In the final paragraph, Mr. Bortnick provides additional calculator tips, specifically for the AP Calculus exam, where the calculator should always be in radian mode. He demonstrates how to calculate the cosine of a value using the calculator and explains the process of rounding the result to three decimal places, which is a requirement for the exam. The instructor encourages students to practice the problems provided and to come to class with any questions they may have, emphasizing the importance of understanding the material and being prepared to ask for help when needed.