Calculus AB/BC – 1.5 Determining Limits Using Algebraic Properties

The Algebros

9 Sept 202009:17

EducationalLearning

32 Likes 10 Comments

TLDRIn this calculus lesson, Mr. Bean introduces the algebraic properties of limits, drawing parallels with middle school algebra. He explains how to handle limits of functions added together, emphasizing the need to identify the correct function and approach values. Examples include evaluating limits with composition and piecewise functions, and distinguishing between function values and limits. The lesson aims to prepare students for mastery checks and AP exams, highlighting the importance of understanding the difference between left and right limits and the overall concept of limit existence.

Takeaways

📚 The lesson is focused on the algebraic properties of limits in calculus.
🔢 Limits can be treated similarly to variables in algebra, such as adding two instances of a function resulting in twice the limit of that function.
📉 When dealing with limits, it's crucial to identify the function in question and the point at which the limit is being taken.
👉 The concept of limits involves understanding the behavior of a function as the input approaches a certain value, not just the value itself.
🤔 In the context of limits, one must consider whether they are approaching a value from the left, right, or both, as it affects the outcome.
📌 The script provides an example of how to handle composition of functions within limits, emphasizing the importance of starting with the inner function.
📈 The lesson includes a table of values to illustrate the difference between function values and limits, highlighting the need to distinguish between them.
📘 The script explains the process of simplifying expressions involving limits, including the subtraction of actual function values from limits.
📌 Piecewise functions are discussed, with an emphasis on taking limits from the left and right sides of a point and recognizing when the limit does not exist due to differing values.
📝 The importance of understanding logarithmic properties is highlighted, as demonstrated in an example where the natural log of e to the power of a number simplifies to the number itself.
🤓 The lesson concludes with a challenge for students to apply what they've learned about limits, including recognizing when a limit does not exist due to different left and right side values.

Q & A

What is the main topic of the calculus lesson presented by Mr. Bean?
-The main topic of the lesson is the properties of limits, specifically focusing on the algebraic properties of limits in calculus.
How does Mr. Bean relate the concept of limits to something familiar from middle school algebra?
-Mr. Bean relates the concept of limits to the idea of adding like terms in algebra, such as adding x plus x to get 2x, to help students understand the addition property of limits.
What is the basic property of limits that Mr. Bean discusses in the context of adding two limits of the same function?
-The basic property discussed is that the limit of a function f(x) plus the limit of the same function f(x) as x approaches a constant c is equal to 2 times the limit of f(x) as x approaches c.
What is the significance of the constant 'c' in the context of limits discussed in the script?
-The constant 'c' represents the value that x approaches as the limit is taken. It is an arbitrary number that helps define the behavior of the function as x gets arbitrarily close to it.
How does Mr. Bean explain the concept of limits involving different functions f and g with different approaches?
-Mr. Bean uses a table to illustrate how to evaluate limits when different functions f and g are involved and when x approaches different values, emphasizing the importance of identifying the correct function and approach value.
What is the trick mentioned by Mr. Bean when dealing with the limit as x approaches 1 in the context of f(x) and g(x)?
-The trick is to recognize that when x approaches 1, the limit of f of negative x is actually being taken, which means considering the behavior of the function as it gets close to negative one, not one.
Can you explain the composition of functions in limits as presented in the second example of the script?
-In the second example, the composition of functions in limits is explained by starting with the inner function as x approaches a certain value, and then using that value as the input for the outer function to find the limit.
What is the difference between the actual function value and the limit value as illustrated in the table of values in the script?
-The actual function value is the value of the function at a specific point, while the limit value is the value that the function approaches as the input gets arbitrarily close to a certain point, which may or may not be the same as the actual function value at that point.
How does Mr. Bean handle the subtraction of an actual function value from a limit in the script's last example?
-Mr. Bean underlines the limit expression and then subtracts the actual value of the function at a specific point (h(5) in the example) from the evaluated limit expression to find the final result.
What is the concept of piecewise functions in limits as discussed in the script?
-Piecewise functions have different expressions for different intervals of the input variable. When taking a limit, one must consider whether they are approaching the point of interest from the left, right, or both, and use the appropriate piece of the function to evaluate the limit.
Why does the limit not exist in the case of the piecewise function as x approaches -5 in the script?
-The limit does not exist because the left and right limits yield different values. For the limit to exist, both the left and right limits must be equal and approach the same value.

Outlines

00:00

📚 Introduction to Algebraic Properties of Limits

This paragraph introduces the topic of algebraic properties of limits in calculus. Mr. Bean explains that understanding these properties is similar to recalling basic algebraic principles from middle school. The lesson focuses on how to handle limits of functions as they approach a constant value, using the analogy of adding 'x's together. The concept is illustrated with the example of adding two limits of the same function, resulting in twice the limit of that function. A table is provided to help students understand the different scenarios where limits are taken, emphasizing the importance of identifying the correct function and the value at which the limit is approached. The paragraph concludes with an example that clarifies the difference between the limit of a function and the function's value at a specific point.

05:01

🔍 Examples of Applying Limit Properties

This paragraph delves into examples that demonstrate the application of limit properties. It begins with a composition of functions, explaining how to approach the limit by starting with the inner function. The process is illustrated with a function f(x) approaching a value of 1 as x approaches 4, leading to the limit of f(x) at x=1. The paragraph then discusses a table of values, distinguishing between actual function values and their corresponding limits, highlighting the importance of recognizing the difference. The summary includes a step-by-step explanation of how to calculate the limit of a function using a given table, emphasizing the need to simplify expressions correctly. The paragraph also touches on piecewise functions, explaining how to determine limits from both the left and right sides of a point and the conditions under which a limit may not exist due to differing left and right limits.

Mindmap

Keywords

💡Calculus

Calculus is a branch of mathematics that deals with rates of change and accumulation. In the video, calculus is the overarching subject, with the focus on understanding limits, which are a fundamental concept in calculus. Limits describe the behavior of a function as the input approaches a certain value, and they are essential for differentiating and integrating functions.

💡Limits

Limits are a foundational concept in calculus that define the value that a function or sequence 'approaches' as the input gets arbitrarily close to a certain point. In the script, the properties of limits are discussed, such as how they can be added or multiplied, which is crucial for understanding more complex calculus concepts.

💡Algebraic Properties

Algebraic properties refer to the rules that govern the operations on algebraic expressions, such as addition, multiplication, and exponentiation. The video script explains how these properties can be applied to limits, such as adding two limits of the same function resulting in twice the limit of that function.

💡Approaches

The term 'approaches' in calculus is used to describe the process of a variable getting arbitrarily close to a certain value, without necessarily reaching it. In the script, it is used to describe the behavior of x as it gets close to specific constants like 'c' or 'four', determining the value of the limit.

💡Constant

In the context of limits, a constant is a value that does not change. The script mentions 'c' as a constant that x approaches. Constants are important in defining the point at which the limit is taken.

💡Function Composition

Function composition is when one function is applied within another. In the script, it is illustrated with an example where the limit of a function within another function is taken as x approaches a certain value. Understanding composition is key to solving more complex limit problems.

💡Table of Values

A table of values is a list that shows the output of a function for different input values. In the script, it is used to demonstrate the difference between the actual values of a function at a certain point and the limit of the function as x approaches that point.

💡Piecewise Functions

Piecewise functions are defined by multiple pieces, each with its own expression for the function. The script explains how to find limits for piecewise functions by considering the left and right sides of a point, which is crucial for understanding the behavior of these functions near certain values.

💡Mastery Check

A mastery check is a test or exercise designed to assess understanding and proficiency in a particular subject. In the script, it is mentioned as a way for students to test their understanding of the concepts taught in the calculus lesson.

💡Properties of Logarithms

The properties of logarithms are rules that describe how logarithmic functions behave and can be manipulated. In the script, the property that the natural logarithm of 'e' is 1 is used to simplify an expression, demonstrating the application of logarithmic properties in solving limit problems.

Highlights

Introduction to the properties of limits in calculus, specifically focusing on algebraic properties.

Comparison of limits to middle school algebra, emphasizing the similarity in handling variables.

Explanation of the limit of f(x) + f(x) as x approaches a constant c, resulting in 2 times the limit of f(x) as x approaches c.

Importance of identifying the correct function when dealing with limits, especially in complex expressions.

Example of calculating the limit as x approaches 1 for a function involving f(x) and g(x), highlighting the need to consider the function's definition域.

Clarification on the difference between the limit as x approaches a value and the function's value at that point.

Demonstration of how to handle composition of functions within limits, starting with the inner function's limit.

The significance of the approach direction in limits, especially when dealing with composition and inner functions.

Example illustrating the calculation of a limit involving a table of function values and the distinction between function values and limits.

Emphasis on the difference between function values at a point and the limit as x approaches that point, using a table of values.

Introduction to piecewise functions and how to calculate limits from the left and right sides of a point.

Explanation of how to determine the limit of a piecewise function when approaching a point from both sides and the conditions for the limit to exist.

The concept of undefined limits in piecewise functions when the left and right side limits do not match.

Encouragement for students to practice calculating limits on their own and verifying their answers with the provided solutions.

Review of logarithmic properties and their application in calculating limits, specifically the natural log of e raised to a power.

Final reminder to distinguish between the value of a function and its limit, highlighting the importance of this distinction in problem-solving.

Transcripts

Browse More Related Video

Calculus AB/BC – 1.9 Connecting Multiple Representations of Limits

AP Calculus AB - 1.5 Determining Limits Using Algebraic Properties of Limits

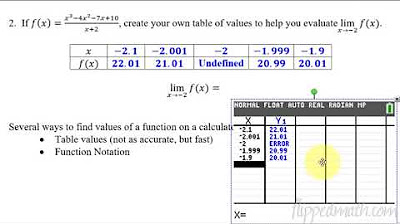
Calculus AB/BC – 1.4 Estimating Limit Values from Tables

AP Calculus AB - 1.9 Connecting Multiple Representations of Limits

Calculus - One Sided Limits

AP Calculus AB - 1.4 Estimating Limit Values from Tables

Calculus AB/BC – 1.5 Determining Limits Using Algebraic Properties

Takeaways

Q & A

What is the main topic of the calculus lesson presented by Mr. Bean?

How does Mr. Bean relate the concept of limits to something familiar from middle school algebra?

What is the basic property of limits that Mr. Bean discusses in the context of adding two limits of the same function?

What is the significance of the constant 'c' in the context of limits discussed in the script?

How does Mr. Bean explain the concept of limits involving different functions f and g with different approaches?

What is the trick mentioned by Mr. Bean when dealing with the limit as x approaches 1 in the context of f(x) and g(x)?

Can you explain the composition of functions in limits as presented in the second example of the script?

What is the difference between the actual function value and the limit value as illustrated in the table of values in the script?

How does Mr. Bean handle the subtraction of an actual function value from a limit in the script's last example?

What is the concept of piecewise functions in limits as discussed in the script?

Why does the limit not exist in the case of the piecewise function as x approaches -5 in the script?