Integral Test as Estimation | MIT 18.01SC Single Variable Calculus, Fall 2010
TLDRThe video script explores the concept of divergent and convergent series, focusing on the series log(n)/n starting from n=3 to infinity. It challenges viewers to estimate how long it takes for the partial sum to reach 5,000, assuming each term's addition takes an incredibly short time. The script uses the integral test to confirm the series' divergence and provides bounds for partial sums. The surprising conclusion is that even with the fastest computers, reaching the sum would take an astronomically long time, humorously compared to the age of the universe.
Takeaways
- 📚 The video script discusses the concept of infinite series, specifically divergent and convergent series, and their rates of divergence.
- 🔍 An experiment is proposed where the series \( \sum_{n=3}^{\infty} \frac{\log n}{n} \) is summed, starting from n=3, to observe the rate of divergence.
- 💻 The script suggests using a computer to track partial sums of the series and stop when the sum exceeds 5,000.
- ⏱️ The time it takes for the computer to add each term is estimated to be \( 10^{-20} \) seconds, highlighting the rapid computation capability.
- 🔢 The series is confirmed to diverge to positive infinity using the integral test, ensuring that the sum will eventually exceed 5,000.
- 📉 The integral test provides a method to estimate the rate of divergence by comparing the area under the curve to the partial sums.
- 📈 The script includes a graphical representation of the function \( y = \frac{\log x}{x} \) to illustrate its behavior and peak value.
- 📚 The integral from 3 to infinity of \( \frac{\log x}{x} \) is calculated to understand the divergence better, showing that it diverges as \( \frac{1}{2} \log^2 x \).
- 🔑 The script uses the integral test to provide bounds for the partial sums, showing that they are closely related to the integral's value.
- 🔢 An approximation is made to estimate when the partial sum S_N reaches approximately 5,000, leading to an estimation of the term count needed.
- 🕒 The total time required to reach the sum of 5,000 is calculated to be an extraordinarily large number, emphasizing the slow convergence of the series.
- 🌌 The final takeaway humorously compares the time required to compute the sum to the age of the universe, indicating it's not a practical endeavor.
Q & A
What is the main topic of the video script?
-The main topic of the video script is the concept of divergent and convergent series, specifically focusing on the series of log(n)/n and its rate of divergence.
What is a divergent series?
-A divergent series is an infinite series that does not converge to a finite limit. It means the sum of its terms continues to increase without bound as more terms are added.
What is a convergent series?
-A convergent series is an infinite series where the sum of its terms approaches a finite limit as more terms are added.
What is the purpose of the experiment described in the script?
-The purpose of the experiment is to observe the rate of divergence of the series log(n)/n by summing its terms and stopping when the partial sum reaches or exceeds 5,000.
Why does the script suggest not to actually run the experiment?
-The script suggests not to run the experiment because the time it would take to reach the partial sum of 5,000 is extraordinarily long, making it impractical to perform.
How does the script use the integral test to analyze the series?
-The script uses the integral test to show that the series diverges to positive infinity by integrating log(x)/x from 3 to infinity and demonstrating that the integral diverges.
What is the significance of the integral test in this context?
-The integral test is significant because it not only determines whether a series diverges or converges but also provides a good estimate of how quickly the series diverges or converges.
How does the script approximate the Nth partial sum of the series?
-The script approximates the Nth partial sum by bounding it between the integral of log(x)/x from 3 to N+1 and the sum of the first term plus the integral from 3 to N+1.
What is the estimated number of terms needed to reach a partial sum of approximately 5,000?
-The estimated number of terms needed is approximately e^100, which is roughly 2 times 10^43.
How long would it take to reach the partial sum of 5,000, assuming each term takes 10^-20 seconds to compute?
-It would take approximately 2 times 10^23 seconds, which is about 50,000 times the age of the universe.
What is the key takeaway from the problem discussed in the script?
-The key takeaway is the practical application of the integral test to estimate the divergence rate of a series and the realization that some mathematical problems are so large in scale that they are not feasible to solve in a human timescale.
Outlines
📚 Introduction to Infinite Series and Experiment Concept
The script begins with a discussion on infinite series, distinguishing between convergent and divergent series and their rates of divergence. The presenter introduces an experimental scenario where the series 'log n over n' is summed starting from n equals 3 to infinity, with the goal of observing the rate of divergence. The experiment involves tracking partial sums and stopping once the sum exceeds 5,000. The presenter humorously suggests not to actually perform the experiment due to its impracticality but encourages the viewer to think about the problem.
🔍 Analyzing the Series' Divergence Using the Integral Test
This paragraph delves into the integral test to determine the divergence of the series. The presenter explains that the integral of 'log x over x' from 3 to infinity diverges, indicating that the series also diverges to positive infinity. The integral test not only confirms divergence but also provides a good estimate of the rate of divergence. The presenter uses a graphical representation to illustrate the function's behavior and its peak, suggesting an exercise to find the exact peak value for interested viewers.
📉 Estimating the Partial Sums and the Time Required for the Experiment
The presenter continues by estimating the number of terms needed in the series to reach a partial sum of approximately 5,000. Using the integral test as a bounding method, the script provides an approximation for the Nth partial sum and relates it to the integral's value. The constants involved are acknowledged to be small, and an approximate sign is introduced for simplicity. The presenter then solves for N when the partial sum is about 5,000, leading to an estimation of the number of terms required.
🕒 Conclusion: The Experiment's Timeframe and the Importance of the Integral Test
The final paragraph concludes the experiment by calculating the time it would take to reach the desired partial sum, assuming each term's computation takes 10 to the minus 20 seconds. The presenter provides a staggering estimate of the time required, comparing it to the age of the universe to emphasize the impracticality of the task. The key takeaway is the integral test's utility in bounding partial sums and providing insights into the series' behavior, making it a central tool in understanding the problem's scale.
Mindmap
Keywords
💡Infinite Series
💡Divergent Series
💡Convergent Series
💡Partial Sums
💡Integral Test
💡Rate of Divergence
💡Logarithmic Function
💡Computational Power
💡Approximation
💡Exponential Function
💡Order of Magnitude
Highlights
Introduction to infinite series of constants and the concepts of divergent and convergent series.
Proposed experiment to sum the series of log n over n starting from n equals 3 to infinity.
Objective to track partial sums and stop when the sum exceeds 5,000.
Estimation of computer speed to add an additional term to the partial sums in 10 to the minus 20 seconds.
Discussion on the possibility of the series converging to a number less than 5,000.
Use of the integral test to confirm the series diverges to positive infinity.
Explanation of the integral test providing an estimate of the rate of divergence.
Graphical representation of the function y equals log of x over x and its behavior as x increases.
Analysis of the peak value of the function and its significance in the context of the problem.
Application of the integral test to bound the partial sums between two expressions.
Derivation of an approximation for the Nth partial sum based on the integral test.
Estimation of the number of terms needed for the partial sum to reach approximately 5,000.
Calculation of the time required to reach the partial sum of 5,000 given the computer's speed.
Comparison of the time required to the age of the universe to emphasize the impracticality of the task.
Final thoughts on the integral test's utility in solving the problem and its limitations.
Humorous conclusion highlighting the impracticality of waiting for the computation to complete.
Transcripts
Browse More Related Video

Infinite series as limit of partial sums | Series | AP Calculus BC | Khan Academy
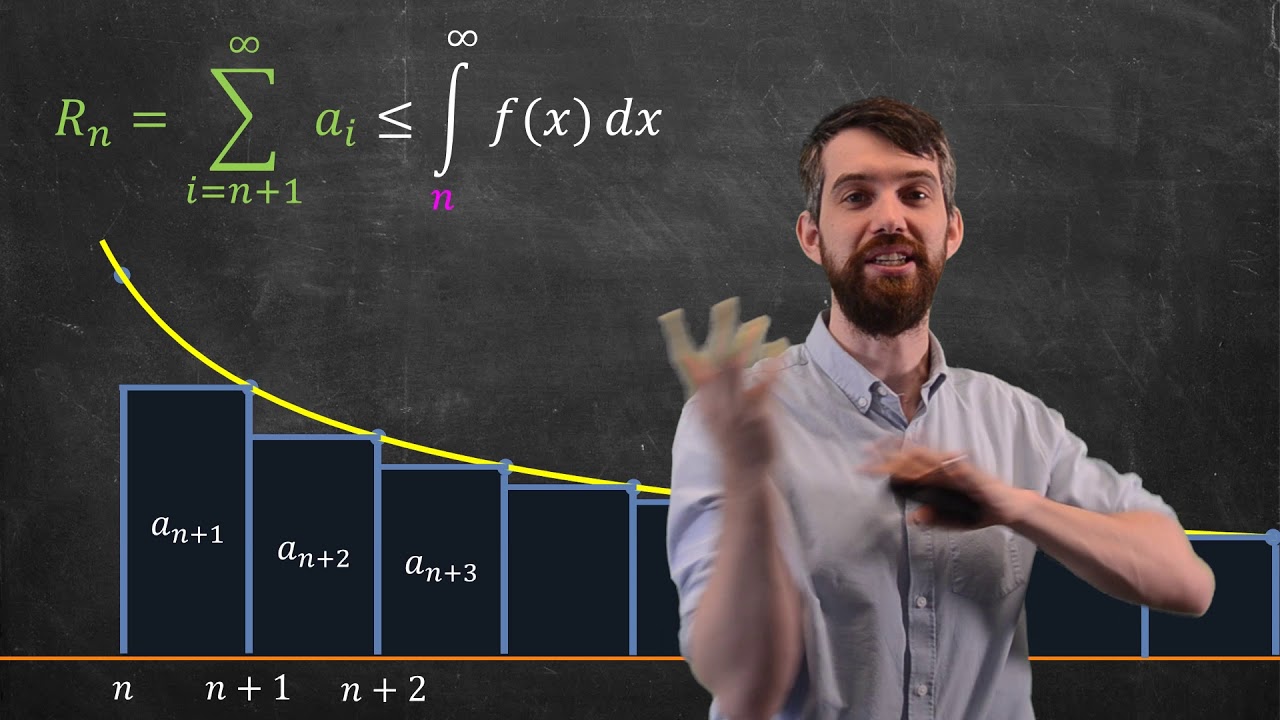
Estimating the Remainder of a Series Approximation via the Integral Test
Calculus 2 Lecture 9.5: Showing Convergence With the Alternating Series Test, Finding Error of Sums
Partial sums intro | Series | AP Calculus BC | Khan Academy
Limit of a Series | MIT 18.01SC Single Variable Calculus, Fall 2010

Worked example: Series estimation with integrals | Series | AP Calculus BC | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: