Taylor Series Day 3
TLDRIn this educational video, the instructor explores the concept of writing power series, specifically focusing on geometric series. They explain how to identify the first term and common ratio 'r', and the importance of 'r' being less than one for convergence. The video includes examples of writing series for different 'r' values, including alternating series, and demonstrates how to manipulate functions into the form 'a / (1 - r)' for easier series expansion. The instructor also discusses the interval of convergence and provides a graphical comparison of actual functions versus their series approximations, highlighting the accuracy within the convergence interval and divergence outside of it.
Takeaways
- 📚 The video is about writing power series for functions that can be represented as geometric series.
- 👧 A student named Mia asked about writing the Taylor series for 1/(1 - 2x) and recognizing it as a geometric series.
- 📈 A geometric series converges only if the absolute value of the common ratio (r) is less than one.
- 📝 The general term of a geometric series is the first term times the ratio raised to the power of n.
- ➕ Example provided: If the first term is 2 and the ratio is 3, the series would be 2 + 6 + 18 + ...
- ➗ If the first term is 1 and the ratio is x, the series would be 1 + x + x^2 + x^3 + ...
- 🔄 For a geometric series like 1/(1 - x^2), the first term is 1 and the ratio is x^2, leading to the series 1 + x^2 + x^4 + x^6 + ...
- 📊 The interval of convergence for a geometric series is determined by the absolute value of the common ratio being less than one.
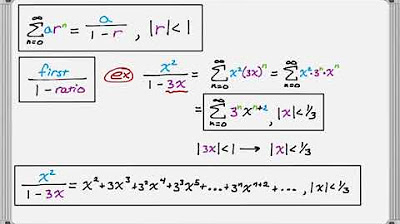
- 🔍 The video explains how to manipulate a function to fit the form of a/(1 - r) for easier series expansion.
- 📉 A geometric series does not converge at the endpoints of its interval of convergence.
Q & A
What is a geometric series?
-A geometric series is a sequence of numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio.
What condition must be met for a geometric series to converge?
-A geometric series converges if the absolute value of the common ratio is less than one. If the absolute value of the common ratio is greater than or equal to one, the series diverges.
How do you write the general term of a geometric series?
-The general term of a geometric series is given by the first term multiplied by the common ratio raised to the power of n, where n is the term number.
What is the first term and common ratio in the series 2 + 6 + 18 + ...?
-The first term is 2 and the common ratio is 3.
How does the sign of the common ratio affect the series?
-If the common ratio is negative, the series will alternate in sign, resulting in an alternating series.
What is the Taylor series and how is it related to geometric series?
-The Taylor series is a representation of a function as an infinite sum of terms calculated from the values of the function's derivatives at a single point. It is related to geometric series in that it can be written in a similar form, with the first term and common ratio derived from the function and its derivatives.
What is the interval of convergence for the series 1 + x^2 + x^4 + ...?
-The series converges for all values of x such that the absolute value of x is less than 1.
How can you manipulate a function to write its Taylor series?
-You can manipulate a function to write its Taylor series by expressing it in the form a / (1 - R), where a is the first term and R is the common ratio, and then expanding it as a geometric series.
What is the interval of convergence for the series 1/4 + x/16 + x^2/64 + ...?
-The series converges for all values of x such that the absolute value of x/4 is less than 1, which translates to -4 < x < 4.
How do you determine the interval of convergence for a Taylor series?
-The interval of convergence for a Taylor series is determined by the condition that the absolute value of the common ratio must be less than one. This involves solving inequalities based on the common ratio.
What happens to the convergence of a geometric series at its endpoints?
-A geometric series never converges at its endpoints. The convergence only applies to values within the interval determined by the condition on the common ratio.
Outlines
📚 Understanding Geometric Series and Power Series
This paragraph introduces the concept of writing a power series by examining a function in the form of a geometric series. The teacher explains that a geometric series converges if the absolute value of the common ratio (r) is less than one, and diverges if r is greater than or equal to one. The paragraph provides examples of geometric series with different first terms and ratios, both convergent and divergent. The teacher also discusses how to write a Taylor series for a function like 1/(1 - 2x) by recognizing it as a geometric series and writing out the first few terms. The importance of the interval of convergence is highlighted, showing that the series converges well within the interval but not outside of it.
🔍 Manipulating Functions to Fit Geometric Series Form
In this paragraph, the teacher demonstrates how to manipulate a function to fit the form required for writing a geometric series. The example given is 1/(4 - x), which is adjusted to 1/(4 - x/4) to fit the a/(1 - r) form. The teacher explains how to write the geometric series for this function, including the first few terms and the general term. The convergence of the series is then discussed, with the interval of convergence being determined by the absolute value of the common ratio being less than one. Another example is provided where the function is further manipulated to be centered at 2, and the geometric series is written out accordingly.
📉 Determining the Interval of Convergence for Geometric Series
The final paragraph focuses on determining the interval of convergence for a geometric series. The teacher uses the example of a series with a common ratio of -2/3 and shows how to find the values of x for which the series converges. The process involves factoring out constants and manipulating the inequality to find the interval. The teacher emphasizes that the geometric series will never converge at the endpoints of the interval. The example provided illustrates the steps needed to find the interval of convergence and how it is used to understand the behavior of the series.
Mindmap
Keywords
💡Power Series
💡Geometric Series
💡Convergence
💡Common Ratio (R)
💡First Term
💡Taylor Series
💡Interval of Convergence
💡Manipulation
💡Polynomial Approximation
💡End Points
💡Factoring
Highlights
Introduction to writing a power series for a function in the form of a geometric series.
Explanation of a student's question about the Taylor series for 1/(1 - 2x).
Review of the convergence criteria for a geometric series.
Practice in writing geometric series with examples.
General term formula for a geometric series.
Divergent geometric series example with first term 2 and common ratio -3.
Writing a geometric series with a common ratio of x.
How to identify the interval of convergence for a power series.
Graphical comparison of a function and its power series approximation.
Demonstration of the difference between the actual function and its approximation outside the interval of convergence.
Technique to manipulate a function into the form a/(1 - R) for power series expansion.
Writing a geometric series for a function with a common ratio of x/4.
Determining the interval of convergence for a manipulated function.
Complex example of manipulating a function to fit the a/(1 - R) form and finding its power series.
Final example of determining the interval of convergence for a complex function.
Summary of the importance of the interval of convergence in power series.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: