AP Calculus BC Lesson 10.2

Elizabeth Fein

31 Dec 202223:14

EducationalLearning

32 Likes 10 Comments

TLDRThis video lesson delves into infinite geometric series, explaining their structure and providing examples. It emphasizes the importance of the common ratio (R) in determining the series' convergence or divergence, with a series converging if |R|<1 and diverging if |R|≥1. The formula for the sum of a converging series is also highlighted, with a/(1-R). The video further illustrates how to manipulate non-standard series into geometric form and solve for their sums, reinforcing the concepts with multiple-choice questions and real-world applications, showcasing the practicality of mathematical concepts.

Takeaways

📚 An infinite geometric series is a sum of the form ∑ from n=0 to Infinity of a * R^n, where 'a' is the first term and 'R' is the common ratio.
🔢 Examples of geometric series include ∑ from n=0 to Infinity of 5 * 2^n and ∑ from n=0 to Infinity of (-2/3)^n.
🌟 A series can be rewritten to fit the geometric series format, such as transforming 4 * (1/3)^n to ∑ from n=0 to Infinity of 4 * (1/3)^n.
📈 The convergence or divergence of a geometric series is determined by the absolute value of the common ratio 'R'. If |R| < 1, the series converges; if |R| ≥ 1, it diverges.
💡 The formula for the sum of a convergent infinite geometric series is S = a / (1 - R), where 'a' is the first term and 'R' is the common ratio.
🔄 If the series does not start at n=0, the first term of the sequence is used as 'a' in the convergence formula.
📊 The common ratio 'R' represents the factor by which each term is multiplied to get the next term in the series.
🌐 The video provides examples of identifying 'a' and 'R' for various series and determining their convergence or divergence.
🧩 The video also demonstrates how to manipulate and rewrite non-standard geometric series to apply the rules for infinite geometric series.
📝 Practice problems and multiple-choice questions are used to illustrate the concepts and application of infinite geometric series.
🎓 Understanding the properties of geometric series, including common ratios and convergence criteria, is essential for working with these mathematical concepts.

Q & A

What is an infinite geometric series?
-An infinite geometric series is a series in the format sum from n equals 0 to Infinity of a times R to the power of n, where 'a' is the first term and 'R' is the common ratio.
What are some examples of geometric series mentioned in the transcript?
-Examples include the series from n equals 0 to Infinity of 5 times 2 to the power of n, the series from n equals 0 to Infinity of negative two-thirds to the power of n, and the series from n equals 0 to Infinity of 4 times 1/3 to the power of n.
How can a series that does not look like an infinite geometric series be rewritten into that format?
-By manipulating the given series into the form of sum from n equals 0 to Infinity of a times R to the power of n, where 'a' is the first term and 'R' is the common ratio.
What is the significance of the common ratio 'R' in a geometric series?
-The common ratio 'R' is the factor by which you multiply each term to get the next term in the series. It is crucial in determining the convergence or divergence of the series.
How can you determine if an infinite geometric series converges or diverges?
-An infinite geometric series converges if the absolute value of R is less than one and diverges if the absolute value of R is greater than or equal to one.
What is the formula used to find the sum of a convergent infinite geometric series?
-The sum of a convergent infinite geometric series is given by the formula S = a / (1 - R), where 'a' is the first term and 'R' is the common ratio.
How does the starting term 'n' affect the application of the convergence formula?
-If 'n' does not equal zero, 'a' in the formula represents the first term of the sequence, not the term when n equals zero.
What happens if the common ratio 'R' is zero?
-The common ratio 'R' cannot be zero because it would result in a division by zero in the convergence formula, which is undefined.
How does the absolute value of 'R' being between 0 and 1 affect the series?
-If the absolute value of 'R' is between 0 and 1, the series converges because it meets the condition for convergence.
What is the sum of the infinite geometric series from n equals 0 to Infinity of negative 2 and R equals 3/4?
-The sum of this series converges to negative 2 over 1 minus 3/4, which simplifies to negative 8.
What is the sum of the infinite geometric series from n equals 2 to Infinity of 1/5 to the power of n?
-The sum of this series converges to 1/25 over 4/5, which simplifies to 1/20.

Outlines

00:00

📚 Introduction to Infinite Geometric Series

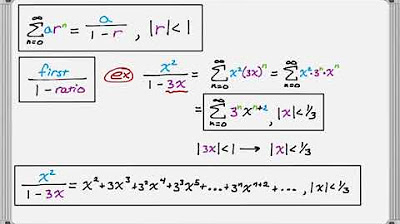
This paragraph introduces the concept of infinite geometric series, defined as the sum from n equals 0 to Infinity of a times R to the power of n. It provides examples of geometric series and explains how to manipulate series into geometric form. The importance of the common ratio (R) is emphasized, and the conditions for the convergence or divergence of a series are discussed, with the absolute value of R being the key factor. The paragraph also presents the formula for the sum of a convergent infinite geometric series, which is a/(1-r), applicable when n starts at zero. The concept is further clarified with examples that demonstrate how to identify the first term (a) and the common ratio (R) for different series.

05:00

🔍 Analyzing Convergence and Divergence in Geometric Series

This paragraph delves into the process of determining whether a geometric series converges or diverges. It explains that if the absolute value of R is less than one, the series converges, and if it's equal to or greater than one, the series diverges. The paragraph provides a step-by-step approach to identify the values of a and R from given series and apply the formula for the sum of an infinite geometric series. It also addresses series that do not start at n equals zero, explaining how to find the first term as a in such cases. The explanation is supported by multiple examples that illustrate the process of analyzing and solving for different types of geometric series.

10:01

🧮 Solving for Convergence and Sum of Geometric Series

The paragraph focuses on solving for the convergence and sum of various geometric series. It begins by addressing a series that does not fit the standard geometric series format and demonstrates how to rewrite it for analysis. The concept of absolute value and its role in determining convergence is reiterated. The paragraph then presents a series of examples, each with a different structure, showing how to identify the first term and the common ratio, and how to apply the formula for the sum of an infinite geometric series. It also covers cases where the series diverges and how to express the result in such instances.

15:03

📈 Graphing Convergence of a Complex Geometric Series

This paragraph discusses the convergence of a complex geometric series involving exponential functions. It explains the process of transforming the series into a recognizable geometric form and the importance of the absolute value of the common ratio in determining convergence. The paragraph then describes a method for graphing the series to find the range of values for K that ensure the series converges. The use of a graphing calculator is highlighted as a tool for finding the points of intersection that define the range of K, and the paragraph concludes with the identification of the correct interval for K based on the graphed results.

20:06

🤔 Exploring the Convergence of Another Geometric Series

The final paragraph explores the convergence of another geometric series, this time involving a term with an exponential function. It outlines the process of identifying the series as geometric and the need to rewrite it in a standard form. The paragraph emphasizes the importance of the absolute value of the common ratio for determining convergence and provides a detailed explanation of how to find the sum of the series if it converges. The process involves finding the first term and applying the formula for the sum of an infinite geometric series. The paragraph concludes with the solution for the series and the correct answer choice based on the given options.

Mindmap

Keywords

💡Infinite Geometric Series

An infinite geometric series is a sequence of numbers where each term is found by multiplying the previous term by a fixed, non-zero number called the common ratio. In the video, this concept is central as it forms the basis for discussing the convergence or divergence of such series. For example, the series 'sum from n equals 0 to Infinity of 5 times 2 to the power of n' is an infinite geometric series with a common ratio of 2.

💡Common Ratio (R)

The common ratio (R) in a geometric series is the factor by which each term is multiplied to obtain the next term. It is crucial in determining whether the series converges or diverges. The video emphasizes that the absolute value of R must be less than 1 for the series to converge, as seen when discussing the series 'sum from n equals 0 to Infinity of 5 times 2 to the power of n' where R is 2.

💡Convergence

In the context of the video, convergence refers to the property of an infinite geometric series where the sum of its terms approaches a finite value as the number of terms increases without bound. This occurs when the absolute value of the common ratio is less than 1. For instance, the series 'sum from n equals 0 to Infinity of 4 times 1/3 to the power of n' converges because the absolute value of 1/3 is less than 1.

💡Divergence

Divergence in an infinite geometric series means that the series does not approach a finite value; instead, the sum increases without bound as more terms are added. This happens when the absolute value of the common ratio is greater than or equal to 1. The video explains that the series 'sum from n equals 1 to Infinity of 2 to the power of n' diverges because the absolute value of 2 is greater than 1.

💡First Term (a)

The first term (a) in an infinite geometric series is the initial value that starts the sequence. It is used in the formula for the sum of a convergent series. In the video, the first term is identified as a crucial component in determining the sum of the series, such as in the series 'sum from n equals 0 to Infinity of 5 times 2 to the power of n' where the first term a is 5.

💡Sum Formula

The sum formula for an infinite geometric series is a = a / (1 - r), where 'a' is the first term and 'r' is the common ratio. This formula is used to find the sum of a convergent series. The video explains that this formula is only applicable when the series starts at n=0, as seen when discussing the sum of the series 'sum from n equals 0 to Infinity of 4 times 1/3 to the power of n'.

💡Rewriting Series

Rewriting series involves manipulating the given series into the standard form of an infinite geometric series, which is 'sum from n equals 0 to Infinity of a times R to the power of n'. This is necessary to apply the rules and formulas associated with geometric series. In the video, the series 'sum from n equals 0 to Infinity of negative two-thirds to the power of n' is rewritten to fit the standard form by identifying a and R correctly.

💡Absolute Value

The absolute value of a number is its distance from zero on the number line, without considering direction. It is used in the context of geometric series to determine whether the series converges or diverges. The video explains that for a series to converge, the absolute value of the common ratio R must be less than 1.

💡Series Manipulation

Series manipulation refers to the process of transforming a given series into a form that allows for the application of specific rules or formulas, such as those for geometric series. This often involves algebraic techniques like factoring and simplification. The video demonstrates series manipulation when converting non-standard series into the form 'a times R to the power of n'.

💡Graphing Calculator

A graphing calculator is a specialized electronic device used to perform mathematical calculations and plot graphs of functions. In the video, it is mentioned as a tool for finding the values of K for which a series converges by graphing the function 'y equals k e to the power of negative 0.24, K' and finding when the absolute value of y is less than 1.

💡Interval Notation

Interval notation is a way of expressing a set of numbers on a number line, typically used in mathematics to define intervals of real numbers. In the video, interval notation is used to express the range of values for K that allow the series to converge, as found using a graphing calculator.

Highlights

Exploration of infinite geometric series, a mathematical concept where the sum of a series is calculated as a times R to the power of n, from n equals 0 to infinity.

Examples of geometric series provided, including 5 times 2 to the power of n and negative two-thirds to the power of n, illustrating the concept with different values of a and R.

Rewriting non-standard geometric series into the standard form to apply specific rules, such as the series with a term of 4 times 1/3 to the power of n.

Explanation of the common ratio (R) in geometric series, which is the factor multiplied to each term to get the next term in the series.

Criteria for the convergence or divergence of an infinite geometric series based on the absolute value of R, with the series converging if |R| is less than one and diverging if |R| is greater than or equal to one.

The formula for the sum of an infinite geometric series when it converges, expressed as a/(1 - R), with the condition that the series must start at n equals zero.

Adjustment of the formula for geometric series that do not start at n equals zero, using the first term of the sequence as 'a' instead.

Demonstration of determining whether a specific geometric series converges or diverges using the absolute value of R, with examples including series with R equal to 2 and R equal to 1/5.

Process of identifying 'a' and 'R' for given geometric series and applying the convergence formula to find the sum of the series.

Detailed walkthrough of solving geometric series problems, including series that do not start at n equals zero and those that require manipulation to fit the standard form.

Use of a graphing calculator to find the values of 'K' for which a specific geometric series converges, demonstrating the practical application of technology in mathematical analysis.

Inclusion of multiple-choice questions to test understanding of infinite geometric series, offering a variety of problem-solving scenarios.

Explanation of how to handle geometric series with non-integer exponents, such as series involving the cosine function and fractional powers.

Discussion of the absolute value of the common ratio in relation to the convergence of the series, emphasizing the importance of |R| being less than one.

Final answer for the specific geometric series problem, providing a clear example of how to apply the rules and formulas learned throughout the lesson.

Transcripts

Browse More Related Video

Geometric Series (Precalculus - College Algebra 72)

Ratio Test

Geometric Series Summing to Functions

Taylor Series Day 3

Another derivation of the sum of an infinite geometric series | Precalculus | Khan Academy

Ch. 12.3 Geometric Sequences

Related Tags

Mathematics InfiniteSeries Convergence Education Geometry Calculus ProblemSolving OnlineLesson Algebra Trigonometry