Sum of an infinite geometric series | Sequences, series and induction | Precalculus | Khan Academy

Khan Academy
8 Nov 201304:45
EducationalLearning
32 Likes 10 Comments

TLDRThis video explores the intriguing concept of the sum of an infinite geometric series, where the first term is 'a' and the common ratio is 'r'. The presenter explains that the sum converges to a finite value only when the absolute value of 'r' is less than one, as the series terms approach zero with increasing exponents. The formula for the sum is a/(1-r), and the video provides an example with a series starting at 1 and having a common ratio of 1/3, resulting in a sum of 3/2. The content is presented in a way that challenges the viewer to think about different scenarios for 'r' and their impact on the series' convergence.

Takeaways
  • 📚 The video discusses the concept of the sum of an infinite geometric series, which is a fascinating mathematical topic.
  • 🔢 The sum of an infinite geometric series is defined as the limit of the sum of the first 'n' terms as 'n' approaches infinity.
  • 📉 For an infinite series to have a finite sum, the absolute value of the common ratio 'r' must be less than one.
  • 🚫 If the absolute value of 'r' is greater than one, the series diverges to infinity and does not have a finite sum.
  • 🚨 If 'r' equals one, the series results in division by zero, which is undefined and breaks the formula.
  • 🌟 The formula for the sum of an infinite geometric series is \( a \over 1 - r \), where 'a' is the first term and 'r' is the common ratio.
  • 📌 The series is only considered geometric if 'r' is not zero, as 'r' equals zero would not constitute a series.
  • 📉 When the absolute value of 'r' is less than one, the terms of the series approach zero as 'n' approaches infinity.
  • 💡 The video uses the example of a series with a first term of 1 and a common ratio of 1/3 to illustrate the concept.
  • 🧮 The sum of the example series is calculated as 3/2 or 1.5, demonstrating how the formula works in practice.
  • 📝 The video encourages viewers to pause and think about the implications of different values of 'r' on the series' convergence or divergence.
Q & A

  • What is the formula for the sum of a finite geometric series?

    -The formula for the sum of a finite geometric series is \( S_n = a \frac{1 - r^n}{1 - r} \), where 'a' is the first term and 'r' is the common ratio.

  • What is an infinite geometric series?

    -An infinite geometric series is a series where the number of terms goes to infinity, and its sum can be finite under certain conditions.

  • What is the condition for the sum of an infinite geometric series to be finite?

    -The sum of an infinite geometric series is finite when the absolute value of the common ratio 'r' is less than one (|r| < 1).

  • What happens to the sum of an infinite geometric series if the absolute value of the common ratio is greater than one?

    -If the absolute value of the common ratio is greater than one, the sum of the series becomes infinitely large.

  • What is special about the common ratio being equal to one in an infinite geometric series?

    -If the common ratio is equal to one, the denominator in the sum formula becomes zero, making the formula invalid and the sum undefined.

  • How does the formula for the sum of an infinite geometric series differ from the finite case?

    -For an infinite geometric series, the formula is \( S = \frac{a}{1 - r} \), which is the limit of the finite series formula as 'n' approaches infinity.

  • What is the significance of the term 'a' in the context of an infinite geometric series?

    -In the context of an infinite geometric series, 'a' represents the first term of the series.

  • Can you provide an example of an infinite geometric series and its sum?

    -An example is a series with the first term 'a' being 1 and a common ratio 'r' of 1/3. The sum of this series would be \( S = \frac{1}{1 - (1/3)} = \frac{3}{2} \) or 1.5.

  • Why does the term in an infinite geometric series with |r| < 1 approach zero as 'n' approaches infinity?

    -The term approaches zero because multiplying a number with an absolute value less than one by itself repeatedly results in a number with a smaller and smaller absolute value.

  • What is the mathematical justification for the sum of an infinite geometric series being finite when |r| < 1?

    -The mathematical justification is that the terms of the series become insignificantly small as 'n' approaches infinity, effectively making the series converge to a finite sum.

  • How does the video script encourage viewers to engage with the material?

    -The script encourages viewers to pause the video and think about different scenarios for 'r', and it provides hints to guide their understanding of the concept.

Outlines
00:00
📚 Understanding Infinite Geometric Series

This paragraph introduces the concept of the sum of an infinite geometric series, contrasting it with the finite case. The presenter emphasizes the surprising nature of obtaining a finite sum from an infinite number of terms, depending on the common ratio. The discussion begins by considering the limit as the number of terms approaches infinity, and then explores the implications for different absolute values of the common ratio: greater than one, equal to one, and less than one. The focus is on the case where the absolute value of the common ratio is between zero and one, where the series converges to a finite sum, which is a fascinating mathematical phenomenon.

Mindmap
Keywords
💡Finite Geometric Series
A finite geometric series is a sequence of numbers with a constant ratio between successive terms, summed up to a certain number of terms. In the video, the concept is introduced to compare it with the sum of an infinite geometric series, highlighting how the former has a definite end point.
💡Infinite Geometric Series
An infinite geometric series is a sequence of numbers with a constant ratio between successive terms, summed over an infinite number of terms. The video explores how such a series can converge to a finite value under specific conditions, which is a mind-blowing concept given its infinite nature.
💡Common Ratio (r)
The common ratio (r) in a geometric series is the constant factor between successive terms. In the video, the behavior of the sum of the series heavily depends on the value of r, especially whether its absolute value is less than, equal to, or greater than one.
💡Limit
The limit refers to the value that a function or sequence 'approaches' as the input or index approaches some value. In the context of the video, taking the limit as n approaches infinity helps determine the sum of the infinite geometric series.
💡Convergence
Convergence in the context of an infinite series means that the sum of the series approaches a finite value as the number of terms increases indefinitely. The video demonstrates that an infinite geometric series converges when the absolute value of the common ratio is less than one.
💡Divergence
Divergence occurs when the sum of an infinite series does not approach a finite value. The video explains that the series diverges if the absolute value of the common ratio is greater than or equal to one.
💡Absolute Value
The absolute value of a number is its non-negative value, disregarding its sign. The video uses this concept to discuss conditions under which the infinite geometric series converges or diverges, focusing on the absolute value of the common ratio.
💡Exponent
An exponent refers to the number of times a number (the base) is multiplied by itself. The video discusses how raising the common ratio to higher exponents affects the sum of the series, especially when the common ratio is less than one.
💡Sum
The sum in the context of a series is the result of adding all its terms together. The video examines the sum of both finite and infinite geometric series, emphasizing the surprising result that an infinite series can yield a finite sum under certain conditions.
💡A over 1 minus r
The formula a/(1-r) represents the sum of an infinite geometric series where 'a' is the first term and 'r' is the common ratio. The video uses this formula to calculate the sum of an infinite series when the absolute value of the common ratio is less than one, providing a concrete example to illustrate the concept.
Highlights

Introduction to the concept of the sum of an infinite geometric series.

Exploration of the sum of an infinite geometric series as a limit of a finite geometric series as n approaches infinity.

Discussion on the behavior of the series when the absolute value of the common ratio r is greater than one, leading to an infinitely large sum.

Analysis of the case where r equals one, resulting in an undefined sum due to division by zero.

Explanation of the scenario where the absolute value of r is less than one, allowing for a finite sum.

Clarification that a geometric series is not defined when r equals zero.

Description of how the terms of the series approach zero as n approaches infinity when the absolute value of r is less than one.

Illustration of the formula for the sum of an infinite geometric series when |r| < 1, which is a / (1 - r).

Example given with the first term a being one and the common ratio r being 1/3, showing the sum calculation.

Explanation of how the sum of an infinite geometric series with a common ratio of 1/3 equals 3/2.

Highlight of the fascinating concept that an infinite number of terms can sum to a finite value under certain conditions.

Emphasis on the importance of the absolute value of the common ratio in determining the convergence of the series.

Invitation for the viewer to pause the video and think about the implications of different values of r.

Encouragement to consider the behavior of the series for r > 1, r = 1, and r < 1.

Insight into how multiplying a number with an absolute value less than one by itself repeatedly results in a smaller number.

Final summary that the sum of an infinite geometric series is finite and calculable when the absolute value of the common ratio is between zero and one.

Transcripts

Browse More Related Video

Another derivation of the sum of an infinite geometric series | Precalculus | Khan Academy

Geometric Series (Precalculus - College Algebra 72)

Ch. 12.3 Geometric Sequences

AP Calculus BC Exam Review - Geometric Series

Finite geometric series formula justification | High School Math | Khan Academy

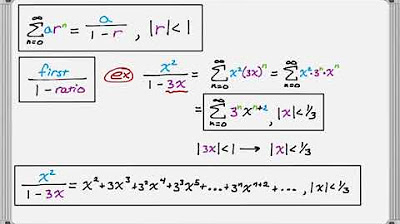
Geometric Series Summing to Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Infinite SeriesGeometric SumMathematicsEducationalSeries LimitCommon RatioAbsolute ValueExponential GrowthEducational VideoMath ConceptsSeries Formula