Riemann Sums and Definite Integrals

Chad Gilliland

3 Nov 201310:41

EducationalLearning

32 Likes 10 Comments

TLDRThis educational video script introduces the concept of integration as a method to calculate the area under a curve. It explains the process of breaking an area into rectangles, using Riemann sums to estimate the area, and then taking the limit as the number of rectangles approaches infinity to find the exact area. The script also covers properties of definite integrals, such as the integral of a constant, and illustrates how to calculate the area under specific functions, including a horizontal line, a linear function, and a semicircle. It emphasizes that there are no product or quotient rules for integration and concludes with examples of calculating net signed areas and integrals over intervals.

Takeaways

📚 The lesson aims to establish a connection between the concepts of area and integration.
📈 The process begins by drawing the function f(x) and identifying the area of interest between points A and B on the x-axis.
📝 The method of finding the area under the curve involves breaking it into rectangles using Riemann sums, which can be left, right, or midpoint.
🔍 The choice of where to place the rectangles does not affect the final area calculation, emphasizing the flexibility of the Riemann sum approach.
🔢 The area of each rectangle is calculated as the product of its width (Δx) and the function value (f(c_k)) at a chosen point c_k within the interval.
🌐 To find the exact area under the curve, the number of rectangles is taken to infinity, which is represented by the limit as n approaches infinity.
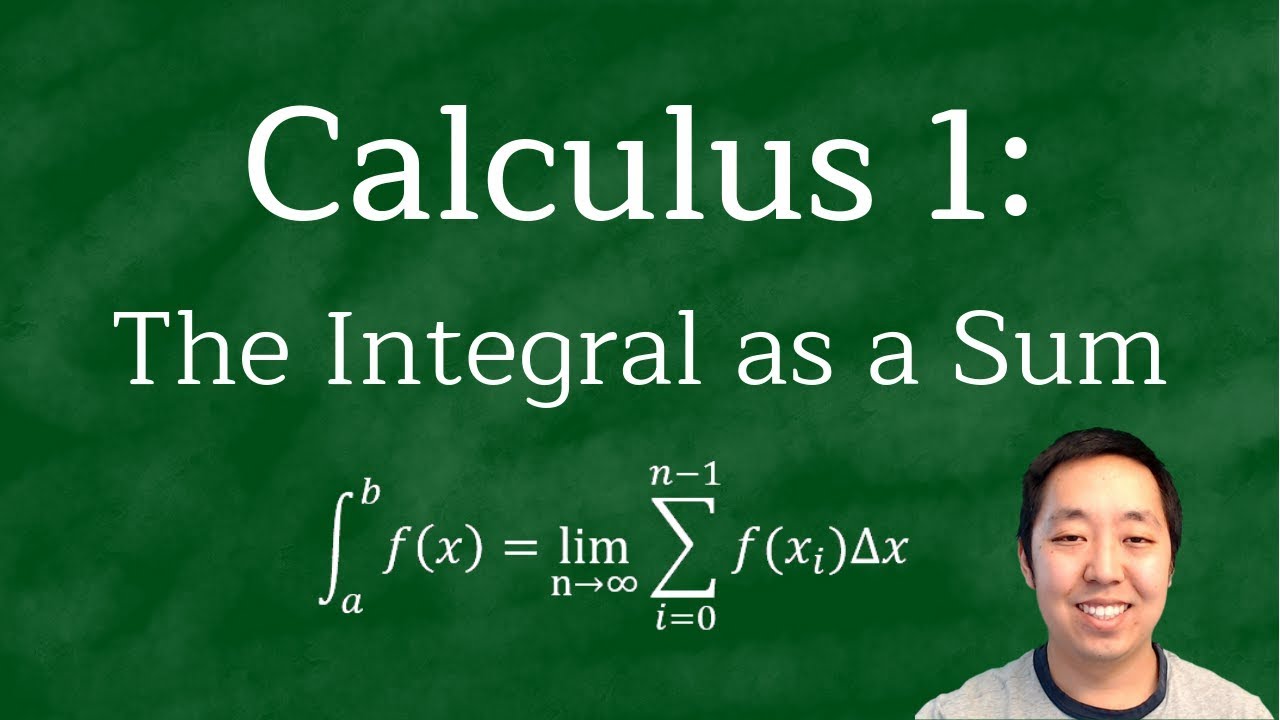
📐 The definition of a definite integral is given as an infinite Riemann sum, illustrating the mathematical foundation of integration.
📉 The integral from A to B of f(x) dx is defined as the area bounded by the curve, the x-axis, and the vertical lines x=A and x=B.
🔄 Properties of definite integrals include the integral from a to a being zero, the integral from B to A being the negation of the integral from A to B, and the ability to factor out constants.
📊 The integral can be split into parts if C lies between A and B, allowing for the calculation of the area in segments.
❌ There is no product or quotient rule for integration, which means integrals of products or quotients cannot be separated into simpler integrals.
📐 Geometric area formulas can be used to calculate the integral of simple functions, such as constants or linear functions, by treating them as geometric shapes like rectangles or trapezoids.
📉 The area under a curve that is a semicircle can be calculated using the formula for the area of a circle and then taking half of that value.
➖ Areas below the x-axis contribute negatively to the total area, which is referred to as the net signed area under the curve.

Q & A

What is the primary focus of the lecture?
-The primary focus of the lecture is to establish a connection between area and integration, explaining how to find the area under a curve using the concept of Riemann sums.
What is a Riemann sum and how is it used to estimate the area under a curve?
-A Riemann sum is a method used to approximate the area under a curve by dividing the area into rectangles. The height of each rectangle is determined by the function value at a chosen point within the interval, and the width is the change in x (Δx). The area under the curve is estimated by summing the areas of these rectangles.
How does the concept of limits relate to finding the exact area under a curve?
-The concept of limits is used to find the exact area under a curve by letting the number of rectangles (n) go to infinity. This turns the Riemann sum into an infinite summation, which is the definition of a definite integral.
What is the definition of a definite integral and how is it related to the area under a curve?
-A definite integral is defined as the limit of the Riemann sum as the number of rectangles (n) approaches infinity. It represents the exact area under the curve from a to b and is denoted as ∫ from a to b of f(x) dx.
What are some properties of definite integrals mentioned in the lecture?
-Some properties of definite integrals mentioned include: the integral from a to a is zero, the integral from b to a is the negation of the integral from a to b, constants can be pulled outside the integral, and integrals can be broken up into pieces or summed across a sum or difference.
Why can't you apply a product rule or quotient rule for integration as you would in differentiation?
-You cannot apply a product rule or quotient rule for integration because integration and differentiation are fundamentally different operations. Integration is about finding the area under a curve, and there is no rule that allows you to separate a product or quotient into separate integrals.
How can you find the area under a constant function from a to b?
-To find the area under a constant function from a to b, you can simply multiply the constant by the width of the interval (b - a). This is because the area forms a rectangle with a constant height.
What is the area under the curve y = x + 2 from 1 to 3, and how is it calculated?
-The area under the curve y = x + 2 from 1 to 3 forms a trapezoid. The area is calculated by averaging the bases (3 and 5), multiplying by the width (2), which results in an area of 8.
What is the area of the upper hemisphere of a circle with a radius of 2, and how is it found?
-The area of the upper hemisphere of a circle with a radius of 2 is half the area of a full circle. The area of a full circle is πr², so the area of the hemisphere is (1/2) * π * 2² = 2π.
How is the net signed area under a curve calculated, and what does it represent?
-The net signed area under a curve is calculated by summing the areas of triangles or other shapes formed under the curve, taking into account the sign of the area (positive for above the x-axis, negative for below). It represents the total area, accounting for regions above and below the x-axis.

Outlines

00:00

📚 Introduction to Area and Integration

This paragraph introduces the concept of connecting area and integration. The speaker begins by drawing a curve and discussing the area under the curve between points A and B. They explain the process of breaking the area into rectangles using Riemann sums, emphasizing that the location of the rectangle within the interval does not matter. The speaker then discusses how to find the exact area under the curve by letting the number of rectangles approach infinity, which leads to the definition of a definite integral. The integral from A to B of f(x) dx is defined as the exact area under the curve, and the speaker highlights the importance of the product of height and width in a Riemann sum.

05:01

📐 Properties of Definite Integrals

The speaker delves into the properties of definite integrals, starting with the fact that the integral from a to a is zero, indicating no area when there is no movement along the x-axis. They also discuss the property that the integral from B to A is the negation of the integral from A to B, which can be useful when switching the limits of integration. The speaker further explains that constants can be factored out of an integral, simplifying the process. They clarify that there are no product or quotient rules for integration, which is a key difference from differentiation. The paragraph concludes with an exploration of how integrals can be used to find areas under curves, even for complex shapes like semicircles and triangles, and how negative areas are handled when the curve is below the x-axis.

10:02

📉 Applications of Integrals in Calculating Areas

In this paragraph, the speaker applies the concept of integrals to calculate areas under different types of curves. They start with a simple example of a constant function, demonstrating how the integral from 1 to 6 of 4 can be calculated as a rectangle with an area of 20. The speaker then moves on to more complex functions, such as a linear function x + 2, and shows how to calculate the area under this curve as a trapezoid. They also discuss the area under a semicircle, explaining that it is half the area of a full circle. The speaker emphasizes the importance of considering the sign of the area when the curve is below the x-axis, as this affects the calculation of the net signed area. The paragraph concludes with examples of how to combine integrals to find the total area over a larger interval, illustrating the additive property of integrals.

Mindmap

Keywords

💡Integration

Integration is a fundamental concept in calculus that deals with finding the area under a curve. In the video, it is used to explain how to calculate the exact area between a curve and the x-axis over a given interval. Integration is described as the limit of an infinite sum of areas of rectangles.

💡Riemann Sum

A Riemann Sum is an approximation of the integral (area under the curve) by summing up areas of multiple rectangles. The video explains that it doesn't matter whether the sample points are taken from the left, right, or midpoint of each subinterval, as long as the number of intervals approaches infinity, the Riemann Sum converges to the exact integral.

💡Limit

The limit is a mathematical concept used to describe the value that a function approaches as the input approaches some value. In the context of the video, taking the limit of the Riemann Sum as the number of intervals approaches infinity gives the exact area under the curve, thus defining the integral.

💡Definite Integral

A definite integral is the evaluation of the integral from a specific lower bound to an upper bound, represented as the area under the curve between these two points. The video introduces the definite integral as the sum of an infinite number of infinitesimally small areas (rectangles) under the curve from point A to point B.

💡Delta X

Delta X represents the width of each subinterval in the Riemann Sum. In the video, it is defined as the change in x, or the distance between consecutive sample points in the partition of the interval from A to B. Delta X becomes very small as the number of subintervals increases, leading to a more accurate approximation of the integral.

💡Height

Height in the context of the video refers to the value of the function at a specific point within each subinterval of the Riemann Sum. The height of the rectangle is determined by the function value at a chosen sample point within each subinterval, which is then multiplied by the width (Delta X) to get the area of the rectangle.

💡Summation

Summation is the process of adding a sequence of numbers or quantities. In the video, summation is used to combine the areas of all the rectangles in the Riemann Sum. The sum of these areas, as the number of rectangles approaches infinity, leads to the calculation of the integral.

💡Interval

An interval is a range of values between two endpoints. In the video, intervals are used to break down the area under the curve into smaller sections for the Riemann Sum. The interval from A to B is divided into smaller subintervals, each contributing to the overall area approximation.

💡Infinite Series

An infinite series is a sum of an infinite sequence of terms. The video explains that the exact area under the curve can be found by taking the limit of an infinite series of Riemann Sums. This series converges to the value of the definite integral.

💡Trapezoid

A trapezoid is a quadrilateral with at least one pair of parallel sides. The video uses the example of finding the area under a linear function, which forms a trapezoid. The area of the trapezoid is calculated by averaging the lengths of the two parallel sides (bases) and multiplying by the height (width of the interval).

💡Semicircle

A semicircle is half of a circle. The video includes an example of finding the area under the curve of a semicircle. The area is calculated using the formula for the area of a circle, divided by two. The video illustrates this with a function that represents the upper half of a circle.

💡Net Signed Area

Net signed area refers to the total area under the curve, accounting for areas above and below the x-axis. In the video, areas below the x-axis are considered negative, while areas above are positive. The net signed area is the sum of these positive and negative areas.

Highlights

Introduction to the concept of integrating area and finding the area under a curve.

Explanation of using Riemann sums to approximate the area under a curve.

Discussion on the flexibility of choosing any point within an interval for integration.

Breaking down the process of integration into smaller intervals.

Definition of a subinterval and choosing a point within it for the Riemann sum.

Visual representation of constructing rectangles to estimate area under the curve.

Calculation of the area of a rectangle using function value and change in X.

Transition from Riemann sum to the definition of a definite integral.

Formulation of the integral as an infinite summation.

Properties of definite integrals, such as integral from a to a being zero.

Explanation of the negation property when switching the limits of integration.

Ability to factor out constants from integrals.

Breaking up integrals into pieces for calculation.

Clarification on the absence of product and quotient rules in integration.

Application of geometric area formulas to calculate the integral of a constant function.

Calculation of the integral of a linear function and its area as a trapezoid.

Integration of a function resulting in a semicircle and its area calculation.

Handling of areas below the x-axis as negative in integral calculations.

Combining integrals from different intervals to find the net signed area.

Special case of integral from a point to itself resulting in zero area.

Transcripts

Browse More Related Video

Calculus 1: Definition of the Integral Examples

AP Calculus AB: Lesson 6.2 Part 2 (Limit Definition of Definite Integral)

BusCalc 13.4 Riemann Sums

Math 1325 Lecture 13 2

What Is an Integral?

The Definite Integral Part III: Evaluating From The Definition