Calculus 1: Definition of the Integral Examples

Allen Tsao The STEM Coach
5 Aug 201917:39
EducationalLearning
32 Likes 10 Comments

TLDRThis video script delves into the concept of integrals through Riemann sums and the method of approximating areas under curves using rectangles. It explains different techniques such as left-hand, right-hand, midpoint, and trapezoid rules to estimate the area under a curve, using a parabola as an example. The instructor illustrates how to set up and calculate these sums, highlighting the importance of understanding these methods in calculus. The script also covers the theoretical computation of integrals by taking the limit as the number of rectangles approaches infinity, providing a foundational understanding of integrals beyond just antiderivatives.

Takeaways
  • 📚 The integral represents the area under a curve, which is a fundamental concept in calculus.
  • 📏 Riemann sums and sums of rectangles are methods to approximate the area under a curve by dividing it into smaller parts.
  • 🔍 There are different types of Riemann sums, including left-hand sums, right-hand sums, midpoint sums, and the trapezoid rule.
  • 📉 In left-hand sums, rectangles are constructed so that the left side touches the curve at a specific point.
  • 📈 For right-hand sums, the rectangles are positioned so that the right side touches the curve, potentially leading to larger or smaller rectangles.
  • 🎯 Midpoint sums use the value in the middle of each interval to determine the height of the rectangles.
  • 🕋 The trapezoid rule combines the left and right sums by averaging the heights and multiplying by the width of the interval.
  • 🔢 The script provides a detailed example of calculating the area under a parabola using different Riemann sum methods.
  • 📝 The video script also explains how to compute the integral using the definition of the integral, which involves taking the limit as the number of rectangles (n) approaches infinity.
  • 🧩 The summation formulas for the sum of cubes and the sum of the first n natural numbers are used in the process of taking the limit to find the integral.
  • 🛠 While the process is tedious and typically not used in practice, understanding the definition and concept of integrals through Riemann sums is important for a solid foundation in calculus.
Q & A

  • What does the integral represent in the context of calculus?

    -In calculus, the integral represents the area under a curve, which is a way to approximate the total quantity or accumulation of a variable over an interval.

  • What are the different methods to approximate the area under a curve using rectangles?

    -The different methods include the left-hand sum, right-hand sum, midpoint rule, and the trapezoid rule. Each method uses a different point on the curve to determine the height of the rectangles used to approximate the area.

  • How does the left-hand sum method work?

    -In the left-hand sum method, rectangles are constructed such that the left side of each rectangle touches the curve at a specific point, and the width of each rectangle is uniform across the interval.

  • Can you explain the right-hand sum method?

    -The right-hand sum method is similar to the left-hand sum, but the rectangles are constructed so that the right side of each rectangle touches the curve. This can result in rectangles of varying sizes depending on the function.

  • What is the midpoint rule for approximating the area under a curve?

    -The midpoint rule involves using the midpoint of each interval on the x-axis to determine the height of the rectangles. This method assumes that the midpoint value is representative of the entire interval's contribution to the area under the curve.

  • How does the trapezoid rule differ from the other methods?

    -The trapezoid rule differs by creating trapezoids instead of rectangles. It uses the average of the left and right y-values (or heights) at the ends of each interval and multiplies it by the width to find the area of each trapezoid.

  • Why might the left-hand sum, right-hand sum, and midpoint rule give different estimates for the area under a curve?

    -These methods give different estimates because they use different points on the curve to approximate the height of the rectangles. Since the curve's slope can vary, different points will yield different areas, leading to varying estimates.

  • What is the definition of an integral in terms of the limit of a sum?

    -The definition of an integral in terms of the limit of a sum involves taking the limit as the number of rectangles (n) goes to infinity. This process refines the approximation of the area under the curve by making the rectangles infinitely thin and closely packed.

  • Can you provide an example of how to compute an integral using the definition of the integral?

    -An example would be to split the interval [0, 1] into n rectangles and compute the sum of the areas of these rectangles using either the left-hand or right-hand sums. Then, take the limit as n approaches infinity to find the exact area under the curve.

  • What is the practical application of knowing how to compute integrals by hand using the definition of the integral?

    -While it's important to understand the conceptual basis of integrals as the limit of a sum, in practice, most integrals are computed using antiderivatives for efficiency. However, knowing the definition helps in understanding the fundamental concept of integration and can be useful in theoretical mathematics or when dealing with complex integrals that do not easily lend themselves to standard techniques.

Outlines
00:00
📊 Understanding Riemann Sums and Integrals as Area Under Curve

The first paragraph introduces the concept of integrals in calculus, specifically focusing on Riemann sums as a method to approximate the area under a curve. The explanation begins with the fundamental idea that integrals represent this area, and then delves into how to approximate it using rectangles. The instructor discusses different techniques for setting up these rectangles, such as left-hand sums, right-hand sums, midpoint sums, and the trapezoid rule, each varying in how the rectangles' heights are determined in relation to the curve. A visual example using a parabola from negative 3 to positive 3 is provided to illustrate the process of creating left-hand sum rectangles and calculating their combined area, which is used to approximate the integral of x^2 over the given interval.

05:02
📐 Calculating Different Types of Riemann Sums for a Parabola

This paragraph continues the discussion on approximating the area under a curve by calculating different types of Riemann sums for a parabola. It explains the process of finding the right-hand sum and midpoint sum, emphasizing the differences in how the rectangles are formed and their heights are determined. The right-hand sum involves rectangles where the right edge touches the curve, while the midpoint sum uses the middle of each interval. The instructor provides a step-by-step calculation for both methods, showing how to sum the areas of these rectangles to estimate the integral. Additionally, the trapezoid rule is introduced, which combines elements of both left and right sums to create trapezoids and then calculates their areas. The paragraph concludes with a comparison of the results from the different summing methods, noting that while they may yield different estimates, they all serve as valid approximations due to the nature of Riemann sums.

10:03
🔍 Detailed Calculation of Integral Using Limit Definition

The third paragraph presents a detailed process of computing an integral using the definition of a limit. The focus is on breaking down the area under a curve into an infinite number of rectangles and then taking the limit as the number of rectangles approaches infinity. The instructor demonstrates how to calculate the width and height of each rectangle for both left and right Riemann sums and then how to set up the summation formula. The summation of cubes is used as an example, with the formula for the sum of the first n cubes provided. The paragraph shows the algebraic manipulation required to simplify the expression and eventually take the limit as n approaches infinity, resulting in the exact value of the integral. The process is illustrated with specific examples, including the setup of the summation, the application of the summation formula, and the simplification steps leading to the final answer.

15:03
📚 Final Thoughts on Integral Calculation and Additional Resources

In the final paragraph, the instructor wraps up the discussion on integral calculation by emphasizing the importance of understanding the conceptual approach of using summations and limits to find integrals, despite the fact that antiderivatives are typically used in practice for their efficiency. The paragraph acknowledges the tedious nature of calculating integrals by hand using the limit definition but highlights its value for understanding the foundational concepts of calculus. The instructor also promotes their website as a resource for additional examples and practice problems, offering free access to over 400 calculus questions with step-by-step solutions. This provides viewers with a platform to further their understanding and practice of integrals and other calculus topics.

Mindmap
Keywords
💡Integral
An integral is a fundamental concept in calculus representing the area under a curve between two points. In the video, the integral is introduced as the primary subject of discussion, with the focus on approximating the area under a curve using different methods such as Riemann sums and sums of rectangles. The script uses the example of a parabola to illustrate how integrals can be calculated by breaking the area into rectangles and summing their areas.
💡Riemann Sums
Riemann sums are a method for approximating the definite integral of a function. The script explains that Riemann sums involve dividing the area under the curve into rectangles and summing their areas to estimate the total area. The video discusses three types of Riemann sums: left-hand sums, right-hand sums, and midpoint sums, each using a different point on the rectangle to approximate the function's value.
💡Left-Hand Sum
A left-hand sum is a specific type of Riemann sum where the value of the function at the left endpoint of each interval is used to determine the height of the rectangle. In the script, the left-hand sum is demonstrated with a visual example where rectangles are drawn touching the curve at the left point, and their areas are summed to approximate the integral.
💡Right-Hand Sum
A right-hand sum is another Riemann sum variant where the function's value at the right endpoint of each interval is used. The script contrasts the right-hand sum with the left-hand sum, showing how rectangles are drawn touching the curve at the right point and their areas are summed to estimate the integral, similar to the left-hand sum but with a different endpoint.
💡Midpoint Sum
The midpoint sum is a Riemann sum that uses the function's value at the midpoint of each interval to determine the height of the rectangle. The script describes this method as a balance between the left and right-hand sums, where rectangles are drawn with their heights based on the midpoint's function value, providing another way to approximate the integral.
💡Trapezoid Rule
The trapezoid rule is a method for approximating the integral of a function by summing the areas of trapezoids instead of rectangles. The script introduces this rule by showing how to create trapezoids between the left and right endpoints and calculating their areas. The example given in the script uses the trapezoid rule to estimate the area under a curve by averaging the left and right function values at each interval.
💡Summation
Summation is the mathematical operation of adding a sequence of numbers to find their total. In the context of the video, summation is used to calculate the total area under the curve by adding the areas of rectangles or trapezoids. The script provides examples of summation in the process of finding left-hand, right-hand, midpoint, and trapezoid rule approximations of the integral.
💡Limit
The limit is a concept in calculus that describes the value that a function or sequence approaches as the input approaches a certain point. The script discusses the concept of taking the limit as the number of rectangles (n) goes to infinity to find the exact value of the integral, which is a more precise way than approximating with finite rectangles.
💡Antiderivatives
Antiderivatives are functions that represent the reverse process of differentiation. While the script focuses on approximating integrals through summation methods, it mentions antiderivatives as the preferred method for finding integrals in practice. Antiderivatives provide a way to find the exact integral without the need for approximation.
💡Symmetry
Symmetry in the context of the video refers to the property of a function where its shape is mirrored around a central point or line. The script notes that due to symmetry in the example function, some of the approximation methods yield the same result, which simplifies the process of finding the integral.
Highlights

Integrals represent the area under a curve.

Approximating the area under a curve by splitting it into rectangles.

Different methods for setting up rectangles: left point, right point, midpoint, and trapezoid rule.

Demonstration of left-hand sum using rectangles that touch the curve at the left point.

Explanation of the right-hand sum with rectangles touching the curve at the right point.

Midpoint sum using the value in between the ends of the interval.

Trapezoid rule combines the left and right points to form trapezoids.

Estimates from different methods may vary but are acceptable as they are approximations.

Computing the integral using the definition involves setting up areas of n rectangles and taking the limit as n approaches infinity.

Example calculation of the integral using the definition with a specific function.

Use of summation formulas for the sum of cubes in the integral calculation.

Limit process in integral calculation simplifies to 1/4 when taking the limit as n approaches infinity.

Another integral calculation example with a different function and interval.

Application of the sum of odd n terms rule in the integral calculation.

Final result of the integral calculation is 8 after taking the limit.

The importance of knowing different methods for integrals in calculus courses.

Practical application of antiderivatives in integrals instead of the tedious definition method.

Resource availability for more examples and step-by-step solutions on the instructor's website.

Transcripts

Browse More Related Video

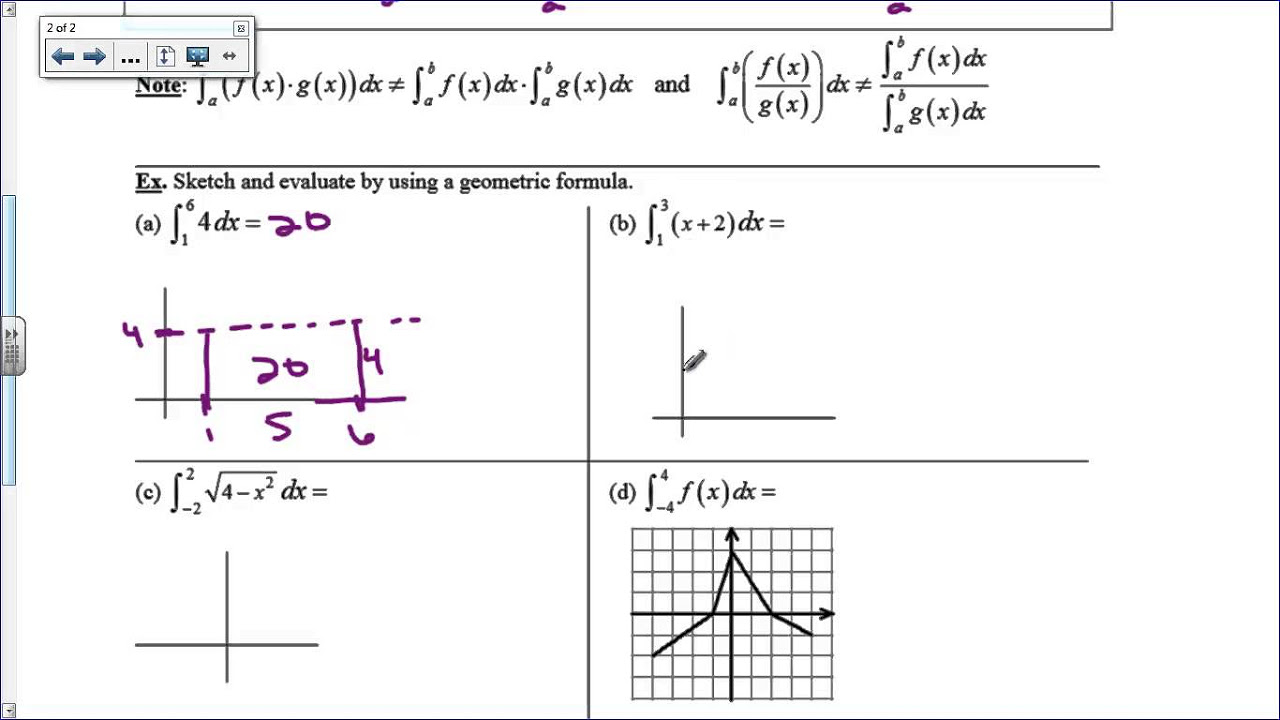
Riemann Sums and Definite Integrals

Business Calculus - Math 1329 - Section 5.3 - The Definite Integral and Fundamental Thm of Calculus

BusCalc 13.4 Riemann Sums

Definite integral as the limit of a Riemann sum | AP Calculus AB | Khan Academy

Riemann Sums

AP Calculus AB - 6.2 Approximating Areas with Riemann Sums

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegralsRiemann SumsArea Under CurveCalculus TutorialLeft Hand RuleRight Hand RuleMidpoint RuleTrapezoid RuleSummation FormulasLimit Definition